Embeddings in Euclidean space: an introduction to their classification
(→Introduction) |
Askopenkov (Talk | contribs) (→Codimension 1 embeddings) |
||
| (329 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{ | + | {{Authors|Askopenkov}} |
== Introduction == | == Introduction == | ||
<wikitex>; | <wikitex>; | ||
| − | This page is intended not only for specialists in embeddings, but also for mathematicians from other areas who want | + | This page is intended not only for specialists in embeddings, but also for mathematicians from other areas who want to apply or to learn the theory of embeddings. |
| − | to apply or to learn the theory of embeddings. | + | Unless otherwise indicated, the word `isotopy' means [[Isotopy|`ambient isotopy']] on this page; the definitions of these terms are given in \cite[$\S$1]{Skopenkov2016i}. |
| − | + | {{beginthm|Remark|(Some motivations)}}\label{r:zee} | |
| − | * '' | + | Three important classical problems in topology are the following, cf. \cite[p. 3]{Zeeman1993}. |
| − | * ''Embedding Problem'': Find the least dimension $m$ such that given | + | * ''The Manifold Problem'': Classify $n$-manifolds. |
| − | * ''Knotting Problem'': Classify [[Embedding_(simple_definition)|embeddings]] of a given | + | * ''The Embedding Problem'': Find the least dimension $m$ such that a given manifold admits an [[Embedding_(simple_definition)|embedding]] into $m$-dimensional Euclidean space $\Rr^m$. |
| − | <!-- | + | * ''The Knotting Problem'': Classify [[Embedding_(simple_definition)|embeddings]] of a given manifold into another given manifold up to isotopy. |
| − | See | + | The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, <!--E. van Kampen, K. Kuratowski, S. MacLane,--> L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others. |
| − | {{beginthm|Remark}}\label{r:oth} Of course there are other important problems of geometric topology. | + | |
| + | The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark \ref{s:list} below). | ||
| + | See also the [[Wikipedia:Knot_theory|Wikipedia article on knot theory]] and \cite[Example 2.3]{Skopenkov2016t}, \cite[$\S$1]{Takase2006}. | ||
| + | {{endthm}} | ||
| + | <!-- {{beginthm|Remark}}\label{r:oth} Of course there are other important problems of geometric topology. | ||
Some of them are interesting because they come from applications (notably problems on vector fields on manifolds). | Some of them are interesting because they come from applications (notably problems on vector fields on manifolds). | ||
Some of them proved to be useful for many other problems, and so gradually became interesting in themselves (notably homotopy-theoretic problems). | Some of them proved to be useful for many other problems, and so gradually became interesting in themselves (notably homotopy-theoretic problems). | ||
| − | {{endthm}} | + | {{endthm}} |
| + | --> | ||
| + | |||
| + | This article gives a short guide to the problem of classifying embeddings of closed manifolds $N$ into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark \ref{r:zee} for embeddings of general manifolds $N$ into $\R^m$ or $S^m$). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of $N$ are isotopic.In Section 3, we establish notation and conventions <!-- and give references to other pages on the Knotting Problem, to which this page serves as an introduction.--> | ||
| + | and in Section 4, we continue by introducing the connected sum operation for embeddings. | ||
| + | We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6. | ||
| + | |||
| + | The most interesting and much studied case of embeddings concerns classical knots, which are embeddings $S^1\to S^3$, or more generally, | ||
| + | [[Knots,_i.e._embeddings_of_spheres#Codimension_2_knots|codimension 2 embeddings of spheres]]. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see [[Wikipedia:Knot_theory|Wikipedia article on knot theory]] and $\S$\ref{s:c2} for more information. | ||
| + | |||
| + | The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which ''readily calculable'' classification results (see Remark \ref{compl}) describing all isotopy classes for embeddings of a closed manifold into Euclidean space $\Rr^m$ are known. Such classification results are presented on the pages listed in Remark \ref{s:list}, in [[#Unknotting_theorems|$\S$\ref{s:ut}]] and in [[#Codimension_1_embeddings|$\S$\ref{s:c1}]]. | ||
| + | <!-- (either in direct form or in the form of more general ''readily calculable'' classification, see Remark \ref{compl}). [[#References to information on the classification of embeddings|]] --> | ||
| + | The statements of those results, although not the proofs, are simple and accessible to non-specialists. | ||
| + | This page and the pages listed in Remark \ref{s:list} concern only such classification results. | ||
| + | As a consequence, we leave aside a large body of work, especially but not only in codimension 2. | ||
| + | |||
| + | The results and remarks given below show the following: | ||
| + | *For a fixed $N$, the more $m$ decreases from $2n$ towards $n+3$, the more complicated the classification of embeddings of $N$ into $\Rr^m$ becomes. | ||
| + | *The complete readily calculable classification of embeddings into $\Rr^m$ of closed connected $n$-manifolds is non-trivial and presently accessible only for $n+3\le m\le 2n$ or for $m=n+1\ge4$; the lowest dimensional cases, i.e. all such pairs $(m,n)$ with $n\le4$, are [[3-manifolds_in_6-space|(6,3)]], (4,3), [[Embeddings_just_below_the_stable_range:_classification|(8,4)]], [[4-manifolds_in_7-space|(7,4)]], (5,4). For information on the cases (6,3), (8,4), (7,4) see \cite{Skopenkov2016t}, \cite{Skopenkov2016e}, \cite{Skopenkov2016f}. | ||
| + | |||
| + | {{beginthm|Remark|(Readily calculable classification)}}\label{compl} The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See \cite[Preface]{Graham&Knuth&Patashnik89} for discussion of similar issues. To a first approximation, a classification of embeddings $N\to\Rr^m$ is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number $m$ and the manifold $N$. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside. | ||
| + | <!-- Mathematicians often discuss what is more useful answer, what is less useful, and what us being useful. | ||
| + | This remark does not intend to give a full description of `readily calculable', but is rather an invitation to further pursue the reflections by Wall (see (c) below), Graham, Knuth, Patashnik , and others. | ||
| + | An `explicit' classification of embeddings for a particular manifold is a clasification in terms of integers (see (i) below; this includes finitely generated abelian groups and other objects `easily' characterized by integers). (given by invariant or a collection of invariants) --> | ||
| + | |||
| + | Since this is a paper on the Knotting Problem of Remark \ref{r:zee}, below `classification' means `classification of embeddings of a manifold $N$ into $\Rr^m$ up to isotopy' (except in (d); some comments like (a) apply for more general classification problems). | ||
| + | |||
| + | (a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is. | ||
| + | |||
| + | (b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold $N$ is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate. | ||
| + | <!-- (c) If a result requires several pages of formulation, several dozen pages of a proof, and recovers only explicit results which can be obtained by much simpler methods, then we would not regard this result as readily calculable. E.g. I regard the classifications given by [[High_codimension_links#Classification_in_the_metastable_range|the Haefliger-Zeeman Theorem]] \cite[Theorem 4.1]{Skopenkov2016h} as readily calculable only because it has stronger explicit corollaries than classification of embeddings $S^n\sqcup S^n\to\Rr^m$ for $m\ge2n+1$. | ||
| + | A readily calculable classification is a `final result' accessible to non-specialists, while an abstract classification is a less accessible `tool' (which might or night not be useful depending on whether the tool gives explicit result). (see also \cite[$\S$6]{Goodwillie&Klein2015}; the relation of section 6 to spaces of Poincaré embeddings is explained in our introduction to that paper) | ||
--> | --> | ||
| − | The | + | (c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See \cite[Corollary 11.3.1]{Wall1999} and a simpler exposition in \cite[pp. 263-267]{Cencelj&Repovs&Skopenkov2004}. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' \cite[p. 119]{Wall1999}. An analogue of this result for classification is \cite[the Browder-Wall Theorem 9]{Cencelj&Repovs&Skopenkov2004}. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to \cite[Corollary 11.3.3]{Wall1999} as exposed in \cite[pp. 265-267]{Cencelj&Repovs&Skopenkov2004}. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see \cite{Skopenkov2016t}, \cite{Skopenkov2016f}) have been obtained by applying Kreck's modified surgery theory, see (d). |
| − | + | (d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark \ref{r:zee}. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory \cite{Kreck1999} was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see \cite{Kreck1999}. <!-- In our understanding, the Homeomorphism Problem of Remark \ref{r:zee} asks for classification up to CAT homeomorphism for CAT = TOP, PL or DIFF. The results for the three categories are closely related, although not completely parallel. Here we only consider smooth category. $I(H^*, ch)$-Poincaré-manifolds, as defined in \cite[$\S$ 3]{Kreck2001?} would be ``readily classifiable'' by definition and indeed | |
| − | + | see \cite{Wall1999} AS: deleted because no reference to a page or a section is given. | |
| − | + | one knows the classification up to homotopy, the computation of the normal invariants, the action of the L-groups on the structure set and the action of the group of homotopy self-equivalences on the structure set | |
| − | + | AS: deleted as a set of technicalities not relevant here | |
| + | The Browder-Novikov-Wall theorem of 1960s classifies higher-dimensional manifolds. (The statement in terms of Poincar\'e complexes is given e.g. in \cite{Wall1999}.) | ||
| + | --> | ||
| − | + | (i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. [[High_codimension_links#Classification_in_the_metastable_range|the Haefliger-Zeeman Theorem]] \cite[Theorem 4.1]{Skopenkov2016h} classifies links in terms of (stable) [[Wikipedia:Homotopy_groups_of_spheres|homotopy groups of spheres]]. These groups are not known in general. However, these groups are described for many particular cases which give [[High_codimension_links#Introduction|the table]] \cite[$\S$1]{Skopenkov2016h}, and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification. | |
| − | <!-- | + | <!-- only the left two columns of the table are recovered by easier proofs, |
| + | DC: This is both because of the central role of stable homotopy groups of spheres in topology and because stable homotopy theory is a generalised homology theory, hence in principle (and often in practice) it is amenable to algebraic calculations. | ||
| + | AS: Something having the central role does not mean that this something is amenable to algebraic calculations (see e.g. the Manifold Problem above). It should be justified by references that if something is (just) a generalised homology theory, then this something in principle (and often in practice) it is amenable to algebraic calculations. | ||
| + | Most of the explicit corollaries of the Haefliger-Weber Theorem are recovered by easier proofs. --> | ||
| − | + | (ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey \cite[$\S$5]{Skopenkov2006} and \cite{Haefliger1963}, \cite{Weber1967}, \cite{Skopenkov2002}. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies | |
| − | + | * the [[Embeddings_just_below_the_stable_range:_classification#Classification_further_below_the_stable_range|Becker-Glover Theorem]] \cite[Theorem 6.5]{Skopenkov2016e} and the [[Knotted_tori#Classification|classification of knotted tori]] \cite[Theorem 3.2]{Skopenkov2016k}, which I regard as readily calculable classifications; | |
| + | * the independence of the classification of embeddings of the smooth or PL structure on the manifold; | ||
| + | * the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) \cite[Theorem 1.1 and text after Theorem 1.4]{Cadek&Krcal&Vokrınek2013}. | ||
| − | + | (iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem \cite{Weiss96}, \cite{Weiss99}, \cite{Goodwillie&Weiss1999}. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings $S^1\to\Rr^n$ \cite{Weiss}, \cite{Arone&Turchin2014}. | |
| − | + | {{endthm}} | |
| − | + | <!-- | |
| + | DC: Another example of an important theorem about classifying embeddings, which has not lead to readily calculable classifications is the Haefliger-Weber Theorem. | ||
| + | AS it did, see (ii). | ||
| + | Then there is the interesting work of Goodwille-Weiss on the calculus of embeddings \cite{Weiss96}, \cite{Goodwillie&Weiss1999}. So far this approach has not lead to new complete readily calculable classification results. | ||
| + | DC: but this was not the main intention of that theory, which has many applications, including | ||
| + | give a modern abstract proof of the Haefliger-Weber Theorem and explicit results on the higher homotopy groups of the space of embeddings $S^1\to\Rr^n$ \cite{Weiss}. | ||
| + | AS: References to many applications should be given. For me, a more complicated proof of a classical result is not an application (although this does not mean that the more complicated proof is completely useless). | ||
| + | For me, higher homotopy groups of the space of embeddings $S^1\to\Rr^n$ is a theory in itself not an application. You might have a different notion of what is an application, and this notion would probably prevail over mine. Please explain this notion. | ||
| − | The | + | `The modern world is full of theories which are proliferating at a wrong level of generality, we're so '''good''' at theorizing, and one theory spawns another, there's a whole industry of abstract activity which people mistake for thinking.' \cite{Murdoch1985}. |
| + | --> | ||
| − | {{beginthm|Remark|( | + | {{beginthm|Remark|(Embeddings into Euclidean space and the sphere)}}\label{spheu} |
| − | + | (a) The embeddings $f,g:S^1\to\Rr^2$ given by $f(x,y)=(x,y)$ and $g(x,y)=(x,-y)$ are not isotopic | |
| − | [[Wikipedia: | + | (because they have distinct turning numbers; readers not familiar with turning number as defined in the [[Wikipedia:Winding_number#Turning_number|Wikipedia article on turning number]] can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of $S^1$ into $S^2$ are isotopic, see Theorem \ref{t:sch}.a and below it. |
| − | + | ||
| − | + | ||
| − | + | (b) For $m\ge n+2$ the classifications of embeddings of compact $n$-manifolds into $S^m$ and into $\Rr^m$ are the same. More precisely, for all integers $m,n$ such that $m\ge n+2$, and for every $n$-manifold $N$, the map $i_* : E_{\Rr^m}(N)\to E_{S^m}(N)$ between the sets of isotopy classes of embeddings $N\to \Rr^m$ and $N\to S^m$, | |
| + | which is induced by composition with the inclusion $i \colon \Rr^m \to S^m$, is a bijection. | ||
| + | |||
| + | Let us prove part (b). Since $n < m$, after a small isotopy an embedding $N \to S^m$ missed the point at infinity and so lies in $i(\Rr^m) \subset S^m$. | ||
| + | Hence $i_*$ is onto. | ||
| + | To prove that $i_*$ is injective, it suffices to show that if the compositions with the inclusion $i:\Rr^m\to S^m$ of two embeddings $f,f':N\to\Rr^m$ of a compact $n$-manifold $N$ are isotopic, then $f$ and $f'$ are isotopic. For showing that assume that $i\circ f$ and $i\circ f'$ are isotopic. Then by general position $f$ and $f'$ are [[Isotopy#Other equivalence relations|non-ambiently isotopic]]. Since every non-ambient isotopy extends to an isotopy \cite[Theorem 1.3]{Skopenkov2016i}, $f$ and $f'$ are isotopic. | ||
{{endthm}} | {{endthm}} | ||
| + | <!-- For a [[Embeddings_in_Euclidean_space:_plan_and_convention#Notation and conventions|notation and conventions]] used below on this page, we refer to \cite[$\S$3]{Skopenkov2016p}.--> | ||
| − | {{beginthm|Remark|( | + | {{beginthm|Remark|(References to information on the classification of embeddings)}}\label{s:list} |
| − | + | The first list is structured by the dimension of the source manifold and the target Euclidean space: | |
| − | + | * [[Embeddings just below the stable range: classification]] \cite{Skopenkov2016e} | |
| − | + | * [[3-manifolds in 6-space]] \cite{Skopenkov2016t} | |
| + | |||
| + | * [[4-manifolds in 7-space]] \cite{Skopenkov2016f} | ||
| + | |||
| + | Information structured by the `complexity' of the source manifold: | ||
| + | |||
| + | * [[Knots, i.e. embeddings of spheres]] \cite{Skopenkov2016s} | ||
| + | |||
| + | * [[High codimension links]] \cite{Skopenkov2016h} | ||
| + | |||
| + | * [[Knotted tori]] \cite{Skopenkov2016k} | ||
| + | |||
| + | * [[Embeddings of manifolds with boundary: classification]] | ||
| + | |||
| + | For more information see e.g. \cite{Skopenkov2006}. | ||
| + | <!--[[Unknotting of projective spaces]] [[Link maps: classification]] | ||
| + | [[Immersions: classification]] | ||
| + | [[Some open problems]] --> | ||
{{endthm}} | {{endthm}} | ||
</wikitex> | </wikitex> | ||
| Line 59: | Line 130: | ||
If the category is omitted, then a result stated below holds in both the [[Wikipedia:Differential_manifold#Differentiable_functions|smooth]] and [[Wikipedia:Piecewise_linear_function|piecewise-linear]] (PL) category. | If the category is omitted, then a result stated below holds in both the [[Wikipedia:Differential_manifold#Differentiable_functions|smooth]] and [[Wikipedia:Piecewise_linear_function|piecewise-linear]] (PL) category. | ||
| − | {{beginthm|General Position Theorem|(\cite{Hirsch1976}, \cite{Rourke&Sanderson1972})}}\label{th1} | + | {{beginthm|General Position Theorem|(\cite[Theorem 3.5]{Hirsch1976}, \cite[Theorem 5.4]{Rourke&Sanderson1972})}}\label{th1} |
| − | For | + | For every compact $n$-manifold $N$ and $m\ge2n+2$, any two embeddings of $N$ into $\Rr^m$ are isotopic. |
{{endthm}} | {{endthm}} | ||
| − | The case $m\ge2n+2$ is called a `stable range'. | + | The case $m\ge2n+2$ is called a `stable range' (for the classification problem; for the existence problem the analogous result with $m\ge2n$ is called |
| + | [[Wikipedia:Whitney_embedding_theorem|strong Whitney embedding theorem]] \cite[Theorem 2.2.a]{Skopenkov2006}). | ||
| − | The restriction $m\ge2n+2$ in Theorem \ref{th1} is sharp for non-connected manifolds, as the [[ | + | The restriction $m\ge2n+2$ in Theorem \ref{th1} is sharp for non-connected manifolds, as the [[High_codimension_links#Examples|Hopf linking]] $S^n\sqcup S^n\to\Rr^{2n+1}$ shows \cite[Example 2.1]{Skopenkov2016h}. |
{{beginthm|Whitney-Wu Unknotting Theorem}}\label{th2} | {{beginthm|Whitney-Wu Unknotting Theorem}}\label{th2} | ||
| − | For | + | For every compact connected $n$-manifold $N$, $n\ge2$ and $m\ge2n+1$, any two embeddings of $N$ into $\Rr^m$ are isotopic. |
{{endthm}} | {{endthm}} | ||
| − | This is proved in \cite{Wu1958}, \cite{Wu1958a} and \cite{Wu1959} using Whitney trick | + | This is proved in \cite{Wu1958c} using ''the Whitney trick'' (see that reference or \cite[$\S$5]{Rourke&Sanderson1972}). See also \cite[Theorem 2.3 (H. Whitney, W.T. Wu)]{Smale1961}. |
| + | <!--\cite{Wu1958}, \cite{Wu1958a} and \cite{Wu1959} using ''the Whitney trick'' (see those references or --> | ||
All the three assumptions in this result are indeed necessary: | All the three assumptions in this result are indeed necessary: | ||
*the assumption $n\ge2$ because of the existence of non-trivial knots $S^1\to S^3$; | *the assumption $n\ge2$ because of the existence of non-trivial knots $S^1\to S^3$; | ||
| − | *the connectedness assumption because of the existence of [[ | + | *the connectedness assumption because of the existence of [[High_codimension_links#Examples|the Hopf link]] \cite[Example 2.1]{Skopenkov2016h}; |
| − | *the assumption $m\ge2n+1$ because of the [[Embeddings just below the stable range#Hudson_tori|example of Hudson tori]] \cite{Skopenkov2016e}. | + | *the assumption $m\ge2n+1$ because of the [[Embeddings just below the stable range#Hudson_tori|example of Hudson tori]] \cite[Example 3.1]{Skopenkov2016e}. |
| − | {{beginthm|Unknotting Spheres Theorem}}\label{sph} | + | {{beginthm|Unknotting Spheres Theorem}}\label{sph} Assume that $m\ge n+3$ in the PL category or $2m\ge 3n+4$ in the smooth category. |
| − | For $N=S^n$, or even for $N$ | + | For $N=S^n$, or even for $N$ an integral homology $n$-sphere, any two embeddings of $N$ into $\Rr^m$ are isotopic. |
{{endthm}} | {{endthm}} | ||
| − | + | For $N=S^n$ this result is proved in \cite{Zeeman1960}, \cite[Corollary 2 of Theorem 9 in Chapter 4]{Zeeman1963} and in \cite[Existence Theorem (b) in p. 47]{Haefliger1961} in the PL and in the smooth category, respectively. For $N$ an integral homology $n$-sphere this result follows from \cite[Theorem 6.2]{Skopenkov2016e}. | |
| + | This result is also true for $m\ge n+3$ in the topological [[Wikipedia:Local flatness|locally flat]] category \cite[Corollary 9.3]{Stallings1963}, \cite[Theorem 1.1]{Gluck1963}; see also textbook \cite[Flattening Theorem 4.5.1]{Rushing1973} and \cite[Corollary 1.3]{Scharlemann1977}. | ||
<!--Here the local flatness assumption is indeed necessary.--> | <!--Here the local flatness assumption is indeed necessary.--> | ||
| − | The case $2m\ge 3n+4$ is called a `metastable range'. | + | The case $2m\ge 3n+4$ is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with $2m\ge3n+3$ \cite[$\S$2, $\S$5]{Skopenkov2006}). |
| − | Knots in codimension 2 and | + | Knots in codimension 2 and [[3-manifolds in 6-space#Examples|the Haefliger trefoil knot]] \cite[Example 2.1]{Skopenkov2016t} show that the dimension restrictions are sharp (even for $N=S^n$) in the Unknotting Spheres Theorem \ref{sph}. |
Theorems \ref{th2} and \ref{sph} may be generalized as follows. | Theorems \ref{th2} and \ref{sph} may be generalized as follows. | ||
{{beginthm|The Haefliger-Zeeman Unknotting Theorem}}\label{haze} | {{beginthm|The Haefliger-Zeeman Unknotting Theorem}}\label{haze} | ||
| − | For | + | For every $n\ge2k+2$, $m\ge2n-k+1$ and closed $k$-connected $n$-manifold $N$, any two embeddings of $N$ into $\Rr^m$ are isotopic. |
{{endthm}} | {{endthm}} | ||
| − | This | + | This is proved in \cite[Corollary 2 of Theorem 24 in Chapter 8]{Zeeman1963}, \cite[Existence Theorem (b) in p. 47]{Haefliger1961} in the PL or in the smooth category, respectively. The PL case uses the ideas of \cite{Penrose&Whitehead&Zeeman1961} and \cite{Irwin1965}; see also textbooks \cite[$\S$11]{Hudson1969}, \cite[$\S$5]{Rourke&Sanderson1972}. Theorem \ref{haze} remains true if ''$k$-connected'' is replaced by ''homologically $k$-connected'' (see $\S$\ref{s:nc} for the definition and |
| + | \cite[Theorem 6.2]{Skopenkov2016e} for [[Embeddings_just_below_the_stable_range:_classification#A_generalization_to_highly-connected_manifolds|justification]]). | ||
| − | + | Note that if $n\le2k+1$, then every closed $k$-connected $n$-manifold is topologically a sphere, so the analogue of Theorem \ref{haze} in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem \ref{sph}. | |
| − | + | Given Theorem \ref{haze} above, the case $m\ge2n-k+1$ can be called a `stable range for $k$-connected manifolds'. | |
| − | For generalizations of | + | For generalizations of Theorem \ref{haze} see [[Embeddings just below the stable range: classification|survey]] \cite{Skopenkov2016e} and \cite{Hudson1967}, \cite{Hacon1968}, \cite{Hudson1972}, \cite{Gordon1972}, \cite{Kearton1979}. See also Theorem \ref{t:sch}. |
</wikitex> | </wikitex> | ||
| Line 107: | Line 182: | ||
<wikitex>; \label{s:nc} | <wikitex>; \label{s:nc} | ||
| − | The following notations and conventions will be used in some other pages about embeddings, including those listed in | + | The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark \ref{s:list}. |
| − | For a manifold $N$ let $E^m_D(N)$ or $E^m_{PL}(N)$ denote the set of | + | Unless otherwise indicated, the word `isotopy' means [[Isotopy|`ambient isotopy']]; see definition in \cite[$\S$1]{Skopenkov2016i}. For a manifold $N$ let $E^m_D(N)$ or $E^m_{PL}(N)$ denote the set of |
[[Wikipedia:Differential_manifold#Differentiable_functions|smooth]] or [[Wikipedia:Piecewise_linear_function|piecewise-linear]] (PL) [[Embedding|embeddings]] $N\to S^m$ up to smooth or PL [[isotopy]]. | [[Wikipedia:Differential_manifold#Differentiable_functions|smooth]] or [[Wikipedia:Piecewise_linear_function|piecewise-linear]] (PL) [[Embedding|embeddings]] $N\to S^m$ up to smooth or PL [[isotopy]]. | ||
| − | If | + | If the category is omitted, then the result holds (or a definition or a construction is given) in both categories. |
| − | + | The sources of all embeddings are assumed to be compact. | |
Let $B^n$ be a closed $n$-ball in a closed connected $n$-manifold $N$. Denote $N_0:=Cl(N-B^n)$. | Let $B^n$ be a closed $n$-ball in a closed connected $n$-manifold $N$. Denote $N_0:=Cl(N-B^n)$. | ||
Let $\varepsilon(k):=1-(-1)^k$ be $0$ for $k$ even and $2$ for $k$ odd, so that $\Zz_{\varepsilon(k)}$ is $\Zz$ for $k$ even and $\Zz_2$ for $k$ odd. | Let $\varepsilon(k):=1-(-1)^k$ be $0$ for $k$ even and $2$ for $k$ odd, so that $\Zz_{\varepsilon(k)}$ is $\Zz$ for $k$ even and $\Zz_2$ for $k$ odd. | ||
| + | |||
| + | Denote by ${\rm pr}_k$ is the projection of a Cartesian product onto the $k$th factor. | ||
Denote by $V_{m,n}$ the Stiefel manifold of orthonormal $n$-frames in $\Rr^m$. | Denote by $V_{m,n}$ the Stiefel manifold of orthonormal $n$-frames in $\Rr^m$. | ||
| Line 123: | Line 200: | ||
We omit $\Zz$-coefficients from the notation of (co)homology groups. | We omit $\Zz$-coefficients from the notation of (co)homology groups. | ||
| − | For a manifold $P$ denote $H_s(P,\partial):=H_s(P,\partial P)$. | + | For a manifold $P$ with boundary $\partial P$ denote $H_s(P,\partial):=H_s(P,\partial P)$. |
| − | A closed manifold $N$ is called ''homologically $k$-connected'', if $N$ is connected and $H_i(N)=0$ for | + | A closed manifold $N$ is called ''homologically $k$-connected'', if $N$ is connected and $H_i(N)=0$ for every $i=1,\dots,k$. |
This condition is equivalent to $\tilde H_i(N)=0$ for each $i=0,\dots,k$, where $\tilde H_i$ are reduced homology groups. | This condition is equivalent to $\tilde H_i(N)=0$ for each $i=0,\dots,k$, where $\tilde H_i$ are reduced homology groups. | ||
| − | A pair $(N,\partial N)$ is called ''homologically $k$-connected'', if $H_i(N,\partial | + | A pair $(N,\partial N)$ is called ''homologically $k$-connected'', if $H_i(N,\partial)=0$ for every $i=0,\dots,k$. |
The ''self-intersection set'' of a map $f:X\to Y$ is $\Sigma(f):=\{x\in X\ :\ |f^{-1}fx|>1\}.$ | The ''self-intersection set'' of a map $f:X\to Y$ is $\Sigma(f):=\{x\in X\ :\ |f^{-1}fx|>1\}.$ | ||
| Line 133: | Line 210: | ||
For a smooth embedding $f:N\to\Rr^m$ denote by | For a smooth embedding $f:N\to\Rr^m$ denote by | ||
* $C_f$ the closure of the complement in $S^m\supset\Rr^m$ to a tight enough tubular neighborhood of $f(N)$ and | * $C_f$ the closure of the complement in $S^m\supset\Rr^m$ to a tight enough tubular neighborhood of $f(N)$ and | ||
| − | * $\nu_f:\partial C_f\to N$ the restriction of the linear normal bundle of $f$ to the | + | * $\nu_f:\partial C_f\to N$ the restriction of the linear normal bundle of $f$ to the subspace of unit length vectors identified with $\partial C_f$. |
| − | + | ||
| − | + | ||
| − | + | Denote by ${\rm i}:S^q\to S^m$ the standard embedding given by ${\rm i}(x_1,\ldots,x_{q+1})=(x_1,\ldots,x_{q+1},0,\ldots,0)$. The natural normal framing by vectors of length 1/2 on ${\rm i}$ defines the standard embedding ${\rm i}_{m,q}:D^{m-q}\times S^q\to S^m$. Denote by the same symbol ${\rm i}_{m,q}$ the restriction of ${\rm i}_{m,q}$ to $S^p\times S^q$ for any $p\le m-q-1$. | |
| − | < | + | <!--The natural ''standard embedding'' ${\rm i}:S^p\times S^q\to S^7$ for $p,q>0$, $p+q\le6$ is defined in \cite[$\S$2.1]{Skopenkov2015a}.--> |
| − | + | Denote by $\Sigma X$ the suspension of a space $X$. Denote by $\Sigma:\pi_q(S^n)\to\pi_{q+1}(S^{n+1})$ the suspension homomorphism. Recall that $\Sigma$ is an isomorphism for $q\le2n-2$. Let $\pi_k^S$ be any of the groups $\pi_{2k+2}(S^{k+2})\cong \pi_{2k+3}(S^{2k+3})\cong\ldots$ identified by the suspension isomorphism. | |
| − | + | Denote by $\Sigma^\infty:\pi_q(S^n)\to \pi_{q+M}(S^{n+M})=\pi_{q-n}^S$ the stable suspension homomorphisms, where $M$ is large. | |
| − | + | </wikitex> | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
== Embedded connected sum == | == Embedded connected sum == | ||
| − | <wikitex>; | + | <wikitex>; \label{s:sum} |
| − | Suppose that $N$ is a closed connected $n$-manifold and, if $N$ is orientable, an orientation of $N$ is chosen. | + | Suppose that $m\ge n+2$, $N$ is a closed connected $n$-manifold and, if $N$ is orientable, an orientation of $N$ is chosen. |
Let us define the ''embedded connected sum operation'' $\#$ of $E^m(S^n)$ on $E^m(N)$. | Let us define the ''embedded connected sum operation'' $\#$ of $E^m(S^n)$ on $E^m(N)$. | ||
Represent isotopy classes $[f]\in E^m(N)$ and $[g]\in E^m(S^n)$ by embeddings $f:N\to\Rr^m$ and $g:S^n\to\Rr^m$ whose images are contained in disjoint balls. | Represent isotopy classes $[f]\in E^m(N)$ and $[g]\in E^m(S^n)$ by embeddings $f:N\to\Rr^m$ and $g:S^n\to\Rr^m$ whose images are contained in disjoint balls. | ||
Join the images of $f,g$ by an arc whose interior misses the images. | Join the images of $f,g$ by an arc whose interior misses the images. | ||
| − | Let $[f]\#[g]$ be the isotopy class of the ''embedded connected sum'' of $f$ and $g$ along this arc (compatible with the orientation, if $N$ is orientable), cf. \cite[ | + | Let $[f]\#[g]$ be the isotopy class of the ''embedded connected sum'' of $f$ and $g$ along this arc (compatible with the orientation, if $N$ is orientable), cf. \cite[1.3, 1.4]{Haefliger1966}. |
This operation is well-defined, | This operation is well-defined, | ||
| − | <!--(If $m\le n+1$, this operation is not well-defined.)--> | + | <!--(If $m\le n+1$, this operation is not well-defined.) --> |
| − | i.e. the isotopy | + | i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes $[f]$ and $[g]$, and is independent of the choice of the path and of the representatives $f,g$. |
The proof of this fact is based on the construction of embedded connected sum of isotopies. | The proof of this fact is based on the construction of embedded connected sum of isotopies. | ||
Although this fact is presumably classical, a proof was not written, cf. \cite[Remark 2.3.a]{Skopenkov2015a}. | Although this fact is presumably classical, a proof was not written, cf. \cite[Remark 2.3.a]{Skopenkov2015a}. | ||
| Line 183: | Line 237: | ||
The proof for arbitrary closed connected $n$-manifold $N$ is analogous. | The proof for arbitrary closed connected $n$-manifold $N$ is analogous. | ||
| − | Moreover, for $m\ge n+3$ | + | Moreover, for $m\ge n+3$ embedded connected sum defines a group structure on $E^m(S^n)$ \cite[1.3-1.7]{Haefliger1966}, and an action $\#$ of $E^m(S^n)$ on $E^m(N)$. |
| − | and an action $\#$ of $E^m(S^n)$ on $E^m(N)$. | + | |
</wikitex> | </wikitex> | ||
| − | == | + | == Some remarks on codimension 2 embeddings == |
<wikitex>; \label{s:c2} | <wikitex>; \label{s:c2} | ||
| − | |||
| − | |||
| − | + | The case of embeddings of $S^n$ into $\Rr^{n+2}$ is the [[Knots,_i.e._embeddings_of_spheres#Codimension_2_knots|most extensively studied]] case of the Knotting Problem. | |
| − | + | In this case there is an overwhelming multitude of isotopy classes of embeddings. | |
| + | We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres. | ||
| + | Let $N$ be a closed connected $n$-manifold. | ||
| + | Using [[#Embedded_connected_sum|embedded connected sum]] ($\S$\ref{s:sum}) we can apparently produce an overwhelming multitude of embeddings $N\to\Rr^{n+2}$ from the overwhelming multitude of embeddings $S^n\to\Rr^{n+2}$. | ||
| + | (However, note that for $n=2$ there are embeddings $f:\Rr P^2\to S^4$ and $g_1,g_2:S^2\to S^4$ such that $g_1$ is not isotopic to $g_2$ but $f\#g_1$ is isotopic to $f\#g_2$ \cite[22 in p. 181]{Viro1973}.) | ||
One can also apply Artin's spinning construction \cite{Artin1928} $E^m(N)\to E^{m+1}(S^1\times N)$ for $m=n+2$. | One can also apply Artin's spinning construction \cite{Artin1928} $E^m(N)\to E^{m+1}(S^1\times N)$ for $m=n+2$. | ||
| − | |||
Thus the description of $E^{n+2}(N)$ is a very hard open problem. | Thus the description of $E^{n+2}(N)$ is a very hard open problem. | ||
| − | It would be interesting to give a more formal (e.g. algorithmic) illustration of hardness of this problem. | + | It would be interesting to give a more formal (e.g. algorithmic) |
| + | illustration of the hardness of this problem. | ||
| − | + | For studies of codimension 2 embeddings of manifolds up to the weaker relation of [[Isotopy|''concordance'']] see e.g. \cite{Cappell&Shaneson1974}. <!-- See open problems on classification `modulo knots' to appear below.--> | |
</wikitex> | </wikitex> | ||
| − | |||
| − | |||
== Codimension 1 embeddings == | == Codimension 1 embeddings == | ||
| Line 209: | Line 262: | ||
{{beginthm|Theorem}}\label{t:sch} | {{beginthm|Theorem}}\label{t:sch} | ||
| − | (a) | + | (a) Any two smooth embeddings of $S^n$ into $S^{n+1}$ are smoothly isotopic for every $n\ne3$. |
| − | (b) | + | (b) Any two smooth embeddings of $S^p\times S^{n-p}$ into $S^{n+1}$ are smoothly isotopic for every $2\le p\le n-p$. |
{{endthm}} | {{endthm}} | ||
| − | + | Part (a) is proved in \cite[Proposition D in $\S$9]{Milnor1965a} as an easy corollary of the $h$-cobordism theorem. | |
| + | Part (b) is proved in \cite[$\S$1, p. 447]{Lucas&Saeki2002} as a corollary of generalizations \cite{Kosinski1961}, \cite{Wall1965}, \cite{Goldstein1967}, \cite{Rubinstein1980}, \cite{Lucas&Neto&Saeki1996} of the following result. | ||
| + | <!-- \cite{Smale1961}, \cite{Smale1962a}, \cite{Barden1965}). \cite[Theorem 2B]{Wall1965}, \cite[Theorem 4.3]{Goldstein1967}--> | ||
| + | |||
| + | {{beginthm|Theorem|(Alexander torus theorem)}}\label{t:ale} \cite{Alexander1924} | ||
| + | For every embedding $S^1\times S^1\to S^3$ there is an autohomeomorphism $h$ of $S^1\times S^1$ such that $f\circ h$ extends to an embedding $D^2\times S^1\to S^3$. | ||
| + | {{endthm}} | ||
| + | |||
| + | Clearly, only the standard embedding extends to both. | ||
| + | |||
| + | {{beginthm|Remark}}\label{r:pltop} (a) (on PL topological category) | ||
| + | The analogue of Theorem \ref{t:sch}.a holds | ||
* for $n=1$ in the PL or topological category (Schöenfliess Theorem, 1912) \cite[$\S$1.8]{Rushing1973}. | * for $n=1$ in the PL or topological category (Schöenfliess Theorem, 1912) \cite[$\S$1.8]{Rushing1973}. | ||
* for $n=2$ in the PL category (Alexander Theorem, 1923) \cite[$\S$1.8]{Rushing1973}. | * for $n=2$ in the PL category (Alexander Theorem, 1923) \cite[$\S$1.8]{Rushing1973}. | ||
* for every $n$ in the topological [[Wikipedia:Local flatness|locally flat]] category (Brown-Mazur-Moise Theorem, 1960) \cite[Generalized Schöenfliess Theorem 1.8.2]{Rushing1973}. | * for every $n$ in the topological [[Wikipedia:Local flatness|locally flat]] category (Brown-Mazur-Moise Theorem, 1960) \cite[Generalized Schöenfliess Theorem 1.8.2]{Rushing1973}. | ||
| − | The famous counterexample to the analogue of | + | The famous counterexample to the analogue of Theorem \ref{t:sch}.a for $n=2$ in the topological category is [[Wikipedia:Alexander_horned_sphere|the Alexander horned sphere]], see the corresponding [[Wikipedia:Alexander_horned_sphere|Wikipedia article]]. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of $S^n$ into $S^{n+1}$ are isotopic for every $n\ge3$ (this is equivalent to the description of $E^{n+1}_{PL}(S^n)$). |
| − | (this is equivalent to the description of $E^{n+1}_{PL}(S^n)$). | + | <!-- Let $E^m_{PL,lf}(N)$ be the set of PL [[Wikipedia:Local flatness|locally flat]] embeddings $N\to S^m$ up to PL locally flat isotopy (see the corresponding [[Wikipedia:Local flatness|Wikipedia article]] for the definition). |
| + | Note that $E^{n+1}_{PL,lf}(S^p\times S^{n-p})$ can admit complete readily calculable classification even when $E^{n+1}_{PL,lf}(S^n)$ does not \cite{Goldstein1967}. | ||
| + | For more on higher-dimensional codimension 1 embeddings see e.g. \cite{Lucas&Saeki2002}.--> | ||
| − | + | (b) (some speculations) If the extension of the Alexander torus theorem \ref{t:ale} respects isotopy, the extension would give a 1-1 correspondence between $E^3(S^1\times S^1)$ and the union of $E^3(S^1)\times\Zz$ and $\Zz\times E^3(S^1)$ with `base points' $i\times0$ and $0\times i$ identified (where $i$ is the isotopy class of the standard inclusion $S^1\to\Rr^3$). So the description of $E^3(S^1\times S^1)$ would be as hopeless as that of $E^3(S^1)$. Thus the description of $E^3(N)$ for $N$ a [[2-manifolds#Orientable_surfaces|sphere with handles]] is apparently hopeless. | |
| − | + | {{endthm}} | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
</wikitex> | </wikitex> | ||
Latest revision as of 09:01, 6 November 2021
| This page has been accepted for publication in the Bulletin of the Manifold Atlas. |
|
The user responsible for this page is Askopenkov. No other user may edit this page at present. |
Contents |
1 Introduction
This page is intended not only for specialists in embeddings, but also for mathematicians from other areas who want to apply or to learn the theory of embeddings.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy' on this page; the definitions of these terms are given in [Skopenkov2016i,  1].
1].
Remark 1.1 (Some motivations). Three important classical problems in topology are the following, cf. [Zeeman1993, p. 3].
- The Manifold Problem: Classify
 -manifolds.
-manifolds.
- The Embedding Problem: Find the least dimension
 such that a given manifold admits an embedding into
such that a given manifold admits an embedding into  -dimensional Euclidean space
-dimensional Euclidean space  .
.
- The Knotting Problem: Classify embeddings of a given manifold into another given manifold up to isotopy.
The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others.
The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark 1.4 below).
See also the Wikipedia article on knot theory and [Skopenkov2016t, Example 2.3], [Takase2006,  1].
1].
This article gives a short guide to the problem of classifying embeddings of closed manifolds  into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds
into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds  into
into  or
or  ). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of
). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of  are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
The most interesting and much studied case of embeddings concerns classical knots, which are embeddings 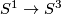 , or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and
, or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and  5 for more information.
5 for more information.
The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which readily calculable classification results (see Remark 1.2) describing all isotopy classes for embeddings of a closed manifold into Euclidean space  are known. Such classification results are presented on the pages listed in Remark 1.4, in
are known. Such classification results are presented on the pages listed in Remark 1.4, in  2 and in
2 and in  6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
The results and remarks given below show the following:
- For a fixed
 , the more
, the more  decreases from
decreases from  towards
towards 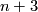 , the more complicated the classification of embeddings of
, the more complicated the classification of embeddings of  into
into  becomes.
becomes.
- The complete readily calculable classification of embeddings into
 of closed connected
of closed connected  -manifolds is non-trivial and presently accessible only for
-manifolds is non-trivial and presently accessible only for 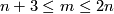 or for
or for 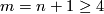 ; the lowest dimensional cases, i.e. all such pairs
; the lowest dimensional cases, i.e. all such pairs 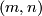 with
with 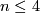 , are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
, are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
Remark 1.2 (Readily calculable classification). The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See [Graham&Knuth&Patashnik89, Preface] for discussion of similar issues. To a first approximation, a classification of embeddings 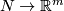 is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number
is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number  and the manifold
and the manifold  . This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
Since this is a paper on the Knotting Problem of Remark 1.1, below `classification' means `classification of embeddings of a manifold  into
into  up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
(a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is.
(b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold  is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
(c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See [Wall1999, Corollary 11.3.1] and a simpler exposition in [Cencelj&Repovs&Skopenkov2004, pp. 263-267]. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' [Wall1999, p. 119]. An analogue of this result for classification is [Cencelj&Repovs&Skopenkov2004, the Browder-Wall Theorem 9]. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to [Wall1999, Corollary 11.3.3] as exposed in [Cencelj&Repovs&Skopenkov2004, pp. 265-267]. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see [Skopenkov2016t], [Skopenkov2016f]) have been obtained by applying Kreck's modified surgery theory, see (d).
(d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark 1.1. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory [Kreck1999] was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see [Kreck1999].
(i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. the Haefliger-Zeeman Theorem [Skopenkov2016h, Theorem 4.1] classifies links in terms of (stable) homotopy groups of spheres. These groups are not known in general. However, these groups are described for many particular cases which give the table [Skopenkov2016h,  1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
(ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey [Skopenkov2006,  5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
- the Becker-Glover Theorem [Skopenkov2016e, Theorem 6.5] and the classification of knotted tori [Skopenkov2016k, Theorem 3.2], which I regard as readily calculable classifications;
- the independence of the classification of embeddings of the smooth or PL structure on the manifold;
- the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) [Cadek&Krcal&Vokrınek2013, Theorem 1.1 and text after Theorem 1.4].
(iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem [Weiss96], [Weiss99], [Goodwillie&Weiss1999]. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings 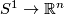 [Weiss], [Arone&Turchin2014].
[Weiss], [Arone&Turchin2014].
Remark 1.3 (Embeddings into Euclidean space and the sphere).
(a) The embeddings 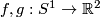 given by
given by 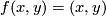 and
and 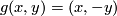 are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of
are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of  into
into  are isotopic, see Theorem 6.1.a and below it.
are isotopic, see Theorem 6.1.a and below it.
(b) For  the classifications of embeddings of compact
the classifications of embeddings of compact  -manifolds into
-manifolds into  and into
and into  are the same. More precisely, for all integers
are the same. More precisely, for all integers 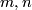 such that
such that  , and for every
, and for every  -manifold
-manifold  , the map
, the map 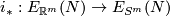 between the sets of isotopy classes of embeddings
between the sets of isotopy classes of embeddings 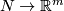 and
and 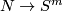 ,
which is induced by composition with the inclusion
,
which is induced by composition with the inclusion 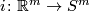 , is a bijection.
, is a bijection.
Let us prove part (b). Since 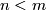 , after a small isotopy an embedding
, after a small isotopy an embedding  missed the point at infinity and so lies in
missed the point at infinity and so lies in 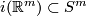 .
Hence
.
Hence  is onto.
To prove that
is onto.
To prove that  is injective, it suffices to show that if the compositions with the inclusion
is injective, it suffices to show that if the compositions with the inclusion 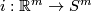 of two embeddings
of two embeddings 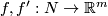 of a compact
of a compact  -manifold
-manifold  are isotopic, then
are isotopic, then  and
and  are isotopic. For showing that assume that
are isotopic. For showing that assume that 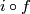 and
and  are isotopic. Then by general position
are isotopic. Then by general position  and
and  are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],
are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],  and
and  are isotopic.
are isotopic.
Remark 1.4 (References to information on the classification of embeddings). The first list is structured by the dimension of the source manifold and the target Euclidean space:
Information structured by the `complexity' of the source manifold:
For more information see e.g. [Skopenkov2006].
2 Unknotting theorems
If the category is omitted, then a result stated below holds in both the smooth and piecewise-linear (PL) category.
General Position Theorem 2.1 ([Hirsch1976, Theorem 3.5], [Rourke&Sanderson1972, Theorem 5.4]).
For every compact  -manifold
-manifold  and
and 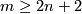 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
The case 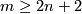 is called a `stable range' (for the classification problem; for the existence problem the analogous result with
is called a `stable range' (for the classification problem; for the existence problem the analogous result with 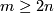 is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
The restriction 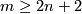 in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking
in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking 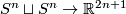 shows [Skopenkov2016h, Example 2.1].
shows [Skopenkov2016h, Example 2.1].
Whitney-Wu Unknotting Theorem 2.2.
For every compact connected  -manifold
-manifold  ,
, 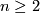 and
and 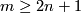 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Wu1958c] using the Whitney trick (see that reference or [Rourke&Sanderson1972,  5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
All the three assumptions in this result are indeed necessary:
- the assumption
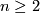 because of the existence of non-trivial knots
because of the existence of non-trivial knots 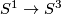 ;
;
- the connectedness assumption because of the existence of the Hopf link [Skopenkov2016h, Example 2.1];
- the assumption
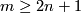 because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
Unknotting Spheres Theorem 2.3. Assume that  in the PL category or
in the PL category or 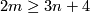 in the smooth category.
For
in the smooth category.
For 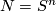 , or even for
, or even for  an integral homology
an integral homology  -sphere, any two embeddings of
-sphere, any two embeddings of  into
into  are isotopic.
are isotopic.
For 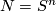 this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For
this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For  an integral homology
an integral homology  -sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for
-sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for  in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
The case 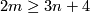 is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with
is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with 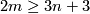 [Skopenkov2006,
[Skopenkov2006,  2,
2,  5]).
5]).
Knots in codimension 2 and the Haefliger trefoil knot [Skopenkov2016t, Example 2.1] show that the dimension restrictions are sharp (even for 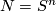 ) in the Unknotting Spheres Theorem 2.3.
) in the Unknotting Spheres Theorem 2.3.
Theorems 2.2 and 2.3 may be generalized as follows.
The Haefliger-Zeeman Unknotting Theorem 2.4.
For every 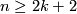 ,
, 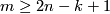 and closed
and closed  -connected
-connected  -manifold
-manifold  , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Zeeman1963, Corollary 2 of Theorem 24 in Chapter 8], [Haefliger1961, Existence Theorem (b) in p. 47] in the PL or in the smooth category, respectively. The PL case uses the ideas of [Penrose&Whitehead&Zeeman1961] and [Irwin1965]; see also textbooks [Hudson1969,  11], [Rourke&Sanderson1972,
11], [Rourke&Sanderson1972,  5]. Theorem 2.4 remains true if
5]. Theorem 2.4 remains true if  -connected is replaced by homologically
-connected is replaced by homologically  -connected (see
-connected (see  3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
Note that if 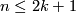 , then every closed
, then every closed  -connected
-connected  -manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
-manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
Given Theorem 2.4 above, the case 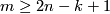 can be called a `stable range for
can be called a `stable range for  -connected manifolds'.
-connected manifolds'.
For generalizations of Theorem 2.4 see survey [Skopenkov2016e] and [Hudson1967], [Hacon1968], [Hudson1972], [Gordon1972], [Kearton1979]. See also Theorem 6.1.
3 Notation and conventions
The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark 1.4.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy'; see definition in [Skopenkov2016i,  1]. For a manifold
1]. For a manifold  let
let 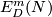 or
or 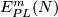 denote the set of
smooth or piecewise-linear (PL) embeddings
denote the set of
smooth or piecewise-linear (PL) embeddings 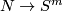 up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
The sources of all embeddings are assumed to be compact.
Let  be a closed
be a closed  -ball in a closed connected
-ball in a closed connected  -manifold
-manifold  . Denote
. Denote 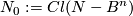 .
.
Let 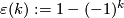 be
be  for
for  even and
even and  for
for  odd, so that
odd, so that 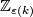 is
is  for
for  even and
even and  for
for  odd.
odd.
Tex syntax erroris the projection of a Cartesian product onto the
 th factor.
th factor.
Denote by 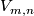 the Stiefel manifold of orthonormal
the Stiefel manifold of orthonormal  -frames in
-frames in  .
.
We omit  -coefficients from the notation of (co)homology groups.
-coefficients from the notation of (co)homology groups.
For a manifold  with boundary
with boundary 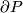 denote
denote 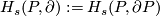 .
.
A closed manifold  is called homologically
is called homologically  -connected, if
-connected, if  is connected and
is connected and 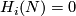 for every
for every 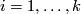 .
This condition is equivalent to
.
This condition is equivalent to 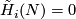 for each
for each 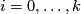 , where
, where 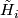 are reduced homology groups.
A pair
are reduced homology groups.
A pair 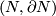 is called homologically
is called homologically  -connected, if
-connected, if  for every
for every 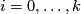 .
.
The self-intersection set of a map 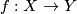 is
is 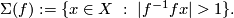
For a smooth embedding  denote by
denote by
-
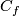 the closure of the complement in
the closure of the complement in 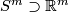 to a tight enough tubular neighborhood of
to a tight enough tubular neighborhood of  and
and
-
 the restriction of the linear normal bundle of
the restriction of the linear normal bundle of  to the subspace of unit length vectors identified with
to the subspace of unit length vectors identified with 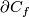 .
.
Tex syntax errorthe standard embedding given by
Tex syntax error. The natural normal framing by vectors of length 1/2 on
Tex syntax errordefines the standard embedding
Tex syntax error. Denote by the same symbol
Tex syntax errorthe restriction of
Tex syntax errorto
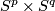 for any
for any 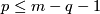 .
.
Denote by 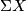 the suspension of a space
the suspension of a space  . Denote by
. Denote by  the suspension homomorphism. Recall that
the suspension homomorphism. Recall that  is an isomorphism for
is an isomorphism for 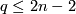 . Let
. Let 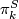 be any of the groups
be any of the groups 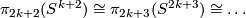 identified by the suspension isomorphism.
Denote by
identified by the suspension isomorphism.
Denote by 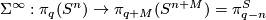 the stable suspension homomorphisms, where
the stable suspension homomorphisms, where  is large.
is large.
4 Embedded connected sum
Suppose that  ,
,  is a closed connected
is a closed connected  -manifold and, if
-manifold and, if  is orientable, an orientation of
is orientable, an orientation of  is chosen.
Let us define the embedded connected sum operation
is chosen.
Let us define the embedded connected sum operation 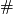 of
of  on
on  .
.
Represent isotopy classes ![[f]\in E^m(N)](/images/math/2/f/5/2f52f1701a659817e8b3dfbab85d0fef.png) and
and ![[g]\in E^m(S^n)](/images/math/0/0/3/003b3d9600f355a6b75926b59ee4b4c6.png) by embeddings
by embeddings  and
and 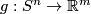 whose images are contained in disjoint balls.
Join the images of
whose images are contained in disjoint balls.
Join the images of 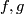 by an arc whose interior misses the images.
Let
by an arc whose interior misses the images.
Let ![[f]\#[g]](/images/math/b/5/0/b50485694938d441de43bc8d0d73e64c.png) be the isotopy class of the embedded connected sum of
be the isotopy class of the embedded connected sum of  and
and  along this arc (compatible with the orientation, if
along this arc (compatible with the orientation, if  is orientable), cf. [Haefliger1966, 1.3, 1.4].
is orientable), cf. [Haefliger1966, 1.3, 1.4].
This operation is well-defined,
i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes ![[f]](/images/math/d/d/4/dd43b82529dd8d403c1585c5d151a163.png) and
and ![[g]](/images/math/0/8/a/08a05aeade465b1c6abe241850947aab.png) , and is independent of the choice of the path and of the representatives
, and is independent of the choice of the path and of the representatives 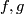 .
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for
.
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for 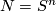 in [Skopenkov2015a,
in [Skopenkov2015a,  3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for
3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for  a point].
The proof for arbitrary closed connected
a point].
The proof for arbitrary closed connected  -manifold
-manifold  is analogous.
is analogous.
Moreover, for  embedded connected sum defines a group structure on
embedded connected sum defines a group structure on  [Haefliger1966, 1.3-1.7], and an action
[Haefliger1966, 1.3-1.7], and an action  of
of  on
on  .
.
5 Some remarks on codimension 2 embeddings
The case of embeddings of  into
into 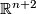 is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
Let  be a closed connected
be a closed connected  -manifold.
Using embedded connected sum (
-manifold.
Using embedded connected sum ( 4) we can apparently produce an overwhelming multitude of embeddings
4) we can apparently produce an overwhelming multitude of embeddings 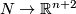 from the overwhelming multitude of embeddings
from the overwhelming multitude of embeddings  .
(However, note that for
.
(However, note that for  there are embeddings
there are embeddings 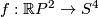 and
and 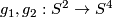 such that
such that 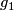 is not isotopic to
is not isotopic to 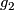 but
but 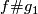 is isotopic to
is isotopic to 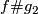 [Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928]
[Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928] 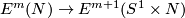 for
for 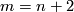 .
Thus the description of
.
Thus the description of 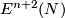 is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
For studies of codimension 2 embeddings of manifolds up to the weaker relation of concordance see e.g. [Cappell&Shaneson1974].
6 Codimension 1 embeddings
Theorem 6.1.
(a) Any two smooth embeddings of  into
into 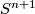 are smoothly isotopic for every
are smoothly isotopic for every  .
.
(b) Any two smooth embeddings of 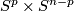 into
into 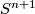 are smoothly isotopic for every
are smoothly isotopic for every 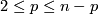 .
.
Part (a) is proved in [Milnor1965a, Proposition D in  9] as an easy corollary of the
9] as an easy corollary of the  -cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,
-cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,  1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
Theorem 6.2 (Alexander torus theorem). [Alexander1924]
For every embedding 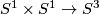 there is an autohomeomorphism
there is an autohomeomorphism  of
of 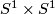 such that
such that  extends to an embedding
extends to an embedding 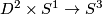 .
.
Clearly, only the standard embedding extends to both.
Remark 6.3. (a) (on PL topological category) The analogue of Theorem 6.1.a holds
- for
 in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,
in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,  1.8].
1.8].
- for
 in the PL category (Alexander Theorem, 1923) [Rushing1973,
in the PL category (Alexander Theorem, 1923) [Rushing1973,  1.8].
1.8].
- for every
 in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
The famous counterexample to the analogue of Theorem 6.1.a for  in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of
in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of  into
into 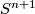 are isotopic for every
are isotopic for every  (this is equivalent to the description of
(this is equivalent to the description of 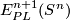 ).
).
(b) (some speculations) If the extension of the Alexander torus theorem 6.2 respects isotopy, the extension would give a 1-1 correspondence between 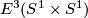 and the union of
and the union of 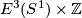 and
and 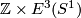 with `base points'
with `base points' 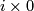 and
and  identified (where
identified (where  is the isotopy class of the standard inclusion
is the isotopy class of the standard inclusion 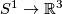 ). So the description of
). So the description of 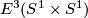 would be as hopeless as that of
would be as hopeless as that of 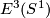 . Thus the description of
. Thus the description of 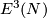 for
for  a sphere with handles is apparently hopeless.
a sphere with handles is apparently hopeless.
7 References
- [Alexander1924] J. W. Alexander, On the subdivision of 3-space by polyhedron, Proc. Nat. Acad. Sci. USA, 10, (1924) 6–8. Zbl 50.0659.01
- [Arone&Turchin2014] G. Arone and V. Turchin, On the rational homology of high-dimensional analogues of spaces of long knots, Geom. and Topol. 18 (2014), 1261-1322.
- [Artin1928] E. Artin, Zur Isotopie zwei-dimensionaler Flaechen im
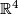 , Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
, Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
- [Cadek&Krcal&Vokrınek2013] M. Cadek, M. Kr\v c\'al, and L. Vok\v r\'ınek. Algorithmic solvability of the lifting-extension problem, arXiv:1307.6444.
- [Cappell&Shaneson1974] S. E. Cappell and J. L. Shaneson, The codimension two placement problem and homology equivalent manifolds, Ann. of Math. (2) 99 (1974), 277–348. MR0339216 (49 #3978) Zbl 0279.57011
- [Cencelj&Repovs&Skopenkov2004] M. Cencelj, D. Repov\v s and A. Skopenkov, On the Browder-Levine-Novikov embedding theorems, Proc. of the Steklov Math. Inst. 247 (2004) 280-290.
- [Gluck1963] H. Gluck, Unknotting
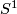 in
in 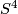 ., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
- [Goldstein1967] R. Z. Goldstein, Piecewise linear unknotting of
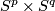 in
in 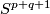 , Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
, Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
- [Goodwillie&Weiss1999] T. Goodwillie and M. Weiss, Embeddings from the point of view of immersion theory, II, Geometry and Topology, 3 (1999), 103-118. MR1694812 (2000c:57055a) Zbl 0927.57028
- [Gordon1972] C. Gordon, Embedding piecewise linear manifolds with boundary., Proc. Camb. Philos. Soc. 72 (1972), 21-25. MR0295359 (45 #4425) Zbl 0236.57009
- [Graham&Knuth&Patashnik89] R. Graham, D. Knuth, and O. Patashnik, Concrete Mathematics: A Foundation for Computer Science, Addison–Wesley, first published in 1989, ISBN 0-201-55802-5, https://www.csie.ntu.edu.tw/~r97002/temp/Concrete%20Mathematics%202e.pdf.
- [Hacon1968] D. Hacon, Embeddings of
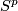 in
in 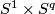 in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
- [Haefliger1961] A. Haefliger, Plongements différentiables de variétés dans variétés., Comment. Math. Helv.36 (1961), 47-82. MR0145538 (26 #3069) Zbl 0102.38603
- [Haefliger1963] A. Haefliger, Plongements différentiables dans le domain stable., Comment. Math. Helv.37 (1963), 155-176.
- [Haefliger1966] A. Haefliger, Differential embeddings of
 in
in  for
for  , Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
- [Hirsch1976] M. W. Hirsch, Differential topology., Graduate Texts in Mathematics, No. 33. Springer-Verlag., New York-Heidelberg, 1976. MR0448362 (56 #6669) Zbl 0356.57001
- [Hudson1967] J. Hudson, Piecewise linear embeddings, Ann. of Math. (2) 85 (1967) 1–31. MR0215308 (35 #6149) Zbl 0153.25601
- [Hudson1969] J. F. P. Hudson, Piecewise linear topology, W. A. Benjamin, Inc., New York-Amsterdam, 1969. MR0248844 (40 #2094) Zbl 0189.54507
- [Hudson1972] J. Hudson, Embeddings of bounded manifolds., Proc. Camb. Philos. Soc. 72 (1972), 11-20. MR0298679 (45 #7728) Zbl 0241.57006
- [Irwin1965] M. Irwin, Embeddings of polyhedral manifolds, Ann. of Math. (2) 82 (1965) 1–14. MR0182978 (32 #460) Zbl 0132.20003
- [Kearton1979] C. Kearton, Obstructions to embedding and isotopy in the metastable range, Math. Ann. 243 (1979), 103-113. MR0543720 (82k:57012) Zbl 0401.57033
- [Kosinski1961] A. Kosinski, On Alexander's theorem and knotted tori, In: Topology of 3-Manifolds, Prentice-Hall, Englewood Cliffs, Ed. M.~K.~Fort, N.J., 1962, 55--57. Cf. Fort1962.
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Lucas&Neto&Saeki1996] L. A. Lucas, O. M. Neto and O. Saeki, A generalization of Alexander's torus theorem to higher dimensions and an unknotting theorem for
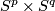 embedded in
embedded in  , Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
, Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
- [Lucas&Saeki2002] L. A. Lucas and O. Saeki, Embeddings of
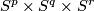 in
in 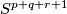 , Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
, Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
- [Milnor1965a] J. Milnor, Lectures on the
 -cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
-cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
- [Penrose&Whitehead&Zeeman1961] R. Penrose, J. Whitehead and E. Zeeman, Imbedding of manifolds in Euclidean space., Ann. of Math. 73 (1961) 613–623. MR0124909 (23 #A2218) Zbl 0113.38101
- [Rourke&Sanderson1972] C. Rourke and B. Sanderson, Introduction to piecewise-linear topology., Ergebnisse der Mathematik und ihrer Grenzgebiete. Band 69. Berlin-Heidelberg-New York: Springer-Verlag. VIII, 1972. MR0665919 (83g:57009) Zbl 0254.57010
- [Rubinstein1980] J.H. Rubinstein, Dehn’s lemma and handle decompositions of some 4-manifolds, Pacific J. Math., 86 (1980), 565-569. MR590570 (81 #M57011) Zbl 0446.57025
- [Rushing1973] T. Rushing, Topological embeddings., Pure and Applied Mathematics, 52. New York-London: Academic Press. XIII, 1973. MRMR0348752 (50 #1247) Zbl 0295.57003
- [Scharlemann1977] M. Scharlemann, Isotopy and cobordism of homology spheres in spheres., J. Lond. Math. Soc., II. Ser. 16 (1977), 559-567. MRMR0464246 (57 #4180) Zbl 0375.57003
- [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015a] A. Skopenkov, A classification of knotted tori, Proc. A of the Royal Society of Edinburgh, 150:2 (2020), 549-567. Full version: http://arxiv.org/abs/1502.04470
- [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
- [Skopenkov2016f] A. Skopenkov, 4-manifolds in 7-space, to appear in Bull. Man. Atl.
- [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
- [Skopenkov2016i] A. Skopenkov, Isotopy, submitted to Bull. Man. Atl.
- [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
- [Skopenkov2016s] A. Skopenkov, Knots, i.e. embeddings of spheres, preprint.
- [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.
- [Smale1961] S. Smale, Generalized Poincaré's conjecture in dimensions greater than four, Ann. of Math. (2) 74 (1961), 391–406. MR0137124 (25 #580) Zbl 0099.39202
- [Stallings1963] J. Stallings, On topologically unknotted spheres., (1963). MRMR0149458 (26 #6946) Zbl 0121.18202
- [Takase2006] M. Takase, Homology 3-spheres in codimension three, Internat. J. Math. 17 (2006), no.8, 869–885.
arxiv:math/0506464 MR2261638 (2007g:57049) Zbl 1113.57013
- [Viro1973] O. J. Viro, Local knotting of sub-manifolds, Mat. Sb. (N.S.) 90(132) (1973), 173–183, 325. MR0370606 (51 #6833)
- [Wall1965] C. T. C. Wall, Unknotting tori in codimension one and spheres in codimension two., Proc. Camb. Philos. Soc. 61 (1965), 659-664. MR0184249 (32 #1722) Zbl 0135.41602
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
- [Weber1967] C. Weber, Plongements de polyedres dans le domaine metastable, Comment. Math. Helv. 42 (1967), 1-27. MR0238330 (38 #6606) Zbl 0152.22402
- [Weiss] M. Weiss, private communication
- [Weiss96] M. Weiss, Calculus of Embeddings, Bull. Amer. Math. Soc. 33 (1996), 177-187.
- [Weiss99] M. Weiss, Embeddings from the point of view of immersion theory, I, Geom. and Topology 3 (1999), 67-101. Erratum in Geom. and Topology 15, 2011.
- [Wu1958c] W. Wu, On the isotopy of
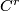 -manifolds of dimension
-manifolds of dimension  in Euclidean
in Euclidean 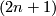 -space, Science Records, New Ser. Vol. II (1958), 271–275.
-space, Science Records, New Ser. Vol. II (1958), 271–275.
- [Zeeman1960] E. Zeeman, Unknotting spheres in five dimensions, Bull. Amer. Math. Soc. 66 (1960) 198. MR0117737 (22 #8512a) Zbl 0117.40904
- [Zeeman1963] E. C. Zeeman, Seminar on Combinatorial Topology, IHES, 1963 (revised 1966).
- [Zeeman1993] E.C. Zeeman, A Brief History of Topology, UC Berkeley, October 27, 1993, On the occasion of Moe Hirsch's 60th birthday, http://zakuski.utsa.edu/~gokhman/ecz/hirsch60.pdf
Remark 1.1 (Some motivations). Three important classical problems in topology are the following, cf. [Zeeman1993, p. 3].
- The Manifold Problem: Classify
 -manifolds.
-manifolds.
- The Embedding Problem: Find the least dimension
 such that a given manifold admits an embedding into
such that a given manifold admits an embedding into  -dimensional Euclidean space
-dimensional Euclidean space  .
.
- The Knotting Problem: Classify embeddings of a given manifold into another given manifold up to isotopy.
The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others.
The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark 1.4 below).
See also the Wikipedia article on knot theory and [Skopenkov2016t, Example 2.3], [Takase2006,  1].
1].
This article gives a short guide to the problem of classifying embeddings of closed manifolds  into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds
into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds  into
into  or
or  ). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of
). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of  are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
The most interesting and much studied case of embeddings concerns classical knots, which are embeddings 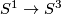 , or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and
, or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and  5 for more information.
5 for more information.
The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which readily calculable classification results (see Remark 1.2) describing all isotopy classes for embeddings of a closed manifold into Euclidean space  are known. Such classification results are presented on the pages listed in Remark 1.4, in
are known. Such classification results are presented on the pages listed in Remark 1.4, in  2 and in
2 and in  6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
The results and remarks given below show the following:
- For a fixed
 , the more
, the more  decreases from
decreases from  towards
towards 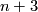 , the more complicated the classification of embeddings of
, the more complicated the classification of embeddings of  into
into  becomes.
becomes.
- The complete readily calculable classification of embeddings into
 of closed connected
of closed connected  -manifolds is non-trivial and presently accessible only for
-manifolds is non-trivial and presently accessible only for 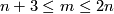 or for
or for 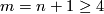 ; the lowest dimensional cases, i.e. all such pairs
; the lowest dimensional cases, i.e. all such pairs 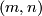 with
with 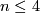 , are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
, are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
Remark 1.2 (Readily calculable classification). The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See [Graham&Knuth&Patashnik89, Preface] for discussion of similar issues. To a first approximation, a classification of embeddings 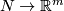 is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number
is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number  and the manifold
and the manifold  . This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
Since this is a paper on the Knotting Problem of Remark 1.1, below `classification' means `classification of embeddings of a manifold  into
into  up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
(a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is.
(b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold  is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
(c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See [Wall1999, Corollary 11.3.1] and a simpler exposition in [Cencelj&Repovs&Skopenkov2004, pp. 263-267]. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' [Wall1999, p. 119]. An analogue of this result for classification is [Cencelj&Repovs&Skopenkov2004, the Browder-Wall Theorem 9]. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to [Wall1999, Corollary 11.3.3] as exposed in [Cencelj&Repovs&Skopenkov2004, pp. 265-267]. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see [Skopenkov2016t], [Skopenkov2016f]) have been obtained by applying Kreck's modified surgery theory, see (d).
(d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark 1.1. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory [Kreck1999] was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see [Kreck1999].
(i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. the Haefliger-Zeeman Theorem [Skopenkov2016h, Theorem 4.1] classifies links in terms of (stable) homotopy groups of spheres. These groups are not known in general. However, these groups are described for many particular cases which give the table [Skopenkov2016h,  1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
(ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey [Skopenkov2006,  5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
- the Becker-Glover Theorem [Skopenkov2016e, Theorem 6.5] and the classification of knotted tori [Skopenkov2016k, Theorem 3.2], which I regard as readily calculable classifications;
- the independence of the classification of embeddings of the smooth or PL structure on the manifold;
- the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) [Cadek&Krcal&Vokrınek2013, Theorem 1.1 and text after Theorem 1.4].
(iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem [Weiss96], [Weiss99], [Goodwillie&Weiss1999]. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings 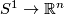 [Weiss], [Arone&Turchin2014].
[Weiss], [Arone&Turchin2014].
Remark 1.3 (Embeddings into Euclidean space and the sphere).
(a) The embeddings 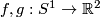 given by
given by 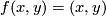 and
and 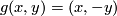 are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of
are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of  into
into  are isotopic, see Theorem 6.1.a and below it.
are isotopic, see Theorem 6.1.a and below it.
(b) For  the classifications of embeddings of compact
the classifications of embeddings of compact  -manifolds into
-manifolds into  and into
and into  are the same. More precisely, for all integers
are the same. More precisely, for all integers  such that
such that  , and for every
, and for every  -manifold
-manifold  , the map
, the map 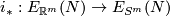 between the sets of isotopy classes of embeddings
between the sets of isotopy classes of embeddings 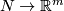 and
and 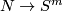 ,
which is induced by composition with the inclusion
,
which is induced by composition with the inclusion 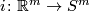 , is a bijection.
, is a bijection.
Let us prove part (b). Since 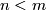 , after a small isotopy an embedding
, after a small isotopy an embedding  missed the point at infinity and so lies in
missed the point at infinity and so lies in 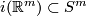 .
Hence
.
Hence  is onto.
To prove that
is onto.
To prove that  is injective, it suffices to show that if the compositions with the inclusion
is injective, it suffices to show that if the compositions with the inclusion 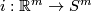 of two embeddings
of two embeddings 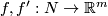 of a compact
of a compact  -manifold
-manifold  are isotopic, then
are isotopic, then  and
and  are isotopic. For showing that assume that
are isotopic. For showing that assume that 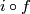 and
and  are isotopic. Then by general position
are isotopic. Then by general position  and
and  are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],
are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],  and
and  are isotopic.
are isotopic.
Remark 1.4 (References to information on the classification of embeddings). The first list is structured by the dimension of the source manifold and the target Euclidean space:
Information structured by the `complexity' of the source manifold:
For more information see e.g. [Skopenkov2006].
2 Unknotting theorems
If the category is omitted, then a result stated below holds in both the smooth and piecewise-linear (PL) category.
General Position Theorem 2.1 ([Hirsch1976, Theorem 3.5], [Rourke&Sanderson1972, Theorem 5.4]).
For every compact  -manifold
-manifold  and
and 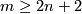 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
The case 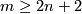 is called a `stable range' (for the classification problem; for the existence problem the analogous result with
is called a `stable range' (for the classification problem; for the existence problem the analogous result with 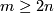 is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
The restriction 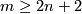 in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking
in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking 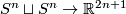 shows [Skopenkov2016h, Example 2.1].
shows [Skopenkov2016h, Example 2.1].
Whitney-Wu Unknotting Theorem 2.2.
For every compact connected  -manifold
-manifold  ,
, 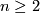 and
and 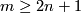 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Wu1958c] using the Whitney trick (see that reference or [Rourke&Sanderson1972,  5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
All the three assumptions in this result are indeed necessary:
- the assumption
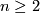 because of the existence of non-trivial knots
because of the existence of non-trivial knots 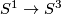 ;
;
- the connectedness assumption because of the existence of the Hopf link [Skopenkov2016h, Example 2.1];
- the assumption
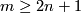 because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
Unknotting Spheres Theorem 2.3. Assume that  in the PL category or
in the PL category or 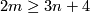 in the smooth category.
For
in the smooth category.
For 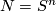 , or even for
, or even for  an integral homology
an integral homology  -sphere, any two embeddings of
-sphere, any two embeddings of  into
into  are isotopic.
are isotopic.
For 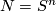 this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For
this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For  an integral homology
an integral homology  -sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for
-sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for  in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
The case 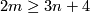 is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with
is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with 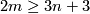 [Skopenkov2006,
[Skopenkov2006,  2,
2,  5]).
5]).
Knots in codimension 2 and the Haefliger trefoil knot [Skopenkov2016t, Example 2.1] show that the dimension restrictions are sharp (even for 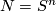 ) in the Unknotting Spheres Theorem 2.3.
) in the Unknotting Spheres Theorem 2.3.
Theorems 2.2 and 2.3 may be generalized as follows.
The Haefliger-Zeeman Unknotting Theorem 2.4.
For every 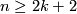 ,
, 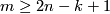 and closed
and closed  -connected
-connected  -manifold
-manifold  , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Zeeman1963, Corollary 2 of Theorem 24 in Chapter 8], [Haefliger1961, Existence Theorem (b) in p. 47] in the PL or in the smooth category, respectively. The PL case uses the ideas of [Penrose&Whitehead&Zeeman1961] and [Irwin1965]; see also textbooks [Hudson1969,  11], [Rourke&Sanderson1972,
11], [Rourke&Sanderson1972,  5]. Theorem 2.4 remains true if
5]. Theorem 2.4 remains true if  -connected is replaced by homologically
-connected is replaced by homologically  -connected (see
-connected (see  3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
Note that if 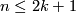 , then every closed
, then every closed  -connected
-connected  -manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
-manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
Given Theorem 2.4 above, the case 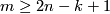 can be called a `stable range for
can be called a `stable range for  -connected manifolds'.
-connected manifolds'.
For generalizations of Theorem 2.4 see survey [Skopenkov2016e] and [Hudson1967], [Hacon1968], [Hudson1972], [Gordon1972], [Kearton1979]. See also Theorem 6.1.
3 Notation and conventions
The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark 1.4.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy'; see definition in [Skopenkov2016i,  1]. For a manifold
1]. For a manifold  let
let 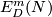 or
or 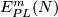 denote the set of
smooth or piecewise-linear (PL) embeddings
denote the set of
smooth or piecewise-linear (PL) embeddings 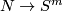 up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
The sources of all embeddings are assumed to be compact.
Let  be a closed
be a closed  -ball in a closed connected
-ball in a closed connected  -manifold
-manifold  . Denote
. Denote 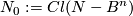 .
.
Let 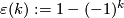 be
be  for
for  even and
even and  for
for  odd, so that
odd, so that 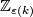 is
is  for
for  even and
even and  for
for  odd.
odd.
Tex syntax erroris the projection of a Cartesian product onto the
 th factor.
th factor.
Denote by 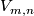 the Stiefel manifold of orthonormal
the Stiefel manifold of orthonormal  -frames in
-frames in  .
.
We omit  -coefficients from the notation of (co)homology groups.
-coefficients from the notation of (co)homology groups.
For a manifold  with boundary
with boundary 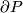 denote
denote 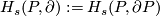 .
.
A closed manifold  is called homologically
is called homologically  -connected, if
-connected, if  is connected and
is connected and 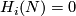 for every
for every  .
This condition is equivalent to
.
This condition is equivalent to 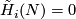 for each
for each 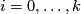 , where
, where 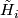 are reduced homology groups.
A pair
are reduced homology groups.
A pair 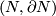 is called homologically
is called homologically  -connected, if
-connected, if  for every
for every 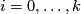 .
.
The self-intersection set of a map 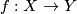 is
is 
For a smooth embedding  denote by
denote by
-
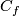 the closure of the complement in
the closure of the complement in 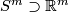 to a tight enough tubular neighborhood of
to a tight enough tubular neighborhood of  and
and
-
 the restriction of the linear normal bundle of
the restriction of the linear normal bundle of  to the subspace of unit length vectors identified with
to the subspace of unit length vectors identified with 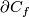 .
.
Tex syntax errorthe standard embedding given by
Tex syntax error. The natural normal framing by vectors of length 1/2 on
Tex syntax errordefines the standard embedding
Tex syntax error. Denote by the same symbol
Tex syntax errorthe restriction of
Tex syntax errorto
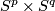 for any
for any 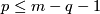 .
.
Denote by 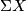 the suspension of a space
the suspension of a space  . Denote by
. Denote by  the suspension homomorphism. Recall that
the suspension homomorphism. Recall that  is an isomorphism for
is an isomorphism for 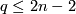 . Let
. Let  be any of the groups
be any of the groups 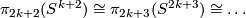 identified by the suspension isomorphism.
Denote by
identified by the suspension isomorphism.
Denote by 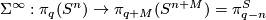 the stable suspension homomorphisms, where
the stable suspension homomorphisms, where  is large.
is large.
4 Embedded connected sum
Suppose that  ,
,  is a closed connected
is a closed connected  -manifold and, if
-manifold and, if  is orientable, an orientation of
is orientable, an orientation of  is chosen.
Let us define the embedded connected sum operation
is chosen.
Let us define the embedded connected sum operation 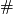 of
of  on
on  .
.
Represent isotopy classes ![[f]\in E^m(N)](/images/math/2/f/5/2f52f1701a659817e8b3dfbab85d0fef.png) and
and ![[g]\in E^m(S^n)](/images/math/0/0/3/003b3d9600f355a6b75926b59ee4b4c6.png) by embeddings
by embeddings  and
and 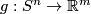 whose images are contained in disjoint balls.
Join the images of
whose images are contained in disjoint balls.
Join the images of 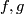 by an arc whose interior misses the images.
Let
by an arc whose interior misses the images.
Let ![[f]\#[g]](/images/math/b/5/0/b50485694938d441de43bc8d0d73e64c.png) be the isotopy class of the embedded connected sum of
be the isotopy class of the embedded connected sum of  and
and  along this arc (compatible with the orientation, if
along this arc (compatible with the orientation, if  is orientable), cf. [Haefliger1966, 1.3, 1.4].
is orientable), cf. [Haefliger1966, 1.3, 1.4].
This operation is well-defined,
i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes ![[f]](/images/math/d/d/4/dd43b82529dd8d403c1585c5d151a163.png) and
and ![[g]](/images/math/0/8/a/08a05aeade465b1c6abe241850947aab.png) , and is independent of the choice of the path and of the representatives
, and is independent of the choice of the path and of the representatives 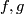 .
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for
.
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for 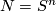 in [Skopenkov2015a,
in [Skopenkov2015a,  3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for
3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for  a point].
The proof for arbitrary closed connected
a point].
The proof for arbitrary closed connected  -manifold
-manifold  is analogous.
is analogous.
Moreover, for  embedded connected sum defines a group structure on
embedded connected sum defines a group structure on  [Haefliger1966, 1.3-1.7], and an action
[Haefliger1966, 1.3-1.7], and an action 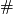 of
of  on
on  .
.
5 Some remarks on codimension 2 embeddings
The case of embeddings of  into
into 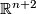 is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
Let  be a closed connected
be a closed connected  -manifold.
Using embedded connected sum (
-manifold.
Using embedded connected sum ( 4) we can apparently produce an overwhelming multitude of embeddings
4) we can apparently produce an overwhelming multitude of embeddings 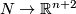 from the overwhelming multitude of embeddings
from the overwhelming multitude of embeddings  .
(However, note that for
.
(However, note that for  there are embeddings
there are embeddings 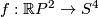 and
and 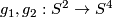 such that
such that 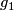 is not isotopic to
is not isotopic to 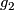 but
but 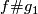 is isotopic to
is isotopic to 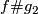 [Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928]
[Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928] 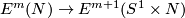 for
for 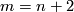 .
Thus the description of
.
Thus the description of 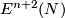 is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
For studies of codimension 2 embeddings of manifolds up to the weaker relation of concordance see e.g. [Cappell&Shaneson1974].
6 Codimension 1 embeddings
Theorem 6.1.
(a) Any two smooth embeddings of  into
into 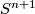 are smoothly isotopic for every
are smoothly isotopic for every  .
.
(b) Any two smooth embeddings of 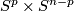 into
into 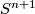 are smoothly isotopic for every
are smoothly isotopic for every 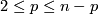 .
.
Part (a) is proved in [Milnor1965a, Proposition D in  9] as an easy corollary of the
9] as an easy corollary of the  -cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,
-cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,  1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
Theorem 6.2 (Alexander torus theorem). [Alexander1924]
For every embedding 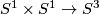 there is an autohomeomorphism
there is an autohomeomorphism  of
of 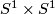 such that
such that  extends to an embedding
extends to an embedding 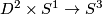 .
.
Clearly, only the standard embedding extends to both.
Remark 6.3. (a) (on PL topological category) The analogue of Theorem 6.1.a holds
- for
 in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,
in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,  1.8].
1.8].
- for
 in the PL category (Alexander Theorem, 1923) [Rushing1973,
in the PL category (Alexander Theorem, 1923) [Rushing1973,  1.8].
1.8].
- for every
 in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
The famous counterexample to the analogue of Theorem 6.1.a for  in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of
in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of  into
into 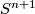 are isotopic for every
are isotopic for every  (this is equivalent to the description of
(this is equivalent to the description of 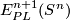 ).
).
(b) (some speculations) If the extension of the Alexander torus theorem 6.2 respects isotopy, the extension would give a 1-1 correspondence between 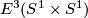 and the union of
and the union of 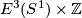 and
and 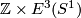 with `base points'
with `base points' 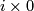 and
and  identified (where
identified (where  is the isotopy class of the standard inclusion
is the isotopy class of the standard inclusion 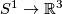 ). So the description of
). So the description of 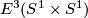 would be as hopeless as that of
would be as hopeless as that of 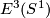 . Thus the description of
. Thus the description of 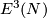 for
for  a sphere with handles is apparently hopeless.
a sphere with handles is apparently hopeless.
7 References
- [Alexander1924] J. W. Alexander, On the subdivision of 3-space by polyhedron, Proc. Nat. Acad. Sci. USA, 10, (1924) 6–8. Zbl 50.0659.01
- [Arone&Turchin2014] G. Arone and V. Turchin, On the rational homology of high-dimensional analogues of spaces of long knots, Geom. and Topol. 18 (2014), 1261-1322.
- [Artin1928] E. Artin, Zur Isotopie zwei-dimensionaler Flaechen im
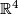 , Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
, Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
- [Cadek&Krcal&Vokrınek2013] M. Cadek, M. Kr\v c\'al, and L. Vok\v r\'ınek. Algorithmic solvability of the lifting-extension problem, arXiv:1307.6444.
- [Cappell&Shaneson1974] S. E. Cappell and J. L. Shaneson, The codimension two placement problem and homology equivalent manifolds, Ann. of Math. (2) 99 (1974), 277–348. MR0339216 (49 #3978) Zbl 0279.57011
- [Cencelj&Repovs&Skopenkov2004] M. Cencelj, D. Repov\v s and A. Skopenkov, On the Browder-Levine-Novikov embedding theorems, Proc. of the Steklov Math. Inst. 247 (2004) 280-290.
- [Gluck1963] H. Gluck, Unknotting
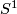 in
in 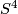 ., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
- [Goldstein1967] R. Z. Goldstein, Piecewise linear unknotting of
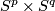 in
in 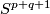 , Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
, Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
- [Goodwillie&Weiss1999] T. Goodwillie and M. Weiss, Embeddings from the point of view of immersion theory, II, Geometry and Topology, 3 (1999), 103-118. MR1694812 (2000c:57055a) Zbl 0927.57028
- [Gordon1972] C. Gordon, Embedding piecewise linear manifolds with boundary., Proc. Camb. Philos. Soc. 72 (1972), 21-25. MR0295359 (45 #4425) Zbl 0236.57009
- [Graham&Knuth&Patashnik89] R. Graham, D. Knuth, and O. Patashnik, Concrete Mathematics: A Foundation for Computer Science, Addison–Wesley, first published in 1989, ISBN 0-201-55802-5, https://www.csie.ntu.edu.tw/~r97002/temp/Concrete%20Mathematics%202e.pdf.
- [Hacon1968] D. Hacon, Embeddings of
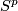 in
in 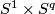 in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
- [Haefliger1961] A. Haefliger, Plongements différentiables de variétés dans variétés., Comment. Math. Helv.36 (1961), 47-82. MR0145538 (26 #3069) Zbl 0102.38603
- [Haefliger1963] A. Haefliger, Plongements différentiables dans le domain stable., Comment. Math. Helv.37 (1963), 155-176.
- [Haefliger1966] A. Haefliger, Differential embeddings of
 in
in  for
for  , Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
- [Hirsch1976] M. W. Hirsch, Differential topology., Graduate Texts in Mathematics, No. 33. Springer-Verlag., New York-Heidelberg, 1976. MR0448362 (56 #6669) Zbl 0356.57001
- [Hudson1967] J. Hudson, Piecewise linear embeddings, Ann. of Math. (2) 85 (1967) 1–31. MR0215308 (35 #6149) Zbl 0153.25601
- [Hudson1969] J. F. P. Hudson, Piecewise linear topology, W. A. Benjamin, Inc., New York-Amsterdam, 1969. MR0248844 (40 #2094) Zbl 0189.54507
- [Hudson1972] J. Hudson, Embeddings of bounded manifolds., Proc. Camb. Philos. Soc. 72 (1972), 11-20. MR0298679 (45 #7728) Zbl 0241.57006
- [Irwin1965] M. Irwin, Embeddings of polyhedral manifolds, Ann. of Math. (2) 82 (1965) 1–14. MR0182978 (32 #460) Zbl 0132.20003
- [Kearton1979] C. Kearton, Obstructions to embedding and isotopy in the metastable range, Math. Ann. 243 (1979), 103-113. MR0543720 (82k:57012) Zbl 0401.57033
- [Kosinski1961] A. Kosinski, On Alexander's theorem and knotted tori, In: Topology of 3-Manifolds, Prentice-Hall, Englewood Cliffs, Ed. M.~K.~Fort, N.J., 1962, 55--57. Cf. Fort1962.
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Lucas&Neto&Saeki1996] L. A. Lucas, O. M. Neto and O. Saeki, A generalization of Alexander's torus theorem to higher dimensions and an unknotting theorem for
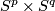 embedded in
embedded in  , Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
, Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
- [Lucas&Saeki2002] L. A. Lucas and O. Saeki, Embeddings of
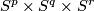 in
in 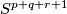 , Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
, Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
- [Milnor1965a] J. Milnor, Lectures on the
 -cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
-cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
- [Penrose&Whitehead&Zeeman1961] R. Penrose, J. Whitehead and E. Zeeman, Imbedding of manifolds in Euclidean space., Ann. of Math. 73 (1961) 613–623. MR0124909 (23 #A2218) Zbl 0113.38101
- [Rourke&Sanderson1972] C. Rourke and B. Sanderson, Introduction to piecewise-linear topology., Ergebnisse der Mathematik und ihrer Grenzgebiete. Band 69. Berlin-Heidelberg-New York: Springer-Verlag. VIII, 1972. MR0665919 (83g:57009) Zbl 0254.57010
- [Rubinstein1980] J.H. Rubinstein, Dehn’s lemma and handle decompositions of some 4-manifolds, Pacific J. Math., 86 (1980), 565-569. MR590570 (81 #M57011) Zbl 0446.57025
- [Rushing1973] T. Rushing, Topological embeddings., Pure and Applied Mathematics, 52. New York-London: Academic Press. XIII, 1973. MRMR0348752 (50 #1247) Zbl 0295.57003
- [Scharlemann1977] M. Scharlemann, Isotopy and cobordism of homology spheres in spheres., J. Lond. Math. Soc., II. Ser. 16 (1977), 559-567. MRMR0464246 (57 #4180) Zbl 0375.57003
- [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015a] A. Skopenkov, A classification of knotted tori, Proc. A of the Royal Society of Edinburgh, 150:2 (2020), 549-567. Full version: http://arxiv.org/abs/1502.04470
- [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
- [Skopenkov2016f] A. Skopenkov, 4-manifolds in 7-space, to appear in Bull. Man. Atl.
- [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
- [Skopenkov2016i] A. Skopenkov, Isotopy, submitted to Bull. Man. Atl.
- [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
- [Skopenkov2016s] A. Skopenkov, Knots, i.e. embeddings of spheres, preprint.
- [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.
- [Smale1961] S. Smale, Generalized Poincaré's conjecture in dimensions greater than four, Ann. of Math. (2) 74 (1961), 391–406. MR0137124 (25 #580) Zbl 0099.39202
- [Stallings1963] J. Stallings, On topologically unknotted spheres., (1963). MRMR0149458 (26 #6946) Zbl 0121.18202
- [Takase2006] M. Takase, Homology 3-spheres in codimension three, Internat. J. Math. 17 (2006), no.8, 869–885.
arxiv:math/0506464 MR2261638 (2007g:57049) Zbl 1113.57013
- [Viro1973] O. J. Viro, Local knotting of sub-manifolds, Mat. Sb. (N.S.) 90(132) (1973), 173–183, 325. MR0370606 (51 #6833)
- [Wall1965] C. T. C. Wall, Unknotting tori in codimension one and spheres in codimension two., Proc. Camb. Philos. Soc. 61 (1965), 659-664. MR0184249 (32 #1722) Zbl 0135.41602
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
- [Weber1967] C. Weber, Plongements de polyedres dans le domaine metastable, Comment. Math. Helv. 42 (1967), 1-27. MR0238330 (38 #6606) Zbl 0152.22402
- [Weiss] M. Weiss, private communication
- [Weiss96] M. Weiss, Calculus of Embeddings, Bull. Amer. Math. Soc. 33 (1996), 177-187.
- [Weiss99] M. Weiss, Embeddings from the point of view of immersion theory, I, Geom. and Topology 3 (1999), 67-101. Erratum in Geom. and Topology 15, 2011.
- [Wu1958c] W. Wu, On the isotopy of
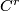 -manifolds of dimension
-manifolds of dimension  in Euclidean
in Euclidean 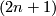 -space, Science Records, New Ser. Vol. II (1958), 271–275.
-space, Science Records, New Ser. Vol. II (1958), 271–275.
- [Zeeman1960] E. Zeeman, Unknotting spheres in five dimensions, Bull. Amer. Math. Soc. 66 (1960) 198. MR0117737 (22 #8512a) Zbl 0117.40904
- [Zeeman1963] E. C. Zeeman, Seminar on Combinatorial Topology, IHES, 1963 (revised 1966).
- [Zeeman1993] E.C. Zeeman, A Brief History of Topology, UC Berkeley, October 27, 1993, On the occasion of Moe Hirsch's 60th birthday, http://zakuski.utsa.edu/~gokhman/ecz/hirsch60.pdf
Remark 1.1 (Some motivations). Three important classical problems in topology are the following, cf. [Zeeman1993, p. 3].
- The Manifold Problem: Classify
 -manifolds.
-manifolds.
- The Embedding Problem: Find the least dimension
 such that a given manifold admits an embedding into
such that a given manifold admits an embedding into  -dimensional Euclidean space
-dimensional Euclidean space  .
.
- The Knotting Problem: Classify embeddings of a given manifold into another given manifold up to isotopy.
The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others.
The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark 1.4 below).
See also the Wikipedia article on knot theory and [Skopenkov2016t, Example 2.3], [Takase2006,  1].
1].
This article gives a short guide to the problem of classifying embeddings of closed manifolds  into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds
into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds  into
into  or
or  ). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of
). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of  are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
The most interesting and much studied case of embeddings concerns classical knots, which are embeddings 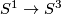 , or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and
, or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and  5 for more information.
5 for more information.
The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which readily calculable classification results (see Remark 1.2) describing all isotopy classes for embeddings of a closed manifold into Euclidean space  are known. Such classification results are presented on the pages listed in Remark 1.4, in
are known. Such classification results are presented on the pages listed in Remark 1.4, in  2 and in
2 and in  6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
The results and remarks given below show the following:
- For a fixed
 , the more
, the more  decreases from
decreases from  towards
towards 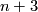 , the more complicated the classification of embeddings of
, the more complicated the classification of embeddings of  into
into  becomes.
becomes.
- The complete readily calculable classification of embeddings into
 of closed connected
of closed connected  -manifolds is non-trivial and presently accessible only for
-manifolds is non-trivial and presently accessible only for 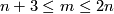 or for
or for 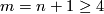 ; the lowest dimensional cases, i.e. all such pairs
; the lowest dimensional cases, i.e. all such pairs 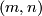 with
with 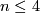 , are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
, are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
Remark 1.2 (Readily calculable classification). The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See [Graham&Knuth&Patashnik89, Preface] for discussion of similar issues. To a first approximation, a classification of embeddings 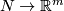 is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number
is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number  and the manifold
and the manifold  . This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
Since this is a paper on the Knotting Problem of Remark 1.1, below `classification' means `classification of embeddings of a manifold  into
into  up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
(a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is.
(b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold  is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
(c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See [Wall1999, Corollary 11.3.1] and a simpler exposition in [Cencelj&Repovs&Skopenkov2004, pp. 263-267]. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' [Wall1999, p. 119]. An analogue of this result for classification is [Cencelj&Repovs&Skopenkov2004, the Browder-Wall Theorem 9]. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to [Wall1999, Corollary 11.3.3] as exposed in [Cencelj&Repovs&Skopenkov2004, pp. 265-267]. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see [Skopenkov2016t], [Skopenkov2016f]) have been obtained by applying Kreck's modified surgery theory, see (d).
(d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark 1.1. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory [Kreck1999] was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see [Kreck1999].
(i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. the Haefliger-Zeeman Theorem [Skopenkov2016h, Theorem 4.1] classifies links in terms of (stable) homotopy groups of spheres. These groups are not known in general. However, these groups are described for many particular cases which give the table [Skopenkov2016h,  1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
(ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey [Skopenkov2006,  5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
- the Becker-Glover Theorem [Skopenkov2016e, Theorem 6.5] and the classification of knotted tori [Skopenkov2016k, Theorem 3.2], which I regard as readily calculable classifications;
- the independence of the classification of embeddings of the smooth or PL structure on the manifold;
- the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) [Cadek&Krcal&Vokrınek2013, Theorem 1.1 and text after Theorem 1.4].
(iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem [Weiss96], [Weiss99], [Goodwillie&Weiss1999]. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings 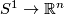 [Weiss], [Arone&Turchin2014].
[Weiss], [Arone&Turchin2014].
Remark 1.3 (Embeddings into Euclidean space and the sphere).
(a) The embeddings 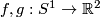 given by
given by 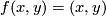 and
and 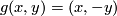 are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of
are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of  into
into  are isotopic, see Theorem 6.1.a and below it.
are isotopic, see Theorem 6.1.a and below it.
(b) For  the classifications of embeddings of compact
the classifications of embeddings of compact  -manifolds into
-manifolds into  and into
and into  are the same. More precisely, for all integers
are the same. More precisely, for all integers 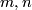 such that
such that  , and for every
, and for every  -manifold
-manifold  , the map
, the map 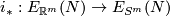 between the sets of isotopy classes of embeddings
between the sets of isotopy classes of embeddings 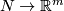 and
and 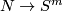 ,
which is induced by composition with the inclusion
,
which is induced by composition with the inclusion 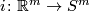 , is a bijection.
, is a bijection.
Let us prove part (b). Since 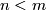 , after a small isotopy an embedding
, after a small isotopy an embedding  missed the point at infinity and so lies in
missed the point at infinity and so lies in 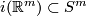 .
Hence
.
Hence  is onto.
To prove that
is onto.
To prove that  is injective, it suffices to show that if the compositions with the inclusion
is injective, it suffices to show that if the compositions with the inclusion 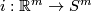 of two embeddings
of two embeddings 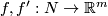 of a compact
of a compact  -manifold
-manifold  are isotopic, then
are isotopic, then  and
and  are isotopic. For showing that assume that
are isotopic. For showing that assume that 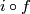 and
and  are isotopic. Then by general position
are isotopic. Then by general position  and
and  are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],
are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],  and
and  are isotopic.
are isotopic.
Remark 1.4 (References to information on the classification of embeddings). The first list is structured by the dimension of the source manifold and the target Euclidean space:
Information structured by the `complexity' of the source manifold:
For more information see e.g. [Skopenkov2006].
2 Unknotting theorems
If the category is omitted, then a result stated below holds in both the smooth and piecewise-linear (PL) category.
General Position Theorem 2.1 ([Hirsch1976, Theorem 3.5], [Rourke&Sanderson1972, Theorem 5.4]).
For every compact  -manifold
-manifold  and
and 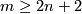 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
The case 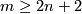 is called a `stable range' (for the classification problem; for the existence problem the analogous result with
is called a `stable range' (for the classification problem; for the existence problem the analogous result with 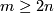 is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
The restriction 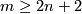 in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking
in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking 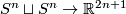 shows [Skopenkov2016h, Example 2.1].
shows [Skopenkov2016h, Example 2.1].
Whitney-Wu Unknotting Theorem 2.2.
For every compact connected  -manifold
-manifold  ,
, 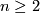 and
and 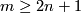 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Wu1958c] using the Whitney trick (see that reference or [Rourke&Sanderson1972,  5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
All the three assumptions in this result are indeed necessary:
- the assumption
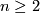 because of the existence of non-trivial knots
because of the existence of non-trivial knots 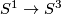 ;
;
- the connectedness assumption because of the existence of the Hopf link [Skopenkov2016h, Example 2.1];
- the assumption
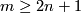 because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
Unknotting Spheres Theorem 2.3. Assume that  in the PL category or
in the PL category or 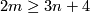 in the smooth category.
For
in the smooth category.
For 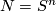 , or even for
, or even for  an integral homology
an integral homology  -sphere, any two embeddings of
-sphere, any two embeddings of  into
into  are isotopic.
are isotopic.
For 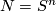 this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For
this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For  an integral homology
an integral homology  -sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for
-sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for  in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
The case 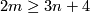 is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with
is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with 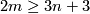 [Skopenkov2006,
[Skopenkov2006,  2,
2,  5]).
5]).
Knots in codimension 2 and the Haefliger trefoil knot [Skopenkov2016t, Example 2.1] show that the dimension restrictions are sharp (even for 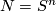 ) in the Unknotting Spheres Theorem 2.3.
) in the Unknotting Spheres Theorem 2.3.
Theorems 2.2 and 2.3 may be generalized as follows.
The Haefliger-Zeeman Unknotting Theorem 2.4.
For every 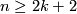 ,
, 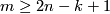 and closed
and closed  -connected
-connected  -manifold
-manifold  , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Zeeman1963, Corollary 2 of Theorem 24 in Chapter 8], [Haefliger1961, Existence Theorem (b) in p. 47] in the PL or in the smooth category, respectively. The PL case uses the ideas of [Penrose&Whitehead&Zeeman1961] and [Irwin1965]; see also textbooks [Hudson1969,  11], [Rourke&Sanderson1972,
11], [Rourke&Sanderson1972,  5]. Theorem 2.4 remains true if
5]. Theorem 2.4 remains true if  -connected is replaced by homologically
-connected is replaced by homologically  -connected (see
-connected (see  3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
Note that if 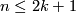 , then every closed
, then every closed  -connected
-connected  -manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
-manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
Given Theorem 2.4 above, the case 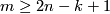 can be called a `stable range for
can be called a `stable range for  -connected manifolds'.
-connected manifolds'.
For generalizations of Theorem 2.4 see survey [Skopenkov2016e] and [Hudson1967], [Hacon1968], [Hudson1972], [Gordon1972], [Kearton1979]. See also Theorem 6.1.
3 Notation and conventions
The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark 1.4.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy'; see definition in [Skopenkov2016i,  1]. For a manifold
1]. For a manifold  let
let 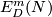 or
or 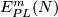 denote the set of
smooth or piecewise-linear (PL) embeddings
denote the set of
smooth or piecewise-linear (PL) embeddings 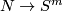 up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
The sources of all embeddings are assumed to be compact.
Let  be a closed
be a closed  -ball in a closed connected
-ball in a closed connected  -manifold
-manifold  . Denote
. Denote 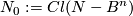 .
.
Let 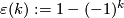 be
be  for
for  even and
even and  for
for  odd, so that
odd, so that 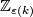 is
is  for
for  even and
even and  for
for  odd.
odd.
Tex syntax erroris the projection of a Cartesian product onto the
 th factor.
th factor.
Denote by 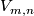 the Stiefel manifold of orthonormal
the Stiefel manifold of orthonormal  -frames in
-frames in  .
.
We omit  -coefficients from the notation of (co)homology groups.
-coefficients from the notation of (co)homology groups.
For a manifold  with boundary
with boundary 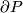 denote
denote 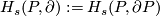 .
.
A closed manifold  is called homologically
is called homologically  -connected, if
-connected, if  is connected and
is connected and 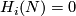 for every
for every 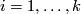 .
This condition is equivalent to
.
This condition is equivalent to 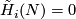 for each
for each 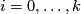 , where
, where 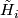 are reduced homology groups.
A pair
are reduced homology groups.
A pair 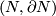 is called homologically
is called homologically  -connected, if
-connected, if  for every
for every 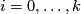 .
.
The self-intersection set of a map 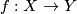 is
is 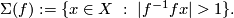
For a smooth embedding  denote by
denote by
-
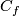 the closure of the complement in
the closure of the complement in 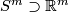 to a tight enough tubular neighborhood of
to a tight enough tubular neighborhood of  and
and
-
 the restriction of the linear normal bundle of
the restriction of the linear normal bundle of  to the subspace of unit length vectors identified with
to the subspace of unit length vectors identified with 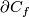 .
.
Tex syntax errorthe standard embedding given by
Tex syntax error. The natural normal framing by vectors of length 1/2 on
Tex syntax errordefines the standard embedding
Tex syntax error. Denote by the same symbol
Tex syntax errorthe restriction of
Tex syntax errorto
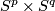 for any
for any 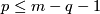 .
.
Denote by 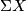 the suspension of a space
the suspension of a space  . Denote by
. Denote by  the suspension homomorphism. Recall that
the suspension homomorphism. Recall that  is an isomorphism for
is an isomorphism for 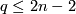 . Let
. Let 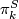 be any of the groups
be any of the groups 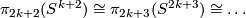 identified by the suspension isomorphism.
Denote by
identified by the suspension isomorphism.
Denote by 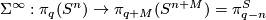 the stable suspension homomorphisms, where
the stable suspension homomorphisms, where  is large.
is large.
4 Embedded connected sum
Suppose that  ,
,  is a closed connected
is a closed connected  -manifold and, if
-manifold and, if  is orientable, an orientation of
is orientable, an orientation of  is chosen.
Let us define the embedded connected sum operation
is chosen.
Let us define the embedded connected sum operation  of
of  on
on  .
.
Represent isotopy classes ![[f]\in E^m(N)](/images/math/2/f/5/2f52f1701a659817e8b3dfbab85d0fef.png) and
and ![[g]\in E^m(S^n)](/images/math/0/0/3/003b3d9600f355a6b75926b59ee4b4c6.png) by embeddings
by embeddings  and
and 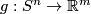 whose images are contained in disjoint balls.
Join the images of
whose images are contained in disjoint balls.
Join the images of 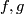 by an arc whose interior misses the images.
Let
by an arc whose interior misses the images.
Let ![[f]\#[g]](/images/math/b/5/0/b50485694938d441de43bc8d0d73e64c.png) be the isotopy class of the embedded connected sum of
be the isotopy class of the embedded connected sum of  and
and  along this arc (compatible with the orientation, if
along this arc (compatible with the orientation, if  is orientable), cf. [Haefliger1966, 1.3, 1.4].
is orientable), cf. [Haefliger1966, 1.3, 1.4].
This operation is well-defined,
i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes ![[f]](/images/math/d/d/4/dd43b82529dd8d403c1585c5d151a163.png) and
and ![[g]](/images/math/0/8/a/08a05aeade465b1c6abe241850947aab.png) , and is independent of the choice of the path and of the representatives
, and is independent of the choice of the path and of the representatives 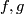 .
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for
.
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for 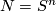 in [Skopenkov2015a,
in [Skopenkov2015a,  3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for
3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for  a point].
The proof for arbitrary closed connected
a point].
The proof for arbitrary closed connected  -manifold
-manifold  is analogous.
is analogous.
Moreover, for  embedded connected sum defines a group structure on
embedded connected sum defines a group structure on  [Haefliger1966, 1.3-1.7], and an action
[Haefliger1966, 1.3-1.7], and an action 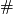 of
of  on
on  .
.
5 Some remarks on codimension 2 embeddings
The case of embeddings of  into
into 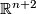 is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
Let  be a closed connected
be a closed connected  -manifold.
Using embedded connected sum (
-manifold.
Using embedded connected sum ( 4) we can apparently produce an overwhelming multitude of embeddings
4) we can apparently produce an overwhelming multitude of embeddings 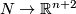 from the overwhelming multitude of embeddings
from the overwhelming multitude of embeddings  .
(However, note that for
.
(However, note that for  there are embeddings
there are embeddings 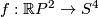 and
and 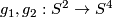 such that
such that 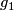 is not isotopic to
is not isotopic to 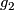 but
but 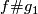 is isotopic to
is isotopic to 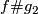 [Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928]
[Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928] 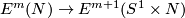 for
for 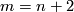 .
Thus the description of
.
Thus the description of 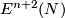 is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
For studies of codimension 2 embeddings of manifolds up to the weaker relation of concordance see e.g. [Cappell&Shaneson1974].
6 Codimension 1 embeddings
Theorem 6.1.
(a) Any two smooth embeddings of  into
into 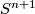 are smoothly isotopic for every
are smoothly isotopic for every  .
.
(b) Any two smooth embeddings of 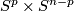 into
into 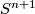 are smoothly isotopic for every
are smoothly isotopic for every 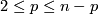 .
.
Part (a) is proved in [Milnor1965a, Proposition D in  9] as an easy corollary of the
9] as an easy corollary of the  -cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,
-cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,  1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
Theorem 6.2 (Alexander torus theorem). [Alexander1924]
For every embedding 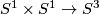 there is an autohomeomorphism
there is an autohomeomorphism  of
of 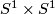 such that
such that  extends to an embedding
extends to an embedding 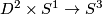 .
.
Clearly, only the standard embedding extends to both.
Remark 6.3. (a) (on PL topological category) The analogue of Theorem 6.1.a holds
- for
 in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,
in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,  1.8].
1.8].
- for
 in the PL category (Alexander Theorem, 1923) [Rushing1973,
in the PL category (Alexander Theorem, 1923) [Rushing1973,  1.8].
1.8].
- for every
 in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
The famous counterexample to the analogue of Theorem 6.1.a for  in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of
in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of  into
into 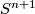 are isotopic for every
are isotopic for every  (this is equivalent to the description of
(this is equivalent to the description of 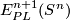 ).
).
(b) (some speculations) If the extension of the Alexander torus theorem 6.2 respects isotopy, the extension would give a 1-1 correspondence between 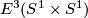 and the union of
and the union of  and
and  with `base points'
with `base points' 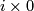 and
and  identified (where
identified (where  is the isotopy class of the standard inclusion
is the isotopy class of the standard inclusion 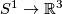 ). So the description of
). So the description of 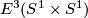 would be as hopeless as that of
would be as hopeless as that of 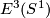 . Thus the description of
. Thus the description of 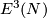 for
for  a sphere with handles is apparently hopeless.
a sphere with handles is apparently hopeless.
7 References
- [Alexander1924] J. W. Alexander, On the subdivision of 3-space by polyhedron, Proc. Nat. Acad. Sci. USA, 10, (1924) 6–8. Zbl 50.0659.01
- [Arone&Turchin2014] G. Arone and V. Turchin, On the rational homology of high-dimensional analogues of spaces of long knots, Geom. and Topol. 18 (2014), 1261-1322.
- [Artin1928] E. Artin, Zur Isotopie zwei-dimensionaler Flaechen im
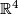 , Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
, Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
- [Cadek&Krcal&Vokrınek2013] M. Cadek, M. Kr\v c\'al, and L. Vok\v r\'ınek. Algorithmic solvability of the lifting-extension problem, arXiv:1307.6444.
- [Cappell&Shaneson1974] S. E. Cappell and J. L. Shaneson, The codimension two placement problem and homology equivalent manifolds, Ann. of Math. (2) 99 (1974), 277–348. MR0339216 (49 #3978) Zbl 0279.57011
- [Cencelj&Repovs&Skopenkov2004] M. Cencelj, D. Repov\v s and A. Skopenkov, On the Browder-Levine-Novikov embedding theorems, Proc. of the Steklov Math. Inst. 247 (2004) 280-290.
- [Gluck1963] H. Gluck, Unknotting
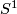 in
in 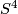 ., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
- [Goldstein1967] R. Z. Goldstein, Piecewise linear unknotting of
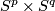 in
in 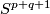 , Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
, Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
- [Goodwillie&Weiss1999] T. Goodwillie and M. Weiss, Embeddings from the point of view of immersion theory, II, Geometry and Topology, 3 (1999), 103-118. MR1694812 (2000c:57055a) Zbl 0927.57028
- [Gordon1972] C. Gordon, Embedding piecewise linear manifolds with boundary., Proc. Camb. Philos. Soc. 72 (1972), 21-25. MR0295359 (45 #4425) Zbl 0236.57009
- [Graham&Knuth&Patashnik89] R. Graham, D. Knuth, and O. Patashnik, Concrete Mathematics: A Foundation for Computer Science, Addison–Wesley, first published in 1989, ISBN 0-201-55802-5, https://www.csie.ntu.edu.tw/~r97002/temp/Concrete%20Mathematics%202e.pdf.
- [Hacon1968] D. Hacon, Embeddings of
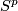 in
in 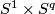 in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
- [Haefliger1961] A. Haefliger, Plongements différentiables de variétés dans variétés., Comment. Math. Helv.36 (1961), 47-82. MR0145538 (26 #3069) Zbl 0102.38603
- [Haefliger1963] A. Haefliger, Plongements différentiables dans le domain stable., Comment. Math. Helv.37 (1963), 155-176.
- [Haefliger1966] A. Haefliger, Differential embeddings of
 in
in  for
for  , Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
- [Hirsch1976] M. W. Hirsch, Differential topology., Graduate Texts in Mathematics, No. 33. Springer-Verlag., New York-Heidelberg, 1976. MR0448362 (56 #6669) Zbl 0356.57001
- [Hudson1967] J. Hudson, Piecewise linear embeddings, Ann. of Math. (2) 85 (1967) 1–31. MR0215308 (35 #6149) Zbl 0153.25601
- [Hudson1969] J. F. P. Hudson, Piecewise linear topology, W. A. Benjamin, Inc., New York-Amsterdam, 1969. MR0248844 (40 #2094) Zbl 0189.54507
- [Hudson1972] J. Hudson, Embeddings of bounded manifolds., Proc. Camb. Philos. Soc. 72 (1972), 11-20. MR0298679 (45 #7728) Zbl 0241.57006
- [Irwin1965] M. Irwin, Embeddings of polyhedral manifolds, Ann. of Math. (2) 82 (1965) 1–14. MR0182978 (32 #460) Zbl 0132.20003
- [Kearton1979] C. Kearton, Obstructions to embedding and isotopy in the metastable range, Math. Ann. 243 (1979), 103-113. MR0543720 (82k:57012) Zbl 0401.57033
- [Kosinski1961] A. Kosinski, On Alexander's theorem and knotted tori, In: Topology of 3-Manifolds, Prentice-Hall, Englewood Cliffs, Ed. M.~K.~Fort, N.J., 1962, 55--57. Cf. Fort1962.
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Lucas&Neto&Saeki1996] L. A. Lucas, O. M. Neto and O. Saeki, A generalization of Alexander's torus theorem to higher dimensions and an unknotting theorem for
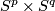 embedded in
embedded in  , Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
, Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
- [Lucas&Saeki2002] L. A. Lucas and O. Saeki, Embeddings of
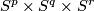 in
in 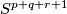 , Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
, Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
- [Milnor1965a] J. Milnor, Lectures on the
 -cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
-cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
- [Penrose&Whitehead&Zeeman1961] R. Penrose, J. Whitehead and E. Zeeman, Imbedding of manifolds in Euclidean space., Ann. of Math. 73 (1961) 613–623. MR0124909 (23 #A2218) Zbl 0113.38101
- [Rourke&Sanderson1972] C. Rourke and B. Sanderson, Introduction to piecewise-linear topology., Ergebnisse der Mathematik und ihrer Grenzgebiete. Band 69. Berlin-Heidelberg-New York: Springer-Verlag. VIII, 1972. MR0665919 (83g:57009) Zbl 0254.57010
- [Rubinstein1980] J.H. Rubinstein, Dehn’s lemma and handle decompositions of some 4-manifolds, Pacific J. Math., 86 (1980), 565-569. MR590570 (81 #M57011) Zbl 0446.57025
- [Rushing1973] T. Rushing, Topological embeddings., Pure and Applied Mathematics, 52. New York-London: Academic Press. XIII, 1973. MRMR0348752 (50 #1247) Zbl 0295.57003
- [Scharlemann1977] M. Scharlemann, Isotopy and cobordism of homology spheres in spheres., J. Lond. Math. Soc., II. Ser. 16 (1977), 559-567. MRMR0464246 (57 #4180) Zbl 0375.57003
- [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015a] A. Skopenkov, A classification of knotted tori, Proc. A of the Royal Society of Edinburgh, 150:2 (2020), 549-567. Full version: http://arxiv.org/abs/1502.04470
- [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
- [Skopenkov2016f] A. Skopenkov, 4-manifolds in 7-space, to appear in Bull. Man. Atl.
- [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
- [Skopenkov2016i] A. Skopenkov, Isotopy, submitted to Bull. Man. Atl.
- [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
- [Skopenkov2016s] A. Skopenkov, Knots, i.e. embeddings of spheres, preprint.
- [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.
- [Smale1961] S. Smale, Generalized Poincaré's conjecture in dimensions greater than four, Ann. of Math. (2) 74 (1961), 391–406. MR0137124 (25 #580) Zbl 0099.39202
- [Stallings1963] J. Stallings, On topologically unknotted spheres., (1963). MRMR0149458 (26 #6946) Zbl 0121.18202
- [Takase2006] M. Takase, Homology 3-spheres in codimension three, Internat. J. Math. 17 (2006), no.8, 869–885.
arxiv:math/0506464 MR2261638 (2007g:57049) Zbl 1113.57013
- [Viro1973] O. J. Viro, Local knotting of sub-manifolds, Mat. Sb. (N.S.) 90(132) (1973), 173–183, 325. MR0370606 (51 #6833)
- [Wall1965] C. T. C. Wall, Unknotting tori in codimension one and spheres in codimension two., Proc. Camb. Philos. Soc. 61 (1965), 659-664. MR0184249 (32 #1722) Zbl 0135.41602
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
- [Weber1967] C. Weber, Plongements de polyedres dans le domaine metastable, Comment. Math. Helv. 42 (1967), 1-27. MR0238330 (38 #6606) Zbl 0152.22402
- [Weiss] M. Weiss, private communication
- [Weiss96] M. Weiss, Calculus of Embeddings, Bull. Amer. Math. Soc. 33 (1996), 177-187.
- [Weiss99] M. Weiss, Embeddings from the point of view of immersion theory, I, Geom. and Topology 3 (1999), 67-101. Erratum in Geom. and Topology 15, 2011.
- [Wu1958c] W. Wu, On the isotopy of
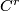 -manifolds of dimension
-manifolds of dimension  in Euclidean
in Euclidean 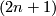 -space, Science Records, New Ser. Vol. II (1958), 271–275.
-space, Science Records, New Ser. Vol. II (1958), 271–275.
- [Zeeman1960] E. Zeeman, Unknotting spheres in five dimensions, Bull. Amer. Math. Soc. 66 (1960) 198. MR0117737 (22 #8512a) Zbl 0117.40904
- [Zeeman1963] E. C. Zeeman, Seminar on Combinatorial Topology, IHES, 1963 (revised 1966).
- [Zeeman1993] E.C. Zeeman, A Brief History of Topology, UC Berkeley, October 27, 1993, On the occasion of Moe Hirsch's 60th birthday, http://zakuski.utsa.edu/~gokhman/ecz/hirsch60.pdf
Remark 1.1 (Some motivations). Three important classical problems in topology are the following, cf. [Zeeman1993, p. 3].
- The Manifold Problem: Classify
 -manifolds.
-manifolds.
- The Embedding Problem: Find the least dimension
 such that a given manifold admits an embedding into
such that a given manifold admits an embedding into  -dimensional Euclidean space
-dimensional Euclidean space  .
.
- The Knotting Problem: Classify embeddings of a given manifold into another given manifold up to isotopy.
The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others.
The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark 1.4 below).
See also the Wikipedia article on knot theory and [Skopenkov2016t, Example 2.3], [Takase2006,  1].
1].
This article gives a short guide to the problem of classifying embeddings of closed manifolds  into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds
into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds  into
into  or
or  ). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of
). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of  are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
The most interesting and much studied case of embeddings concerns classical knots, which are embeddings 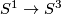 , or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and
, or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and  5 for more information.
5 for more information.
The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which readily calculable classification results (see Remark 1.2) describing all isotopy classes for embeddings of a closed manifold into Euclidean space  are known. Such classification results are presented on the pages listed in Remark 1.4, in
are known. Such classification results are presented on the pages listed in Remark 1.4, in  2 and in
2 and in  6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
The results and remarks given below show the following:
- For a fixed
 , the more
, the more  decreases from
decreases from  towards
towards 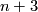 , the more complicated the classification of embeddings of
, the more complicated the classification of embeddings of  into
into  becomes.
becomes.
- The complete readily calculable classification of embeddings into
 of closed connected
of closed connected  -manifolds is non-trivial and presently accessible only for
-manifolds is non-trivial and presently accessible only for 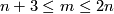 or for
or for 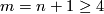 ; the lowest dimensional cases, i.e. all such pairs
; the lowest dimensional cases, i.e. all such pairs 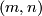 with
with 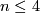 , are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
, are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
Remark 1.2 (Readily calculable classification). The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See [Graham&Knuth&Patashnik89, Preface] for discussion of similar issues. To a first approximation, a classification of embeddings 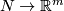 is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number
is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number  and the manifold
and the manifold  . This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
Since this is a paper on the Knotting Problem of Remark 1.1, below `classification' means `classification of embeddings of a manifold  into
into  up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
(a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is.
(b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold  is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
(c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See [Wall1999, Corollary 11.3.1] and a simpler exposition in [Cencelj&Repovs&Skopenkov2004, pp. 263-267]. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' [Wall1999, p. 119]. An analogue of this result for classification is [Cencelj&Repovs&Skopenkov2004, the Browder-Wall Theorem 9]. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to [Wall1999, Corollary 11.3.3] as exposed in [Cencelj&Repovs&Skopenkov2004, pp. 265-267]. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see [Skopenkov2016t], [Skopenkov2016f]) have been obtained by applying Kreck's modified surgery theory, see (d).
(d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark 1.1. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory [Kreck1999] was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see [Kreck1999].
(i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. the Haefliger-Zeeman Theorem [Skopenkov2016h, Theorem 4.1] classifies links in terms of (stable) homotopy groups of spheres. These groups are not known in general. However, these groups are described for many particular cases which give the table [Skopenkov2016h,  1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
(ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey [Skopenkov2006,  5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
- the Becker-Glover Theorem [Skopenkov2016e, Theorem 6.5] and the classification of knotted tori [Skopenkov2016k, Theorem 3.2], which I regard as readily calculable classifications;
- the independence of the classification of embeddings of the smooth or PL structure on the manifold;
- the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) [Cadek&Krcal&Vokrınek2013, Theorem 1.1 and text after Theorem 1.4].
(iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem [Weiss96], [Weiss99], [Goodwillie&Weiss1999]. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings 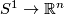 [Weiss], [Arone&Turchin2014].
[Weiss], [Arone&Turchin2014].
Remark 1.3 (Embeddings into Euclidean space and the sphere).
(a) The embeddings 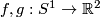 given by
given by 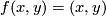 and
and 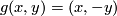 are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of
are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of  into
into  are isotopic, see Theorem 6.1.a and below it.
are isotopic, see Theorem 6.1.a and below it.
(b) For  the classifications of embeddings of compact
the classifications of embeddings of compact  -manifolds into
-manifolds into  and into
and into  are the same. More precisely, for all integers
are the same. More precisely, for all integers 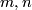 such that
such that  , and for every
, and for every  -manifold
-manifold  , the map
, the map 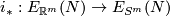 between the sets of isotopy classes of embeddings
between the sets of isotopy classes of embeddings 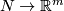 and
and 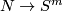 ,
which is induced by composition with the inclusion
,
which is induced by composition with the inclusion 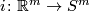 , is a bijection.
, is a bijection.
Let us prove part (b). Since 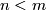 , after a small isotopy an embedding
, after a small isotopy an embedding  missed the point at infinity and so lies in
missed the point at infinity and so lies in 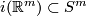 .
Hence
.
Hence  is onto.
To prove that
is onto.
To prove that  is injective, it suffices to show that if the compositions with the inclusion
is injective, it suffices to show that if the compositions with the inclusion 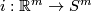 of two embeddings
of two embeddings 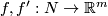 of a compact
of a compact  -manifold
-manifold  are isotopic, then
are isotopic, then  and
and  are isotopic. For showing that assume that
are isotopic. For showing that assume that 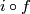 and
and  are isotopic. Then by general position
are isotopic. Then by general position  and
and  are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],
are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],  and
and  are isotopic.
are isotopic.
Remark 1.4 (References to information on the classification of embeddings). The first list is structured by the dimension of the source manifold and the target Euclidean space:
Information structured by the `complexity' of the source manifold:
For more information see e.g. [Skopenkov2006].
2 Unknotting theorems
If the category is omitted, then a result stated below holds in both the smooth and piecewise-linear (PL) category.
General Position Theorem 2.1 ([Hirsch1976, Theorem 3.5], [Rourke&Sanderson1972, Theorem 5.4]).
For every compact  -manifold
-manifold  and
and 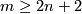 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
The case 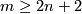 is called a `stable range' (for the classification problem; for the existence problem the analogous result with
is called a `stable range' (for the classification problem; for the existence problem the analogous result with 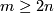 is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
The restriction 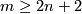 in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking
in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking 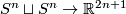 shows [Skopenkov2016h, Example 2.1].
shows [Skopenkov2016h, Example 2.1].
Whitney-Wu Unknotting Theorem 2.2.
For every compact connected  -manifold
-manifold  ,
, 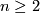 and
and 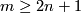 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Wu1958c] using the Whitney trick (see that reference or [Rourke&Sanderson1972,  5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
All the three assumptions in this result are indeed necessary:
- the assumption
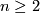 because of the existence of non-trivial knots
because of the existence of non-trivial knots 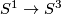 ;
;
- the connectedness assumption because of the existence of the Hopf link [Skopenkov2016h, Example 2.1];
- the assumption
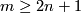 because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
Unknotting Spheres Theorem 2.3. Assume that  in the PL category or
in the PL category or 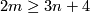 in the smooth category.
For
in the smooth category.
For 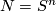 , or even for
, or even for  an integral homology
an integral homology  -sphere, any two embeddings of
-sphere, any two embeddings of  into
into  are isotopic.
are isotopic.
For 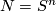 this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For
this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For  an integral homology
an integral homology  -sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for
-sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for  in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
The case 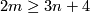 is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with
is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with 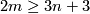 [Skopenkov2006,
[Skopenkov2006,  2,
2,  5]).
5]).
Knots in codimension 2 and the Haefliger trefoil knot [Skopenkov2016t, Example 2.1] show that the dimension restrictions are sharp (even for 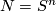 ) in the Unknotting Spheres Theorem 2.3.
) in the Unknotting Spheres Theorem 2.3.
Theorems 2.2 and 2.3 may be generalized as follows.
The Haefliger-Zeeman Unknotting Theorem 2.4.
For every 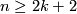 ,
, 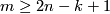 and closed
and closed  -connected
-connected  -manifold
-manifold  , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Zeeman1963, Corollary 2 of Theorem 24 in Chapter 8], [Haefliger1961, Existence Theorem (b) in p. 47] in the PL or in the smooth category, respectively. The PL case uses the ideas of [Penrose&Whitehead&Zeeman1961] and [Irwin1965]; see also textbooks [Hudson1969,  11], [Rourke&Sanderson1972,
11], [Rourke&Sanderson1972,  5]. Theorem 2.4 remains true if
5]. Theorem 2.4 remains true if  -connected is replaced by homologically
-connected is replaced by homologically  -connected (see
-connected (see  3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
Note that if 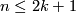 , then every closed
, then every closed  -connected
-connected  -manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
-manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
Given Theorem 2.4 above, the case 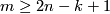 can be called a `stable range for
can be called a `stable range for  -connected manifolds'.
-connected manifolds'.
For generalizations of Theorem 2.4 see survey [Skopenkov2016e] and [Hudson1967], [Hacon1968], [Hudson1972], [Gordon1972], [Kearton1979]. See also Theorem 6.1.
3 Notation and conventions
The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark 1.4.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy'; see definition in [Skopenkov2016i,  1]. For a manifold
1]. For a manifold  let
let 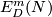 or
or 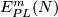 denote the set of
smooth or piecewise-linear (PL) embeddings
denote the set of
smooth or piecewise-linear (PL) embeddings 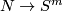 up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
The sources of all embeddings are assumed to be compact.
Let  be a closed
be a closed  -ball in a closed connected
-ball in a closed connected  -manifold
-manifold  . Denote
. Denote 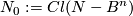 .
.
Let 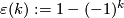 be
be  for
for  even and
even and  for
for  odd, so that
odd, so that 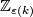 is
is  for
for  even and
even and  for
for  odd.
odd.
Tex syntax erroris the projection of a Cartesian product onto the
 th factor.
th factor.
Denote by 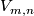 the Stiefel manifold of orthonormal
the Stiefel manifold of orthonormal  -frames in
-frames in  .
.
We omit  -coefficients from the notation of (co)homology groups.
-coefficients from the notation of (co)homology groups.
For a manifold  with boundary
with boundary 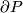 denote
denote 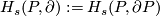 .
.
A closed manifold  is called homologically
is called homologically  -connected, if
-connected, if  is connected and
is connected and 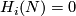 for every
for every 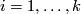 .
This condition is equivalent to
.
This condition is equivalent to  for each
for each  , where
, where  are reduced homology groups.
A pair
are reduced homology groups.
A pair 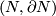 is called homologically
is called homologically  -connected, if
-connected, if  for every
for every 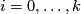 .
.
The self-intersection set of a map 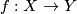 is
is 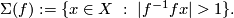
For a smooth embedding  denote by
denote by
-
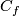 the closure of the complement in
the closure of the complement in 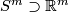 to a tight enough tubular neighborhood of
to a tight enough tubular neighborhood of  and
and
-
 the restriction of the linear normal bundle of
the restriction of the linear normal bundle of  to the subspace of unit length vectors identified with
to the subspace of unit length vectors identified with 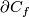 .
.
Tex syntax errorthe standard embedding given by
Tex syntax error. The natural normal framing by vectors of length 1/2 on
Tex syntax errordefines the standard embedding
Tex syntax error. Denote by the same symbol
Tex syntax errorthe restriction of
Tex syntax errorto
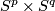 for any
for any 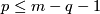 .
.
Denote by 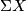 the suspension of a space
the suspension of a space  . Denote by
. Denote by  the suspension homomorphism. Recall that
the suspension homomorphism. Recall that  is an isomorphism for
is an isomorphism for 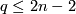 . Let
. Let 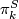 be any of the groups
be any of the groups 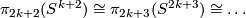 identified by the suspension isomorphism.
Denote by
identified by the suspension isomorphism.
Denote by 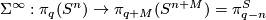 the stable suspension homomorphisms, where
the stable suspension homomorphisms, where  is large.
is large.
4 Embedded connected sum
Suppose that  ,
,  is a closed connected
is a closed connected  -manifold and, if
-manifold and, if  is orientable, an orientation of
is orientable, an orientation of  is chosen.
Let us define the embedded connected sum operation
is chosen.
Let us define the embedded connected sum operation  of
of  on
on  .
.
Represent isotopy classes ![[f]\in E^m(N)](/images/math/2/f/5/2f52f1701a659817e8b3dfbab85d0fef.png) and
and ![[g]\in E^m(S^n)](/images/math/0/0/3/003b3d9600f355a6b75926b59ee4b4c6.png) by embeddings
by embeddings  and
and 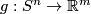 whose images are contained in disjoint balls.
Join the images of
whose images are contained in disjoint balls.
Join the images of 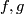 by an arc whose interior misses the images.
Let
by an arc whose interior misses the images.
Let ![[f]\#[g]](/images/math/b/5/0/b50485694938d441de43bc8d0d73e64c.png) be the isotopy class of the embedded connected sum of
be the isotopy class of the embedded connected sum of  and
and  along this arc (compatible with the orientation, if
along this arc (compatible with the orientation, if  is orientable), cf. [Haefliger1966, 1.3, 1.4].
is orientable), cf. [Haefliger1966, 1.3, 1.4].
This operation is well-defined,
i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes ![[f]](/images/math/d/d/4/dd43b82529dd8d403c1585c5d151a163.png) and
and ![[g]](/images/math/0/8/a/08a05aeade465b1c6abe241850947aab.png) , and is independent of the choice of the path and of the representatives
, and is independent of the choice of the path and of the representatives 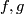 .
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for
.
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for 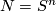 in [Skopenkov2015a,
in [Skopenkov2015a,  3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for
3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for  a point].
The proof for arbitrary closed connected
a point].
The proof for arbitrary closed connected  -manifold
-manifold  is analogous.
is analogous.
Moreover, for  embedded connected sum defines a group structure on
embedded connected sum defines a group structure on  [Haefliger1966, 1.3-1.7], and an action
[Haefliger1966, 1.3-1.7], and an action  of
of  on
on  .
.
5 Some remarks on codimension 2 embeddings
The case of embeddings of  into
into 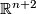 is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
Let  be a closed connected
be a closed connected  -manifold.
Using embedded connected sum (
-manifold.
Using embedded connected sum ( 4) we can apparently produce an overwhelming multitude of embeddings
4) we can apparently produce an overwhelming multitude of embeddings 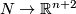 from the overwhelming multitude of embeddings
from the overwhelming multitude of embeddings  .
(However, note that for
.
(However, note that for  there are embeddings
there are embeddings 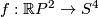 and
and 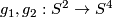 such that
such that 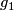 is not isotopic to
is not isotopic to 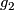 but
but 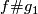 is isotopic to
is isotopic to 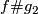 [Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928]
[Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928] 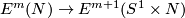 for
for 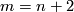 .
Thus the description of
.
Thus the description of 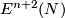 is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
For studies of codimension 2 embeddings of manifolds up to the weaker relation of concordance see e.g. [Cappell&Shaneson1974].
6 Codimension 1 embeddings
Theorem 6.1.
(a) Any two smooth embeddings of  into
into 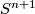 are smoothly isotopic for every
are smoothly isotopic for every  .
.
(b) Any two smooth embeddings of 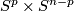 into
into 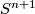 are smoothly isotopic for every
are smoothly isotopic for every 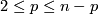 .
.
Part (a) is proved in [Milnor1965a, Proposition D in  9] as an easy corollary of the
9] as an easy corollary of the  -cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,
-cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,  1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
Theorem 6.2 (Alexander torus theorem). [Alexander1924]
For every embedding 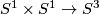 there is an autohomeomorphism
there is an autohomeomorphism  of
of 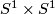 such that
such that  extends to an embedding
extends to an embedding 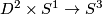 .
.
Clearly, only the standard embedding extends to both.
Remark 6.3. (a) (on PL topological category) The analogue of Theorem 6.1.a holds
- for
 in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,
in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,  1.8].
1.8].
- for
 in the PL category (Alexander Theorem, 1923) [Rushing1973,
in the PL category (Alexander Theorem, 1923) [Rushing1973,  1.8].
1.8].
- for every
 in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
The famous counterexample to the analogue of Theorem 6.1.a for  in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of
in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of  into
into 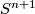 are isotopic for every
are isotopic for every  (this is equivalent to the description of
(this is equivalent to the description of 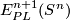 ).
).
(b) (some speculations) If the extension of the Alexander torus theorem 6.2 respects isotopy, the extension would give a 1-1 correspondence between 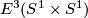 and the union of
and the union of 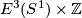 and
and 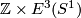 with `base points'
with `base points' 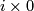 and
and  identified (where
identified (where  is the isotopy class of the standard inclusion
is the isotopy class of the standard inclusion 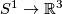 ). So the description of
). So the description of 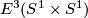 would be as hopeless as that of
would be as hopeless as that of 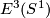 . Thus the description of
. Thus the description of 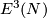 for
for  a sphere with handles is apparently hopeless.
a sphere with handles is apparently hopeless.
7 References
- [Alexander1924] J. W. Alexander, On the subdivision of 3-space by polyhedron, Proc. Nat. Acad. Sci. USA, 10, (1924) 6–8. Zbl 50.0659.01
- [Arone&Turchin2014] G. Arone and V. Turchin, On the rational homology of high-dimensional analogues of spaces of long knots, Geom. and Topol. 18 (2014), 1261-1322.
- [Artin1928] E. Artin, Zur Isotopie zwei-dimensionaler Flaechen im
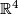 , Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
, Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
- [Cadek&Krcal&Vokrınek2013] M. Cadek, M. Kr\v c\'al, and L. Vok\v r\'ınek. Algorithmic solvability of the lifting-extension problem, arXiv:1307.6444.
- [Cappell&Shaneson1974] S. E. Cappell and J. L. Shaneson, The codimension two placement problem and homology equivalent manifolds, Ann. of Math. (2) 99 (1974), 277–348. MR0339216 (49 #3978) Zbl 0279.57011
- [Cencelj&Repovs&Skopenkov2004] M. Cencelj, D. Repov\v s and A. Skopenkov, On the Browder-Levine-Novikov embedding theorems, Proc. of the Steklov Math. Inst. 247 (2004) 280-290.
- [Gluck1963] H. Gluck, Unknotting
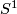 in
in 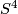 ., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
- [Goldstein1967] R. Z. Goldstein, Piecewise linear unknotting of
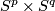 in
in 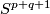 , Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
, Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
- [Goodwillie&Weiss1999] T. Goodwillie and M. Weiss, Embeddings from the point of view of immersion theory, II, Geometry and Topology, 3 (1999), 103-118. MR1694812 (2000c:57055a) Zbl 0927.57028
- [Gordon1972] C. Gordon, Embedding piecewise linear manifolds with boundary., Proc. Camb. Philos. Soc. 72 (1972), 21-25. MR0295359 (45 #4425) Zbl 0236.57009
- [Graham&Knuth&Patashnik89] R. Graham, D. Knuth, and O. Patashnik, Concrete Mathematics: A Foundation for Computer Science, Addison–Wesley, first published in 1989, ISBN 0-201-55802-5, https://www.csie.ntu.edu.tw/~r97002/temp/Concrete%20Mathematics%202e.pdf.
- [Hacon1968] D. Hacon, Embeddings of
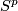 in
in 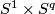 in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
- [Haefliger1961] A. Haefliger, Plongements différentiables de variétés dans variétés., Comment. Math. Helv.36 (1961), 47-82. MR0145538 (26 #3069) Zbl 0102.38603
- [Haefliger1963] A. Haefliger, Plongements différentiables dans le domain stable., Comment. Math. Helv.37 (1963), 155-176.
- [Haefliger1966] A. Haefliger, Differential embeddings of
 in
in  for
for  , Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
- [Hirsch1976] M. W. Hirsch, Differential topology., Graduate Texts in Mathematics, No. 33. Springer-Verlag., New York-Heidelberg, 1976. MR0448362 (56 #6669) Zbl 0356.57001
- [Hudson1967] J. Hudson, Piecewise linear embeddings, Ann. of Math. (2) 85 (1967) 1–31. MR0215308 (35 #6149) Zbl 0153.25601
- [Hudson1969] J. F. P. Hudson, Piecewise linear topology, W. A. Benjamin, Inc., New York-Amsterdam, 1969. MR0248844 (40 #2094) Zbl 0189.54507
- [Hudson1972] J. Hudson, Embeddings of bounded manifolds., Proc. Camb. Philos. Soc. 72 (1972), 11-20. MR0298679 (45 #7728) Zbl 0241.57006
- [Irwin1965] M. Irwin, Embeddings of polyhedral manifolds, Ann. of Math. (2) 82 (1965) 1–14. MR0182978 (32 #460) Zbl 0132.20003
- [Kearton1979] C. Kearton, Obstructions to embedding and isotopy in the metastable range, Math. Ann. 243 (1979), 103-113. MR0543720 (82k:57012) Zbl 0401.57033
- [Kosinski1961] A. Kosinski, On Alexander's theorem and knotted tori, In: Topology of 3-Manifolds, Prentice-Hall, Englewood Cliffs, Ed. M.~K.~Fort, N.J., 1962, 55--57. Cf. Fort1962.
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Lucas&Neto&Saeki1996] L. A. Lucas, O. M. Neto and O. Saeki, A generalization of Alexander's torus theorem to higher dimensions and an unknotting theorem for
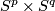 embedded in
embedded in  , Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
, Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
- [Lucas&Saeki2002] L. A. Lucas and O. Saeki, Embeddings of
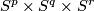 in
in 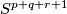 , Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
, Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
- [Milnor1965a] J. Milnor, Lectures on the
 -cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
-cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
- [Penrose&Whitehead&Zeeman1961] R. Penrose, J. Whitehead and E. Zeeman, Imbedding of manifolds in Euclidean space., Ann. of Math. 73 (1961) 613–623. MR0124909 (23 #A2218) Zbl 0113.38101
- [Rourke&Sanderson1972] C. Rourke and B. Sanderson, Introduction to piecewise-linear topology., Ergebnisse der Mathematik und ihrer Grenzgebiete. Band 69. Berlin-Heidelberg-New York: Springer-Verlag. VIII, 1972. MR0665919 (83g:57009) Zbl 0254.57010
- [Rubinstein1980] J.H. Rubinstein, Dehn’s lemma and handle decompositions of some 4-manifolds, Pacific J. Math., 86 (1980), 565-569. MR590570 (81 #M57011) Zbl 0446.57025
- [Rushing1973] T. Rushing, Topological embeddings., Pure and Applied Mathematics, 52. New York-London: Academic Press. XIII, 1973. MRMR0348752 (50 #1247) Zbl 0295.57003
- [Scharlemann1977] M. Scharlemann, Isotopy and cobordism of homology spheres in spheres., J. Lond. Math. Soc., II. Ser. 16 (1977), 559-567. MRMR0464246 (57 #4180) Zbl 0375.57003
- [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015a] A. Skopenkov, A classification of knotted tori, Proc. A of the Royal Society of Edinburgh, 150:2 (2020), 549-567. Full version: http://arxiv.org/abs/1502.04470
- [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
- [Skopenkov2016f] A. Skopenkov, 4-manifolds in 7-space, to appear in Bull. Man. Atl.
- [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
- [Skopenkov2016i] A. Skopenkov, Isotopy, submitted to Bull. Man. Atl.
- [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
- [Skopenkov2016s] A. Skopenkov, Knots, i.e. embeddings of spheres, preprint.
- [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.
- [Smale1961] S. Smale, Generalized Poincaré's conjecture in dimensions greater than four, Ann. of Math. (2) 74 (1961), 391–406. MR0137124 (25 #580) Zbl 0099.39202
- [Stallings1963] J. Stallings, On topologically unknotted spheres., (1963). MRMR0149458 (26 #6946) Zbl 0121.18202
- [Takase2006] M. Takase, Homology 3-spheres in codimension three, Internat. J. Math. 17 (2006), no.8, 869–885.
arxiv:math/0506464 MR2261638 (2007g:57049) Zbl 1113.57013
- [Viro1973] O. J. Viro, Local knotting of sub-manifolds, Mat. Sb. (N.S.) 90(132) (1973), 173–183, 325. MR0370606 (51 #6833)
- [Wall1965] C. T. C. Wall, Unknotting tori in codimension one and spheres in codimension two., Proc. Camb. Philos. Soc. 61 (1965), 659-664. MR0184249 (32 #1722) Zbl 0135.41602
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
- [Weber1967] C. Weber, Plongements de polyedres dans le domaine metastable, Comment. Math. Helv. 42 (1967), 1-27. MR0238330 (38 #6606) Zbl 0152.22402
- [Weiss] M. Weiss, private communication
- [Weiss96] M. Weiss, Calculus of Embeddings, Bull. Amer. Math. Soc. 33 (1996), 177-187.
- [Weiss99] M. Weiss, Embeddings from the point of view of immersion theory, I, Geom. and Topology 3 (1999), 67-101. Erratum in Geom. and Topology 15, 2011.
- [Wu1958c] W. Wu, On the isotopy of
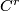 -manifolds of dimension
-manifolds of dimension  in Euclidean
in Euclidean 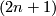 -space, Science Records, New Ser. Vol. II (1958), 271–275.
-space, Science Records, New Ser. Vol. II (1958), 271–275.
- [Zeeman1960] E. Zeeman, Unknotting spheres in five dimensions, Bull. Amer. Math. Soc. 66 (1960) 198. MR0117737 (22 #8512a) Zbl 0117.40904
- [Zeeman1963] E. C. Zeeman, Seminar on Combinatorial Topology, IHES, 1963 (revised 1966).
- [Zeeman1993] E.C. Zeeman, A Brief History of Topology, UC Berkeley, October 27, 1993, On the occasion of Moe Hirsch's 60th birthday, http://zakuski.utsa.edu/~gokhman/ecz/hirsch60.pdf
Remark 1.1 (Some motivations). Three important classical problems in topology are the following, cf. [Zeeman1993, p. 3].
- The Manifold Problem: Classify
 -manifolds.
-manifolds.
- The Embedding Problem: Find the least dimension
 such that a given manifold admits an embedding into
such that a given manifold admits an embedding into  -dimensional Euclidean space
-dimensional Euclidean space  .
.
- The Knotting Problem: Classify embeddings of a given manifold into another given manifold up to isotopy.
The Embedding and Knotting Problems have played an outstanding role in the development of topology. Various methods for the investigation of these problems were created by such classical figures as J. W. Alexander, H. Hopf, L. S. Pontryagin, R. Thom, H. Whitney, M. Atiyah, F. Hirzebruch, R. Penrose, J. H. C. Whitehead, C. Zeeman, W. Browder, J. Levine, S. P. Novikov, A. Haefliger, M. Hirsch, M. Irwin, J. F. P. Hudson and others.
The Knotting Problem is related to other areas of mathematics, most importantly, to algebraic topology (see Remark 1.4 below).
See also the Wikipedia article on knot theory and [Skopenkov2016t, Example 2.3], [Takase2006,  1].
1].
This article gives a short guide to the problem of classifying embeddings of closed manifolds  into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds
into Euclidean space or the sphere up to isotopy (i.e., to the Knotting Problem of Remark 1.1 for embeddings of general manifolds  into
into  or
or  ). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of
). After making some general remarks and giving references, in Section 2 we record some of the dimension ranges where no knotting is possible, i.e. where any two embeddings of  are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
are isotopic.In Section 3, we establish notation and conventions
and in Section 4, we continue by introducing the connected sum operation for embeddings.
We then make some remarks on codimension 2 embeddings in Section 5 and conclude with a brief review of some important results about codimension 1 embeddings in Section 6.
The most interesting and much studied case of embeddings concerns classical knots, which are embeddings 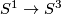 , or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and
, or more generally,
codimension 2 embeddings of spheres. Although there have been many wonderful results in this subject in the last 100 years, these results were not directly aiming at a complete classification, which remains wide open. Almost nothing is said here about this, but see Wikipedia article on knot theory and  5 for more information.
5 for more information.
The Knotting Problem is known to be hard. To the best of the author's knowledge, at the time of writing there are only a few cases in which readily calculable classification results (see Remark 1.2) describing all isotopy classes for embeddings of a closed manifold into Euclidean space  are known. Such classification results are presented on the pages listed in Remark 1.4, in
are known. Such classification results are presented on the pages listed in Remark 1.4, in  2 and in
2 and in  6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
6.
The statements of those results, although not the proofs, are simple and accessible to non-specialists.
This page and the pages listed in Remark 1.4 concern only such classification results.
As a consequence, we leave aside a large body of work, especially but not only in codimension 2.
The results and remarks given below show the following:
- For a fixed
 , the more
, the more  decreases from
decreases from  towards
towards 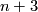 , the more complicated the classification of embeddings of
, the more complicated the classification of embeddings of  into
into  becomes.
becomes.
- The complete readily calculable classification of embeddings into
 of closed connected
of closed connected  -manifolds is non-trivial and presently accessible only for
-manifolds is non-trivial and presently accessible only for 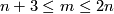 or for
or for 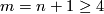 ; the lowest dimensional cases, i.e. all such pairs
; the lowest dimensional cases, i.e. all such pairs 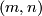 with
with 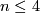 , are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
, are (6,3), (4,3), (8,4), (7,4), (5,4). For information on the cases (6,3), (8,4), (7,4) see [Skopenkov2016t], [Skopenkov2016e], [Skopenkov2016f].
Remark 1.2 (Readily calculable classification). The informal concept of `readily calculable (concrete) classification' is complementary to `abstract classification' or `reduction' as described by Wall (see (c) below). See [Graham&Knuth&Patashnik89, Preface] for discussion of similar issues. To a first approximation, a classification of embeddings 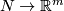 is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number
is `readily calculable' if it involves a 1-1 correspondence with a set or a group which is `easily' calculated from the given number  and the manifold
and the manifold  . This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
. This reflects the taste of the author and is intended to be used as informative but imprecise concept. Readers happy with this may skip the rest of this Remark. For other readers, we further illustrate our use of the term `readily calculable' with the following general remarks (a)-(d) and the more specific examples (i)-(iii). We feel that the advantages of the idea of `readily calculable' outweigh its imprecision. Here we describe this concept with only as much precision as sufficient to see why some classifications of embeddings are presented and some others are left aside.
Since this is a paper on the Knotting Problem of Remark 1.1, below `classification' means `classification of embeddings of a manifold  into
into  up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
up to isotopy' (except in (d); some comments like (a) apply for more general classification problems).
(a) An important feature of a readily calculable classification is the accessibility of the statement to a general mathematical audience, which may only be familiar with basic notions of the area; this in turn may be viewed as an approximation to beauty. Another important feature is whether the classification gives an algorithm to classify the object considered, and if so, how fast the algorithm is.
(b) In the above description of a readily calculable classification, the notion of `easily' depends upon how the manifold  is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
is presented. Here we assume that the manifold is equipped with a triangulation. Then a classification involving invariants which are `easily' calculated from the (co)homology of the manifold (with integral or finite cyclic coefficients), from basic extra structures on (co)homology (like the intersection product and characteristic classes), when they are known, is readily calculable. Since we do not specify the extra structures exhaustively, this explanation is open-ended. If the manifold is presented in a different way (e.g. by a system of equations), the integral homology may not be easy to calculate.
(c) The Browder-Casson-Haefliger-Sullivan-Wall theorem of 1960s gives necessary and sufficient conditions for embeddability of manifolds in codimension greater than 2 (in terms of Poincaré embeddings). See [Wall1999, Corollary 11.3.1] and a simpler exposition in [Cencelj&Repovs&Skopenkov2004, pp. 263-267]. `It is misleading to regard this as a complete solution to the problem of embeddings: the problems raised seem to the author in some respects to be harder than the original geometrical problems.' [Wall1999, p. 119]. An analogue of this result for classification is [Cencelj&Repovs&Skopenkov2004, the Browder-Wall Theorem 9]. The proof is likely to be obtained (at least for the smooth category) using surgery analogously to [Wall1999, Corollary 11.3.3] as exposed in [Cencelj&Repovs&Skopenkov2004, pp. 265-267]. However, the proof did not appear in the literature. So I regard this result as a conjecture and I apologize that it is stated as a theorem (I recognize that other mathematicians have different reliability standards and might call it a theorem). The above citation of Wall applies to this conjecture because the classifications provided by this conjecture are not readily calculable in general. On the other hand, some readily calculable classifications (see [Skopenkov2016t], [Skopenkov2016f]) have been obtained by applying Kreck's modified surgery theory, see (d).
(d) Here we consider the analogous problem of the classification of manifolds, see the Manifold Problem of Remark 1.1. Classical surgery theory as developed by Browder, Novikov, Sullivan and Wall, gives a procedure for classifying smooth manifolds. While this is a major achievement of 20th century topology, with many celebrated applications, it may or may not lead to readily calculable classification results for a given class of manifolds. One of the motivations for Kreck's modified surgery theory [Kreck1999] was to develop a surgery theory which produced readily calculable classifications of manifolds more frequently and easily than classical surgery. For references to such classifications see [Kreck1999].
(i) A reduction of a classification to calculation of standard objects of mathematics when these are known is typically a readily calculable classification. E.g. the Haefliger-Zeeman Theorem [Skopenkov2016h, Theorem 4.1] classifies links in terms of (stable) homotopy groups of spheres. These groups are not known in general. However, these groups are described for many particular cases which give the table [Skopenkov2016h,  1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
1], and homotopy groups of spheres is a `standard object' of mathematics. Thus for the cases when these groups are known, I regard this as a readily calculable classification.
(ii) The Haefliger-Weber Theorem classifies embeddings of manifolds (in a `metastable range') in terms of equivariant homotopy classes of certain equivariant maps from the `deleted product' of the manifold (defined to be the configuration space of distinct ordered pairs of points from the manifold). See survey [Skopenkov2006,  5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
5] and [Haefliger1963], [Weber1967], [Skopenkov2002]. Such equivariant homotopy classes have not been calculated in general. Moreover, the deleted product of a general manifold is a less `standard object' of mathematics (if at all) than the homotopy groups of spheres. Thus I regard the Haefliger-Weber classification not to be readily calculable in general. However, it is an important result and implies
- the Becker-Glover Theorem [Skopenkov2016e, Theorem 6.5] and the classification of knotted tori [Skopenkov2016k, Theorem 3.2], which I regard as readily calculable classifications;
- the independence of the classification of embeddings of the smooth or PL structure on the manifold;
- the existence of an algorithm recognizing PL isotopy of given PL embeddings (and of an algorithm recognizing PL embeddability of a PL manifold) [Cadek&Krcal&Vokrınek2013, Theorem 1.1 and text after Theorem 1.4].
(iii) There is an interesting approach of Goodwille and Weiss (the calculus of embeddings) to the Knotting Problem [Weiss96], [Weiss99], [Goodwillie&Weiss1999]. So far that approach has not lead to any new readily calculable classifications, but it gives a modern abstract proof of the Haefliger-Weber Theorem (see (ii)), and it gives explicit results on higher homotopy groups of the space of embeddings 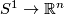 [Weiss], [Arone&Turchin2014].
[Weiss], [Arone&Turchin2014].
Remark 1.3 (Embeddings into Euclidean space and the sphere).
(a) The embeddings 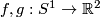 given by
given by 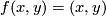 and
and 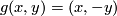 are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of
are not isotopic
(because they have distinct turning numbers; readers not familiar with turning number as defined in the Wikipedia article on turning number can accept this intuitively clear assertion without proof). On the other hand, any two embeddings of  into
into  are isotopic, see Theorem 6.1.a and below it.
are isotopic, see Theorem 6.1.a and below it.
(b) For  the classifications of embeddings of compact
the classifications of embeddings of compact  -manifolds into
-manifolds into  and into
and into  are the same. More precisely, for all integers
are the same. More precisely, for all integers  such that
such that  , and for every
, and for every  -manifold
-manifold  , the map
, the map 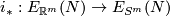 between the sets of isotopy classes of embeddings
between the sets of isotopy classes of embeddings 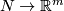 and
and 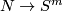 ,
which is induced by composition with the inclusion
,
which is induced by composition with the inclusion 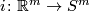 , is a bijection.
, is a bijection.
Let us prove part (b). Since 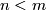 , after a small isotopy an embedding
, after a small isotopy an embedding  missed the point at infinity and so lies in
missed the point at infinity and so lies in 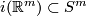 .
Hence
.
Hence  is onto.
To prove that
is onto.
To prove that  is injective, it suffices to show that if the compositions with the inclusion
is injective, it suffices to show that if the compositions with the inclusion 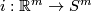 of two embeddings
of two embeddings 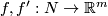 of a compact
of a compact  -manifold
-manifold  are isotopic, then
are isotopic, then  and
and  are isotopic. For showing that assume that
are isotopic. For showing that assume that 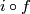 and
and  are isotopic. Then by general position
are isotopic. Then by general position  and
and  are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],
are non-ambiently isotopic. Since every non-ambient isotopy extends to an isotopy [Skopenkov2016i, Theorem 1.3],  and
and  are isotopic.
are isotopic.
Remark 1.4 (References to information on the classification of embeddings). The first list is structured by the dimension of the source manifold and the target Euclidean space:
Information structured by the `complexity' of the source manifold:
For more information see e.g. [Skopenkov2006].
2 Unknotting theorems
If the category is omitted, then a result stated below holds in both the smooth and piecewise-linear (PL) category.
General Position Theorem 2.1 ([Hirsch1976, Theorem 3.5], [Rourke&Sanderson1972, Theorem 5.4]).
For every compact  -manifold
-manifold  and
and 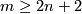 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
The case 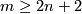 is called a `stable range' (for the classification problem; for the existence problem the analogous result with
is called a `stable range' (for the classification problem; for the existence problem the analogous result with 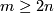 is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
is called
strong Whitney embedding theorem [Skopenkov2006, Theorem 2.2.a]).
The restriction 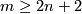 in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking
in Theorem 2.1 is sharp for non-connected manifolds, as the Hopf linking 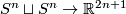 shows [Skopenkov2016h, Example 2.1].
shows [Skopenkov2016h, Example 2.1].
Whitney-Wu Unknotting Theorem 2.2.
For every compact connected  -manifold
-manifold  ,
, 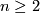 and
and 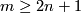 , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Wu1958c] using the Whitney trick (see that reference or [Rourke&Sanderson1972,  5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
5]). See also [Smale1961, Theorem 2.3 (H. Whitney, W.T. Wu)].
All the three assumptions in this result are indeed necessary:
- the assumption
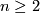 because of the existence of non-trivial knots
because of the existence of non-trivial knots 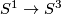 ;
;
- the connectedness assumption because of the existence of the Hopf link [Skopenkov2016h, Example 2.1];
- the assumption
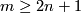 because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
because of the example of Hudson tori [Skopenkov2016e, Example 3.1].
Unknotting Spheres Theorem 2.3. Assume that  in the PL category or
in the PL category or 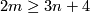 in the smooth category.
For
in the smooth category.
For 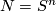 , or even for
, or even for  an integral homology
an integral homology  -sphere, any two embeddings of
-sphere, any two embeddings of  into
into  are isotopic.
are isotopic.
For 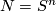 this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For
this result is proved in [Zeeman1960], [Zeeman1963, Corollary 2 of Theorem 9 in Chapter 4] and in [Haefliger1961, Existence Theorem (b) in p. 47] in the PL and in the smooth category, respectively. For  an integral homology
an integral homology  -sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for
-sphere this result follows from [Skopenkov2016e, Theorem 6.2].
This result is also true for  in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
in the topological locally flat category [Stallings1963, Corollary 9.3], [Gluck1963, Theorem 1.1]; see also textbook [Rushing1973, Flattening Theorem 4.5.1] and [Scharlemann1977, Corollary 1.3].
The case 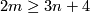 is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with
is called a `metastable range' (for the classification problem; for the existence problem there are analogous results with 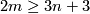 [Skopenkov2006,
[Skopenkov2006,  2,
2,  5]).
5]).
Knots in codimension 2 and the Haefliger trefoil knot [Skopenkov2016t, Example 2.1] show that the dimension restrictions are sharp (even for 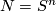 ) in the Unknotting Spheres Theorem 2.3.
) in the Unknotting Spheres Theorem 2.3.
Theorems 2.2 and 2.3 may be generalized as follows.
The Haefliger-Zeeman Unknotting Theorem 2.4.
For every 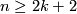 ,
, 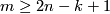 and closed
and closed  -connected
-connected  -manifold
-manifold  , any two embeddings of
, any two embeddings of  into
into  are isotopic.
are isotopic.
This is proved in [Zeeman1963, Corollary 2 of Theorem 24 in Chapter 8], [Haefliger1961, Existence Theorem (b) in p. 47] in the PL or in the smooth category, respectively. The PL case uses the ideas of [Penrose&Whitehead&Zeeman1961] and [Irwin1965]; see also textbooks [Hudson1969,  11], [Rourke&Sanderson1972,
11], [Rourke&Sanderson1972,  5]. Theorem 2.4 remains true if
5]. Theorem 2.4 remains true if  -connected is replaced by homologically
-connected is replaced by homologically  -connected (see
-connected (see  3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
3 for the definition and
[Skopenkov2016e, Theorem 6.2] for justification).
Note that if 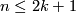 , then every closed
, then every closed  -connected
-connected  -manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
-manifold is topologically a sphere, so the analogue of Theorem 2.4 in the smooth category is wrong, and in the PL category gives nothing more than the Unknotting Spheres Theorem 2.3.
Given Theorem 2.4 above, the case 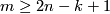 can be called a `stable range for
can be called a `stable range for  -connected manifolds'.
-connected manifolds'.
For generalizations of Theorem 2.4 see survey [Skopenkov2016e] and [Hudson1967], [Hacon1968], [Hudson1972], [Gordon1972], [Kearton1979]. See also Theorem 6.1.
3 Notation and conventions
The following notations and conventions will be used below in this page and also in some other pages about embeddings, including those listed in Remark 1.4.
Unless otherwise indicated, the word `isotopy' means `ambient isotopy'; see definition in [Skopenkov2016i,  1]. For a manifold
1]. For a manifold  let
let 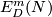 or
or 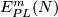 denote the set of
smooth or piecewise-linear (PL) embeddings
denote the set of
smooth or piecewise-linear (PL) embeddings 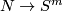 up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
up to smooth or PL isotopy.
If the category is omitted, then the result holds (or a definition or a construction is given) in both categories.
The sources of all embeddings are assumed to be compact.
Let  be a closed
be a closed  -ball in a closed connected
-ball in a closed connected  -manifold
-manifold  . Denote
. Denote 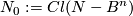 .
.
Let 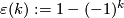 be
be  for
for  even and
even and  for
for  odd, so that
odd, so that 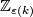 is
is  for
for  even and
even and  for
for  odd.
odd.
Tex syntax erroris the projection of a Cartesian product onto the
 th factor.
th factor.
Denote by 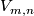 the Stiefel manifold of orthonormal
the Stiefel manifold of orthonormal  -frames in
-frames in  .
.
We omit  -coefficients from the notation of (co)homology groups.
-coefficients from the notation of (co)homology groups.
For a manifold  with boundary
with boundary 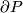 denote
denote 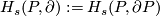 .
.
A closed manifold  is called homologically
is called homologically  -connected, if
-connected, if  is connected and
is connected and 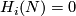 for every
for every 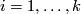 .
This condition is equivalent to
.
This condition is equivalent to 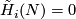 for each
for each 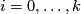 , where
, where  are reduced homology groups.
A pair
are reduced homology groups.
A pair 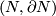 is called homologically
is called homologically  -connected, if
-connected, if  for every
for every 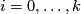 .
.
The self-intersection set of a map 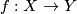 is
is 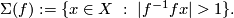
For a smooth embedding  denote by
denote by
-
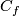 the closure of the complement in
the closure of the complement in 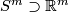 to a tight enough tubular neighborhood of
to a tight enough tubular neighborhood of  and
and
-
 the restriction of the linear normal bundle of
the restriction of the linear normal bundle of  to the subspace of unit length vectors identified with
to the subspace of unit length vectors identified with 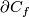 .
.
Tex syntax errorthe standard embedding given by
Tex syntax error. The natural normal framing by vectors of length 1/2 on
Tex syntax errordefines the standard embedding
Tex syntax error. Denote by the same symbol
Tex syntax errorthe restriction of
Tex syntax errorto
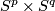 for any
for any 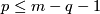 .
.
Denote by 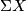 the suspension of a space
the suspension of a space  . Denote by
. Denote by  the suspension homomorphism. Recall that
the suspension homomorphism. Recall that  is an isomorphism for
is an isomorphism for 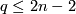 . Let
. Let 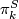 be any of the groups
be any of the groups 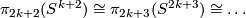 identified by the suspension isomorphism.
Denote by
identified by the suspension isomorphism.
Denote by 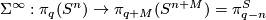 the stable suspension homomorphisms, where
the stable suspension homomorphisms, where  is large.
is large.
4 Embedded connected sum
Suppose that  ,
,  is a closed connected
is a closed connected  -manifold and, if
-manifold and, if  is orientable, an orientation of
is orientable, an orientation of  is chosen.
Let us define the embedded connected sum operation
is chosen.
Let us define the embedded connected sum operation  of
of  on
on  .
.
Represent isotopy classes ![[f]\in E^m(N)](/images/math/2/f/5/2f52f1701a659817e8b3dfbab85d0fef.png) and
and ![[g]\in E^m(S^n)](/images/math/0/0/3/003b3d9600f355a6b75926b59ee4b4c6.png) by embeddings
by embeddings  and
and 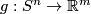 whose images are contained in disjoint balls.
Join the images of
whose images are contained in disjoint balls.
Join the images of 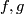 by an arc whose interior misses the images.
Let
by an arc whose interior misses the images.
Let ![[f]\#[g]](/images/math/b/5/0/b50485694938d441de43bc8d0d73e64c.png) be the isotopy class of the embedded connected sum of
be the isotopy class of the embedded connected sum of  and
and  along this arc (compatible with the orientation, if
along this arc (compatible with the orientation, if  is orientable), cf. [Haefliger1966, 1.3, 1.4].
is orientable), cf. [Haefliger1966, 1.3, 1.4].
This operation is well-defined,
i.e. the isotopy class of the embedded connected sum depends only on the the isotopy classes ![[f]](/images/math/d/d/4/dd43b82529dd8d403c1585c5d151a163.png) and
and ![[g]](/images/math/0/8/a/08a05aeade465b1c6abe241850947aab.png) , and is independent of the choice of the path and of the representatives
, and is independent of the choice of the path and of the representatives 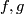 .
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for
.
The proof of this fact is based on the construction of embedded connected sum of isotopies.
Although this fact is presumably classical, a proof was not written, cf. [Skopenkov2015a, Remark 2.3.a].
The proof is written for 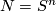 in [Skopenkov2015a,
in [Skopenkov2015a,  3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for
3, proof of the Standardization Lemma 2.1.b and beginning of proof of the Group structure Lemma 2.2 for  a point].
The proof for arbitrary closed connected
a point].
The proof for arbitrary closed connected  -manifold
-manifold  is analogous.
is analogous.
Moreover, for  embedded connected sum defines a group structure on
embedded connected sum defines a group structure on  [Haefliger1966, 1.3-1.7], and an action
[Haefliger1966, 1.3-1.7], and an action  of
of  on
on  .
.
5 Some remarks on codimension 2 embeddings
The case of embeddings of  into
into 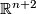 is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
is the most extensively studied case of the Knotting Problem.
In this case there is an overwhelming multitude of isotopy classes of embeddings.
We present some speculations as to why the classification in codimension 2 should not be accessible for manifolds, not only for spheres.
Let  be a closed connected
be a closed connected  -manifold.
Using embedded connected sum (
-manifold.
Using embedded connected sum ( 4) we can apparently produce an overwhelming multitude of embeddings
4) we can apparently produce an overwhelming multitude of embeddings 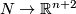 from the overwhelming multitude of embeddings
from the overwhelming multitude of embeddings  .
(However, note that for
.
(However, note that for  there are embeddings
there are embeddings 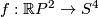 and
and 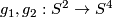 such that
such that 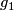 is not isotopic to
is not isotopic to 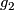 but
but 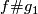 is isotopic to
is isotopic to 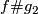 [Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928]
[Viro1973, 22 in p. 181].)
One can also apply Artin's spinning construction [Artin1928] 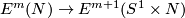 for
for 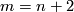 .
Thus the description of
.
Thus the description of 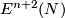 is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
is a very hard open problem.
It would be interesting to give a more formal (e.g. algorithmic)
illustration of the hardness of this problem.
For studies of codimension 2 embeddings of manifolds up to the weaker relation of concordance see e.g. [Cappell&Shaneson1974].
6 Codimension 1 embeddings
Theorem 6.1.
(a) Any two smooth embeddings of  into
into 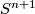 are smoothly isotopic for every
are smoothly isotopic for every  .
.
(b) Any two smooth embeddings of 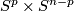 into
into 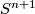 are smoothly isotopic for every
are smoothly isotopic for every 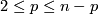 .
.
Part (a) is proved in [Milnor1965a, Proposition D in  9] as an easy corollary of the
9] as an easy corollary of the  -cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,
-cobordism theorem.
Part (b) is proved in [Lucas&Saeki2002,  1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
1, p. 447] as a corollary of generalizations [Kosinski1961], [Wall1965], [Goldstein1967], [Rubinstein1980], [Lucas&Neto&Saeki1996] of the following result.
Theorem 6.2 (Alexander torus theorem). [Alexander1924]
For every embedding 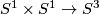 there is an autohomeomorphism
there is an autohomeomorphism  of
of 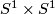 such that
such that  extends to an embedding
extends to an embedding 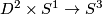 .
.
Clearly, only the standard embedding extends to both.
Remark 6.3. (a) (on PL topological category) The analogue of Theorem 6.1.a holds
- for
 in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,
in the PL or topological category (Schöenfliess Theorem, 1912) [Rushing1973,  1.8].
1.8].
- for
 in the PL category (Alexander Theorem, 1923) [Rushing1973,
in the PL category (Alexander Theorem, 1923) [Rushing1973,  1.8].
1.8].
- for every
 in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
in the topological locally flat category (Brown-Mazur-Moise Theorem, 1960) [Rushing1973, Generalized Schöenfliess Theorem 1.8.2].
The famous counterexample to the analogue of Theorem 6.1.a for  in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of
in the topological category is the Alexander horned sphere, see the corresponding Wikipedia article. The well-known very hard Schöenfliess Problem asks whether any two PL embeddings of  into
into 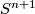 are isotopic for every
are isotopic for every  (this is equivalent to the description of
(this is equivalent to the description of 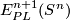 ).
).
(b) (some speculations) If the extension of the Alexander torus theorem 6.2 respects isotopy, the extension would give a 1-1 correspondence between 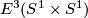 and the union of
and the union of 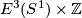 and
and 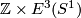 with `base points'
with `base points' 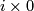 and
and  identified (where
identified (where  is the isotopy class of the standard inclusion
is the isotopy class of the standard inclusion 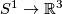 ). So the description of
). So the description of 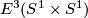 would be as hopeless as that of
would be as hopeless as that of 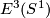 . Thus the description of
. Thus the description of 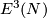 for
for  a sphere with handles is apparently hopeless.
a sphere with handles is apparently hopeless.
7 References
- [Alexander1924] J. W. Alexander, On the subdivision of 3-space by polyhedron, Proc. Nat. Acad. Sci. USA, 10, (1924) 6–8. Zbl 50.0659.01
- [Arone&Turchin2014] G. Arone and V. Turchin, On the rational homology of high-dimensional analogues of spaces of long knots, Geom. and Topol. 18 (2014), 1261-1322.
- [Artin1928] E. Artin, Zur Isotopie zwei-dimensionaler Flaechen im
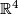 , Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
, Abh. Math. Sem. Hamburg Univ. 4 (1928) 174–177.
- [Cadek&Krcal&Vokrınek2013] M. Cadek, M. Kr\v c\'al, and L. Vok\v r\'ınek. Algorithmic solvability of the lifting-extension problem, arXiv:1307.6444.
- [Cappell&Shaneson1974] S. E. Cappell and J. L. Shaneson, The codimension two placement problem and homology equivalent manifolds, Ann. of Math. (2) 99 (1974), 277–348. MR0339216 (49 #3978) Zbl 0279.57011
- [Cencelj&Repovs&Skopenkov2004] M. Cencelj, D. Repov\v s and A. Skopenkov, On the Browder-Levine-Novikov embedding theorems, Proc. of the Steklov Math. Inst. 247 (2004) 280-290.
- [Gluck1963] H. Gluck, Unknotting
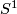 in
in 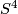 ., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
., Bull. Am. Math. Soc. 69 (1963), 91-94. MR0142114 (25 #5507) Zbl 0108.36503
- [Goldstein1967] R. Z. Goldstein, Piecewise linear unknotting of
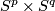 in
in 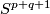 , Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
, Michigan Math. J. 14 (1967), 405–415. MR0220299 (36 #3365) Zbl 0157.54801
- [Goodwillie&Weiss1999] T. Goodwillie and M. Weiss, Embeddings from the point of view of immersion theory, II, Geometry and Topology, 3 (1999), 103-118. MR1694812 (2000c:57055a) Zbl 0927.57028
- [Gordon1972] C. Gordon, Embedding piecewise linear manifolds with boundary., Proc. Camb. Philos. Soc. 72 (1972), 21-25. MR0295359 (45 #4425) Zbl 0236.57009
- [Graham&Knuth&Patashnik89] R. Graham, D. Knuth, and O. Patashnik, Concrete Mathematics: A Foundation for Computer Science, Addison–Wesley, first published in 1989, ISBN 0-201-55802-5, https://www.csie.ntu.edu.tw/~r97002/temp/Concrete%20Mathematics%202e.pdf.
- [Hacon1968] D. Hacon, Embeddings of
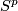 in
in 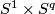 in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
in the metastable range., Topology 7 (1968), 1-10. MR0222903 (36 #5953) Zbl 0153.53902
- [Haefliger1961] A. Haefliger, Plongements différentiables de variétés dans variétés., Comment. Math. Helv.36 (1961), 47-82. MR0145538 (26 #3069) Zbl 0102.38603
- [Haefliger1963] A. Haefliger, Plongements différentiables dans le domain stable., Comment. Math. Helv.37 (1963), 155-176.
- [Haefliger1966] A. Haefliger, Differential embeddings of
 in
in  for
for  , Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
- [Hirsch1976] M. W. Hirsch, Differential topology., Graduate Texts in Mathematics, No. 33. Springer-Verlag., New York-Heidelberg, 1976. MR0448362 (56 #6669) Zbl 0356.57001
- [Hudson1967] J. Hudson, Piecewise linear embeddings, Ann. of Math. (2) 85 (1967) 1–31. MR0215308 (35 #6149) Zbl 0153.25601
- [Hudson1969] J. F. P. Hudson, Piecewise linear topology, W. A. Benjamin, Inc., New York-Amsterdam, 1969. MR0248844 (40 #2094) Zbl 0189.54507
- [Hudson1972] J. Hudson, Embeddings of bounded manifolds., Proc. Camb. Philos. Soc. 72 (1972), 11-20. MR0298679 (45 #7728) Zbl 0241.57006
- [Irwin1965] M. Irwin, Embeddings of polyhedral manifolds, Ann. of Math. (2) 82 (1965) 1–14. MR0182978 (32 #460) Zbl 0132.20003
- [Kearton1979] C. Kearton, Obstructions to embedding and isotopy in the metastable range, Math. Ann. 243 (1979), 103-113. MR0543720 (82k:57012) Zbl 0401.57033
- [Kosinski1961] A. Kosinski, On Alexander's theorem and knotted tori, In: Topology of 3-Manifolds, Prentice-Hall, Englewood Cliffs, Ed. M.~K.~Fort, N.J., 1962, 55--57. Cf. Fort1962.
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
- [Lucas&Neto&Saeki1996] L. A. Lucas, O. M. Neto and O. Saeki, A generalization of Alexander's torus theorem to higher dimensions and an unknotting theorem for
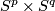 embedded in
embedded in  , Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
, Kobe J. Math. 13 (1996), no.2, 145–165. MR1442202 (98e:57041) Zbl 876.57045
- [Lucas&Saeki2002] L. A. Lucas and O. Saeki, Embeddings of
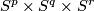 in
in 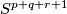 , Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
, Pacific J. Math. 207 (2002), no.2, 447–462. MR1972255 (2004c:57045) Zbl 1058.57022
- [Milnor1965a] J. Milnor, Lectures on the
 -cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
-cobordism theorem, Princeton University Press, Princeton, N.J., 1965. MR0190942 (32 #8352) Zbl 0161.20302
- [Penrose&Whitehead&Zeeman1961] R. Penrose, J. Whitehead and E. Zeeman, Imbedding of manifolds in Euclidean space., Ann. of Math. 73 (1961) 613–623. MR0124909 (23 #A2218) Zbl 0113.38101
- [Rourke&Sanderson1972] C. Rourke and B. Sanderson, Introduction to piecewise-linear topology., Ergebnisse der Mathematik und ihrer Grenzgebiete. Band 69. Berlin-Heidelberg-New York: Springer-Verlag. VIII, 1972. MR0665919 (83g:57009) Zbl 0254.57010
- [Rubinstein1980] J.H. Rubinstein, Dehn’s lemma and handle decompositions of some 4-manifolds, Pacific J. Math., 86 (1980), 565-569. MR590570 (81 #M57011) Zbl 0446.57025
- [Rushing1973] T. Rushing, Topological embeddings., Pure and Applied Mathematics, 52. New York-London: Academic Press. XIII, 1973. MRMR0348752 (50 #1247) Zbl 0295.57003
- [Scharlemann1977] M. Scharlemann, Isotopy and cobordism of homology spheres in spheres., J. Lond. Math. Soc., II. Ser. 16 (1977), 559-567. MRMR0464246 (57 #4180) Zbl 0375.57003
- [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015a] A. Skopenkov, A classification of knotted tori, Proc. A of the Royal Society of Edinburgh, 150:2 (2020), 549-567. Full version: http://arxiv.org/abs/1502.04470
- [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
- [Skopenkov2016f] A. Skopenkov, 4-manifolds in 7-space, to appear in Bull. Man. Atl.
- [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
- [Skopenkov2016i] A. Skopenkov, Isotopy, submitted to Bull. Man. Atl.
- [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
- [Skopenkov2016s] A. Skopenkov, Knots, i.e. embeddings of spheres, preprint.
- [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.
- [Smale1961] S. Smale, Generalized Poincaré's conjecture in dimensions greater than four, Ann. of Math. (2) 74 (1961), 391–406. MR0137124 (25 #580) Zbl 0099.39202
- [Stallings1963] J. Stallings, On topologically unknotted spheres., (1963). MRMR0149458 (26 #6946) Zbl 0121.18202
- [Takase2006] M. Takase, Homology 3-spheres in codimension three, Internat. J. Math. 17 (2006), no.8, 869–885.
arxiv:math/0506464 MR2261638 (2007g:57049) Zbl 1113.57013
- [Viro1973] O. J. Viro, Local knotting of sub-manifolds, Mat. Sb. (N.S.) 90(132) (1973), 173–183, 325. MR0370606 (51 #6833)
- [Wall1965] C. T. C. Wall, Unknotting tori in codimension one and spheres in codimension two., Proc. Camb. Philos. Soc. 61 (1965), 659-664. MR0184249 (32 #1722) Zbl 0135.41602
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
- [Weber1967] C. Weber, Plongements de polyedres dans le domaine metastable, Comment. Math. Helv. 42 (1967), 1-27. MR0238330 (38 #6606) Zbl 0152.22402
- [Weiss] M. Weiss, private communication
- [Weiss96] M. Weiss, Calculus of Embeddings, Bull. Amer. Math. Soc. 33 (1996), 177-187.
- [Weiss99] M. Weiss, Embeddings from the point of view of immersion theory, I, Geom. and Topology 3 (1999), 67-101. Erratum in Geom. and Topology 15, 2011.
- [Wu1958c] W. Wu, On the isotopy of
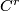 -manifolds of dimension
-manifolds of dimension  in Euclidean
in Euclidean 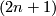 -space, Science Records, New Ser. Vol. II (1958), 271–275.
-space, Science Records, New Ser. Vol. II (1958), 271–275.
- [Zeeman1960] E. Zeeman, Unknotting spheres in five dimensions, Bull. Amer. Math. Soc. 66 (1960) 198. MR0117737 (22 #8512a) Zbl 0117.40904
- [Zeeman1963] E. C. Zeeman, Seminar on Combinatorial Topology, IHES, 1963 (revised 1966).
- [Zeeman1993] E.C. Zeeman, A Brief History of Topology, UC Berkeley, October 27, 1993, On the occasion of Moe Hirsch's 60th birthday, http://zakuski.utsa.edu/~gokhman/ecz/hirsch60.pdf