Stiefel-Whitney characteristic classes
Askopenkov (Talk | contribs) |
|||
| Line 7: | Line 7: | ||
Let $G_k=G_{k,N}$ be $\Zz$ if either $k=n$ or $k>1$ is odd, and $\Zz_2$ if either $k=1$ or $k<n$ is even. | Let $G_k=G_{k,N}$ be $\Zz$ if either $k=n$ or $k>1$ is odd, and $\Zz_2$ if either $k=1$ or $k<n$ is even. | ||
| − | Stiefel defined ''the homology Stiefel-Whitney class'' $PDW_k(N)\in H_{n-k}(N,\partial N;G_k)$ of $N$ to be the homology class of a degeneracy subset of a general position collection of $n+1-k$ ''tangent'' vector fields on $N$ | + | Stiefel defined ''the homology Stiefel-Whitney class'' $PDW_k(N)\in H_{n-k}(N,\partial N;G_k)$ of $N$ to be the homology class of a degeneracy subset of a general position collection of $n+1-k$ ''tangent'' vector fields on $N$. |
| − | Whitney defined ''the homology normal Stiefel-Whitney class'' $PD\overline{W}_k(N)\in H_{n-k}(N,\partial N;G_k)$ of $N$ to be the homology class of a degeneracy subset of a general position collection of $n+1-k$ ''normal'' vector fields on $N$. Let $\overline{w}_k(N)$ be the | + | Whitney defined ''the homology normal Stiefel-Whitney class'' $PD\overline{W}_k(N)\in H_{n-k}(N,\partial N;G_k)$ of $N$ to be the homology class of a degeneracy subset of a general position collection of $n+1-k$ ''normal'' vector fields on $N$. |
| + | |||
| + | Let $w_k(N)$ and $\overline{w}_k(N)$ be the reductions of $W_k(N)$ and $\overline{W}_k(N)$ modulo 2. | ||
| + | |||
| + | Let $w_0(N)=\overline w_0(N)=[N]$. | ||
See details e.g. in \cite[$\S$12]{Milnor&Stasheff1974}, \cite[$\S$19.C]{Fomenko&Fuchs2016}, \cite[$\S\S$ 9,11,12]{Skopenkov2015b}. | See details e.g. in \cite[$\S$12]{Milnor&Stasheff1974}, \cite[$\S$19.C]{Fomenko&Fuchs2016}, \cite[$\S\S$ 9,11,12]{Skopenkov2015b}. | ||
| Line 16: | Line 20: | ||
See also [[wikipedia:Stiefel–Whitney_class|Wikipedia article]]. | See also [[wikipedia:Stiefel–Whitney_class|Wikipedia article]]. | ||
| + | </wikitex> | ||
| + | |||
| + | == The Wu formula == | ||
| + | <wikitex>; | ||
| + | |||
| + | |||
| + | |||
| + | {{beginthm|Theorem|(Wu formula)}}\label{t:wu} | ||
| + | If $N$ is a closed smooth $n$-manifold, $f:N\to\R^m$ a smooth immersion and $k>0$ an integer, then | ||
| + | $$\sum_{i=0}^{k}\overline w_{k-i}(f)\cap w_i(N)=0$$. | ||
| + | |||
| + | \begin{proof} | ||
| + | (This proof should be known but is, in this short and explicit form, absent from textbooks. This text is written by M. Fedorov and A. Skopenkov in frame of the course `Algorithms for recognition of realizability of hypergraphs'.) | ||
| + | |||
| + | Denote by $x_k(f)$ the obstruction to existence of $m-k+1$ linearly independent fields on $f(N)$. Clearly $x_k(N)=0$. Let us show that $x_k(f) = \sum_{i=0}^{k}\overline w_{k-i}(f)\cap w_i(N)$. | ||
| + | |||
| + | Take general position collection of normal fields $u_1,\ldots,u_{m-n}$ on $f(N)$ such that for each $i=1,\ldots,k$ the collection $u_1,\ldots,u_{m-n-i+1}$ is linearly dependent on $n-i$ subcomplex $\omega^*_i$ representing $\overline w_{i} = [\omega^*_i]$. | ||
| + | |||
| + | Take general position collection of tangent fields $v_1,\ldots,v_n$ on $f(N)$ such that for each $i=1,\ldots,k$ the collection $u_i,\ldots,u_n$ is linearly dependent on $n-i$ subcomplex $\omega_i$ representing $w_{i} = [\omega_i]$. | ||
| + | |||
| + | Now take the following collection of $m-k$ vector fields on $f(N)$ | ||
| + | $$C=u_1, \ldots u_{m-n-k+1}, \alpha_{m-n-k+2}u_{m-n-k+2} + \beta_1v_1,\ldots,\alpha_{m-n}u_{m-n}+\beta_{k-1}v_{k-1}, v_k,\ldots,v_n,$$ | ||
| + | where $\alpha_i=\mathrm{vol}(u_1,\ldots,u_i)$ and $\beta_i = \mathrm{vol}(v_i, \ldots v_n)$. | ||
| + | |||
| + | Thus all non-zero vectors among $\alpha_1u_1,\ldots,\alpha_{m-n}u_{m-n},\beta_1v_1,\ldots,\beta_nv_n$ are linearly independent. Hence collection $C$ is linearly dependent if and only if $C$ contains zero vector or $u_1,\ldots,u_{m-n-k+1}$ are linearly dependent or $v_k,\ldots,v_n$ are linearly dependent. But $C$ contains a zero vector if and only if there exist $j$ such that $\alpha_{m-n-k+1+j} = \beta_j=0$. | ||
| + | This means $C$ is linearly dependent on $\bigcup_{i=0}^{k}(\omega^*_{k-i}\cap\omega_i)$. | ||
</wikitex> | </wikitex> | ||
Revision as of 15:23, 14 February 2021
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Definition
Let  be a compact smooth
be a compact smooth  -manifold (possibly with boundary).
Denote by
-manifold (possibly with boundary).
Denote by  the Poincaré duality isomorphism.
Here for non-orientable
the Poincaré duality isomorphism.
Here for non-orientable  the coefficients in cohomology are twisted (by the orientation double covering) and the coefficients in homology are non-twisted.
Let
the coefficients in cohomology are twisted (by the orientation double covering) and the coefficients in homology are non-twisted.
Let 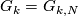 be
be  if either
if either 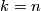 or
or  is odd, and
is odd, and  if either
if either 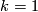 or
or  is even.
is even.
Stiefel defined the homology Stiefel-Whitney class 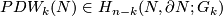 of
of  to be the homology class of a degeneracy subset of a general position collection of
to be the homology class of a degeneracy subset of a general position collection of 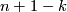 tangent vector fields on
tangent vector fields on  .
.
Whitney defined the homology normal Stiefel-Whitney class 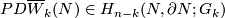 of
of  to be the homology class of a degeneracy subset of a general position collection of
to be the homology class of a degeneracy subset of a general position collection of 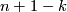 normal vector fields on
normal vector fields on  .
.
Let 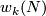 and
and 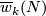 be the reductions of
be the reductions of 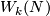 and
and 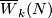 modulo 2.
modulo 2.
Let ![w_0(N)=\overline w_0(N)=[N]](/images/math/8/7/4/874e8a8d6533883a2bdaf04e70bf9ad8.png) .
.
See details e.g. in [Milnor&Stasheff1974,  12], [Fomenko&Fuchs2016,
12], [Fomenko&Fuchs2016,  19.C], [Skopenkov2015b,
19.C], [Skopenkov2015b, 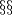 9,11,12].
9,11,12].
There is an alternative definition of 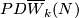 [Skopenkov2006,
[Skopenkov2006,  2.3 `the Whitney obstruction'] analogous to definition of the Whitney invariant.
2.3 `the Whitney obstruction'] analogous to definition of the Whitney invariant.
See also Wikipedia article.
2 The Wu formula
Theorem 2.1 (Wu formula).
If  is a closed smooth
is a closed smooth  -manifold,
-manifold,  a smooth immersion and
a smooth immersion and 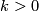 an integer, then
an integer, then
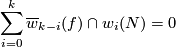
\begin{proof} (This proof should be known but is, in this short and explicit form, absent from textbooks. This text is written by M. Fedorov and A. Skopenkov in frame of the course `Algorithms for recognition of realizability of hypergraphs'.)
Denote by 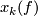 the obstruction to existence of
the obstruction to existence of 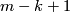 linearly independent fields on
linearly independent fields on  . Clearly
. Clearly 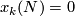 . Let us show that
. Let us show that 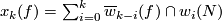 .
.
Take general position collection of normal fields 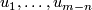 on
on  such that for each
such that for each 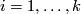 the collection
the collection  is linearly dependent on
is linearly dependent on  subcomplex
subcomplex 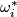 representing
representing ![\overline w_{i} = [\omega^*_i]](/images/math/1/8/8/188da5e1fd71b18e0d9d91d46144219d.png) .
.
Take general position collection of tangent fields 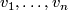 on
on  such that for each
such that for each 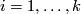 the collection
the collection 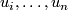 is linearly dependent on
is linearly dependent on  subcomplex
subcomplex 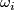 representing
representing ![w_{i} = [\omega_i]](/images/math/f/5/a/f5aa60a63175259233c81f7d4c3e370c.png) .
.
Now take the following collection of 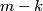 vector fields on
vector fields on 
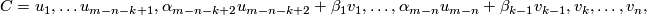
where  and
and 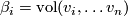 .
.
Thus all non-zero vectors among 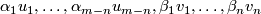 are linearly independent. Hence collection
are linearly independent. Hence collection  is linearly dependent if and only if
is linearly dependent if and only if  contains zero vector or
contains zero vector or 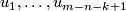 are linearly dependent or
are linearly dependent or 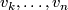 are linearly dependent. But
are linearly dependent. But  contains a zero vector if and only if there exist
contains a zero vector if and only if there exist  such that
such that 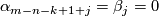 .
.
 is linearly dependent on
is linearly dependent on 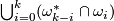 .
.
3 References
- [Fomenko&Fuchs2016] A. T. Fomenko and D. B. Fuks, Homotopical Topology. Translated from the Russian. Graduate Texts in Mathematics, 273. Springer-Verlag, Berlin, 2016. DOI 10.1007/978-3-319-23488-5.
- [Milnor&Stasheff1974] J. W. Milnor and J. D. Stasheff, Characteristic classes, Princeton University Press, Princeton, N. J., 1974. MR0440554 (55 #13428) Zbl 1079.57504
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015b] A. Skopenkov, Algebraic Topology From Geometric Viewpoint (in Russian), MCCME, Moscow, 2015, 2020. Accepted for English translation by `Moscow Lecture Notes' series of Springer. Preprint of a part