Middle-dimensional surgery kernel (Ex)
m |
m |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
The goal of this exercise is to prove the following statement which will be indispensible in defining the surgery obstruction. | The goal of this exercise is to prove the following statement which will be indispensible in defining the surgery obstruction. | ||
| − | {{beginthm|Proposition}} Let $f:M\rightarrow X$ be a degree 1 normal map from a $2k$-dimensional (resp. $(2k+1)$-dimensional manifold to a geometric Poincaré complex, inducing the isomorphism $f_\ast:\pi_1(M)\cong\pi_1(X)=:\pi$. Denote by $K_i(M)=K_i(\widetilde{M})$ the homology surgery kernel $\mathbb{Z}[\pi]$-module. If $f$ is $k$-connected the kernel module $K_k(M)$ is finitely generated and stably free. | + | {{beginthm|Proposition}} Let $f:M\rightarrow X$ be a degree 1 normal map from a $2k$-dimensional (resp. $(2k+1)$-dimensional) manifold to a geometric Poincaré complex, inducing the isomorphism $f_\ast:\pi_1(M)\cong\pi_1(X)=:\pi$. Denote by $K_i(M)=K_i(\widetilde{M})$ the homology surgery kernel $\mathbb{Z}[\pi]$-module. If $f$ is $k$-connected the kernel module $K_k(M)$ is finitely generated and stably free. |
{{endthm|Proposition}} | {{endthm|Proposition}} | ||
The statement essentially follows from the technical lemma which you are asked to prove. | The statement essentially follows from the technical lemma which you are asked to prove. | ||
| Line 12: | Line 12: | ||
| − | '''2)''' If in addition for $j>n$, $H^j(C)=0$ for the same integer $n$ then | + | '''2)''' If in addition for $j>n$, $H^j(C)=0$ for the same integer $n$ then there are isomorphisms$$H_n(C)\oplus\sum_{i\in\mathbb{Z}}C_{n+2i+1}\cong\sum_{j\in\mathbb{Z}}C_{n+2j}, $$$$H^n(C)\oplus\sum_{i\in\mathbb{Z}}C^{n+2i+1}\cong\sum_{j\in\mathbb{Z}}C^{n+2j},$$where under further assumption that $C$ is a chain complex of free modules, the latter isomorphism implies that $H_n(C)$ and $H^n(C)$ are stably free and hence $H_n(C)$ and $H^n(C)$ are dual. |
{{endthm|Lemma}} | {{endthm|Lemma}} | ||
| Line 18: | Line 18: | ||
</wikitex> | </wikitex> | ||
[[Category:Exercises]] | [[Category:Exercises]] | ||
| + | [[Category:Exercises without solution]] | ||
Latest revision as of 14:51, 1 April 2012
The goal of this exercise is to prove the following statement which will be indispensible in defining the surgery obstruction.
Proposition 0.1. Let 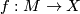 be a degree 1 normal map from a
be a degree 1 normal map from a  -dimensional (resp.
-dimensional (resp. 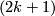 -dimensional) manifold to a geometric Poincaré complex, inducing the isomorphism
-dimensional) manifold to a geometric Poincaré complex, inducing the isomorphism 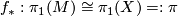 . Denote by
. Denote by 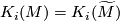 the homology surgery kernel
the homology surgery kernel ![\mathbb{Z}[\pi]](/images/math/5/3/9/5393263cfc1a4eb9ce95b62982af4354.png) -module. If
-module. If  is
is  -connected the kernel module
-connected the kernel module 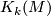 is finitely generated and stably free.
is finitely generated and stably free.
The statement essentially follows from the technical lemma which you are asked to prove.
Lemma 0.2 [Ranicki2002, Lemma 10.26].
Let  be a ring with involution and
be a ring with involution and 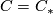 a finite chain complex of finitely generated projective (left)
a finite chain complex of finitely generated projective (left)  -modules.
-modules.
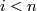 ,
, 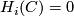 for some integer
for some integer  then the
then the  -module
-module 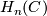 is finitely generated and
is finitely generated and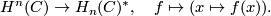
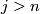 ,
, 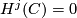 for the same integer
for the same integer  then there are isomorphisms
then there are isomorphisms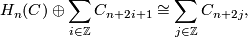

 is a chain complex of free modules, the latter isomorphism implies that
is a chain complex of free modules, the latter isomorphism implies that 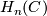 and
and 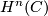 are stably free and hence
are stably free and hence 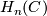 and
and 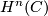 are dual.
are dual.
The proposition is given as lemma 4.19 in [Lück2001], however the proof is incomplete. Alternatively a good proof can be found in [Wall1999] and a more detailed one in [Ranicki2002].