Knots, i.e. embeddings of spheres
Askopenkov (Talk | contribs) (→Codimension 2 knots) |
Askopenkov (Talk | contribs) (→Codimension 2 knots) |
||
| Line 28: | Line 28: | ||
<wikitex>; | <wikitex>; | ||
| − | There is a vast literature on | + | A description of $E^3(S^1)$ and, more generally, of $E^{n+2}(S^n)$ is a well-known very hard open problem. |
| + | |||
| + | There is a vast literature on codimension 2 knots, most of which does not present a readily calculable classification (in the sense of | ||
[[Embeddings_in_Euclidean_space:_an_introduction_to_their_classification#Introduction|Remark 1.1]] of \cite{Skopenkov2016c}). | [[Embeddings_in_Euclidean_space:_an_introduction_to_their_classification#Introduction|Remark 1.1]] of \cite{Skopenkov2016c}). | ||
See e.g. interesting papers \cite{Farber1981}, \cite{Farber1983}, \cite{Kearton1983}, \cite{Farber1984}. | See e.g. interesting papers \cite{Farber1981}, \cite{Farber1983}, \cite{Kearton1983}, \cite{Farber1984}. | ||
Revision as of 12:07, 1 September 2017
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
See general introduction on embeddings, notation and conventions in [Skopenkov2016c,  1,
1,  2].
2].
2 Examples
Analogously to the Haefliger trefoil knot for  one constructs a smooth embedding
one constructs a smooth embedding 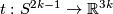 .
For
.
For  even this embedding is a generator of
even this embedding is a generator of 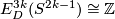 ; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for
; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for  odd this embedding is a generator of
odd this embedding is a generator of 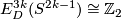 .
The last phrase of [Haefliger1962t] suggests that this is true for
.
The last phrase of [Haefliger1962t] suggests that this is true for 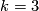 .
.
3 Readily calculable classification
For  the group
the group  has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs
has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs 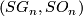 for
for 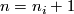 and
and 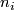 [Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here
[Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here 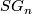 is the space of maps
is the space of maps 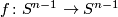 of degree
of degree  .
Restricting an element of
.
Restricting an element of 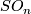 to
to 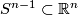 identifies
identifies 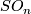 as a subspace of
as a subspace of 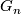 .
.
Some readily calculable corollaries of this classification are recalled in [Skopenkov2006,  3.3].
3.3].
4 Codimension 2 knots
A description of 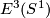 and, more generally, of
and, more generally, of 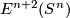 is a well-known very hard open problem.
is a well-known very hard open problem.
There is a vast literature on codimension 2 knots, most of which does not present a readily calculable classification (in the sense of Remark 1.1 of [Skopenkov2016c]). See e.g. interesting papers [Farber1981], [Farber1983], [Kearton1983], [Farber1984].
5 References
- [Farber1981] M. Sh. Farber, Classification of stable fibered knots, Mat. Sb. (N.S.), 115(157):2(6) (1981) 223–262.
- [Farber1983] M. Sh. Farber, The classification of simple knots, Russian Math. Surveys, 38:5 (1983).
- [Farber1984] M. Sh. Farber, An algebraic classification of some even-dimensional spherical knots I, II, Trans. Amer. Math. Soc. 281 (1984), 507-528; 529-570.
- [Habegger1986] N. Habegger, Knots and links in codimension greater than 2, Topology, 25:3 (1986) 253--260.
- [Haefliger1962] A. Haefliger, Knotted
 -spheres in
-spheres in  -space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
-space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
- [Haefliger1962t] A. Haefliger, Differentiable links, Topology, 1 (1962) 241--244
- [Haefliger1966a] A. Haefliger, Enlacements de sphères en co-dimension supérieure à 2, Comment. Math. Helv.41 (1966), 51-72. MR0212818 (35 #3683) Zbl 0149.20801
- [Kearton1983] C. Kearton, An algebraic classification of certain simple even-dimensional knots, Trans. Amer. Math. Soc. 176 (1983), 1–53.
- [Levine1965] J. Levine, A classification of differentiable knots, Ann. of Math. (2) 82 (1965), 15–50. MR0180981 (31 #5211) Zbl 0136.21102
- [Milgram1972] R. J. Milgram, On the Haefliger knot groups, Bull. of the Amer. Math. Soc., 78:5 (1972) 861--865.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.
 2].
2].
2 Examples
Analogously to the Haefliger trefoil knot for  one constructs a smooth embedding
one constructs a smooth embedding 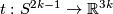 .
For
.
For  even this embedding is a generator of
even this embedding is a generator of 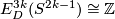 ; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for
; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for  odd this embedding is a generator of
odd this embedding is a generator of 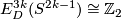 .
The last phrase of [Haefliger1962t] suggests that this is true for
.
The last phrase of [Haefliger1962t] suggests that this is true for 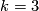 .
.
3 Readily calculable classification
For  the group
the group  has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs
has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs 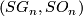 for
for 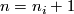 and
and 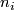 [Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here
[Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here 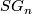 is the space of maps
is the space of maps 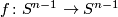 of degree
of degree  .
Restricting an element of
.
Restricting an element of 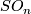 to
to 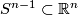 identifies
identifies 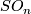 as a subspace of
as a subspace of 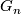 .
.
Some readily calculable corollaries of this classification are recalled in [Skopenkov2006,  3.3].
3.3].
4 Codimension 2 knots
A description of 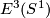 and, more generally, of
and, more generally, of 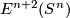 is a well-known very hard open problem.
is a well-known very hard open problem.
There is a vast literature on codimension 2 knots, most of which does not present a readily calculable classification (in the sense of Remark 1.1 of [Skopenkov2016c]). See e.g. interesting papers [Farber1981], [Farber1983], [Kearton1983], [Farber1984].
5 References
- [Farber1981] M. Sh. Farber, Classification of stable fibered knots, Mat. Sb. (N.S.), 115(157):2(6) (1981) 223–262.
- [Farber1983] M. Sh. Farber, The classification of simple knots, Russian Math. Surveys, 38:5 (1983).
- [Farber1984] M. Sh. Farber, An algebraic classification of some even-dimensional spherical knots I, II, Trans. Amer. Math. Soc. 281 (1984), 507-528; 529-570.
- [Habegger1986] N. Habegger, Knots and links in codimension greater than 2, Topology, 25:3 (1986) 253--260.
- [Haefliger1962] A. Haefliger, Knotted
 -spheres in
-spheres in  -space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
-space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
- [Haefliger1962t] A. Haefliger, Differentiable links, Topology, 1 (1962) 241--244
- [Haefliger1966a] A. Haefliger, Enlacements de sphères en co-dimension supérieure à 2, Comment. Math. Helv.41 (1966), 51-72. MR0212818 (35 #3683) Zbl 0149.20801
- [Kearton1983] C. Kearton, An algebraic classification of certain simple even-dimensional knots, Trans. Amer. Math. Soc. 176 (1983), 1–53.
- [Levine1965] J. Levine, A classification of differentiable knots, Ann. of Math. (2) 82 (1965), 15–50. MR0180981 (31 #5211) Zbl 0136.21102
- [Milgram1972] R. J. Milgram, On the Haefliger knot groups, Bull. of the Amer. Math. Soc., 78:5 (1972) 861--865.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.
 2].
2].
2 Examples
Analogously to the Haefliger trefoil knot for  one constructs a smooth embedding
one constructs a smooth embedding 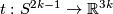 .
For
.
For  even this embedding is a generator of
even this embedding is a generator of 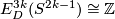 ; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for
; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if for  odd this embedding is a generator of
odd this embedding is a generator of 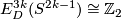 .
The last phrase of [Haefliger1962t] suggests that this is true for
.
The last phrase of [Haefliger1962t] suggests that this is true for 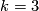 .
.
3 Readily calculable classification
For  the group
the group  has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs
has been described in terms of exact sequences involving the homotopy groups of spheres and the homotopy groups of the pairs 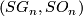 for
for 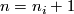 and
and 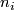 [Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here
[Haefliger1966a], cf. [Levine1965], [Milgram1972], [Habegger1986].
Here 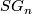 is the space of maps
is the space of maps 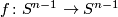 of degree
of degree  .
Restricting an element of
.
Restricting an element of 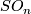 to
to 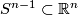 identifies
identifies 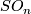 as a subspace of
as a subspace of 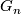 .
.
Some readily calculable corollaries of this classification are recalled in [Skopenkov2006,  3.3].
3.3].
4 Codimension 2 knots
A description of 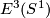 and, more generally, of
and, more generally, of 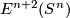 is a well-known very hard open problem.
is a well-known very hard open problem.
There is a vast literature on codimension 2 knots, most of which does not present a readily calculable classification (in the sense of Remark 1.1 of [Skopenkov2016c]). See e.g. interesting papers [Farber1981], [Farber1983], [Kearton1983], [Farber1984].
5 References
- [Farber1981] M. Sh. Farber, Classification of stable fibered knots, Mat. Sb. (N.S.), 115(157):2(6) (1981) 223–262.
- [Farber1983] M. Sh. Farber, The classification of simple knots, Russian Math. Surveys, 38:5 (1983).
- [Farber1984] M. Sh. Farber, An algebraic classification of some even-dimensional spherical knots I, II, Trans. Amer. Math. Soc. 281 (1984), 507-528; 529-570.
- [Habegger1986] N. Habegger, Knots and links in codimension greater than 2, Topology, 25:3 (1986) 253--260.
- [Haefliger1962] A. Haefliger, Knotted
 -spheres in
-spheres in  -space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
-space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
- [Haefliger1962t] A. Haefliger, Differentiable links, Topology, 1 (1962) 241--244
- [Haefliger1966a] A. Haefliger, Enlacements de sphères en co-dimension supérieure à 2, Comment. Math. Helv.41 (1966), 51-72. MR0212818 (35 #3683) Zbl 0149.20801
- [Kearton1983] C. Kearton, An algebraic classification of certain simple even-dimensional knots, Trans. Amer. Math. Soc. 176 (1983), 1–53.
- [Levine1965] J. Levine, A classification of differentiable knots, Ann. of Math. (2) 82 (1965), 15–50. MR0180981 (31 #5211) Zbl 0136.21102
- [Milgram1972] R. J. Milgram, On the Haefliger knot groups, Bull. of the Amer. Math. Soc., 78:5 (1972) 861--865.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.