Embeddings of manifolds with boundary: classification
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
Most of this page is intended not only for specialists in embeddings, but also for mathematician from other areas who want to apply or to learn the theory of embeddings.
Recall that some Unknotting Theorems hold for manifolds with boundary [Skopenkov2016c,  3], [Skopenkov2006,
3], [Skopenkov2006,  2]. In this page we present results peculiar for manifold with non-empty boundary.
2]. In this page we present results peculiar for manifold with non-empty boundary.
For a general introduction to embeddings as well as the notation and conventions used on this page, we refer to [Skopenkov2016c,  1,
1,  3].
3].
2 Unknotting Theorems
Theorem 2.1.
Assume  is a compact connected
is a compact connected  -manifold and one of the following conditions holds:
-manifold and one of the following conditions holds:
(a) (General position theorem)
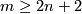 ;
;
(b) (Whitney-Wu unknotting theorem)
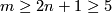 ;
;
(c)
 has non-empty boundary,
has non-empty boundary, 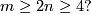
(d)
 has non-empty boundary and is
has non-empty boundary and is  -connected,
-connected, 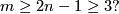
Then any two embeddings of  into
into  are isotopic.
are isotopic.
[Horvatic1971] ...
3 Construction and examples
...
4 Invariants
...
5 Classification
6 Further discussion
...
7 References
- [Becker&Glover1971] J. Becker and H. Glover, Note on the embedding of manifolds in Euclidean space, Proc. Am. Math. Soc. 27 (1971), 405-410. MR0268903 (42 #3800) Zbl 0207.22402
- [Horvatic1971] K. Horvatic, On embedding polyhedra and manifolds, Trans. Am. Math. Soc. 157 (1971), 417-436.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.
 2]. In this page we present results peculiar for manifold with non-empty boundary.
2]. In this page we present results peculiar for manifold with non-empty boundary.
For a general introduction to embeddings as well as the notation and conventions used on this page, we refer to [Skopenkov2016c,  1,
1,  3].
3].
2 Unknotting Theorems
Theorem 2.1.
Assume  is a compact connected
is a compact connected  -manifold and one of the following conditions holds:
-manifold and one of the following conditions holds:
(a) (General position theorem)
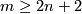 ;
;
(b) (Whitney-Wu unknotting theorem)
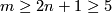 ;
;
(c)
 has non-empty boundary,
has non-empty boundary, 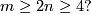
(d)
 has non-empty boundary and is
has non-empty boundary and is  -connected,
-connected, 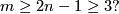
Then any two embeddings of  into
into  are isotopic.
are isotopic.
[Horvatic1971] ...
3 Construction and examples
...
4 Invariants
...
5 Classification
6 Further discussion
...
7 References
- [Becker&Glover1971] J. Becker and H. Glover, Note on the embedding of manifolds in Euclidean space, Proc. Am. Math. Soc. 27 (1971), 405-410. MR0268903 (42 #3800) Zbl 0207.22402
- [Horvatic1971] K. Horvatic, On embedding polyhedra and manifolds, Trans. Am. Math. Soc. 157 (1971), 417-436.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.
 2]. In this page we present results peculiar for manifold with non-empty boundary.
2]. In this page we present results peculiar for manifold with non-empty boundary.
For a general introduction to embeddings as well as the notation and conventions used on this page, we refer to [Skopenkov2016c,  1,
1,  3].
3].
2 Unknotting Theorems
Theorem 2.1.
Assume  is a compact connected
is a compact connected  -manifold and one of the following conditions holds:
-manifold and one of the following conditions holds:
(a) (General position theorem)
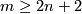 ;
;
(b) (Whitney-Wu unknotting theorem)
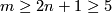 ;
;
(c)
 has non-empty boundary,
has non-empty boundary, 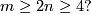
(d)
 has non-empty boundary and is
has non-empty boundary and is  -connected,
-connected, 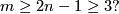
Then any two embeddings of  into
into  are isotopic.
are isotopic.
[Horvatic1971] ...
3 Construction and examples
...
4 Invariants
...
5 Classification
6 Further discussion
...
7 References
- [Becker&Glover1971] J. Becker and H. Glover, Note on the embedding of manifolds in Euclidean space, Proc. Am. Math. Soc. 27 (1971), 405-410. MR0268903 (42 #3800) Zbl 0207.22402
- [Horvatic1971] K. Horvatic, On embedding polyhedra and manifolds, Trans. Am. Math. Soc. 157 (1971), 417-436.
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.