Linking form
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
[edit] 1 Definition
After Seifert [Seifert1933], a closed oriented  -manifold
-manifold  has a bilinear linking form
has a bilinear linking form
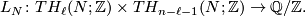
Here the torsion part of an abelian group  is the subgroup
is the subgroup
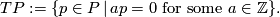
The linking form is the analogue for the torsion part of the homology of the intersection product which vanishes on the torsion part.
The linking form is defined as follows. Take ![[x] \in TH_\ell(N;\Zz)](/images/math/5/4/a/54a71d3560b264c8973b3f2b6202d733.png) and
and ![[y] \in TH_{n-\ell-1}(N;\Zz)](/images/math/4/e/8/4e84e08eecedab4e876a31ffe7e0c48e.png) represented by cycles
represented by cycles 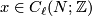 and
and 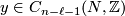 .
There is
.
There is 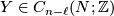 such that
such that 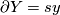 for some
for some  .
Define
.
Define
![\displaystyle L_N([x],[y]):= \langle x, Y \rangle/s \in \mathbb{Q}/\Zz](/images/math/c/1/6/c168e70fdf945d1ec37493ac73b7dc5d.png)
 and
and  divided by
divided by  and taken modulo 1.
and taken modulo 1.
For fixed ![N,[x],[y]](/images/math/e/d/a/edacd2968283db9ea53eeae013d8cfce.png) the resulting `residie modulo 1' is independent of the choices of
the resulting `residie modulo 1' is independent of the choices of 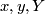 and
and  .
.
We have
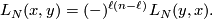
[edit] 2 Definition via cohomology
Let  and let
and let  . Note that we have Poincaré duality isomorphisms
. Note that we have Poincaré duality isomorphisms
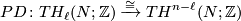
and
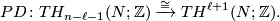
Associated to the short exact sequence of coefficients
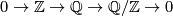
is the Bockstein long exact sequence in cohomology:
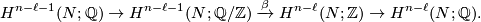
Choose 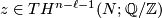 such that
such that 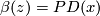 . This is always possible since torsion elements in
. This is always possible since torsion elements in  map to zero in
map to zero in 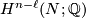 . There is a cup product:
. There is a cup product:
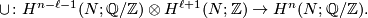
Then the Kronecker pairing,
![\displaystyle \langle z \cup PD(y),[N] \rangle \in \mathbb{Q}/\mathbb{Z},](/images/math/5/9/f/59f39628bc85fc39b6ac97035e7e5ea8.png)
of 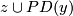 with the fundamental class of
with the fundamental class of  yields
yields 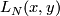 .
.
[edit] 3 Examples of 3-dimensional projective and lens spaces
As an example, let 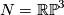 , so that
, so that 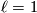 and
and  . Now
. Now 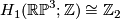 . Let
. Let 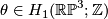 be the non-trivial element. To compute the linking
be the non-trivial element. To compute the linking 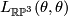 , consider
, consider 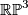 modelled as
modelled as 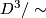 , with antipodal points on
, with antipodal points on 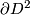 identified, and choose two representative
identified, and choose two representative  -chains
-chains  and
and  for
for 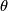 . Let
. Let  be the straight line between north and south poles and let
be the straight line between north and south poles and let  be half of the equator. Now
be half of the equator. Now 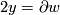 , where
, where 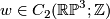 is the 2-disk whose boundary is the equator. We see that
is the 2-disk whose boundary is the equator. We see that 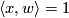 , so that
, so that
![\displaystyle L_{\mathbb{RP}^3}(\theta,\theta) = L_{\mathbb{RP}^3}([x],[y]) = \langle x,y \rangle/2 = 1/2.](/images/math/0/5/7/05725e0e70a522c8cbd35b7582e9aedd.png)
Generalising the above example, the 3-dimensional lens space 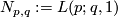 has
has 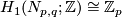 . The linking form is given on a generator
. The linking form is given on a generator 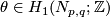 by
by 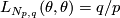 . Note that
. Note that  , so this is consistent with the above example.
, so this is consistent with the above example.
[edit] 4 Presentations of linking forms
A presentation for a middle dimensional linking form on 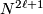
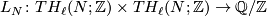
is an exact sequence:
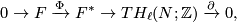
where  is a free abelain group and the linking
is a free abelain group and the linking 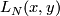 can be computed as follows. Let
can be computed as follows. Let 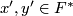 be such that
be such that 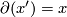 and
and 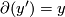 . Then we can tensor with
. Then we can tensor with 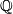 to obtain an isomorphism
to obtain an isomorphism
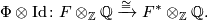
The linking form of  is then given by:
is then given by:
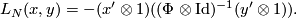
This formula, in particular the appearance of the sign, is explained in [Gordon&Litherland1978, Section 3] and [Alexander&Hamrick&Vick1976, Proof of Theorem 2.1].
Let 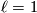 , so
, so 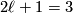 . Every 3-manifold
. Every 3-manifold  is the boundary of a simply connected 4-manifold, which is obtained by glueing 2-handles to an integrally framed link in
is the boundary of a simply connected 4-manifold, which is obtained by glueing 2-handles to an integrally framed link in  [Lickorish1962], [Wallace1960]. This is sometimes called a surgery presentation for
[Lickorish1962], [Wallace1960]. This is sometimes called a surgery presentation for  . Suppose that
. Suppose that  is a rational homology 3-sphere. Let
is a rational homology 3-sphere. Let  be the matrix of (self-) linking numbers of the surgery presentation link. Taking the number of link components in the surgery presentation for
be the matrix of (self-) linking numbers of the surgery presentation link. Taking the number of link components in the surgery presentation for  as the rank of
as the rank of  , the linking matrix
, the linking matrix  determines a map
determines a map 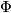 as above, which presents the linking form of
as above, which presents the linking form of  . The intersection form on a simply connected 4-manifold
. The intersection form on a simply connected 4-manifold  whose boundary is
whose boundary is  presents the linking form of
presents the linking form of  . This follows from the long exact sequence of the pair
. This follows from the long exact sequence of the pair 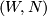 and Poincaré duality. See [Boyer1986] for more details and the use of such presentations for the classification of simply connected 4-manifolds with a given boundary.
and Poincaré duality. See [Boyer1986] for more details and the use of such presentations for the classification of simply connected 4-manifolds with a given boundary.
For example, the lens space 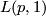 is the boundary of the
is the boundary of the 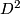 bundle over
bundle over  with Euler number
with Euler number 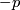 , so the presentation
, so the presentation 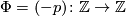 presents the linking form of
presents the linking form of 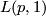 [Gompf&Stipsicz1999, Example 5.3.2].
[Gompf&Stipsicz1999, Example 5.3.2].
[edit] 5 Role in the classification of odd-dimensional manifolds
Linking forms play an important role in the classification of odd-dimensional manifolds.
For closed simply connected 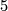 -manifolds
-manifolds  , the linking form is a complete invariant if
, the linking form is a complete invariant if 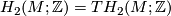 . For more information in dimension
. For more information in dimension 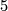 , see the page on simply-connected 5-manifolds.
, see the page on simply-connected 5-manifolds.
For the role of linking forms in the classification of smooth 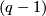 -connected
-connected 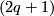 manifolds with boundary a homotopy sphere, see [Wall1967, Theorem 7].
manifolds with boundary a homotopy sphere, see [Wall1967, Theorem 7].
[edit] 6 Algebraic classification
An algebraic linking form is a non-singular bi-linear pairing
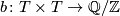
on a finite abelian group  . It is called symmetric if
. It is called symmetric if 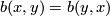 and skew-symmetric
if
and skew-symmetric
if  .
.
The classification of skew-symmetric linking forms is rather simple and is due to Wall, [Wall1963, Theorem 3]. It is described in detail in the page on simply-connected 5-manifolds.
The classification of symmetric linking forms is rather intricate. It was begun in [Wall1963] and completed by Kawauchi and Kojima: see [Kawauchi&Kojima1980, Theorem 4.1].
[edit] 7 References
- [Alexander&Hamrick&Vick1976] J. P. Alexander, G. C. Hamrick and J. W. Vick, Linking forms and maps of odd prime order, Trans. Amer. Math. Soc. 221 (1976), no.1, 169–185. MR0402786 (53 #6600) Zbl 0357.57009
- [Boyer1986] S. Boyer, Simply-connected
 -manifolds with a given boundary, Trans. Amer. Math. Soc. 298 (1986), no.1, 331–357. MR857447 (88b:57023) Zbl 0790.57009
-manifolds with a given boundary, Trans. Amer. Math. Soc. 298 (1986), no.1, 331–357. MR857447 (88b:57023) Zbl 0790.57009
- [Gompf&Stipsicz1999] R. E. Gompf and A. I. Stipsicz,
 -manifolds and Kirby calculus, American Mathematical Society, 1999. MR1707327 (2000h:57038) Zbl 0933.57020
-manifolds and Kirby calculus, American Mathematical Society, 1999. MR1707327 (2000h:57038) Zbl 0933.57020
- [Gordon&Litherland1978] C. M. Gordon and R. A. Litherland, On the signature of a link, Invent. Math. 47 (1978), no.1, 53–69. MR0500905 (58 #18407) Zbl 0391.57004
- [Kawauchi&Kojima1980] A. Kawauchi and S. Kojima, Algebraic classification of linking pairings on
 -manifolds, Math. Ann. 253 (1980), no.1, 29–42. MR594531 (82b:57007) Zbl 0427.57001
-manifolds, Math. Ann. 253 (1980), no.1, 29–42. MR594531 (82b:57007) Zbl 0427.57001
- [Lickorish1962] W. B. R. Lickorish, A representation of orientable combinatorial
 -manifolds, Ann. of Math. (2) 76 (1962), 531–540. MR0151948 (27 #1929) Zbl 0106.37102
-manifolds, Ann. of Math. (2) 76 (1962), 531–540. MR0151948 (27 #1929) Zbl 0106.37102
- [Seifert1933] H. Seifert, Verschlingungsinvarianten, Sitzungsber. Preuß. Akad. Wiss., Phys.-Math. Kl. 1933, No.26-29, (1933) 811-828. Zbl 0008.18101
- [Wall1963] C. T. C. Wall, Quadratic forms on finite groups, and related topics, Topology 2 (1963), 281–298. MR0156890 (28 #133) Zbl 0215.39903
- [Wall1967] C. T. C. Wall, Classification problems in differential topology. VI. Classification of
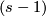 -connected
-connected 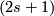 -manifolds, Topology 6 (1967), 273–296. MR0216510 (35 #7343) Zbl 0173.26102
-manifolds, Topology 6 (1967), 273–296. MR0216510 (35 #7343) Zbl 0173.26102
- [Wallace1960] A. H. Wallace, Modifications and cobounding manifolds, Canad. J. Math. 12 (1960), 503–528. MR0125588 (23 #A2887) Zbl 0116.40401
[edit] 8 External links
- The Wikipedia page on Poincaré duality