Stiefel-Whitney characteristic classes
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Definition
Let  be a compact smooth
be a compact smooth  -manifold (possibly with boundary).
Denote by
-manifold (possibly with boundary).
Denote by  the Poincaré duality isomorphism.
Here for non-orientable
the Poincaré duality isomorphism.
Here for non-orientable  the coefficients in cohomology are twisted (by the orientation double covering) and the coefficients in homology are non-twisted.
Let
the coefficients in cohomology are twisted (by the orientation double covering) and the coefficients in homology are non-twisted.
Let 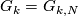 be
be  if either
if either 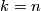 or
or  is odd, and
is odd, and  if either
if either 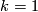 or
or  is even.
is even.
Stiefel defined the homology Stiefel-Whitney class 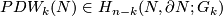 of
of  to be the homology class of a degeneracy subset of a general position collection of
to be the homology class of a degeneracy subset of a general position collection of 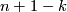 tangent vector fields on
tangent vector fields on  .
.
Whitney defined the homology normal Stiefel-Whitney class 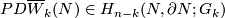 of
of  to be the homology class of a degeneracy subset of a general position collection of
to be the homology class of a degeneracy subset of a general position collection of 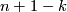 normal vector fields on
normal vector fields on 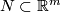 .
.
Let 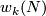 and
and 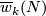 be the reductions of
be the reductions of 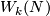 and
and 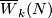 modulo 2.
modulo 2.
Let ![w_0(N)=\overline w_0(N)=[N]](/images/math/8/7/4/874e8a8d6533883a2bdaf04e70bf9ad8.png) .
.
See details e.g. in [Milnor&Stasheff1974,  12], [Fomenko&Fuchs2016,
12], [Fomenko&Fuchs2016,  19.C], [Skopenkov2015b,
19.C], [Skopenkov2015b,  9,11,12].
9,11,12].
There is an alternative definition of 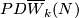 [Skopenkov2006,
[Skopenkov2006,  2.3 `the Whitney obstruction'] analogous to definition of the Whitney invariant.
2.3 `the Whitney obstruction'] analogous to definition of the Whitney invariant.
See also Wikipedia article.
2 The Wu formula
Theorem 2.1 (Wu formula).
If  is a closed smooth
is a closed smooth  -submanifold of
-submanifold of  and
and 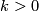 is an integer, then
is an integer, then
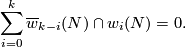
Proof. (This proof should be known but is absent from textbooks. This text is written by M. Fedorov and A. Skopenkov in frame of the course `Algebraic topology of manifolds in interesting results'.)
In this proof we abbreviate 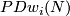 to just
to just 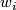 and the same for
and the same for 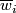 .
Denote by
.
Denote by 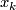 the obstruction to existence of
the obstruction to existence of 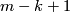 linearly independent fields on
linearly independent fields on  .
Clearly
.
Clearly 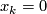 .
So it suffices to show that
.
So it suffices to show that 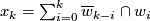 .
.
Take general position collection of normal fields 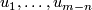 on
on  such that for each
such that for each 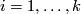 the collection
the collection  is linearly dependent on
is linearly dependent on  subcomplex
subcomplex 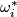 representing
representing 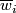 .
.
Take general position collection of tangent fields 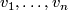 on
on  such that for each
such that for each 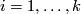 the collection
the collection 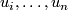 is linearly dependent on
is linearly dependent on  subcomplex
subcomplex 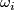 representing
representing 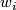 .
.
Define 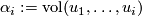 and
and 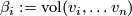 .
Denote by
.
Denote by  the following collection
the following collection  of
of 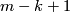 vector fields on
vector fields on 
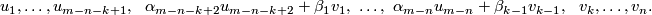
This is a general position collection, so 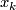 is represented by set where
is represented by set where  is linearly dependent. Clearly, all non-zero vectors among
is linearly dependent. Clearly, all non-zero vectors among
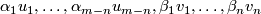 are linearly independent. Hence
are linearly independent. Hence 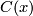 is linearly dependent if and only if either
is linearly dependent if and only if either 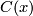 contains zero vector or
contains zero vector or 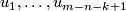 are linearly dependent at
are linearly dependent at  or
or 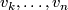 are linearly dependent at
are linearly dependent at  .
The collection
.
The collection 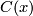 contains a zero vector if and only if
contains a zero vector if and only if 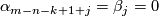 at
at  for some
for some  .
Thus ???
.
Thus ??? 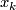 is represented by
is represented by 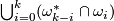 .
.
3 References
- [Fomenko&Fuchs2016] A. T. Fomenko and D. B. Fuks, Homotopical Topology. Translated from the Russian. Graduate Texts in Mathematics, 273. Springer-Verlag, Berlin, 2016. DOI 10.1007/978-3-319-23488-5.
- [Milnor&Stasheff1974] J. W. Milnor and J. D. Stasheff, Characteristic classes, Princeton University Press, Princeton, N. J., 1974. MR0440554 (55 #13428) Zbl 1079.57504
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
- [Skopenkov2015b] A. Skopenkov, Algebraic Topology From Geometric Viewpoint (in Russian), MCCME, Moscow, 2015, 2020. Accepted for English translation by `Moscow Lecture Notes' series of Springer. Preprint of a part