Orientation covering
| (28 intermediate revisions by one user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{Authors|Matthias Kreck}} | |
| − | + | <wikitex>; | |
| − | <wikitex | + | Let $M$ be a $n$-dimensional topological manifold. We construct an oriented manifold $\hat M$ and a $2$-fold covering $p : \hat M \to M$ called the orientation covering. The non-trivial deck transformation of this covering is orientation-reversing. As a set $\hat M$ is the set of pairs $(x, o_x)$, where $o_x$ is a local orientation of $M$ at $x$ given by a generator of the infinite cyclic group $H_n(M, M-x;\mathbb Z)$. The map $p$ assignes $x$ to $(x,o_x)$. Since there are precisely two local orientations, the fibres of this map have cardinality $2$. |
| − | Let $M$ be a $n$-dimensional topological | + | |
| − | Next we define a topology on this set. Let $\varphi : U \to V\subset \mathbb R^n$ be a chart of $M$ | + | Next we define a topology on this set. Let $\varphi : U \to V\subset \mathbb R^n$ be a chart of $M$. We orient $\mathbb R^n$ by the standard orientation given by the standard basis $e_1$, $e_2$, ..., $e_n$, from which we define a a continuous local orientation by identifying the tangent space with $\mathbb R^n$. Since for a smooth manifold a tangential orientation defines a homological orientation, this also gives a [[Orientation_of_manifolds#Reformulation_in_terms_of_local_homological_orientations|homological orientation]]: see \cite[§3]{Kreck2013}. We call the standard local orientation at $x \in \mathbb R^n$ by $sto_x$. Using the chart we transport this standard orientation to $U$ by the induced map on homology. The local orientations given by this orientation of $U$ determine a subset of $\hat M$, which we require to be open. Doing the same starting with the non-standard orientation of $\mathbb R^n$ we obtain another subset, which we also call open. We give $\hat M$ the topology generated by these open subsets, where we vary about all charts. By construction each of these open subsets is are homeomorphic to an open subset of $\mathbb R^n$, and so we obtain an atlas of $\hat M$. The map $p$ is by construction a $2$-fold covering. By construction $\hat M$ is oriented in a tautological way and the non-trivial [[Wikipedia:Covering_space#Deck_transformation_group.2C_regular_covers|deck transformation]] of the covering is orientation reversing. |
| + | |||
| + | Thus we have constructed a $2$-fold covering of $M$ by an oriented manifold $\hat M$, which is smooth, if $M$ is smooth. This covering is called the '''orientation covering'''. | ||
| + | |||
| + | If $M$ is smooth one can use the local tangential orientation of $T_xM$ instead of the homological orientation to construct the orientation covering (for the equivalence of these data see the Manifold Atlas page [[Orientation_of_manifolds#Reformulations_of_orientation_for_smooth_manifolds|Orientation of manifolds]]; \cite[§6]{Kreck2013}). Since a countable covering of a smooth manifold has a unique smooth structure such that the projection map is a local diffeomorphism, in the smooth case $\hat M$ is a smooth manifold and $p$ a local diffeomorphism. | ||
| + | |||
| + | For more information and a discussion placing the orientation covering in a wider setting, see \cite{Dold1995|VIII § 2}. | ||
| + | </wikitex> | ||
| + | |||
| + | ==Characterization of the orientation covering== | ||
| + | <wikitex>; | ||
| + | One can easily characterize the orientation covering: | ||
| + | {{beginthm|Proposition}} If $N $ is an oriented manifold and $p: N \to M$ is a $2$-fold covering with orientation reversing non-trivial deck transformation, then it is isomorphic to the orientation covering. | ||
| + | {{endthm}} | ||
| + | |||
| + | {{beginproof}} | ||
| + | We have a map $N \to \hat M$ by mapping $y \in N$ to $(p(y), orientation \,\, induced \,\, by \,\, p)$. This is an isomorphism of these two coverings. | ||
| + | {{endproof}} | ||
| + | If $M$ is orientable, we pick an orientation and see that $\hat M$ is the disjoint union of $\{(x,o_x)| \,\, o_x \,\, is \,\, the \,\, local \,\, orientation \,\, given \,\, by \,\, the \,\, orientation \,\, of \,\, M\}$ and its complement, so it is isomorphic to the trivial covering $ M \times \mathbb Z/2$. In turn if the orientation covering is trivial it decomposes $\hat M$ into two open (and thus oriented) subsets homeomorphic to $M$ and so $M$ is orientable. Thus we have shown: | ||
| + | {{beginthm|Proposition}} $M$ is orientable if and only if the orientation covering is trivial. If $M$ is connected, $M$ is non-orientable if and only if $\hat M$ is connected. In particular, any simply-connected manifold is orientable. | ||
| + | {{endthm}} | ||
| + | </wikitex> | ||
| + | |||
| + | ==Relation to the orientation character== | ||
| + | <wikitex>; | ||
| + | We assume now that $M$ is connected. The [[Orientation character|orientation character]] is a homomorphism $w: \pi_1(M) \to \{ \pm 1\}$, which attaches $+1$ to a loop $S^1 \to M$ if and only if the pull back of the orientation covering is trivial. By the classification of coverings this implies that $w$ is trivial if and only if $M$ is orientable. | ||
</wikitex> | </wikitex> | ||
| − | == | + | == Examples == |
| − | <wikitex | + | <wikitex>; |
| − | If $M$ is orientable | + | Here are some examples of orientation coverings. |
| + | # If $M$ is orientable then $p \colon \hat M \to M$ is isomorphic to the projection $M \times \mathbb Z/2 \to M$. | ||
| + | # If $n$ is even, $\mathbb R P^n$ is non-orientable and the orientation cover is the canonical projection $S^n \to \mathbb R P^n$. The deck transformation of the orientation covering is the [[Wikipedia:Antipodal point|antipodal map]] on $S^n$. | ||
| + | # The orientation cover of the [[Wikipedia:Klein bottle|Klein bottle]] $K^2$ is the canonical projection from the [[2-manifolds#Orientable_surfaces|2-torus]]; $p \colon T^2 \to K^2$. | ||
| + | # The orientation cover of the open [[Wikipedia:Mobius_strip|Möbius strip]] $Mö$ is the canonical projection from the cylinder; $p \colon S^1 \times \Rr \to Mö$. | ||
</wikitex> | </wikitex> | ||
== References == | == References == | ||
| Line 16: | Line 44: | ||
* The Encylopedia of Mathematics article on [http://www.encyclopediaofmath.org/index.php/Orientation orientation]. | * The Encylopedia of Mathematics article on [http://www.encyclopediaofmath.org/index.php/Orientation orientation]. | ||
* The Wikipedia page on the [[Wikipedia:Orientability#Orientable_double_cover|orientability]]. | * The Wikipedia page on the [[Wikipedia:Orientability#Orientable_double_cover|orientability]]. | ||
| − | [[Category: | + | [[Category:Definitions]] |
| + | [[Category:Definitions]] | ||
Latest revision as of 20:40, 7 March 2014
|
An earlier version of this page was published in the Definitions section of the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 18:40, 7 March 2014 and the covering&diff=cur&oldid=11510 changes since publication. |
|
The user responsible for this page is Matthias Kreck. No other user may edit this page at present. |
Let  be a
be a  -dimensional topological manifold. We construct an oriented manifold
-dimensional topological manifold. We construct an oriented manifold  and a
and a  -fold covering
-fold covering 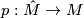 called the orientation covering. The non-trivial deck transformation of this covering is orientation-reversing. As a set
called the orientation covering. The non-trivial deck transformation of this covering is orientation-reversing. As a set  is the set of pairs
is the set of pairs 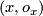 , where
, where 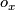 is a local orientation of
is a local orientation of  at
at  given by a generator of the infinite cyclic group
given by a generator of the infinite cyclic group 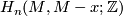 . The map
. The map  assignes
assignes  to
to 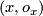 . Since there are precisely two local orientations, the fibres of this map have cardinality
. Since there are precisely two local orientations, the fibres of this map have cardinality  .
.
Next we define a topology on this set. Let 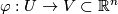 be a chart of
be a chart of  . We orient
. We orient 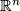 by the standard orientation given by the standard basis
by the standard orientation given by the standard basis 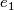 ,
, 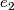 , ...,
, ...,  , from which we define a a continuous local orientation by identifying the tangent space with
, from which we define a a continuous local orientation by identifying the tangent space with 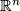 . Since for a smooth manifold a tangential orientation defines a homological orientation, this also gives a homological orientation: see [Kreck2013, §3]. We call the standard local orientation at
. Since for a smooth manifold a tangential orientation defines a homological orientation, this also gives a homological orientation: see [Kreck2013, §3]. We call the standard local orientation at 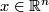 by
by 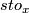 . Using the chart we transport this standard orientation to
. Using the chart we transport this standard orientation to  by the induced map on homology. The local orientations given by this orientation of
by the induced map on homology. The local orientations given by this orientation of  determine a subset of
determine a subset of  , which we require to be open. Doing the same starting with the non-standard orientation of
, which we require to be open. Doing the same starting with the non-standard orientation of 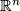 we obtain another subset, which we also call open. We give
we obtain another subset, which we also call open. We give  the topology generated by these open subsets, where we vary about all charts. By construction each of these open subsets is are homeomorphic to an open subset of
the topology generated by these open subsets, where we vary about all charts. By construction each of these open subsets is are homeomorphic to an open subset of 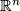 , and so we obtain an atlas of
, and so we obtain an atlas of  . The map
. The map  is by construction a
is by construction a  -fold covering. By construction
-fold covering. By construction  is oriented in a tautological way and the non-trivial deck transformation of the covering is orientation reversing.
is oriented in a tautological way and the non-trivial deck transformation of the covering is orientation reversing.
Thus we have constructed a  -fold covering of
-fold covering of  by an oriented manifold
by an oriented manifold  , which is smooth, if
, which is smooth, if  is smooth. This covering is called the orientation covering.
is smooth. This covering is called the orientation covering.
If  is smooth one can use the local tangential orientation of
is smooth one can use the local tangential orientation of 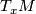 instead of the homological orientation to construct the orientation covering (for the equivalence of these data see the Manifold Atlas page Orientation of manifolds; [Kreck2013, §6]). Since a countable covering of a smooth manifold has a unique smooth structure such that the projection map is a local diffeomorphism, in the smooth case
instead of the homological orientation to construct the orientation covering (for the equivalence of these data see the Manifold Atlas page Orientation of manifolds; [Kreck2013, §6]). Since a countable covering of a smooth manifold has a unique smooth structure such that the projection map is a local diffeomorphism, in the smooth case  is a smooth manifold and
is a smooth manifold and  a local diffeomorphism.
a local diffeomorphism.
For more information and a discussion placing the orientation covering in a wider setting, see [Dold1995, VIII § 2].
Contents |
1 Characterization of the orientation covering
One can easily characterize the orientation covering:
Proposition 1.1. If  is an oriented manifold and
is an oriented manifold and 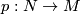 is a
is a  -fold covering with orientation reversing non-trivial deck transformation, then it is isomorphic to the orientation covering.
-fold covering with orientation reversing non-trivial deck transformation, then it is isomorphic to the orientation covering.
Proof.
We have a map 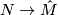 by mapping
by mapping 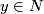 to
to 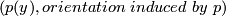 . This is an isomorphism of these two coverings.
. This is an isomorphism of these two coverings.

If  is orientable, we pick an orientation and see that
is orientable, we pick an orientation and see that  is the disjoint union of
is the disjoint union of 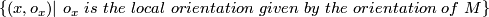 and its complement, so it is isomorphic to the trivial covering
and its complement, so it is isomorphic to the trivial covering 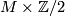 . In turn if the orientation covering is trivial it decomposes
. In turn if the orientation covering is trivial it decomposes  into two open (and thus oriented) subsets homeomorphic to
into two open (and thus oriented) subsets homeomorphic to  and so
and so  is orientable. Thus we have shown:
is orientable. Thus we have shown:
Proposition 1.2.  is orientable if and only if the orientation covering is trivial. If
is orientable if and only if the orientation covering is trivial. If  is connected,
is connected,  is non-orientable if and only if
is non-orientable if and only if  is connected. In particular, any simply-connected manifold is orientable.
is connected. In particular, any simply-connected manifold is orientable.
2 Relation to the orientation character
We assume now that  is connected. The orientation character is a homomorphism
is connected. The orientation character is a homomorphism  , which attaches
, which attaches 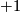 to a loop
to a loop 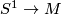 if and only if the pull back of the orientation covering is trivial. By the classification of coverings this implies that
if and only if the pull back of the orientation covering is trivial. By the classification of coverings this implies that  is trivial if and only if
is trivial if and only if  is orientable.
is orientable.
3 Examples
Here are some examples of orientation coverings.
- If
 is orientable then
is orientable then 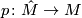 is isomorphic to the projection
is isomorphic to the projection 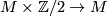 .
.
- If
 is even,
is even, 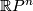 is non-orientable and the orientation cover is the canonical projection
is non-orientable and the orientation cover is the canonical projection 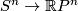 . The deck transformation of the orientation covering is the antipodal map on
. The deck transformation of the orientation covering is the antipodal map on  .
.
- The orientation cover of the Klein bottle
 is the canonical projection from the 2-torus;
is the canonical projection from the 2-torus; 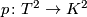 .
.
- The orientation cover of the open Möbius strip
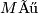 is the canonical projection from the cylinder;
is the canonical projection from the cylinder; 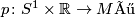 .
.
4 References
- [Dold1995] A. Dold, Lectures on algebraic topology, Springer-Verlag, 1995. MR1335915 (96c:55001) Zbl 0872.55001
- [Kreck2013] M. Kreck, Orientation of manifolds, Bull. Man. Atl. (2013).
5 External links
- The Encylopedia of Mathematics article on orientation.
- The Wikipedia page on the orientability.