Knots, i.e. embeddings of spheres
Askopenkov (Talk | contribs) |
Askopenkov (Talk | contribs) (→The Haefliger trefoil knot) |
||
| Line 12: | Line 12: | ||
See Figures 3.5 and 3.6 of \cite{Skopenkov2006}. | See Figures 3.5 and 3.6 of \cite{Skopenkov2006}. | ||
| − | Analogously for $k>1$ one constructs a smooth embedding $t:S^{2k-1}\to\Rr^{3k}$ | + | Analogously for $k>1$ one constructs a smooth embedding $t:S^{2k-1}\to\Rr^{3k}$. |
| + | For $k$ even this embedding is a generator of $E_D^{3k}(S^{2k-1})\cong\Zz$; it is not ''smoothly'' isotopic to the standard embedding, but is ''piecewise smoothly'' isotopic to it \cite{Haefliger1962}. | ||
| + | It would be interesting to know if this embedding is a generator of $E_D^{3k}(S^{2k-1})\cong\Zz_2$. | ||
| + | The last phrase of \cite{Haefliger1962a} suggests that this is true for $k=3$. | ||
</wikitex> | </wikitex> | ||
Revision as of 10:01, 26 April 2016
|
This page has not been refereed. The information given here might be incomplete or provisional. |
For notation and conventions throughout this page see high codimension embeddings.
Contents |
1 Examples
1.1 The Haefliger trefoil knot
Let us construct a smooth embedding 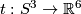 (which is a generator of
(which is a generator of 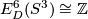 ) [Haefliger1962], 4.1. A miraculous property of this embedding is that it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to the standard embedding.
) [Haefliger1962], 4.1. A miraculous property of this embedding is that it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to the standard embedding.
Denote coordinates in  by
by 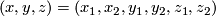 . The higher-dimensional trefoil knot
. The higher-dimensional trefoil knot  is obtained by joining with two tubes the higher-dimensional Borromean rings, i.e. the three spheres given by the following three systems of equations:
is obtained by joining with two tubes the higher-dimensional Borromean rings, i.e. the three spheres given by the following three systems of equations:
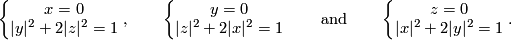
See Figures 3.5 and 3.6 of [Skopenkov2006].
Analogously for  one constructs a smooth embedding
one constructs a smooth embedding 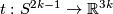 .
For
.
For  even this embedding is a generator of
even this embedding is a generator of 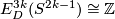 ; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if this embedding is a generator of
; it is not smoothly isotopic to the standard embedding, but is piecewise smoothly isotopic to it [Haefliger1962].
It would be interesting to know if this embedding is a generator of 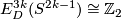 .
The last phrase of [Haefliger1962a] suggests that this is true for
.
The last phrase of [Haefliger1962a] suggests that this is true for 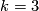 .
.
2 Classification
(I would suggest including the classification of simple knots a la Kearton et. al. in this section.---John Klein)
3 References
- [Haefliger1962] A. Haefliger, Knotted
 -spheres in
-spheres in  -space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
-space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
- [Haefliger1962a] A. Haefliger, Variétés feuilletées, Ann. Scuola Norm. Sup. Pisa (3) 16 (1962), 367–397. MR0189060 (32 #6487) Zbl 0196.25005
- [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.