Fake lens spaces
Tibor Macko (Talk | contribs) (→Construction and examples) |
m (→Construction and examples) |
||
| Line 175: | Line 175: | ||
Classical [[lens spaces]] are of course examples of fake lens spaces. To get fake lens spaces which are not homeomorphic to classical ones one can employ the construction of [[fake complex projective spaces]]. Note that a fake complex projective space is an orbit space of a free tame action of $S^1$ on $S^{2d-1}$ and that we obviously have $\Zz_N \cong G < S^1$. Restricting the action to the subgroup we obtain a fake lens space. Its $\rho$-[[rho-invariant|invariant]] can be calculated by the naturality using the formula ... | Classical [[lens spaces]] are of course examples of fake lens spaces. To get fake lens spaces which are not homeomorphic to classical ones one can employ the construction of [[fake complex projective spaces]]. Note that a fake complex projective space is an orbit space of a free tame action of $S^1$ on $S^{2d-1}$ and that we obviously have $\Zz_N \cong G < S^1$. Restricting the action to the subgroup we obtain a fake lens space. Its $\rho$-[[rho-invariant|invariant]] can be calculated by the naturality using the formula ... | ||
| − | The above | + | The above construction does not exhaust all the fake lens spaces. To get all of them there is a construction which produces from a given fake lens space $L$ another fake lens space $L'$ such that the difference of their $\rho$-invariants is a prescribed element |
$$ | $$ | ||
\rho (L) - \rho(L') = x \in 4 \cdot R_{\widehat G}^{(-1)^d}. | \rho (L) - \rho(L') = x \in 4 \cdot R_{\widehat G}^{(-1)^d}. | ||
Revision as of 11:27, 8 June 2010
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 15:18, 25 April 2013 and the changes since publication. |
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
A fake lens space is the orbit space of a free action of a finite cyclic group on a sphere. It is a generalization of the notion of a lens space which is the orbit space of such a free action which comes from a unitary representation.
The classification of fake lens spaces can be seen as one of the basic questions in topology of manifolds. It is systematically obtained in three stages. First, homotopy classification using classical homotopy theory. Second, simple homotopy classification using Reidemeister torsion. Finally, surgery theory is employed to obtain a classification within the respective simple homotopy types. In fact, this classification was one of the early spectacular applications of surgery theory.
2 Definition
Throughout this page we use the following notation. By  is denoted the finite cyclic group of order
is denoted the finite cyclic group of order  .
.
Let  be a free action of
be a free action of  on the sphere
on the sphere  . By
. By 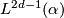 is denoted the orbit space of
is denoted the orbit space of  . Sometimes, when the dimension and the action are clear, we leave them from notation and simply write
. Sometimes, when the dimension and the action are clear, we leave them from notation and simply write  .
.
3 Invariants
For 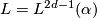 we have
we have
-
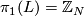 ,
, 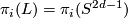 for
for 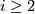
-
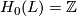 ,
, 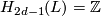 ,
, 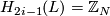 for
for 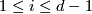 ,
, 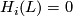 for all other values of
for all other values of  .
.
Interesting invariants for fake lens spaces are
- the Reidemeister torsion
![\Delta (L) \in \Qq [t] / (t^N - 1)](/images/math/e/1/4/e14c55dab04ab1ba91c3ddb4df51a1a1.png) and
and
- the
 -invariant
-invariant 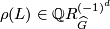 .
.
4 Homotopy Classification and simple homotopy classification
All the results are taken from chapter 14E of [Wall1999].
Notation
Recall the arithmetic (Rim) square:
![\displaystyle \xymatrix{ \Zz G \ar[r]^{\eta} \ar[d]_{\varepsilon} & R_G \ar[d]^{\varepsilon'} \\ \Zz \ar[r]_{\eta'} & \Zz_N }](/images/math/3/4/d/34da3249bf4eb2eac0505f1c05c1a32f.png)
where 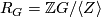 with
with 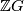 be the group ring of
be the group ring of  and
and  is the ideal generated by the norm element
is the ideal generated by the norm element 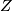 of
of  . The maps
. The maps  ,
, 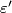 are the augmentation maps.
are the augmentation maps.
Recall that the Reidemeister torsion is a unit in 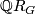 where
where 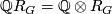 .
.
The homotopy classification is stated in the a priori broader context of finite CW-complexes  with
with 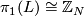 and with the universal cover homotopy equivalent to
and with the universal cover homotopy equivalent to  of which fake lens spaces are obviously a special case. It is convenient to make the
following definition.
of which fake lens spaces are obviously a special case. It is convenient to make the
following definition.
Definition 4.1.
Let  be a CW-complex with
be a CW-complex with 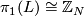 and with universal cover homotopy equivalent to
and with universal cover homotopy equivalent to  .
.
A polarization of  is a pair
is a pair 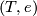 where
where  is a choice of a generator of
is a choice of a generator of 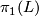 and
and  is a choice of a homotopy equivalence
is a choice of a homotopy equivalence 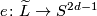 .
.
Recall the classical lens space 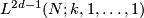 . By
. By 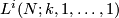 is denoted its
is denoted its  -skeleton with respect to the standard cell decomposition. If
-skeleton with respect to the standard cell decomposition. If  is odd this is a lens space, if
is odd this is a lens space, if  is even this is a CW-complex obtained by attaching an
is even this is a CW-complex obtained by attaching an  -cell to the lens space of dimension
-cell to the lens space of dimension 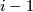 .
.
Theorem 4.2 Wall.
Let  be a finite CW-complex with
be a finite CW-complex with 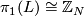 and universal cover
and universal cover  polarized by
polarized by 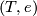 . Then there exists a simple homotopy equivalence
. Then there exists a simple homotopy equivalence
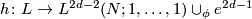
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 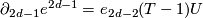 for some
for some
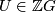 which maps to a unit
which maps to a unit  . Furthermore,
. Furthermore,  is a simple Poincare complex with Reidemeister torsion
is a simple Poincare complex with Reidemeister torsion 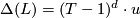 .
.
- The polarized homotopy types of such
 are in one-to-one correspondence with the units in
are in one-to-one correspondence with the units in 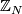 . The correspondence is given by
. The correspondence is given by 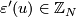 . The invariant
. The invariant 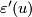 can be identified with the first non-trivial
can be identified with the first non-trivial  -invariant of
-invariant of  (in the sense of homotopy theory)
(in the sense of homotopy theory) 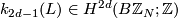 .
.
- The polarized simple homotopy types of such
 are in one-to-one correspondence with the units in
are in one-to-one correspondence with the units in 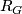 . The correspondence is given by
. The correspondence is given by  .
.
See [Wall1999, Theorem 14E.3].
The existence of a fake lens space in the homotopy type of such  is addressed in [Wall1999, Theorem 14E.4].
is addressed in [Wall1999, Theorem 14E.4].
Since the units 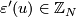 are exhausted by the lens spaces
are exhausted by the lens spaces
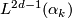 we obtain the following corollary.
we obtain the following corollary.
Corollary 4.3.
For any fake lens space 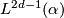 there exists
there exists 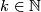 and a homotopy equivalence
and a homotopy equivalence
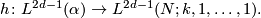
5 Homeomorphism classification
The homeomorphism classification, as already noted, is an excellent application of the non-simply connected surgery theory. Recall that for a topological manifold  the surgery theoretic homeomorphism classification of manifolds wihin the homotopy type of
the surgery theoretic homeomorphism classification of manifolds wihin the homotopy type of  is stated in terms of the surgery structure set
is stated in terms of the surgery structure set 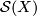 and that the primary tool for its calculation is the surgery exact sequence.
and that the primary tool for its calculation is the surgery exact sequence.
For a fake lens space  there is enough information about the normal invariants, the L-groups and the surgery obstruction in the surgery exact sequence so that one is left with just an extension problem. The strategy to proceed further is to relate the surgery exact sequence to representation theory of
there is enough information about the normal invariants, the L-groups and the surgery obstruction in the surgery exact sequence so that one is left with just an extension problem. The strategy to proceed further is to relate the surgery exact sequence to representation theory of  . This is done via the following commutative diagram of abelian groups and homomorphisms with exact rows
. This is done via the following commutative diagram of abelian groups and homomorphisms with exact rows
![\displaystyle \xymatrix{ 0 \ar[r] & {\widetilde L}^s_{2d} (G) \ar[r]^(0.4){\partial} \ar[d]_{\cong}^{G-sign} & {\mathcal S}^s (L^{2d-1}(\alpha)) \ar[r]^{\eta} \ar[d]^{\widetilde \rho}& \widetilde {\mathcal N} (L^{2d-1}(\alpha)) \ar[r] \ar[d]^{[\widetilde \rho]}& 0 \\ 0 \ar[r] & 4 \cdot R^{(-1)^d}_{\widehat G} \ar[r] & {\mathbb Q} R^{(-1)^d}_{\widehat G} \ar[r] & {\mathbb Q} R^{(-1)^d}_{\widehat G}/ 4 \cdot R^{(-1)^d}_{\widehat G} \ar[r] & 0 }](/images/math/c/e/0/ce0adafd80f8f574c18c1ece6266bff7.png)
where ![[\widetilde \rho]](/images/math/1/a/6/1a65cbd449686c64de628afc09f22dd2.png) is the homomorphism induced by
is the homomorphism induced by 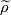 (see ?).
(see ?).
Theorem 5.1 Wall.
If  is odd, then the map
is odd, then the map
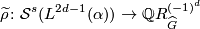
is injective.
See [Wall1999, Theorem 14E.7].
For  odd Wall managed to obtain an even beter result, namely the complete classification of fake lens spaces of a given dimension
odd Wall managed to obtain an even beter result, namely the complete classification of fake lens spaces of a given dimension 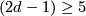 with the fundamental group
with the fundamental group 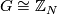 which goes as follows:
which goes as follows:
Theorem 5.2 Wall.
Let 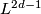 and
and 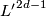 be oriented fake lens spaces with fundamental group
be oriented fake lens spaces with fundamental group  cyclic of odd order
cyclic of odd order  . Then there is an orientation preserving homeomorphism
. Then there is an orientation preserving homeomorphism 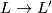 inducing the identity on
inducing the identity on  if and only if
if and only if 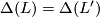 and
and  .
.
Given 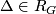 and
and 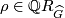 , there exists a corresponding fake lens space
, there exists a corresponding fake lens space 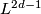 if and only if the following four statements hold:
if and only if the following four statements hold:
-
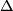 and
and  are both real (
are both real ( even) or imaginary (
even) or imaginary ( odd).
odd).
-
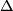 generates
generates 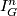 ,
, 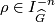 .
.
- The classes of
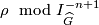 and
and 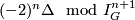 correspond under
correspond under
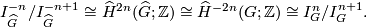
-
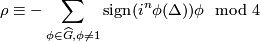 .
.
The following theorem is taken from [Wall1999, Theorem 14E.7].
For general  the following theorem is proved in [Macko&Wegner2008, Theorem 1.2]).
the following theorem is proved in [Macko&Wegner2008, Theorem 1.2]).
Theorem 5.3.
Let 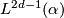 be a fake lens space with
be a fake lens space with 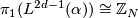 where
where 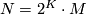 with
with 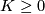 ,
,  odd and
odd and 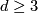 . Then we have
. Then we have

where 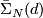 is a free abelian group. If
is a free abelian group. If  is odd then its rank is
is odd then its rank is 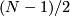 . If
. If  is even then its rank is
is even then its rank is 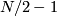 if
if 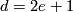 and
and  if
if 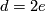 . In the torsion summand we have
. In the torsion summand we have 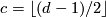 .
.
6 Construction and examples
Classical lens spaces are of course examples of fake lens spaces. To get fake lens spaces which are not homeomorphic to classical ones one can employ the construction of fake complex projective spaces. Note that a fake complex projective space is an orbit space of a free tame action of  on
on  and that we obviously have
and that we obviously have 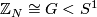 . Restricting the action to the subgroup we obtain a fake lens space. Its
. Restricting the action to the subgroup we obtain a fake lens space. Its  -invariant can be calculated by the naturality using the formula ...
-invariant can be calculated by the naturality using the formula ...
The above construction does not exhaust all the fake lens spaces. To get all of them there is a construction which produces from a given fake lens space  another fake lens space
another fake lens space 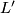 such that the difference of their
such that the difference of their  -invariants is a prescribed element
-invariants is a prescribed element
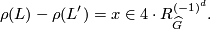
The construction is just the Wall realization from surgery theory, alias a non-simply connected generalization of the plumbing construction.
7 Further discussion
- ...
8 References
- [Macko&Wegner2008] T. Macko and C. Wegner, On the classification of fake lens spaces, to appear in Forum. Math. Available at the arXiv:0810.1196.
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003
 .
.
Let  be a free action of
be a free action of  on the sphere
on the sphere  . By
. By 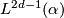 is denoted the orbit space of
is denoted the orbit space of  . Sometimes, when the dimension and the action are clear, we leave them from notation and simply write
. Sometimes, when the dimension and the action are clear, we leave them from notation and simply write  .
.
3 Invariants
For 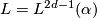 we have
we have
-
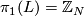 ,
, 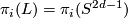 for
for 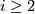
-
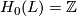 ,
, 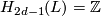 ,
, 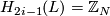 for
for 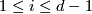 ,
, 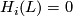 for all other values of
for all other values of  .
.
Interesting invariants for fake lens spaces are
- the Reidemeister torsion
![\Delta (L) \in \Qq [t] / (t^N - 1)](/images/math/e/1/4/e14c55dab04ab1ba91c3ddb4df51a1a1.png) and
and
- the
 -invariant
-invariant 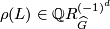 .
.
4 Homotopy Classification and simple homotopy classification
All the results are taken from chapter 14E of [Wall1999].
Notation
Recall the arithmetic (Rim) square:
![\displaystyle \xymatrix{ \Zz G \ar[r]^{\eta} \ar[d]_{\varepsilon} & R_G \ar[d]^{\varepsilon'} \\ \Zz \ar[r]_{\eta'} & \Zz_N }](/images/math/3/4/d/34da3249bf4eb2eac0505f1c05c1a32f.png)
where 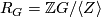 with
with 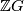 be the group ring of
be the group ring of  and
and  is the ideal generated by the norm element
is the ideal generated by the norm element 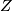 of
of  . The maps
. The maps  ,
, 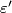 are the augmentation maps.
are the augmentation maps.
Recall that the Reidemeister torsion is a unit in 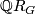 where
where 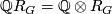 .
.
The homotopy classification is stated in the a priori broader context of finite CW-complexes  with
with 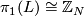 and with the universal cover homotopy equivalent to
and with the universal cover homotopy equivalent to  of which fake lens spaces are obviously a special case. It is convenient to make the
following definition.
of which fake lens spaces are obviously a special case. It is convenient to make the
following definition.
Definition 4.1.
Let  be a CW-complex with
be a CW-complex with 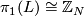 and with universal cover homotopy equivalent to
and with universal cover homotopy equivalent to  .
.
A polarization of  is a pair
is a pair 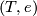 where
where  is a choice of a generator of
is a choice of a generator of 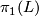 and
and  is a choice of a homotopy equivalence
is a choice of a homotopy equivalence 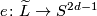 .
.
Recall the classical lens space 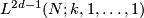 . By
. By 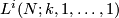 is denoted its
is denoted its  -skeleton with respect to the standard cell decomposition. If
-skeleton with respect to the standard cell decomposition. If  is odd this is a lens space, if
is odd this is a lens space, if  is even this is a CW-complex obtained by attaching an
is even this is a CW-complex obtained by attaching an  -cell to the lens space of dimension
-cell to the lens space of dimension 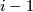 .
.
Theorem 4.2 Wall.
Let  be a finite CW-complex with
be a finite CW-complex with 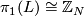 and universal cover
and universal cover  polarized by
polarized by 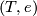 . Then there exists a simple homotopy equivalence
. Then there exists a simple homotopy equivalence
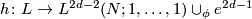
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 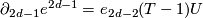 for some
for some
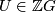 which maps to a unit
which maps to a unit  . Furthermore,
. Furthermore,  is a simple Poincare complex with Reidemeister torsion
is a simple Poincare complex with Reidemeister torsion 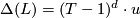 .
.
- The polarized homotopy types of such
 are in one-to-one correspondence with the units in
are in one-to-one correspondence with the units in 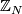 . The correspondence is given by
. The correspondence is given by 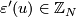 . The invariant
. The invariant 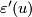 can be identified with the first non-trivial
can be identified with the first non-trivial  -invariant of
-invariant of  (in the sense of homotopy theory)
(in the sense of homotopy theory) 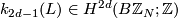 .
.
- The polarized simple homotopy types of such
 are in one-to-one correspondence with the units in
are in one-to-one correspondence with the units in 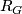 . The correspondence is given by
. The correspondence is given by  .
.
See [Wall1999, Theorem 14E.3].
The existence of a fake lens space in the homotopy type of such  is addressed in [Wall1999, Theorem 14E.4].
is addressed in [Wall1999, Theorem 14E.4].
Since the units 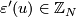 are exhausted by the lens spaces
are exhausted by the lens spaces
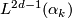 we obtain the following corollary.
we obtain the following corollary.
Corollary 4.3.
For any fake lens space 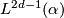 there exists
there exists 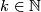 and a homotopy equivalence
and a homotopy equivalence
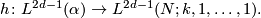
5 Homeomorphism classification
The homeomorphism classification, as already noted, is an excellent application of the non-simply connected surgery theory. Recall that for a topological manifold  the surgery theoretic homeomorphism classification of manifolds wihin the homotopy type of
the surgery theoretic homeomorphism classification of manifolds wihin the homotopy type of  is stated in terms of the surgery structure set
is stated in terms of the surgery structure set 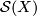 and that the primary tool for its calculation is the surgery exact sequence.
and that the primary tool for its calculation is the surgery exact sequence.
For a fake lens space  there is enough information about the normal invariants, the L-groups and the surgery obstruction in the surgery exact sequence so that one is left with just an extension problem. The strategy to proceed further is to relate the surgery exact sequence to representation theory of
there is enough information about the normal invariants, the L-groups and the surgery obstruction in the surgery exact sequence so that one is left with just an extension problem. The strategy to proceed further is to relate the surgery exact sequence to representation theory of  . This is done via the following commutative diagram of abelian groups and homomorphisms with exact rows
. This is done via the following commutative diagram of abelian groups and homomorphisms with exact rows
![\displaystyle \xymatrix{ 0 \ar[r] & {\widetilde L}^s_{2d} (G) \ar[r]^(0.4){\partial} \ar[d]_{\cong}^{G-sign} & {\mathcal S}^s (L^{2d-1}(\alpha)) \ar[r]^{\eta} \ar[d]^{\widetilde \rho}& \widetilde {\mathcal N} (L^{2d-1}(\alpha)) \ar[r] \ar[d]^{[\widetilde \rho]}& 0 \\ 0 \ar[r] & 4 \cdot R^{(-1)^d}_{\widehat G} \ar[r] & {\mathbb Q} R^{(-1)^d}_{\widehat G} \ar[r] & {\mathbb Q} R^{(-1)^d}_{\widehat G}/ 4 \cdot R^{(-1)^d}_{\widehat G} \ar[r] & 0 }](/images/math/c/e/0/ce0adafd80f8f574c18c1ece6266bff7.png)
where ![[\widetilde \rho]](/images/math/1/a/6/1a65cbd449686c64de628afc09f22dd2.png) is the homomorphism induced by
is the homomorphism induced by 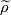 (see ?).
(see ?).
Theorem 5.1 Wall.
If  is odd, then the map
is odd, then the map
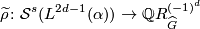
is injective.
See [Wall1999, Theorem 14E.7].
For  odd Wall managed to obtain an even beter result, namely the complete classification of fake lens spaces of a given dimension
odd Wall managed to obtain an even beter result, namely the complete classification of fake lens spaces of a given dimension 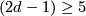 with the fundamental group
with the fundamental group 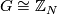 which goes as follows:
which goes as follows:
Theorem 5.2 Wall.
Let 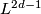 and
and 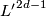 be oriented fake lens spaces with fundamental group
be oriented fake lens spaces with fundamental group  cyclic of odd order
cyclic of odd order  . Then there is an orientation preserving homeomorphism
. Then there is an orientation preserving homeomorphism 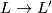 inducing the identity on
inducing the identity on  if and only if
if and only if 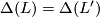 and
and  .
.
Given 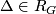 and
and 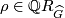 , there exists a corresponding fake lens space
, there exists a corresponding fake lens space 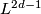 if and only if the following four statements hold:
if and only if the following four statements hold:
-
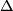 and
and  are both real (
are both real ( even) or imaginary (
even) or imaginary ( odd).
odd).
-
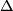 generates
generates 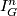 ,
, 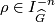 .
.
- The classes of
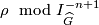 and
and 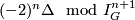 correspond under
correspond under
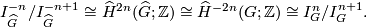
-
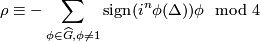 .
.
The following theorem is taken from [Wall1999, Theorem 14E.7].
For general  the following theorem is proved in [Macko&Wegner2008, Theorem 1.2]).
the following theorem is proved in [Macko&Wegner2008, Theorem 1.2]).
Theorem 5.3.
Let 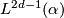 be a fake lens space with
be a fake lens space with 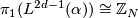 where
where 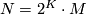 with
with 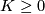 ,
,  odd and
odd and 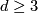 . Then we have
. Then we have

where 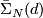 is a free abelian group. If
is a free abelian group. If  is odd then its rank is
is odd then its rank is 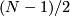 . If
. If  is even then its rank is
is even then its rank is 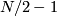 if
if 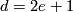 and
and  if
if 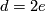 . In the torsion summand we have
. In the torsion summand we have 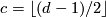 .
.
6 Construction and examples
Classical lens spaces are of course examples of fake lens spaces. To get fake lens spaces which are not homeomorphic to classical ones one can employ the construction of fake complex projective spaces. Note that a fake complex projective space is an orbit space of a free tame action of  on
on  and that we obviously have
and that we obviously have 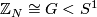 . Restricting the action to the subgroup we obtain a fake lens space. Its
. Restricting the action to the subgroup we obtain a fake lens space. Its  -invariant can be calculated by the naturality using the formula ...
-invariant can be calculated by the naturality using the formula ...
The above construction does not exhaust all the fake lens spaces. To get all of them there is a construction which produces from a given fake lens space  another fake lens space
another fake lens space 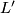 such that the difference of their
such that the difference of their  -invariants is a prescribed element
-invariants is a prescribed element
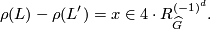
The construction is just the Wall realization from surgery theory, alias a non-simply connected generalization of the plumbing construction.
7 Further discussion
- ...
8 References
- [Macko&Wegner2008] T. Macko and C. Wegner, On the classification of fake lens spaces, to appear in Forum. Math. Available at the arXiv:0810.1196.
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003