Complex bordism
Taras Panov (Talk | contribs) |
Taras Panov (Talk | contribs) |
||
| Line 12: | Line 12: | ||
this, the complex cobordism theory found the most stricking and | this, the complex cobordism theory found the most stricking and | ||
important applications in algebraic topology and beyond. Many of | important applications in algebraic topology and beyond. Many of | ||
| − | these applications, including the [[#Formal group laws and genera|formal group techniques]] and [[#Adams-Novikov spectral sequence|Adams-Novikov spectral sequence]] were outlined in the pioneering work \cite{ | + | these applications, including the [[#Formal group laws and genera|formal group techniques]] and [[#Adams-Novikov spectral sequence|Adams-Novikov spectral sequence]] were outlined in the pioneering work \cite{Novikov1967}. |
</wikitex> | </wikitex> | ||
| Line 18: | Line 18: | ||
<wikitex>; | <wikitex>; | ||
A direct attempt to define the | A direct attempt to define the | ||
| − | [[Bordism and cobordism# | + | [[Bordism and cobordism#The bordism relation|bordism relation]] on complex manifolds fails because the manifold |
$W$ is odd-dimensional and therefore cannot be complex. In order to work with complex manifolds in the bordism theory, one needs to weaken the notion of the complex structures. This leads directly to considering ''stably complex'' (also known as | $W$ is odd-dimensional and therefore cannot be complex. In order to work with complex manifolds in the bordism theory, one needs to weaken the notion of the complex structures. This leads directly to considering ''stably complex'' (also known as | ||
''weakly almost complex'', ''stably almost complex'' or ''quasicomplex'') manifolds. | ''weakly almost complex'', ''stably almost complex'' or ''quasicomplex'') manifolds. | ||
| Line 67: | Line 67: | ||
== Definition of bordism and cobordism == | == Definition of bordism and cobordism == | ||
| + | <wikitex>; | ||
| + | The bordism relation can be defined between stably complex | ||
| + | manifolds. Like the case of unoriented bordisms, the set of | ||
| + | bordism classes $[M,c_{\mathcal T}]$ of stably complex manifolds | ||
| + | is an Abelian group with respect to the disjoint union. This group | ||
| + | is called the ''group of $n$-dimensional complex bordisms'' and | ||
| + | denoted $\varOmega^U_n$. A zero is represented by the bordism | ||
| + | class of any manifold $M$ which bounds and whose stable tangent | ||
| + | bundle is trivial (and therefore isomorphic to a product complex | ||
| + | vector bundle $M\times\mathbb C^k$). The sphere $S^n$ provides an example | ||
| + | of such a manifold. The opposite element to the bordism class | ||
| + | $[M,c_{\mathcal T}]$ in the group $\varOmega^U_n$ may be | ||
| + | represented by the same manifold $M$ with the stably complex | ||
| + | structure determined by the isomorphism | ||
| + | $$ | ||
| + | {\mathcal T}\!M\oplus\underline{\mathbb R}^k\oplus\underline{\mathbb R}^2\stackrel{c_{\mathcal T}\oplus | ||
| + | e}{\relbar\joinrel\hspace{-1pt}\relbar\joinrel\hspace{-1pt}\longrightarrow}\xi\oplus\underline{\mathbb C} | ||
| + | $$ | ||
| + | where $e\colon\mathbb R^2\to\mathbb C$ is given by $e(x,y)=x-iy$. | ||
| + | |||
| + | An abbreviated notation $[M]$ for the complex | ||
| + | bordism class will be used whenever the stably complex structure $c_{\mathcal | ||
| + | T}$ is clear from the context. | ||
| + | |||
| + | The ''groups of complex bordisms'' and ''cobordisms'' of a space | ||
| + | $X$ are defined similarly to the [[Bordism and cobordism#Unoriented bordism|unoriented]] case: | ||
| + | $$ | ||
| + | \begin{aligned} | ||
| + | U_n(X)&=\lim_{k\to\infty}\pi_{2k+n}((X_+)\wedge MU(k)),\\ | ||
| + | U^n(X)&=\lim_{k\to\infty}[\Sigma^{2k-n}(X_+),MU(k)] | ||
| + | \end{aligned} | ||
| + | $$ | ||
| + | where $MU(k)$ is the Thom space of the universal complex $k$-plane | ||
| + | bundle $EU(k)\to BU(k)$. These groups are $\varOmega_*^U$-modules | ||
| + | and give rise to a multiplicative [[Wikipedia:Homology_theory|(co)homology theory]]. In | ||
| + | particular, $U^*(X)=\oplus_n U^n(X)$ is a graded ring. The graded | ||
| + | ring $\varOmega^*_U$ with $\varOmega^{n}_U=\varOmega_{-n}^U$ is | ||
| + | called the ''complex cobordism ring''; it has nontrivial | ||
| + | elements only in nonpositively graded components. | ||
| + | </wikitex> | ||
== Geometric cobordisms == | == Geometric cobordisms == | ||
Revision as of 16:40, 10 March 2010
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 09:39, 1 April 2011 and the changes since publication. |
|
The user responsible for this page is Taras Panov. No other user may edit this page at present. |
Contents |
1 Introduction
Complex bordisms (also known as unitary bordisms) is the bordism theory of stably complex manifolds. It is one of the most important theory of bordisms with additional structure, or B-bordisms.
The theory of complex bordisms is much richer than its unoriented analogue, and at the same time is not as complicated as oriented bordisms or other bordisms with additional structure (B-bordisms). Thanks to this, the complex cobordism theory found the most stricking and important applications in algebraic topology and beyond. Many of these applications, including the formal group techniques and Adams-Novikov spectral sequence were outlined in the pioneering work [Novikov1967].
2 Stably complex structures
A direct attempt to define the
bordism relation on complex manifolds fails because the manifold
 is odd-dimensional and therefore cannot be complex. In order to work with complex manifolds in the bordism theory, one needs to weaken the notion of the complex structures. This leads directly to considering stably complex (also known as
weakly almost complex, stably almost complex or quasicomplex) manifolds.
is odd-dimensional and therefore cannot be complex. In order to work with complex manifolds in the bordism theory, one needs to weaken the notion of the complex structures. This leads directly to considering stably complex (also known as
weakly almost complex, stably almost complex or quasicomplex) manifolds.
Let 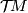 denote the tangent bundle of
denote the tangent bundle of  , and
, and 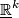 the product vector bundle
the product vector bundle 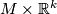 over
over  . A tangential stably complex structure on
. A tangential stably complex structure on  is
determined by a choice of an isomorphism
is
determined by a choice of an isomorphism
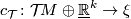
between the "stable" tangent bundle and a complex vector
bundle  over
over  . Some of the choices of such isomorphisms
are deemed to be equivalent, i.e. determining the same stably
complex structures (see details in Chapters II and VII of [Stong1968]). In
particular, two stably complex structures are equivalent if they
differ by a trivial complex summand. A normal stably complex structure on
. Some of the choices of such isomorphisms
are deemed to be equivalent, i.e. determining the same stably
complex structures (see details in Chapters II and VII of [Stong1968]). In
particular, two stably complex structures are equivalent if they
differ by a trivial complex summand. A normal stably complex structure on  is determined by a choice of a complex bundle
structure in the normal bundle
is determined by a choice of a complex bundle
structure in the normal bundle  of an embedding
of an embedding
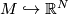 . A tangential and normal stably
complex structures on
. A tangential and normal stably
complex structures on  determine each other by means of the
canonical isomorphism
determine each other by means of the
canonical isomorphism 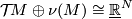 . We therefore may restrict
our attention to tangential structures only.
. We therefore may restrict
our attention to tangential structures only.
A stably complex manifold is a pair 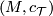 consisting of a manifold
consisting of a manifold  and a stably complex structure
and a stably complex structure
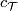 on it. This is a generalisation to a complex and
almost complex manifold (where the latter means a manifold
with a choice of a complex structure on
on it. This is a generalisation to a complex and
almost complex manifold (where the latter means a manifold
with a choice of a complex structure on 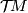 , i.e. a
stably complex structure
, i.e. a
stably complex structure 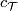 with
with  ).
).
Example 2.1.
Let 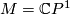 . The standard complex structure on
. The standard complex structure on  is
equivalent to a stably complex structure determined by the
isomorphism
is
equivalent to a stably complex structure determined by the
isomorphism
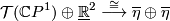
where  is the Hopf line bundle. On the other hand, the
isomorphism
is the Hopf line bundle. On the other hand, the
isomorphism
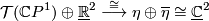
determines a trivial stably complex structure on 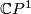 .
.
3 Definition of bordism and cobordism
The bordism relation can be defined between stably complex
manifolds. Like the case of unoriented bordisms, the set of
bordism classes ![[M,c_{\mathcal T}]](/images/math/b/2/f/b2fc6d73eb430a4b0c96071988365163.png) of stably complex manifolds
is an Abelian group with respect to the disjoint union. This group
is called the group of
of stably complex manifolds
is an Abelian group with respect to the disjoint union. This group
is called the group of  -dimensional complex bordisms and
denoted
-dimensional complex bordisms and
denoted 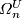 . A zero is represented by the bordism
class of any manifold
. A zero is represented by the bordism
class of any manifold  which bounds and whose stable tangent
bundle is trivial (and therefore isomorphic to a product complex
vector bundle
which bounds and whose stable tangent
bundle is trivial (and therefore isomorphic to a product complex
vector bundle 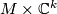 ). The sphere
). The sphere  provides an example
of such a manifold. The opposite element to the bordism class
provides an example
of such a manifold. The opposite element to the bordism class
![[M,c_{\mathcal T}]](/images/math/b/2/f/b2fc6d73eb430a4b0c96071988365163.png) in the group
in the group 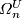 may be
represented by the same manifold
may be
represented by the same manifold  with the stably complex
structure determined by the isomorphism
with the stably complex
structure determined by the isomorphism
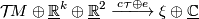
where 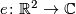 is given by
is given by  .
.
An abbreviated notation ![[M]](/images/math/f/a/0/fa08c3d5d2f54260952acc8a646b5025.png) for the complex
bordism class will be used whenever the stably complex structure
for the complex
bordism class will be used whenever the stably complex structure 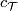 is clear from the context.
is clear from the context.
The groups of complex bordisms and cobordisms of a space
 are defined similarly to the unoriented case:
are defined similarly to the unoriented case:
![\displaystyle \begin{aligned} U_n(X)&=\lim_{k\to\infty}\pi_{2k+n}((X_+)\wedge MU(k)),\\ U^n(X)&=\lim_{k\to\infty}[\Sigma^{2k-n}(X_+),MU(k)] \end{aligned}](/images/math/3/8/8/388203cfec203d491a8ba0f9062a963f.png)
where 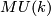 is the Thom space of the universal complex
is the Thom space of the universal complex  -plane
bundle
-plane
bundle  . These groups are
. These groups are 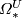 -modules
and give rise to a multiplicative (co)homology theory. In
particular,
-modules
and give rise to a multiplicative (co)homology theory. In
particular, 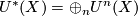 is a graded ring. The graded
ring
is a graded ring. The graded
ring 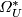 with
with 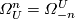 is
called the complex cobordism ring; it has nontrivial
elements only in nonpositively graded components.
is
called the complex cobordism ring; it has nontrivial
elements only in nonpositively graded components.
4 Geometric cobordisms
5 Structure results
6 Multiplicative generators
7 Formal group laws and genera
8 Adams-Novikov spectral sequence
9 References
- [Novikov1967] S. P. Novikov, Methods of algebraic topology from the point of view of cobordism theory, Math. USSR, Izv. 1, (1967) 827–913. MR0221509 (36 #4561) Zbl 0176.52401
- [Stong1968] R. E. Stong, Notes on cobordism theory, Princeton University Press, Princeton, N.J., 1968. MR0248858 (40 #2108) Zbl 0277.57010
|
This page has not been refereed. The information given here might be incomplete or provisional. |