Cell attachments for surgery (Ex)
From Manifold Atlas
(Difference between revisions)
m |
|||
| (4 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
<wikitex>; | <wikitex>; | ||
| − | The exercises on this page come directly from {{citeD|Lück2001|pp. 70- | + | The exercises on this page come directly from {{citeD|Lück2001|pp. 70-71}} and we use the notation found there. |
Let $f \colon Y \to X$ be a map of CW-complexes and let $\omega \in \pi_{k+1}(f)$ be represented by the following commutative diagram | Let $f \colon Y \to X$ be a map of CW-complexes and let $\omega \in \pi_{k+1}(f)$ be represented by the following commutative diagram | ||
| Line 11: | Line 11: | ||
{{beginthm|Exercise|{{citeD|Lück2001|Lemma 3.55}}}} | {{beginthm|Exercise|{{citeD|Lück2001|Lemma 3.55}}}} | ||
Let $f \colon Y \to X$ be a $(k-1)$-connected map of CW-complexes for some $k \geq 2$ where $X$ is connected. Consider a lift $\tilde f \colon \tilde Y \to \tilde X$ on the universal coverings. Show the following: | Let $f \colon Y \to X$ be a $(k-1)$-connected map of CW-complexes for some $k \geq 2$ where $X$ is connected. Consider a lift $\tilde f \colon \tilde Y \to \tilde X$ on the universal coverings. Show the following: | ||
| − | # The homology groups | + | # The natural map $\pi_k(\tilde f) \to \pi_k(f)$ is $\pi_1$-equivariant and bijective. |
| + | # The homology groups $H_k(\tilde f)$ are finitely generated $\Zz\pi_1(Y)$ modules. | ||
# One can make $f$ $k$-connected by attaching finitely many cells. | # One can make $f$ $k$-connected by attaching finitely many cells. | ||
{{endthm}} | {{endthm}} | ||
| + | The exercises on this page were sent by Nicolas Ginoux and Carolina Neira-Jiménez. | ||
</wikitex> | </wikitex> | ||
| − | |||
| − | |||
[[Category:Exercises]] | [[Category:Exercises]] | ||
| + | [[Category:Exercises without solution]] | ||
Latest revision as of 09:14, 1 April 2012
The exercises on this page come directly from [Lück2001, pp. 70-71] and we use the notation found there.
Let 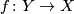 be a map of CW-complexes and let
be a map of CW-complexes and let  be represented by the following commutative diagram
be represented by the following commutative diagram
![\displaystyle \xymatrix{S^k \ar[r]^q \ar[d]_j & Y \ar[d]^f \\ D^{k+1} \ar[r]^{Q} & X}.](/images/math/9/7/f/97f432db013831026ac61bd3b5662601.png)
Let 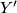 be the push out of
be the push out of  : i.e.
: i.e. 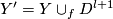 is the space obtained by attaching an
is the space obtained by attaching an 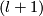 -cell to
-cell to  along
along  . There is an induced map
. There is an induced map
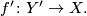
Exercise 0.1.
With notation above assume that  is
is  -connected with
-connected with  . Prove that the kernel of the surjection
. Prove that the kernel of the surjection 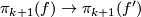 is the
is the 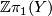 -module generated by
-module generated by  .
.
Exercise 0.2 [Lück2001, Lemma 3.55].
Let 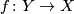 be a
be a 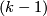 -connected map of CW-complexes for some
-connected map of CW-complexes for some  where
where  is connected. Consider a lift
is connected. Consider a lift  on the universal coverings. Show the following:
on the universal coverings. Show the following:
- The natural map
 is
is 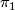 -equivariant and bijective.
-equivariant and bijective.
- The homology groups
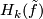 are finitely generated
are finitely generated  modules.
modules.
- One can make

 -connected by attaching finitely many cells.
-connected by attaching finitely many cells.
The exercises on this page were sent by Nicolas Ginoux and Carolina Neira-Jiménez.