Whitehead torsion V (Ex)

Exercise 0.1.

1. Show that the operations (1)-(5) from [Lück2001, Section 1.4] used in the definition of the Whitehead group in fact yield equivalence classes of invertible matrices of arbitrary size.
2. Show that addition is well-defined in the Whitehead group via

$$\displaystyle [A] + [B] = \left [\begin{array}{cc} A & 0 \\ 0 & B \end{array} \right] = [A \cdot (B \oplus \text{Id}_{n-m})].$$

Here $<wikitex>; {{beginthm|Exercise}} # Show that the operations (1)-(5) from {{citeD|Lück2001|Section 1.4}} used in the definition of the Whitehead group in fact yield equivalence classes of invertible matrices of arbitrary size. # Show that addition is well-defined in the Whitehead group via $$ [A] + [B] = \left [\begin{array}{cc} A & 0 \ 0 & B \end{array} \right] = [(A) \cdot (B \oplus \text{Id}_{n-m})].$$ Here $A$ is an $n \times n$ matrix, $B$ is an $m \times m$ matrix with $n \geq m$, $O$ denotes the zero $n \times m$ and $m \times n$ matricies and $\cdot$ denotes matrix multiplication. # Show that $Wh(e)$ is trivial. {{endthm}} The exercises and hints on this page were sent by Alex Koenen and Arkadi Schelling. 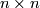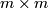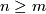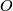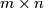</wikitex> [[Category:Exercises]]A$ and $B$ are invertible matricies over the group ring $\Zz[\pi]$, $A$ is an $n \times n$ matrix, $B$ is an $m \times m$ matrix with $n \geq m$, $O$ denotes the zero $n \times m$ and $m \times n$ matricies and $\cdot$ denotes matrix multiplication.

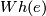1. Show that $Wh(e)$ is trivial.