Tangent bundles of bundles (Ex)
From Manifold Atlas
(Difference between revisions)
| (2 intermediate revisions by one user not shown) | |||
| Line 19: | Line 19: | ||
{{endthm}} | {{endthm}} | ||
| − | As an explicit example, recall that there are principal bundles $S^1 \to S^{4k+3} \to \CP^{2k+1}$ and $S^3 \to S^{4k+ | + | As an explicit example, recall that there are principal bundles $S^1 \to S^{4k+3} \to \CP^{2k+1}$ and $S^3 \to S^{4k+3} \to \Hh P^k$. It follows that there is an $S^2$ fibre bundle $S^2 \to \CP^{2k+1} \to \Hh P^k$ with structure group $S^3$ acting on $S^2$ via $S^3 \to S^3/S^1 \cong S^2$. |
{{beginthm|Exercise}} Compute the total Pontrjagin class of $\Hh P^n$, [[Wikipedia:Quaternionic_projective_space|quaternionic projective space]]. (This was first achieved in {{citeD|Hirzebruch1953}}). | {{beginthm|Exercise}} Compute the total Pontrjagin class of $\Hh P^n$, [[Wikipedia:Quaternionic_projective_space|quaternionic projective space]]. (This was first achieved in {{citeD|Hirzebruch1953}}). | ||
</wikitex> | </wikitex> | ||
| − | == References == | + | <!-- == References == |
| − | {{#RefList:}} | + | {{#RefList:}} --> |
[[Category:Exercises]] | [[Category:Exercises]] | ||
| + | [[Category:Exercises with solution]] | ||
Latest revision as of 22:28, 29 May 2012
Let  be a smooth fiber bundle so that all spaces
be a smooth fiber bundle so that all spaces  and
and  are manifolds.
are manifolds.
Exercise 0.1.
Show that  , the tangent bundle of
, the tangent bundle of  , splits as the sum of two bundles
, splits as the sum of two bundles
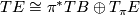
where 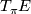 consists of those tangent vectors tangent to the fibres of
consists of those tangent vectors tangent to the fibres of  .
.
Question 0.2.
Is the bundle 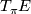 the pullback of some bundle over
the pullback of some bundle over  ?
?
Exercise 0.3.
Suppose that 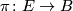 is itself a smooth vector bundle. Determine
is itself a smooth vector bundle. Determine  in terms of
in terms of 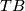 and
and  regarded as a vector bundle.
regarded as a vector bundle.
Exercise 0.4.
Suppose that 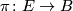 is the sphere bundle of a vector bundle. Determine the stable tangent bundle of
is the sphere bundle of a vector bundle. Determine the stable tangent bundle of  in terms of
in terms of  and
and 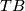 .
.
As an explicit example, recall that there are principal bundles 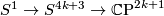 and
and 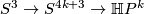 . It follows that there is an
. It follows that there is an  fibre bundle
fibre bundle 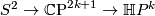 with structure group
with structure group  acting on
acting on  via
via 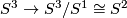 .
.
Exercise 0.5. Compute the total Pontrjagin class of 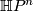 , quaternionic projective space. (This was first achieved in [Hirzebruch1953]).
, quaternionic projective space. (This was first achieved in [Hirzebruch1953]).
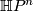 , quaternionic projective space. (This was first achieved in [Hirzebruch1953]).
, quaternionic projective space. (This was first achieved in [Hirzebruch1953]).