Talk:Normal bordism - definitions (Ex)
In both parts let  be a connected finite Poincare complex of dimension
be a connected finite Poincare complex of dimension  and let
and let 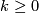 .
.
Part 1
The following definition of the set of normal maps 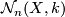 is similar to [Lück2001, Definition 3.46].
We define
is similar to [Lück2001, Definition 3.46].
We define
![\displaystyle \mathcal{N}_n(X,k):= \left\{ [\xi,M,i,f,\overline{f}] | \begin{array}{l} \xi\textrm{ vector bundle of rank }k\textrm{ over }X,\, M\textrm{ closed manifold of dimension }n,\, i:\,M\to\mathbb{R}^{n+k}\textrm{ embedding},\, (f,\overline{f}):\,\nu(M,i)\to\xi\textrm{ bundle map},\, f:\,M\to X\textrm{ of degree }1 \end{array} \right\}](/images/math/9/5/e/95eac2d8c047fc766e68218c6e2cd0fc.png)
where we identify 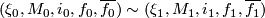 iff
iff
1) There exists a compact manifold  of dimension
of dimension 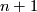 whose boundary can be written as
whose boundary can be written as  .
.
2) There exists an embedding  :
: ![W\to\mathbb{R}^{n+k}\times[0,1]](/images/math/a/b/1/ab18bc810bf5b65819b8394370060ae0.png) such that for
such that for 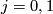 we have
we have
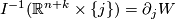 and
and  meets
meets 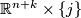 transversally.
transversally.
3) There exists a vector bundle  :
: ![E'\to X\times[0,1]](/images/math/8/6/f/86f9e8c19953967e1608f5175d2d4f00.png) of rank
of rank  and for
and for 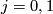 there exist vector bundle isomorphisms
there exist vector bundle isomorphisms 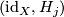 :
: 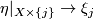 .
.
4) There exists a bundle map 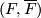 :
: 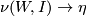 such that for
such that for 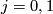 we have
we have 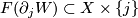 and such that
and such that  :
: ![(W,\partial W)\to(X\times[0,1],X\times\partial[0,1])](/images/math/1/9/5/19515ecbc55dfa41d99b7f8f412e4624.png) has degree one as a map between Poincare pairs.
has degree one as a map between Poincare pairs.
5) For 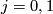 there exist diffeomorphisms
there exist diffeomorphisms 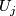 :
: 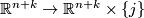 such that
such that
a) 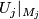 :
: 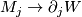 is a diffeomorphism
is a diffeomorphism
b) 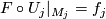
c) the induced bundle map 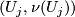 :
: 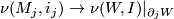 satisfies
satisfies 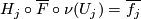 .
.
Part 2
The following definition of the set of tangential normal maps  differs from [Lück2001, Definition 3.50].
We define
differs from [Lück2001, Definition 3.50].
We define
![\displaystyle \mathcal{N}^T_n(X,k):= \left\{ [\xi,M,a,f,\overline{f}] | \begin{array}{l} \xi\textrm{ vector bundle of rank }k\textrm{ over }X,\, M\textrm{ closed manifold of dimension }n,\, a\in\mathbb{N}_0,\, (f,\overline{f}):\,TM\oplus\underline{\mathbb{R}^a}\to\xi\textrm{ bundle map},\, f:\,M\to X\textrm{ of degree }1 \end{array} \right\}](/images/math/1/c/d/1cd45eeb72d7fe8f65eded6c4e63162e.png)
where we identify 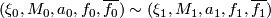 iff
iff
1) There exists a compact manifold  of dimension
of dimension 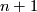 whose boundary can be written as
whose boundary can be written as  .
.
2) There exists a vector bundle  :
: ![E'\to X\times[0,1]](/images/math/8/6/f/86f9e8c19953967e1608f5175d2d4f00.png) and there exist
and there exist 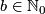 and
a bundle map
and
a bundle map 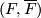 :
: 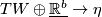 such that for
such that for 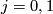 we have
we have 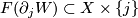 and such that
and such that  :
: ![(W,\partial W)\to(X\times[0,1],X\times\partial[0,1])](/images/math/1/9/5/19515ecbc55dfa41d99b7f8f412e4624.png) has degree one as a map between Poincare pairs.
has degree one as a map between Poincare pairs.
3) For 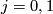 there exist diffeomorphisms
there exist diffeomorphisms 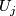 :
: 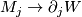 such that
such that 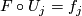 .
.
4) For 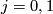 there exist bundle isomorphisms
there exist bundle isomorphisms 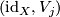 :
: 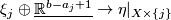 such that
such that
![\displaystyle \xymatrix{TM_j\oplus\underline{\mathbb{R}}\oplus\underline{\mathbb{R}^b} \ar[rr]^{\overline{f_j}\oplus\mathrm{id}_{\underline{\mathbb{R}^{b-a_j+1}}}} \ar[d]_{TU_j\oplus n_j\oplus\mathrm{id}_{\underline{\mathbb{R}^b}}} & & \xi_j\oplus\underline{\mathbb{R}^{b-a_j+1}} \ar[d]^{V_j}\\ TW|_{\partial_jW}\oplus\underline{\mathbb{R}^b} \ar[rr]^{\overline{F}|_{\partial_jW}} & & \eta|_{X\times\{j\}} }](/images/math/7/4/e/74e20705c840b6ca855d5ac331779b90.png)
commutes.
Here 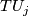 :
: 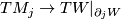 is the differential of
is the differential of 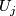 and
and 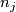 :
: 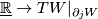 is given by an inward normal field of
is given by an inward normal field of 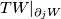 .
.