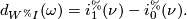Structured chain complexes III (Ex)
From Manifold Atlas
(Difference between revisions)
(Created page with "<wikitex>; Let $(\bar \Zz,\nu)$ be the $0$-dimensional symmetric complex where $$ \bar \Zz_i := \begin{cases} \Zz & i = 0 \\ 0 & i \neq 0, \end{...") |
m |
||
| Line 14: | Line 14: | ||
$$ d_{W^{\%}{I}} (\omega) = i_1^{\%} (\nu) - i_0^{\%} (\nu). $$ | $$ d_{W^{\%}{I}} (\omega) = i_1^{\%} (\nu) - i_0^{\%} (\nu). $$ | ||
</wikitex> | </wikitex> | ||
| − | == References == | + | == References== |
{{#RefList:}} | {{#RefList:}} | ||
[[Category:Exercises]] | [[Category:Exercises]] | ||
[[Category:Exercises without solution]] | [[Category:Exercises without solution]] | ||
Latest revision as of 12:46, 30 July 2013
Let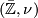 
be the 
-dimensional symmetric complex where
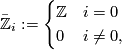
and the symmetric structure is given by
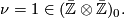
Let 
be the cellular chain complex of the unit interval and let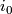 
, 
be the inclusions of the two ends. Find a 
-dimensional chain 
such that
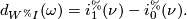
References
$-dimensional symmetric complex where $$ \bar \Zz_i := \begin{cases} \Zz & i = 0 \ 0 & i \neq 0, \end{cases} $$ and the symmetric structure is given by $$ \nu = 1 \in (\bar \Zz \otimes \bar \Zz)_0. $$ Let $I$ be the cellular chain complex of the unit interval and let $i_0$, $i_1$ be the inclusions of the two ends. Find a (\bar \Zz,\nu) be the-dimensional symmetric complex where
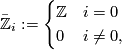
and the symmetric structure is given by
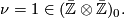
Let be the cellular chain complex of the unit interval and let 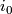, be the inclusions of the two ends. Find a -dimensional chain such that
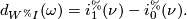
References
$-dimensional chain $\omega \in W^{\%}(I)$ such that $$ d_{W^{\%}{I}} (\omega) = i_1^{\%} (\nu) - i_0^{\%} (\nu). $$ == References == {{#RefList:}} [[Category:Exercises]] [[Category:Exercises without solution]](\bar \Zz,\nu) be the-dimensional symmetric complex where
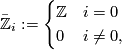
and the symmetric structure is given by
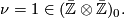
Let be the cellular chain complex of the unit interval and let 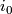, be the inclusions of the two ends. Find a -dimensional chain such that
