Spivak normal fibration (Ex)
From Manifold Atlas
(Difference between revisions)
m |
m |
||
| (4 intermediate revisions by one user not shown) | |||
| Line 1: | Line 1: | ||
<wikitex>; | <wikitex>; | ||
| − | In the following exercises $X$ | + | In the following exercises $X$ is a connected Poincaré complex of formal dimension $n$ and $M$ is a compact manifold of dimension $n$. |
| + | |||
{{beginthm|Exercise}} | {{beginthm|Exercise}} | ||
| + | Let $(M, \partial M)$ be a compact, connected, oriented, $n$-dimensional manifold with boundary, embedded in the $n$-sphere $S^n$. The collapse map $c:S^n\to M/\partial M$ is defined by | ||
| + | $$c(x)=\begin{cases} x&\text{if }x\in M-\partial M\\\{\partial M\}&\text{else}\end{cases}$$ | ||
| + | Let $h:\pi_n(M, \partial M)\to H_n(M, \partial M)$ be the [[Hurewicz homomorphism]], show that | ||
| + | $$h([c])=\pm [M,\partial M]$$ | ||
| + | {{endthm}} | ||
| + | |||
| + | {{beginthm|Exercise}} \label{ex1} | ||
Let $\xi \colon E \to X$ be a spherical fibration $X$ with homotopy fibre $S^k$. Show that $E$ is homotopy equivalent to a Poincaré complex of formal dimension $n + k$. | Let $\xi \colon E \to X$ be a spherical fibration $X$ with homotopy fibre $S^k$. Show that $E$ is homotopy equivalent to a Poincaré complex of formal dimension $n + k$. | ||
{{endthm}} | {{endthm}} | ||
| Line 8: | Line 16: | ||
Determine the Spivak normal fibration of $E$ above in terms of $\xi$ and the Spivak normal fibration of $X$. | Determine the Spivak normal fibration of $E$ above in terms of $\xi$ and the Spivak normal fibration of $X$. | ||
{{endthm}} | {{endthm}} | ||
| + | |||
Here are some hints for this problem: [[Tangent bundles of bundles (Ex)]], {{citeD|Wall1966a}}, {{citeD|Chazin1975}} | Here are some hints for this problem: [[Tangent bundles of bundles (Ex)]], {{citeD|Wall1966a}}, {{citeD|Chazin1975}} | ||
| − | {{ | + | |
| − | + | {{beginrem|Remark}} | |
| − | {{ | + | Exercise \ref{ex1} is a diffcult problem. It was solved in greater generality in {{citeD|Klein2001|Theorem I}}. |
| + | {{endrem}} | ||
</wikitex> | </wikitex> | ||
<!-- == References == | <!-- == References == | ||
Latest revision as of 07:41, 30 May 2012
 is a connected Poincaré complex of formal dimension
is a connected Poincaré complex of formal dimension  and
and Tex syntax erroris a compact manifold of dimension
 .
.
Exercise 0.1.
Let  be a compact, connected, oriented,
be a compact, connected, oriented,  -dimensional manifold with boundary, embedded in the
-dimensional manifold with boundary, embedded in the  -sphere
-sphere  . The collapse map
. The collapse map 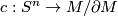 is defined by
is defined by
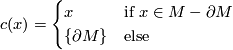
Let  be the Hurewicz homomorphism, show that
be the Hurewicz homomorphism, show that
![\displaystyle h([c])=\pm [M,\partial M]](/images/math/2/e/3/2e3fa87f344c35e33af28fa205bdcead.png)
Exercise 0.2.
Let 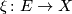 be a spherical fibration
be a spherical fibration  with homotopy fibre
with homotopy fibre 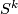 . Show that
. Show that  is homotopy equivalent to a Poincaré complex of formal dimension
is homotopy equivalent to a Poincaré complex of formal dimension  .
.
Here is an interesting problem we now confront
Problem 0.3.
Determine the Spivak normal fibration of  above in terms of
above in terms of  and the Spivak normal fibration of
and the Spivak normal fibration of  .
.
Here are some hints for this problem: Tangent bundles of bundles (Ex), [Wall1966a], [Chazin1975]