Plumbing (Ex)
The exercises below are about plumbing manifolds. For the details of the construction, see the page Plumbing. In this page we use slightly different notation. For 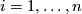 let
let  be a closed connected oriented manifold of dimension
be a closed connected oriented manifold of dimension 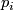 and let
and let

be an oriented 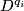 -bundle over
-bundle over  where
where 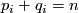 is fixed and each
is fixed and each 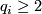 . Let
. Let  be a graph with verticies
be a graph with verticies  where the edge set between
where the edge set between 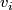 and
and 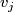 is non-empty only if
is non-empty only if 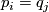 and
and 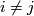 .
.
Starting from the dijoint union of the total spaces 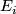 we form a manifolds as follows: given an edge in
we form a manifolds as follows: given an edge in  connecting
connecting 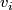 and
and 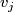 , let
, let 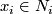 and let
and let 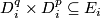 be a neighbourhood of
be a neighbourhood of 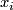 , such that
, such that 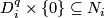 and
and 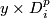 are the fibers of
are the fibers of 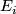 . Let
. Let 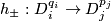 and
and 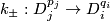 be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing
be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing 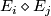 of
of 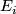 and
and 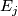 at
at 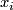 and
and 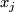 by taking
by taking 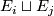 and identifying
and identifying 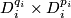 and
and 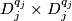 via
via
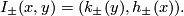
Proceeding in this way for each edge of  we obtain the plumbing manifold
we obtain the plumbing manifold
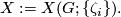
Exercise 0.1.
Let 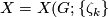 be connected. Show the following:
be connected. Show the following:
 is free.
is free.
 for
for 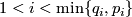 ??
??
Hint 0.2.
The statement is trivial for  , since
, since  is homotopy equivalent to a wedge of 1-spheres and
is homotopy equivalent to a wedge of 1-spheres and 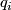 -spheres. Now use van Kampen's theorem for
-spheres. Now use van Kampen's theorem for 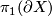 and for
and for 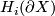 use the Mayer-Vietories Sequence with
use the Mayer-Vietories Sequence with 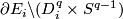 and show that all components involved are
and show that all components involved are 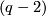 connected.
connected.
Exercise 0.3.
Choose 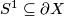 representing a generator of
representing a generator of 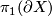 and let
and let  be the trace of a surgery on this
be the trace of a surgery on this  . Define
. Define 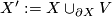 . Show that
. Show that
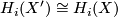 for
for  and
and
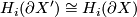 for
for 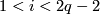
Hint 0.4.
For (1) use the long exact sequence of the pair 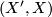 and
and 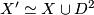 . For (2) use the long exact sequence of the pair
. For (2) use the long exact sequence of the pair 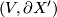 as well as Poincaré Duality and the Universal Coefficient Theorem.
as well as Poincaré Duality and the Universal Coefficient Theorem.
Exercise 0.5.
Assume now that each 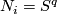 and that each bundle
and that each bundle 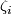 is some multiple of
is some multiple of  , the unit disc bundle of the tangent bundle of the
, the unit disc bundle of the tangent bundle of the  -sphere. Show that there is a degree 1 normal map
-sphere. Show that there is a degree 1 normal map 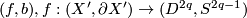 .
.
Hint 0.6.
Use that  is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of
is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of  is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of
is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of 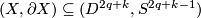 is trivial for
is trivial for  large. Then show that the map can be extend over
large. Then show that the map can be extend over  .
.
References
 be a closed connected oriented manifold of dimension
be a closed connected oriented manifold of dimension 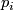 and let
and let

be an oriented 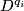 -bundle over
-bundle over  where
where 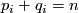 is fixed and each
is fixed and each 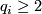 . Let
. Let  be a graph with verticies
be a graph with verticies  where the edge set between
where the edge set between 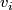 and
and 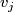 is non-empty only if
is non-empty only if 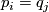 and
and 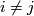 .
.
Starting from the dijoint union of the total spaces 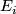 we form a manifolds as follows: given an edge in
we form a manifolds as follows: given an edge in  connecting
connecting 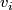 and
and 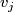 , let
, let 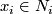 and let
and let 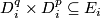 be a neighbourhood of
be a neighbourhood of 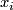 , such that
, such that 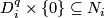 and
and 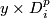 are the fibers of
are the fibers of 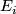 . Let
. Let 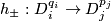 and
and 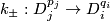 be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing
be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing 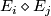 of
of 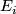 and
and 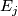 at
at 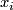 and
and 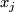 by taking
by taking 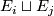 and identifying
and identifying 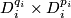 and
and 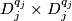 via
via
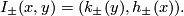
Proceeding in this way for each edge of  we obtain the plumbing manifold
we obtain the plumbing manifold
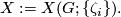
Exercise 0.1.
Let 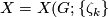 be connected. Show the following:
be connected. Show the following:
 is free.
is free.
 for
for 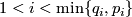 ??
??
Hint 0.2.
The statement is trivial for  , since
, since  is homotopy equivalent to a wedge of 1-spheres and
is homotopy equivalent to a wedge of 1-spheres and 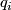 -spheres. Now use van Kampen's theorem for
-spheres. Now use van Kampen's theorem for 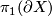 and for
and for 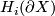 use the Mayer-Vietories Sequence with
use the Mayer-Vietories Sequence with 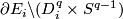 and show that all components involved are
and show that all components involved are 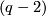 connected.
connected.
Exercise 0.3.
Choose 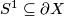 representing a generator of
representing a generator of 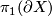 and let
and let  be the trace of a surgery on this
be the trace of a surgery on this  . Define
. Define 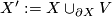 . Show that
. Show that
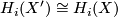 for
for  and
and
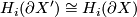 for
for 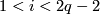
Hint 0.4.
For (1) use the long exact sequence of the pair 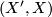 and
and 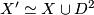 . For (2) use the long exact sequence of the pair
. For (2) use the long exact sequence of the pair 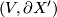 as well as Poincaré Duality and the Universal Coefficient Theorem.
as well as Poincaré Duality and the Universal Coefficient Theorem.
Exercise 0.5.
Assume now that each 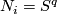 and that each bundle
and that each bundle 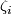 is some multiple of
is some multiple of  , the unit disc bundle of the tangent bundle of the
, the unit disc bundle of the tangent bundle of the  -sphere. Show that there is a degree 1 normal map
-sphere. Show that there is a degree 1 normal map 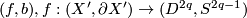 .
.
Hint 0.6.
Use that  is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of
is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of  is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of
is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of 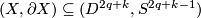 is trivial for
is trivial for  large. Then show that the map can be extend over
large. Then show that the map can be extend over  .
.
References
 be a closed connected oriented manifold of dimension
be a closed connected oriented manifold of dimension 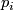 and let
and let

be an oriented 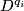 -bundle over
-bundle over  where
where 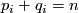 is fixed and each
is fixed and each 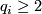 . Let
. Let  be a graph with verticies
be a graph with verticies  where the edge set between
where the edge set between 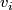 and
and 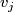 is non-empty only if
is non-empty only if 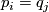 and
and 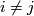 .
.
Starting from the dijoint union of the total spaces 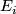 we form a manifolds as follows: given an edge in
we form a manifolds as follows: given an edge in  connecting
connecting 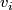 and
and 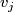 , let
, let 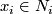 and let
and let 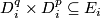 be a neighbourhood of
be a neighbourhood of 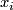 , such that
, such that 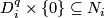 and
and 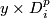 are the fibers of
are the fibers of 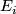 . Let
. Let 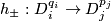 and
and 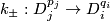 be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing
be orientation preserving (resp. reversing) diffeomorphisms. We define the plumbing 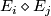 of
of 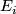 and
and 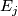 at
at 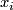 and
and 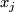 by taking
by taking 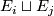 and identifying
and identifying 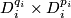 and
and 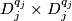 via
via
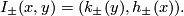
Proceeding in this way for each edge of  we obtain the plumbing manifold
we obtain the plumbing manifold
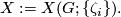
Exercise 0.1.
Let 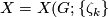 be connected. Show the following:
be connected. Show the following:
 is free.
is free.
 for
for 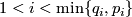 ??
??
Hint 0.2.
The statement is trivial for  , since
, since  is homotopy equivalent to a wedge of 1-spheres and
is homotopy equivalent to a wedge of 1-spheres and 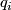 -spheres. Now use van Kampen's theorem for
-spheres. Now use van Kampen's theorem for 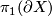 and for
and for 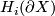 use the Mayer-Vietories Sequence with
use the Mayer-Vietories Sequence with 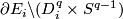 and show that all components involved are
and show that all components involved are 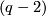 connected.
connected.
Exercise 0.3.
Choose 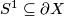 representing a generator of
representing a generator of 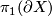 and let
and let  be the trace of a surgery on this
be the trace of a surgery on this  . Define
. Define 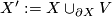 . Show that
. Show that
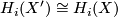 for
for  and
and
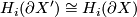 for
for 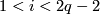
Hint 0.4.
For (1) use the long exact sequence of the pair 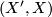 and
and 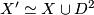 . For (2) use the long exact sequence of the pair
. For (2) use the long exact sequence of the pair 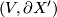 as well as Poincaré Duality and the Universal Coefficient Theorem.
as well as Poincaré Duality and the Universal Coefficient Theorem.
Exercise 0.5.
Assume now that each 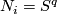 and that each bundle
and that each bundle 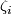 is some multiple of
is some multiple of  , the unit disc bundle of the tangent bundle of the
, the unit disc bundle of the tangent bundle of the  -sphere. Show that there is a degree 1 normal map
-sphere. Show that there is a degree 1 normal map 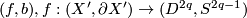 .
.
Hint 0.6.
Use that  is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of
is homotopy equivalent to a wedge of 1-spheres and q-spheres and that the tangent bundle of  is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of
is stabily trivial (since it is so on every component of the wedge), so that the normal bundle of 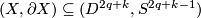 is trivial for
is trivial for  large. Then show that the map can be extend over
large. Then show that the map can be extend over  .
.