Oriented cover
(Added two links to other pages) |
|||
| Line 2: | Line 2: | ||
== Introduction == | == Introduction == | ||
<wikitex>; | <wikitex>; | ||
| − | This page is based on \cite{Ranicki2002|Definition 4.56}. Let $p:\widetilde{X} \to X$ be a regular covering of a connected space with orientation character $w(X)\in H^1(X;\Zz_2) = \Hom(\pi_1(X),\Zz_2)$. Let $\pi$ denote the group of covering translations. Since $\widetilde{X}$ is a regular cover $\pi_1(\widetilde{X})$ is a normal subgroup of $\pi_1(X)$ and $\pi \cong \pi_1(X)/\pi_1(\widetilde{X})$ (See \cite{Hatcher2002|Proposition 1.39}). Let $q: \pi_1(X) \to \pi$ denote the quotient map. The orientation character of the cover factors as $$w(\widetilde{X}) = w(X)\circ p_*,$$ which corresponds to the intuition that the cover $\widetilde{X}$ is orientable if all loops in $\widetilde{X}$ project to orientable loops in $X$. | + | This page is based on \cite{Ranicki2002|Definition 4.56}. Let $p:\widetilde{X} \to X$ be a regular covering of a connected space with [[Orientation character|orientation character]] $w(X)\in H^1(X;\Zz_2) = \Hom(\pi_1(X),\Zz_2)$. Let $\pi$ denote the group of covering translations. Since $\widetilde{X}$ is a regular cover $\pi_1(\widetilde{X})$ is a normal subgroup of $\pi_1(X)$ and $\pi \cong \pi_1(X)/\pi_1(\widetilde{X})$ (See \cite{Hatcher2002|Proposition 1.39}). Let $q: \pi_1(X) \to \pi$ denote the quotient map. The orientation character of the cover factors as $$w(\widetilde{X}) = w(X)\circ p_*,$$ which corresponds to the intuition that the cover $\widetilde{X}$ is orientable if all loops in $\widetilde{X}$ project to orientable loops in $X$. |
{{beginthm|Lemma}} | {{beginthm|Lemma}} | ||
| Line 27: | Line 27: | ||
== Examples == | == Examples == | ||
<wikitex>; | <wikitex>; | ||
| − | The two most important examples of oriented covers are the universal cover $(\widetilde{X},\pi_1(X),w(X))$ and the orientation double cover $(X^w,\Zz_2,\id_{\Zz_2})$. These correspond to the two extreme cases of factoring the orientation character via $\pi_1(X)$ and $\Zz_2$ respectively. Every oriented cover is a regular cover of $X^w$ and has $\widetilde{X}$ as a regular cover. | + | The two most important examples of oriented covers are the universal cover $(\widetilde{X},\pi_1(X),w(X))$ and the [[Orientation covering|orientation double cover]] $(X^w,\Zz_2,\id_{\Zz_2})$. These correspond to the two extreme cases of factoring the orientation character via $\pi_1(X)$ and $\Zz_2$ respectively. Every oriented cover is a regular cover of $X^w$ and has $\widetilde{X}$ as a regular cover. |
</wikitex> | </wikitex> | ||
Revision as of 17:03, 19 April 2013
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
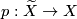 be a regular covering of a connected space with orientation character
be a regular covering of a connected space with orientation character 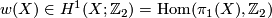 . Let
. Let  denote the group of covering translations. Since
denote the group of covering translations. Since 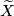 is a regular cover
is a regular cover  is a normal subgroup of
is a normal subgroup of 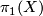 and
and 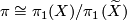 (See [Hatcher2002, Proposition 1.39]). Let
(See [Hatcher2002, Proposition 1.39]). Let 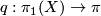 denote the quotient map. The orientation character of the cover factors as
denote the quotient map. The orientation character of the cover factors as 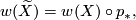
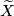 is orientable if all loops in
is orientable if all loops in 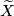 project to orientable loops in
project to orientable loops in  .
.
Lemma 1.1.
The cover 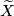 is orientable if and only if the orientation character
is orientable if and only if the orientation character 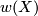 factors through
factors through  .
.
Proof. Consider the diagram
![\displaystyle \xymatrix{ \pi_1(\widetilde{X}) \ar[dr]^-{w(\widetilde{X})} \ar[d] ^-{p_*}& \\ \pi_1(X) \ar[d]^-{q}\ar[r]^{w(X)} & \Zz_2 \\ \pi \ar@{-->}[ur]^-{w} & }](/images/math/b/6/4/b64ea561411c95b6573af97daff2a0cf.png)
If there exists a  such that
such that 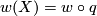 , then
, then 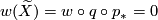 . Conversely if
. Conversely if 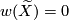 then the map
then the map
![\displaystyle \begin{array}{rcl} w:\pi & \to & \Zz_2 \\ {[\alpha]} & \mapsto & w(X)(\alpha)\end{array}](/images/math/d/5/1/d5172ae95d0e25d03d671e7163b9093a.png)
for any representative  of
of ![[\alpha]\in \pi](/images/math/f/2/d/f2db939a6cc390c32c31277802a708da.png) is well defined and factors
is well defined and factors 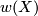 .
.

In light of this we make the following definition.
2 Definition
An oriented cover 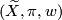 of a (connected) space
of a (connected) space  with an orientation character
with an orientation character 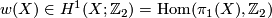 is a regular covering of
is a regular covering of  with group of covering translations
with group of covering translations  , together with an orientation character
, together with an orientation character 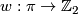 such that
such that
![\displaystyle \xymatrix{ w(X): \pi_1(X) \ar[r] & \pi \ar[r]^-{w} & \Zz_2. }](/images/math/b/e/9/be97428a3e1bede98322d68b277a57c9.png)
3 Examples
The two most important examples of oriented covers are the universal cover 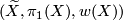 and the orientation double cover
and the orientation double cover 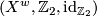 . These correspond to the two extreme cases of factoring the orientation character via
. These correspond to the two extreme cases of factoring the orientation character via 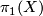 and
and  respectively. Every oriented cover is a regular cover of
respectively. Every oriented cover is a regular cover of 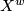 and has
and has 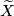 as a regular cover.
as a regular cover.
4 References
- [Hatcher2002] A. Hatcher, Algebraic topology, Cambridge University Press, 2002. MR1867354 (2002k:55001) Zbl 1044.55001
- [Ranicki2002] A. Ranicki, Algebraic and geometric surgery, The Clarendon Press Oxford University Press, Oxford, 2002. MR2061749 (2005e:57075) Zbl 1003.57001