Intersection form
(Created page with '<!-- COMMENT: To achieve a unified layout, along with using the template below, please OBSERVE the following: besides, $...$ and $$...$$, you should use two environments: - Fo…') |
|||
| Line 25: | Line 25: | ||
$q$ descends to a bilinear pairing on the free module $H^{2n}(X;\mathbb{Z}) / \text{Torsion}$. It is a symmetric and unimodular (in particular non-degenerate) pairing, the latter follows from Poincaré duality. | $q$ descends to a bilinear pairing on the free module $H^{2n}(X;\mathbb{Z}) / \text{Torsion}$. It is a symmetric and unimodular (in particular non-degenerate) pairing, the latter follows from Poincaré duality. | ||
| − | == Algebraic | + | == Algebraic invariants == |
Let $q$ and $q'$ be unimodular symmetric bilinear forms on underlying free $\mathbb{Z}$-modules $V$ and $V'$ respectively. The two forms $q$ and $q'$ are said equivalent if there is an isomorphism $f:V \to V'$ such that $q = f^* q'$. | Let $q$ and $q'$ be unimodular symmetric bilinear forms on underlying free $\mathbb{Z}$-modules $V$ and $V'$ respectively. The two forms $q$ and $q'$ are said equivalent if there is an isomorphism $f:V \to V'$ such that $q = f^* q'$. | ||
| Line 38: | Line 38: | ||
The form $q$ may have two different ''types''. It is of type ''even'' if $q(x,x)$ is an even number for any element $x$. Equivalently, if $q$ is written as a square matrix in a basis, it is even if the elements on the diagonal are all even. Otherwise, $q$ is said of type ''odd''. | The form $q$ may have two different ''types''. It is of type ''even'' if $q(x,x)$ is an even number for any element $x$. Equivalently, if $q$ is written as a square matrix in a basis, it is even if the elements on the diagonal are all even. Otherwise, $q$ is said of type ''odd''. | ||
| + | |||
| + | == Classification of indefinite forms == | ||
There is a simple classification result of indefinite forms: | There is a simple classification result of indefinite forms: | ||
Revision as of 14:16, 7 June 2010
1 Introduction
For a closed (topological or smooth) manifold  of dimension
of dimension 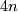 the intersection form
the intersection form

is obtained by the formula
![\displaystyle q_X(x,y) = \langle x \smile y , [X] \rangle ,](/images/math/f/5/7/f57e1e8038d92f22b87cb9c7943813d8.png)
i.e. the cup product of  and
and  is evaluated on the fundamental cycle given by the manifold
is evaluated on the fundamental cycle given by the manifold  .
.
 descends to a bilinear pairing on the free module
descends to a bilinear pairing on the free module 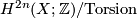 . It is a symmetric and unimodular (in particular non-degenerate) pairing, the latter follows from Poincaré duality.
. It is a symmetric and unimodular (in particular non-degenerate) pairing, the latter follows from Poincaré duality.
1 Algebraic invariants
Let  and
and 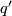 be unimodular symmetric bilinear forms on underlying free
be unimodular symmetric bilinear forms on underlying free 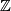 -modules
-modules  and
and 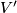 respectively. The two forms
respectively. The two forms  and
and 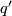 are said equivalent if there is an isomorphism
are said equivalent if there is an isomorphism 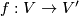 such that
such that  .
.
A form  is called definite if it is positive or negative definite, otherwise it is called indefinite.
The rank of q is the rank of the underlying
is called definite if it is positive or negative definite, otherwise it is called indefinite.
The rank of q is the rank of the underlying 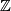 -module V.
-module V.
As  is symmetric it is diagonalisable over the real numbers. If
is symmetric it is diagonalisable over the real numbers. If 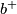 denotes the dimension of a maximal subspace on which the form is positive definite, and if
denotes the dimension of a maximal subspace on which the form is positive definite, and if 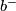 is the dimension of a maximal subspace on which the form is negative definite, then the signature of
is the dimension of a maximal subspace on which the form is negative definite, then the signature of  is defined to be
is defined to be
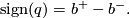
The form  may have two different types. It is of type even if
may have two different types. It is of type even if 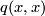 is an even number for any element
is an even number for any element  . Equivalently, if
. Equivalently, if  is written as a square matrix in a basis, it is even if the elements on the diagonal are all even. Otherwise,
is written as a square matrix in a basis, it is even if the elements on the diagonal are all even. Otherwise,  is said of type odd.
is said of type odd.
2 Classification of indefinite forms
There is a simple classification result of indefinite forms:
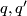 over
over 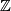 are equivalent if and only if
are equivalent if and only if  and
and 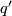 have the same rank, signature and type.
have the same rank, signature and type.
2 References
|
This page has not been refereed. The information given here might be incomplete or provisional. |