Inertia group II (Ex)
From Manifold Atlas
(Difference between revisions)
(Created page with "<wikitex>; Let $M$ be a closed smooth oriented $n$-manifold. The ''homotopy inertia group of $M$'', $I_H(M), is the subgroup of homotopy $n$-spheres $\Theta_n$ defined by $$...") |
|||
| (4 intermediate revisions by one user not shown) | |||
| Line 1: | Line 1: | ||
<wikitex>; | <wikitex>; | ||
| − | Let $M$ be a closed smooth oriented $n$-manifold. The ''homotopy inertia group of $M$'', $I_H(M), | + | Let $M$ be a closed smooth oriented $n$-manifold. The ''homotopy inertia group of $M$'', $I_H(M)$, |
is the subgroup of homotopy $n$-spheres $\Theta_n$ defined by | is the subgroup of homotopy $n$-spheres $\Theta_n$ defined by | ||
$$ I_H(M) : = \{ \Sigma | \exists f \colon \Sigma \sharp M \cong M, f \simeq {\rm Id}_M \},$$ | $$ I_H(M) : = \{ \Sigma | \exists f \colon \Sigma \sharp M \cong M, f \simeq {\rm Id}_M \},$$ | ||
| − | where for the statement $f \simeq {\rm Id}_M$ we regard $M$ and $\Sigma \sharp M$ as the same | + | where for the statement $f \simeq {\rm Id}_M$ we regard $M$ and $\Sigma \sharp M$ as the same topological space (with different smooth structures). |
| − | topological space (with different smooth structures). | + | |
{{beginthm|Exercise}} | {{beginthm|Exercise}} | ||
| − | # Show that $\Sigma \in I_H(M)$ if and only if ${\rm Id}_M \colon \Sigma \sharp M \to M$ is equivalent | + | # Show that $\Sigma \in I_H(M)$ if and only if ${\rm Id}_M \colon \Sigma \sharp M \to M$ is equivalent to ${\rm Id}_M \colon M \to M$ in $\mathcal{S}(M)$. |
| − | to ${\rm Id}_M \colon M \to M$ in $\mathcal{S}(M)$. | + | # Given that $\Theta_8 \cong \textup{Coker}(J_8) \cong \Zz/2$, determine $I_H(\Hh P^2)$. |
| − | # | + | # Assuming that $I(\Hh P^2) = \Theta_8$, deduce that $\Hh P^2$ admits a self-homotopy equivalence which is not homotopic to a diffeomorphism. |
{{endthm}} | {{endthm}} | ||
</wikitex> | </wikitex> | ||
| Line 14: | Line 13: | ||
{{#RefList:}} | {{#RefList:}} | ||
[[Category:Exercises]] | [[Category:Exercises]] | ||
| − | [[Category:Exercises | + | [[Category:Exercises with solution]] |
Latest revision as of 18:35, 29 August 2013
Let  be a closed smooth oriented
be a closed smooth oriented  -manifold. The homotopy inertia group of
-manifold. The homotopy inertia group of  ,
, 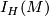 ,
is the subgroup of homotopy
,
is the subgroup of homotopy  -spheres
-spheres  defined by
defined by
Tex syntax error
Tex syntax errorwe regard
 and
and  as the same topological space (with different smooth structures).
as the same topological space (with different smooth structures).
Exercise 0.1.
- Show that
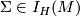 if and only if
if and only if Tex syntax error
is equivalent toTex syntax error
in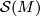 .
.
- Given that
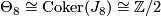 , determine
, determine 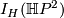 .
.
- Assuming that
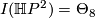 , deduce that
, deduce that  admits a self-homotopy equivalence which is not homotopic to a diffeomorphism.
admits a self-homotopy equivalence which is not homotopic to a diffeomorphism.