Hirzebruch surfaces
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 09:48, 1 April 2011 and the changes since publication. |
Contents |
1 Introduction
Hirzebruch surfaces were introduced by Hirzebruch (without that name) in his thesis [Hirzebruch1953a]. They are algebraic surfaces over the complex numbers. Here we consider them as smooth manifolds.
2 Construction and examples
Let ![L:= \{[x],y)\in \mathbb {CP}^1 \times \mathbb C^2 |y = \lambda x](/images/math/8/8/d/88df819a4f4cb39ea67b3a4edf99397a.png) for some
for some 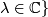 be the tautological line bundle over
be the tautological line bundle over 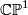 . For
. For 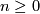 define
define  as the total space of the projective line bundle associated to
as the total space of the projective line bundle associated to 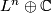 , where
, where  is the tensor product of
is the tensor product of  copies of
copies of  with itself. For
with itself. For 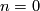 the bundle
the bundle 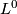 is by definition the trivial bundle. For
is by definition the trivial bundle. For  we define
we define  as the corresponding construction with
as the corresponding construction with  , the complex conjugated bundle, instead of
, the complex conjugated bundle, instead of  . These are the
. These are the 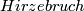
 . They come with a complex structure but we consider them as smooth manifolds.
. They come with a complex structure but we consider them as smooth manifolds.
3 Invariants
The dimension as a smooth manifold is  .
.
Since the Hirzebruch surfaces are - as real manifold -  -bundles over
-bundles over  they are closed and - by the orientation coming from the complex structure - oriented
they are closed and - by the orientation coming from the complex structure - oriented  -dimensional manifolds and the homotopy sequence of a fibration implies that they are simply connected.
-dimensional manifolds and the homotopy sequence of a fibration implies that they are simply connected.
The signature of  is zero since the Hirzebruch surfaces are the boundary of the associated
is zero since the Hirzebruch surfaces are the boundary of the associated 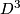 -bundle.
-bundle.
The homology is trivial except in degree  and
and  , where it is
, where it is 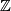 and in degree
and in degree  where it is isomorphic to
where it is isomorphic to 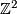 with basis given by the homology class represented by a fibre and the homology class of the base considered as homology class of
with basis given by the homology class represented by a fibre and the homology class of the base considered as homology class of  using the section of points at infinity.
using the section of points at infinity.
The intersection form on 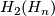 is with respect to this basis given by the matrix
is with respect to this basis given by the matrix
0 1
1 -n
The Euler characteristic is  .
.
4 Classification
The intersection form implies that if  is diffeomorphic to
is diffeomorphic to 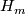 , then
, then  mod
mod  . On the other hand they are - as smooth manifolds - total spaces of the sphere bundle of a
. On the other hand they are - as smooth manifolds - total spaces of the sphere bundle of a  -dimensional vector bundle over
-dimensional vector bundle over  . these bundles are classified by
. these bundles are classified by 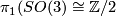 . Thus there are at most two diffeomorphism types of the fibre and so we conclude:
. Thus there are at most two diffeomorphism types of the fibre and so we conclude:
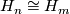
if and only if  mod
mod  , where
, where 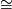 means diffeomorphic. By construction
means diffeomorphic. By construction 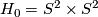 and by an easy consideration
and by an easy consideration  #
# 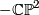 .
.
For more information on Hirzebruch surfaces see [Hirzebruch1953a].
5 Further remarks
The Hirzebruch surfaces are the first stage of the so called Bott tower, which is inductively constructed staring from a point as the total space of a projective bundle associated to  , where
, where  is a line bundle over a lower Bott tower. The classification of the Bott manifolds up to homeomorphism or diffeomorphism is an interesting open problem. In particular one can ask wether the integral cohomology ring determines the homeomorphism or diffeomorphism type.
is a line bundle over a lower Bott tower. The classification of the Bott manifolds up to homeomorphism or diffeomorphism is an interesting open problem. In particular one can ask wether the integral cohomology ring determines the homeomorphism or diffeomorphism type.
6 References
- [[Template: |[ ]]] {{ }}
Please modify these headings or choose other headings according to your needs.MediaWiki:Stub
$ and $, where it is $\mathbb Z$ and in degree $ where it is isomorphic to $\mathbb Z^2$ with basis given by the homology class represented by a fibre and the homology class of the base considered as homology class of $H_n$ using the section of points at infinity. The intersection form on $H_2(H_n)$ is with respect to this basis given by the matrix 0 1 1 -n The Euler characteristic is $e(H_n) =4$. == Classification ==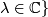 be the tautological line bundle over
be the tautological line bundle over 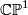 . For
. For 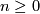 define
define  as the total space of the projective line bundle associated to
as the total space of the projective line bundle associated to 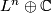 , where
, where  is the tensor product of
is the tensor product of  copies of
copies of  with itself. For
with itself. For 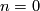 the bundle
the bundle 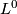 is by definition the trivial bundle. For
is by definition the trivial bundle. For  we define
we define  as the corresponding construction with
as the corresponding construction with  , the complex conjugated bundle, instead of
, the complex conjugated bundle, instead of  . These are the
. These are the 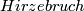
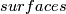 . They come with a complex structure but we consider them as smooth manifolds.
. They come with a complex structure but we consider them as smooth manifolds.
3 Invariants
The dimension as a smooth manifold is  .
.
Since the Hirzebruch surfaces are - as real manifold -  -bundles over
-bundles over  they are closed and - by the orientation coming from the complex structure - oriented
they are closed and - by the orientation coming from the complex structure - oriented  -dimensional manifolds and the homotopy sequence of a fibration implies that they are simply connected.
-dimensional manifolds and the homotopy sequence of a fibration implies that they are simply connected.
The signature of  is zero since the Hirzebruch surfaces are the boundary of the associated
is zero since the Hirzebruch surfaces are the boundary of the associated 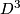 -bundle.
-bundle.
The homology is trivial except in degree  and
and  , where it is
, where it is 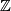 and in degree
and in degree  where it is isomorphic to
where it is isomorphic to 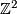 with basis given by the homology class represented by a fibre and the homology class of the base considered as homology class of
with basis given by the homology class represented by a fibre and the homology class of the base considered as homology class of  using the section of points at infinity.
using the section of points at infinity.
The intersection form on 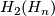 is with respect to this basis given by the matrix
is with respect to this basis given by the matrix
0 1
1 -n
The Euler characteristic is  .
.
4 Classification
The intersection form implies that if  is diffeomorphic to
is diffeomorphic to 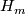 , then
, then  mod
mod  . On the other hand they are - as smooth manifolds - total spaces of the sphere bundle of a
. On the other hand they are - as smooth manifolds - total spaces of the sphere bundle of a  -dimensional vector bundle over
-dimensional vector bundle over  . these bundles are classified by
. these bundles are classified by 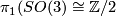 . Thus there are at most two diffeomorphism types of the fibre and so we conclude:
. Thus there are at most two diffeomorphism types of the fibre and so we conclude:
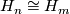
if and only if  mod
mod  , where
, where 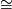 means diffeomorphic. By construction
means diffeomorphic. By construction 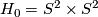 and by an easy consideration
and by an easy consideration  #
# 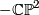 .
.
For more information on Hirzebruch surfaces see [Hirzebruch1953a].
5 Further remarks
The Hirzebruch surfaces are the first stage of the so called Bott tower, which is inductively constructed staring from a point as the total space of a projective bundle associated to  , where
, where  is a line bundle over a lower Bott tower. The classification of the Bott manifolds up to homeomorphism or diffeomorphism is an interesting open problem. In particular one can ask wether the integral cohomology ring determines the homeomorphism or diffeomorphism type.
is a line bundle over a lower Bott tower. The classification of the Bott manifolds up to homeomorphism or diffeomorphism is an interesting open problem. In particular one can ask wether the integral cohomology ring determines the homeomorphism or diffeomorphism type.
6 References
- [[Template: |[ ]]] {{ }}
Please modify these headings or choose other headings according to your needs.MediaWiki:Stub