Fake lens spaces
m (→Introduction) |
(→Invariants) |
||
| Line 25: | Line 25: | ||
== Invariants == | == Invariants == | ||
| − | <wikitex>; ... </wikitex> | + | <wikitex>; |
| + | |||
| + | * $\pi_1 (L) = \Zz_m$, $\pi_i (L) = \pi_i (S^{2d-1})$ for $i \geq 2$ | ||
| + | |||
| + | * $H_0 (L) = \Zz$, $H_{2d-1} (L) = \Zz$, $H_{2i-1} (L) = \Zz_m$ for $1 \leq i \leq d-1$, $H_i (L) = 0$ for all other values of $i$. | ||
| + | |||
| + | * $\Delta$, $\rho$, ... | ||
| + | |||
| + | </wikitex> | ||
== Homotopy Classification == | == Homotopy Classification == | ||
Revision as of 14:52, 7 June 2010
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 15:18, 25 April 2013 and the changes since publication. |
Contents |
1 Introduction
A fake lens space is the orbit space of a free action of a finite cyclic group  on a sphere
on a sphere  . It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
. It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
2 Construction and examples
- ...
3 Invariants
-
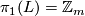 ,
, 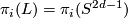 for
for 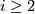
-
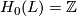 ,
, 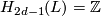 ,
, 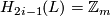 for
for 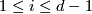 ,
, 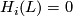 for all other values of
for all other values of  .
.
-
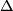 ,
,  , ...
, ...
4 Homotopy Classification
- We cite mainly from
[Wall(1999), chapter 14E].
We start by introducing some notation for {\it lens spaces} which
are a special sort of fake lens spaces. LetTex syntax error,
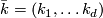 , where
, where Tex syntax errorare such that
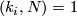 .
When
.
When Tex syntax errordefine a representation
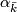 of
of  on
on
Tex syntax errorby
 . Any free representation of
. Any free representation of  on a
on a
 -dimensional complex vector space is isomorphic to some
-dimensional complex vector space is isomorphic to some
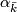 . The representation
. The representation 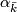 induces a
free action of
induces a
free action of  on
on  which we still denote
which we still denote 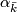 .
.
\begin{defn}
A {\it lens space} 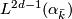 is a manifold
obtained as the orbit pace of a free action
is a manifold
obtained as the orbit pace of a free action 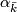 of the
of the
Tex syntax erroron
 for some
for some  as above.\footnote{In the notation of [Wall(1999), chapter
as above.\footnote{In the notation of [Wall(1999), chapter
14E] we have 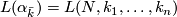 .}
\end{defn}
.}
\end{defn}
The lens space 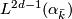 is a
is a 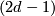 -dimensional
-dimensional
Tex syntax error. Its
universal cover is  , hence
, hence 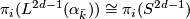 for
for 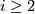 . There exists a
convenient choice of a CW-structure for
. There exists a
convenient choice of a CW-structure for 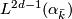 with one cell
with one cell  in each dimension
in each dimension 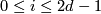 .
.
Tex syntax errorwhen
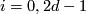 ,
, Tex syntax errorwhen
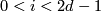 is odd and
is odd and 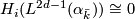
when 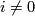 is even.
is even.
The classification of the lens spaces up to homotopy equivalence and simple homotopy equivalence is presented for example in [Milnor(1966)]. The simple homotopy classification is stated in
terms of Reidemeister torsion which is a unit inTex syntax error. This ring is defined as
Tex syntax errorwith
Tex syntax errorwhere
Tex syntax errorbe the group ring of
 and
and
 is the ideal generated by the norm element
is the ideal generated by the norm element 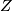
 . We also suppose that a generator
. We also suppose that a generator Tex syntax errorof
 is chosen. There
is also an augmentation map
is chosen. There
is also an augmentation map Tex syntax error
[Wall(1999), page 214]. The homotopy classification is stated
in terms of a certain unit inTex syntax error. These invariants also suffice
for the homotopy and simple homotopy classification of finite
CW-complexes with
with Tex syntax errorand with the universal
cover homotopy equivalent to  of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex
of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex  as above is a pair
as above is a pair 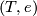
Tex syntax erroris a choice of a generator of
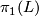 and
and  is a
choice of a homotopy equivalence
is a
choice of a homotopy equivalence Tex syntax error.
\end{defn}
\noindent Denote further by 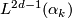 the lens space
the lens space
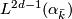 with
with 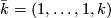 . By
. By
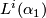 is denoted the
is denoted the  -skeleton of the lens space
-skeleton of the lens space
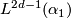 . If
. If  is odd this is a lens space, if
is odd this is a lens space, if  is
even this is a CW-complex obtained by attaching an
is
even this is a CW-complex obtained by attaching an  -cell to the
lens space of dimension
-cell to the
lens space of dimension 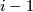 .
\begin{prop}Wall(1999)
Let
.
\begin{prop}Wall(1999)
Let  be a finite CW-complex as
above polarized by
be a finite CW-complex as
above polarized by 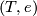 . Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of
. Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 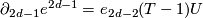 for some
for some
Tex syntax errorwhich maps to a unit
 . Furthermore, the
. Furthermore, the
complex  is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such
is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such  are in
are in
Tex syntax error. The correspondence is given by
Tex syntax error.
\item The polarized simple homotopy types of such  are in
one-to-one correspondence with the units in
are in
one-to-one correspondence with the units in 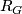 . The
correspondence is given by
. The
correspondence is given by  .
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such
.
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such  is addressed in [Wall(1999), Theorem 14E.4]. Since the
is addressed in [Wall(1999), Theorem 14E.4]. Since the
Tex syntax errorare exhausted by the lens spaces
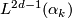 we obtain the following corollary.
\begin{cor}
we obtain the following corollary.
\begin{cor}
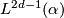 there exists
there exists Tex syntax error
and a homotopy equivalence \[ h \co L^{2d-1}(\alpha) \lra L^{2d-1}(\alpha_k). \] \end{cor}
5 Homeomorphism classification
- ...
6 Further discussion
- ...
7 References
|
This page has not been refereed. The information given here might be incomplete or provisional. |
 . It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
. It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
2 Construction and examples
- ...
3 Invariants
-
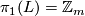 ,
, 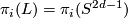 for
for 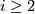
-
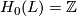 ,
, 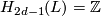 ,
, 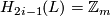 for
for 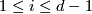 ,
, 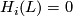 for all other values of
for all other values of  .
.
-
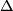 ,
,  , ...
, ...
4 Homotopy Classification
- We cite mainly from
[Wall(1999), chapter 14E].
We start by introducing some notation for {\it lens spaces} which
are a special sort of fake lens spaces. LetTex syntax error,
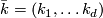 , where
, where Tex syntax errorare such that
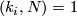 .
When
.
When Tex syntax errordefine a representation
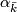 of
of  on
on
Tex syntax errorby
 . Any free representation of
. Any free representation of  on a
on a
 -dimensional complex vector space is isomorphic to some
-dimensional complex vector space is isomorphic to some
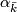 . The representation
. The representation 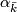 induces a
free action of
induces a
free action of  on
on  which we still denote
which we still denote 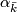 .
.
\begin{defn}
A {\it lens space} 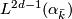 is a manifold
obtained as the orbit pace of a free action
is a manifold
obtained as the orbit pace of a free action 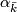 of the
of the
Tex syntax erroron
 for some
for some  as above.\footnote{In the notation of [Wall(1999), chapter
as above.\footnote{In the notation of [Wall(1999), chapter
14E] we have 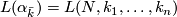 .}
\end{defn}
.}
\end{defn}
The lens space 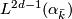 is a
is a 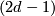 -dimensional
-dimensional
Tex syntax error. Its
universal cover is  , hence
, hence 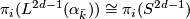 for
for 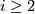 . There exists a
convenient choice of a CW-structure for
. There exists a
convenient choice of a CW-structure for 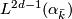 with one cell
with one cell  in each dimension
in each dimension 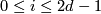 .
.
Tex syntax errorwhen
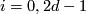 ,
, Tex syntax errorwhen
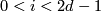 is odd and
is odd and 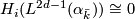
when 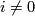 is even.
is even.
The classification of the lens spaces up to homotopy equivalence and simple homotopy equivalence is presented for example in [Milnor(1966)]. The simple homotopy classification is stated in
terms of Reidemeister torsion which is a unit inTex syntax error. This ring is defined as
Tex syntax errorwith
Tex syntax errorwhere
Tex syntax errorbe the group ring of
 and
and
 is the ideal generated by the norm element
is the ideal generated by the norm element 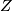
 . We also suppose that a generator
. We also suppose that a generator Tex syntax errorof
 is chosen. There
is also an augmentation map
is chosen. There
is also an augmentation map Tex syntax error
[Wall(1999), page 214]. The homotopy classification is stated
in terms of a certain unit inTex syntax error. These invariants also suffice
for the homotopy and simple homotopy classification of finite
CW-complexes with
with Tex syntax errorand with the universal
cover homotopy equivalent to  of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex
of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex  as above is a pair
as above is a pair 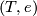
Tex syntax erroris a choice of a generator of
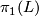 and
and  is a
choice of a homotopy equivalence
is a
choice of a homotopy equivalence Tex syntax error.
\end{defn}
\noindent Denote further by 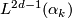 the lens space
the lens space
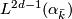 with
with 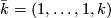 . By
. By
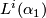 is denoted the
is denoted the  -skeleton of the lens space
-skeleton of the lens space
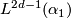 . If
. If  is odd this is a lens space, if
is odd this is a lens space, if  is
even this is a CW-complex obtained by attaching an
is
even this is a CW-complex obtained by attaching an  -cell to the
lens space of dimension
-cell to the
lens space of dimension 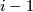 .
\begin{prop}Wall(1999)
Let
.
\begin{prop}Wall(1999)
Let  be a finite CW-complex as
above polarized by
be a finite CW-complex as
above polarized by 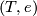 . Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of
. Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 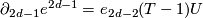 for some
for some
Tex syntax errorwhich maps to a unit
 . Furthermore, the
. Furthermore, the
complex  is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such
is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such  are in
are in
Tex syntax error. The correspondence is given by
Tex syntax error.
\item The polarized simple homotopy types of such  are in
one-to-one correspondence with the units in
are in
one-to-one correspondence with the units in 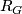 . The
correspondence is given by
. The
correspondence is given by  .
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such
.
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such  is addressed in [Wall(1999), Theorem 14E.4]. Since the
is addressed in [Wall(1999), Theorem 14E.4]. Since the
Tex syntax errorare exhausted by the lens spaces
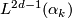 we obtain the following corollary.
\begin{cor}
we obtain the following corollary.
\begin{cor}
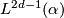 there exists
there exists Tex syntax error
and a homotopy equivalence \[ h \co L^{2d-1}(\alpha) \lra L^{2d-1}(\alpha_k). \] \end{cor}
5 Homeomorphism classification
- ...
6 Further discussion
- ...
7 References
|
This page has not been refereed. The information given here might be incomplete or provisional. |
 . It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
. It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
2 Construction and examples
- ...
3 Invariants
-
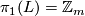 ,
, 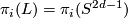 for
for 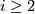
-
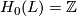 ,
, 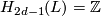 ,
, 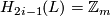 for
for 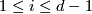 ,
, 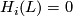 for all other values of
for all other values of  .
.
-
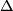 ,
,  , ...
, ...
4 Homotopy Classification
- We cite mainly from
[Wall(1999), chapter 14E].
We start by introducing some notation for {\it lens spaces} which
are a special sort of fake lens spaces. LetTex syntax error,
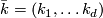 , where
, where Tex syntax errorare such that
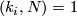 .
When
.
When Tex syntax errordefine a representation
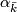 of
of  on
on
Tex syntax errorby
 . Any free representation of
. Any free representation of  on a
on a
 -dimensional complex vector space is isomorphic to some
-dimensional complex vector space is isomorphic to some
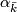 . The representation
. The representation 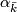 induces a
free action of
induces a
free action of  on
on  which we still denote
which we still denote 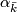 .
.
\begin{defn}
A {\it lens space} 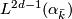 is a manifold
obtained as the orbit pace of a free action
is a manifold
obtained as the orbit pace of a free action 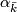 of the
of the
Tex syntax erroron
 for some
for some  as above.\footnote{In the notation of [Wall(1999), chapter
as above.\footnote{In the notation of [Wall(1999), chapter
14E] we have 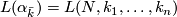 .}
\end{defn}
.}
\end{defn}
The lens space 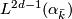 is a
is a 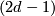 -dimensional
-dimensional
Tex syntax error. Its
universal cover is  , hence
, hence 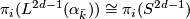 for
for 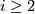 . There exists a
convenient choice of a CW-structure for
. There exists a
convenient choice of a CW-structure for 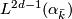 with one cell
with one cell  in each dimension
in each dimension 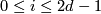 .
.
Tex syntax errorwhen
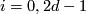 ,
, Tex syntax errorwhen
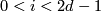 is odd and
is odd and 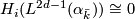
when 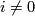 is even.
is even.
The classification of the lens spaces up to homotopy equivalence and simple homotopy equivalence is presented for example in [Milnor(1966)]. The simple homotopy classification is stated in
terms of Reidemeister torsion which is a unit inTex syntax error. This ring is defined as
Tex syntax errorwith
Tex syntax errorwhere
Tex syntax errorbe the group ring of
 and
and
 is the ideal generated by the norm element
is the ideal generated by the norm element 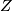
 . We also suppose that a generator
. We also suppose that a generator Tex syntax errorof
 is chosen. There
is also an augmentation map
is chosen. There
is also an augmentation map Tex syntax error
[Wall(1999), page 214]. The homotopy classification is stated
in terms of a certain unit inTex syntax error. These invariants also suffice
for the homotopy and simple homotopy classification of finite
CW-complexes with
with Tex syntax errorand with the universal
cover homotopy equivalent to  of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex
of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex  as above is a pair
as above is a pair 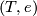
Tex syntax erroris a choice of a generator of
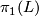 and
and  is a
choice of a homotopy equivalence
is a
choice of a homotopy equivalence Tex syntax error.
\end{defn}
\noindent Denote further by 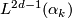 the lens space
the lens space
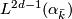 with
with 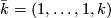 . By
. By
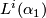 is denoted the
is denoted the  -skeleton of the lens space
-skeleton of the lens space
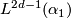 . If
. If  is odd this is a lens space, if
is odd this is a lens space, if  is
even this is a CW-complex obtained by attaching an
is
even this is a CW-complex obtained by attaching an  -cell to the
lens space of dimension
-cell to the
lens space of dimension 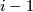 .
\begin{prop}Wall(1999)
Let
.
\begin{prop}Wall(1999)
Let  be a finite CW-complex as
above polarized by
be a finite CW-complex as
above polarized by 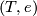 . Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of
. Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 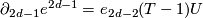 for some
for some
Tex syntax errorwhich maps to a unit
 . Furthermore, the
. Furthermore, the
complex  is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such
is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such  are in
are in
Tex syntax error. The correspondence is given by
Tex syntax error.
\item The polarized simple homotopy types of such  are in
one-to-one correspondence with the units in
are in
one-to-one correspondence with the units in 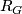 . The
correspondence is given by
. The
correspondence is given by  .
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such
.
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such  is addressed in [Wall(1999), Theorem 14E.4]. Since the
is addressed in [Wall(1999), Theorem 14E.4]. Since the
Tex syntax errorare exhausted by the lens spaces
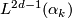 we obtain the following corollary.
\begin{cor}
we obtain the following corollary.
\begin{cor}
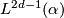 there exists
there exists Tex syntax error
and a homotopy equivalence \[ h \co L^{2d-1}(\alpha) \lra L^{2d-1}(\alpha_k). \] \end{cor}
5 Homeomorphism classification
- ...
6 Further discussion
- ...
7 References
|
This page has not been refereed. The information given here might be incomplete or provisional. |
 . It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
. It is a generalization of the notion of a lens space which is
the orbit space of a free action which comes from a unitary representation.
2 Construction and examples
- ...
3 Invariants
-
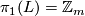 ,
, 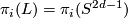 for
for 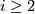
-
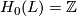 ,
, 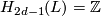 ,
, 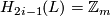 for
for 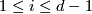 ,
, 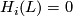 for all other values of
for all other values of  .
.
-
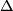 ,
,  , ...
, ...
4 Homotopy Classification
- We cite mainly from
[Wall(1999), chapter 14E].
We start by introducing some notation for {\it lens spaces} which
are a special sort of fake lens spaces. LetTex syntax error,
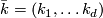 , where
, where Tex syntax errorare such that
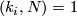 .
When
.
When Tex syntax errordefine a representation
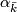 of
of  on
on
Tex syntax errorby
 . Any free representation of
. Any free representation of  on a
on a
 -dimensional complex vector space is isomorphic to some
-dimensional complex vector space is isomorphic to some
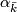 . The representation
. The representation 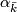 induces a
free action of
induces a
free action of  on
on  which we still denote
which we still denote 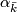 .
.
\begin{defn}
A {\it lens space} 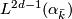 is a manifold
obtained as the orbit pace of a free action
is a manifold
obtained as the orbit pace of a free action 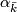 of the
of the
Tex syntax erroron
 for some
for some  as above.\footnote{In the notation of [Wall(1999), chapter
as above.\footnote{In the notation of [Wall(1999), chapter
14E] we have 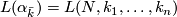 .}
\end{defn}
.}
\end{defn}
The lens space 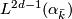 is a
is a 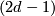 -dimensional
-dimensional
Tex syntax error. Its
universal cover is  , hence
, hence 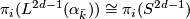 for
for 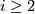 . There exists a
convenient choice of a CW-structure for
. There exists a
convenient choice of a CW-structure for 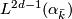 with one cell
with one cell  in each dimension
in each dimension 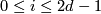 .
.
Tex syntax errorwhen
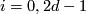 ,
, Tex syntax errorwhen
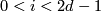 is odd and
is odd and 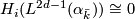
when 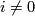 is even.
is even.
The classification of the lens spaces up to homotopy equivalence and simple homotopy equivalence is presented for example in [Milnor(1966)]. The simple homotopy classification is stated in
terms of Reidemeister torsion which is a unit inTex syntax error. This ring is defined as
Tex syntax errorwith
Tex syntax errorwhere
Tex syntax errorbe the group ring of
 and
and
 is the ideal generated by the norm element
is the ideal generated by the norm element 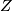
 . We also suppose that a generator
. We also suppose that a generator Tex syntax errorof
 is chosen. There
is also an augmentation map
is chosen. There
is also an augmentation map Tex syntax error
[Wall(1999), page 214]. The homotopy classification is stated
in terms of a certain unit inTex syntax error. These invariants also suffice
for the homotopy and simple homotopy classification of finite
CW-complexes with
with Tex syntax errorand with the universal
cover homotopy equivalent to  of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex
of which fake lens spaces
are obviously a special case. It is convenient to make the following
definition.
\begin{defn}
A {\it polarization} of a CW-complex  as above is a pair
as above is a pair 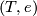
Tex syntax erroris a choice of a generator of
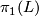 and
and  is a
choice of a homotopy equivalence
is a
choice of a homotopy equivalence Tex syntax error.
\end{defn}
\noindent Denote further by 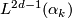 the lens space
the lens space
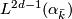 with
with 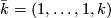 . By
. By
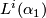 is denoted the
is denoted the  -skeleton of the lens space
-skeleton of the lens space
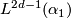 . If
. If  is odd this is a lens space, if
is odd this is a lens space, if  is
even this is a CW-complex obtained by attaching an
is
even this is a CW-complex obtained by attaching an  -cell to the
lens space of dimension
-cell to the
lens space of dimension 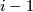 .
\begin{prop}Wall(1999)
Let
.
\begin{prop}Wall(1999)
Let  be a finite CW-complex as
above polarized by
be a finite CW-complex as
above polarized by 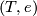 . Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of
. Then there exists a simple homotopy
equivalence
\[
h \co L \lra L^{2d-2}(\alpha_1) \cup_\phi e^{2d-1}
\]
preserving the polarization. It is unique up to homotopy and the
action of  . The chain complex differential on the right hand side
is given by
. The chain complex differential on the right hand side
is given by 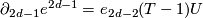 for some
for some
Tex syntax errorwhich maps to a unit
 . Furthermore, the
. Furthermore, the
complex  is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such
is a Poincar\'e complex.
\begin{enumerate}
\item The polarized homotopy types of such  are in
are in
Tex syntax error. The correspondence is given by
Tex syntax error.
\item The polarized simple homotopy types of such  are in
one-to-one correspondence with the units in
are in
one-to-one correspondence with the units in 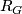 . The
correspondence is given by
. The
correspondence is given by  .
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such
.
\end{enumerate}
\end{prop}
\noindent The existence of a fake lens space in the homotopy type of
such  is addressed in [Wall(1999), Theorem 14E.4]. Since the
is addressed in [Wall(1999), Theorem 14E.4]. Since the
Tex syntax errorare exhausted by the lens spaces
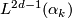 we obtain the following corollary.
\begin{cor}
we obtain the following corollary.
\begin{cor}
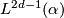 there exists
there exists Tex syntax error
and a homotopy equivalence \[ h \co L^{2d-1}(\alpha) \lra L^{2d-1}(\alpha_k). \] \end{cor}
5 Homeomorphism classification
- ...
6 Further discussion
- ...
7 References
|
This page has not been refereed. The information given here might be incomplete or provisional. |