Equivariant intersection number of π-trivial immersions
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
This is a work in progress! Initial blurb.
Let 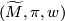 be an oriented cover of a connected manifold
be an oriented cover of a connected manifold 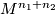 and let
and let 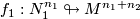 ,
, 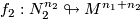 be
be  -trivial immersions of manifolds in
-trivial immersions of manifolds in  . The equivariant intersection number
. The equivariant intersection number ![\lambda([N_1],[N_2])\in\Z[\pi]](/images/math/b/c/d/bcdfc6dc4b56e4b2b7b24ca843038bd8.png) counts with elements of
counts with elements of ![\Z[\pi]](/images/math/1/e/c/1ecba37f7392a1697efda3ee93c740e2.png) the number of intersection points that the two immersions have. The intersection number is an obstruction to perturbing the immersions into being disjoint, which when zero can often be achieved using the Whitney trick.
the number of intersection points that the two immersions have. The intersection number is an obstruction to perturbing the immersions into being disjoint, which when zero can often be achieved using the Whitney trick.
The intersection number is also used in defining the intersection form of a  -dimensional manifold and in turn its signature - a very important invariant used in the classification of manifolds and the primary surgery obstruction.
-dimensional manifold and in turn its signature - a very important invariant used in the classification of manifolds and the primary surgery obstruction.
2 Definition
 with respect to an oriented cover
with respect to an oriented cover 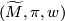
![\displaystyle \begin{array}{rcl}\lambda: H_n(\widetilde{M})\times H_{m-n}(\widetilde{M}) &\to& \Z[\pi]\\ (a,b) &\mapsto& \lambda(a,b)\end{array}](/images/math/0/b/4/0b4b53e55cd8acf4980d2c5535ca1469.png)
![\displaystyle \lambda(a,b) = a^*(b)\in \Z[\pi]](/images/math/b/0/f/b0fcae216fdc9e5f0f4c0c3575d15bcd.png)
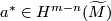 the Poincar\'{e} dual of
the Poincar\'{e} dual of  with respect to Universal Poincar\'{e} duality, such that
with respect to Universal Poincar\'{e} duality, such that ![\displaystyle \lambda(b,a) = (-1)^{n(m-n)}\overline{\lambda(a,b)}\in \Z[\pi].](/images/math/7/a/9/7a959480e00e19774331363df44ca591.png)
 -trivial maps
-trivial maps 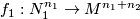 ,
, 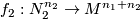 with prescribed lifts
with prescribed lifts 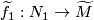 ,
, 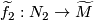 is the homology intersection of the homology classes
is the homology intersection of the homology classes ![(\widetilde{f}_1)_*[N_1]\in H_{n_1}(\widetilde{M})](/images/math/c/4/6/c46e32560c8615e67d873ca8c7ff648d.png) ,
, ![(\widetilde{f}_2)_*[N_2]\in H_{n_2}(\widetilde{M})](/images/math/1/f/e/1febc73b71c95f1718e9acbbff3df2c1.png) :
: ![\displaystyle \lambda^{\mathrm{alg}}(N_1,N_2) = \lambda((\widetilde{f}_1)_*[N_1],(\widetilde{f}_2)_*[N_2])\in \Z[\pi].](/images/math/0/a/5/0a5d108cbbc13596efbbc744b332d2cd.png)
3 Alternative Description
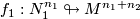 ,
, 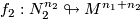 be
be  -trivial immersions with prescribed lifts to the oriented cover
-trivial immersions with prescribed lifts to the oriented cover 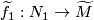 ,
,  . At a transverse double point
. At a transverse double point 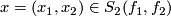 let
let 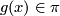 be the unique covering translation
be the unique covering translation 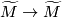 such that
such that 
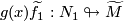 ,
, 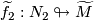 have a transverse double point
have a transverse double point 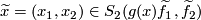
 -dimensional vector spaces
-dimensional vector spaces 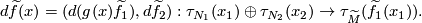
![I(x)\in\Z[\pi]](/images/math/5/f/f/5ffce5e793f9e8c62ad8efee6f130718.png) of a transverse double point
of a transverse double point 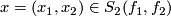 is
is ![\displaystyle I(x) = w(x)g(x)\in{\pm\pi}\subset \Z[\pi]](/images/math/1/a/c/1acb377eeb39fd20abd919a8e9be5b55.png)
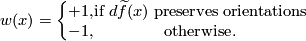
Instead of thinking in terms of lifts the equivariant index can also be defined in terms of paths. Let  be a basepoint of
be a basepoint of  ,
, 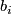 a basepoint of
a basepoint of  for
for 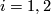 and let
and let 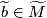 be some choice of lift. For a transverse double point
be some choice of lift. For a transverse double point 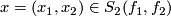 , an isotopy class of paths from
, an isotopy class of paths from 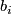 to
to 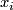 corresponds to a lift
corresponds to a lift 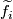 as follows.
as follows.
![\displaystyle I(x_2,x_1) = (-1)^{n_1n_2}\overline{I(x_1,x_2)}\in\Z[\pi]](/images/math/a/e/d/aede0071954c04f02bad98282381d414.png)
![\Z[\pi]\to \Z[\pi];a \mapsto \overline{a}](/images/math/a/8/7/a8741f19d2532c7dba3b6941e56c3b69.png) the
the  -twisted involution
-twisted involution 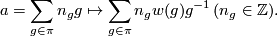
The geometric intersection number of transverse immersions 
 is
is
![\displaystyle \lambda^{\mathrm{geo}}(N_1,N_2) = \sum_{x\in S_2(f_1,f_2)}{I(x)}\in \Z[\pi].](/images/math/1/d/1/1d191be0a6f9a0c566a9030f40a3ea2a.png)
4 Equivalence of definitions
The algebraic and geometric intersection numbers agree. See REFERENCE
5 Examples
...