Curvature properties of exotic spheres
|
The user responsible for this page is Joachim. No other user may edit this page at present. |
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Introduction
This page is in the construction process!!!
A homotopy sphere of dimension  is an oriented closed smooth
manifold which is homotopy equivalent to the standard sphere
is an oriented closed smooth
manifold which is homotopy equivalent to the standard sphere  . A homotopy sphere is called an exotic sphere if it not diffeoemorphic to a standard sphere.
General information about homotopy spheres (and exotic spheres) is given on Exotic spheres. One prominent question concerning the geometry of exotic spheres is:
Given an exotic sphere, are there Riemannian metrics which fulfil specific positivity criteria? One typically considers the following three types.
. A homotopy sphere is called an exotic sphere if it not diffeoemorphic to a standard sphere.
General information about homotopy spheres (and exotic spheres) is given on Exotic spheres. One prominent question concerning the geometry of exotic spheres is:
Given an exotic sphere, are there Riemannian metrics which fulfil specific positivity criteria? One typically considers the following three types.
1 Homotopy spheres with positive sectional curvature
2 Homotopy spheres with positive Ricci curvature
3 Homotopy spheres with positive scalar curvature
Hitchin (based on work of Lichnerowicz) proved that the so-called {\it  -invariant} of a closed spin manifold provides an obstruction to the existence of a metric of positive scalar curvature on it (cf. [Hitchin1974], [Lichnerowicz1963]). The
-invariant} of a closed spin manifold provides an obstruction to the existence of a metric of positive scalar curvature on it (cf. [Hitchin1974], [Lichnerowicz1963]). The  -invariant for a closed
-invariant for a closed  -dimensional spin manifold (compare Spin bordism Invariants) is given as follows: Let
-dimensional spin manifold (compare Spin bordism Invariants) is given as follows: Let 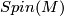 the principal
the principal 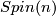 -bundle of
-bundle of  , and let
, and let  be choice of an irreducible
be choice of an irreducible 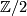 -graded module over the real Clifford algebra. The real spinors of
-graded module over the real Clifford algebra. The real spinors of  are defined to be ... to be continued.
are defined to be ... to be continued.
Proposition 7.1.
In case 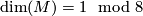 the space of harmonic spinors canonically has the structure of a complex vector space, while
in case
the space of harmonic spinors canonically has the structure of a complex vector space, while
in case  the space of harmonic spinors canonically carries the structure of a quarternionic vector space.
The
the space of harmonic spinors canonically carries the structure of a quarternionic vector space.
The  -invariant of a homotopy sphere
-invariant of a homotopy sphere  is given by
is given by
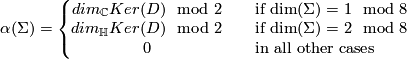
2 References
- [Hitchin1974] N. Hitchin, Harmonic spinors, Advances in Math. 14 (1974), 1–55. MR0358873 (50 #11332) Zbl 0284.58016
- [Lichnerowicz1963] A. Lichnerowicz, Spineurs harmoniques, C. R. Acad. Sci. Paris 257 (1963), 7–9. MR0156292 (27 #6218) Zbl 0714.53041