Connection on a principal bundle
(Created page with "{{Stub}} == Introduction == <wikitex include>; Let $G$ be a Lie group with Lie algebra $\frak{g}$ and $F \to M$ a principal bundle for $G$ over a smooth ...") |
(→Introduction) |
||
| Line 16: | Line 16: | ||
The $\frak{g}$-valued 2-form $\Omega := d\omega + [\omega,\omega]$ is called ''curvature form'' | The $\frak{g}$-valued 2-form $\Omega := d\omega + [\omega,\omega]$ is called ''curvature form'' | ||
| − | and measures the non-integrability of the distribution $\mathcal{H}$, see the page [[Connection]] | + | and measures the non-integrability of the distribution $\mathcal{H}$, see the theory page [[Connection]] |
for details. | for details. | ||
Revision as of 11:54, 15 March 2013
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Introduction
Let  be a Lie group with Lie algebra
be a Lie group with Lie algebra 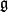 and
and
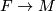 a principal bundle for
a principal bundle for  over a
smooth manifold
over a
smooth manifold  . A connection on
. A connection on  is a distribution
(a subbundle of the tangent bundle)
is a distribution
(a subbundle of the tangent bundle) 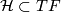 on
on  , called the "horizontal distribution", which is
, called the "horizontal distribution", which is  -invariant and
complementary to the vertical distribution
-invariant and
complementary to the vertical distribution 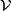 on
on  .
.
The decomposition 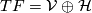 can be given by the projection
can be given by the projection
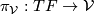 onto the vertical distribution. Since each vertical
space
onto the vertical distribution. Since each vertical
space 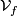 can be identified with
can be identified with 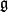 (see Principal bundle),
this map
(see Principal bundle),
this map 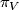 can be viewed as a
can be viewed as a 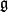 -valued 1-form on
-valued 1-form on  ,
a linear map
,
a linear map 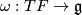 ; this is called the connection form.
; this is called the connection form.
The 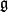 -valued 2-form
-valued 2-form ![\Omega := d\omega + [\omega,\omega]](/images/math/8/9/0/890f4329f822771ebae49340bdf3b095.png) is called curvature form
and measures the non-integrability of the distribution
is called curvature form
and measures the non-integrability of the distribution 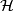 , see the theory page Connection
for details.
, see the theory page Connection
for details.
A connection 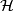 on a
on a  -principal bundles
-principal bundles  induces a distribution on any associated
bundle
induces a distribution on any associated
bundle 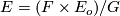 (see Principal bundle) since
(see Principal bundle) since 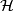 passes
trivially to
passes
trivially to 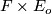 and by
and by  -invariance to
-invariance to  . The induced distribution is called a connection on
. The induced distribution is called a connection on  . If
. If 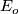 is a vector bundle (the action of
is a vector bundle (the action of  on
on 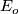 is linear), the connection
on
is linear), the connection
on  is closely related to a covariant derivative (see Connection).
is closely related to a covariant derivative (see Connection).