Bundle structures and lifting problems (Ex)
Given a (pointed) map  of pointed topological space, we define the homotopy fiber as\footnote{Here
of pointed topological space, we define the homotopy fiber as\footnote{Here
 is the space of paths starting at the base-point of
is the space of paths starting at the base-point of 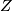 .}
.}
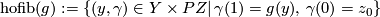
and we denote by  :
: 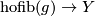 the projection.
the projection.
Show the following statement:\\A map
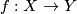 of pointed space has a lift
of pointed space has a lift Tex syntax erroralong

if and only if  is homotopic to the constant map.
is homotopic to the constant map.
Hint: This is a special case of Proposition 4.72 in [A. Hatcher, Algebraic Topology].
\subsection*{Classification of orientations and spin structures on vector bundles}
Recall the Definition of Eilenberg-MacLane-spaces.
We denote the space of pointed loops in a spaceby
.
\begin{enumerate}
\item Show: There is a homotopy equivalenceTex syntax error.\\
Hint: Use the uniqueness of Eilenberg-MacLane-spaces and the long exact sequence in homotopy
associated to the path-space-fibration
Tex syntax error
Recall that the path-space is contractible.
\item Show that the set of homotopy classes of pointed maps ![[Y,\Omega X]](/images/math/3/d/3/3d36331b6ffc21a47fc28e20effd0b46.png) has a group structure
induced by composition of paths.\\
Hint: This is similar to the group structure of the fundamental group.
has a group structure
induced by composition of paths.\\
Hint: This is similar to the group structure of the fundamental group.
\end{enumerate}
Tex syntax erroracts free and transitively on the set
of spin structures of an oriented vector bundle 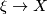 (X a compact pointed space).
(X a compact pointed space).
Tex syntax erroris due to the Exercise 3.2.}
Tex syntax error, where
Tex syntax errordenotes an Eilenberg-MacLane-space.
This looks similar to the statement of Theorem 3.45 in our talk.
So we first prove the statement about classification of spin structures. The warm-up is the classification of orientations: \begin{itemize}
\item The first Stiefel-Whitney class is a mapTex syntax error.
\item The homotopy fiber hofib() is
. \item The projection
is the map induced by
.
\end{itemize}
Assume that the homotopy groups of\item Calculate the homotopy groups ofare known. \begin{enumerate} \item Calculate the homotopy groups of
using the fibration
.
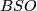 using the fibration
using the fibration Tex syntax error
\item Calculate the homotopy fibre of 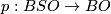 .
\end{enumerate}
.
\end{enumerate}
Now we can classify the orientations on a vector bundle 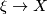 .
For this we need to know that the sequence
.
For this we need to know that the sequence
Tex syntax error
fits into the following diagram\footnote{It's non-trivial
to see that the functor  can be applied to each of those spaces.}:
\begin{center}
\begin{tikzpicture}[description/.style={fill=white,inner sep=2pt}]
\matrix (m) [matrix of math nodes, row sep=3em,
column sep=2.5em, text height=1.5ex, text depth=0.25ex]
{ \text{hofib}(p) & & BSO & & BO \\
can be applied to each of those spaces.}:
\begin{center}
\begin{tikzpicture}[description/.style={fill=white,inner sep=2pt}]
\matrix (m) [matrix of math nodes, row sep=3em,
column sep=2.5em, text height=1.5ex, text depth=0.25ex]
{ \text{hofib}(p) & & BSO & & BO \\
\Omega B\rm{hofib}(p) & & \Omega BBSO & & \Omega BBO \\ };
\path[->,font=\scriptsize]
(m-1-1) edge node[auto] { } (m-1-3)
(m-1-3) edge node[auto] {
} (m-1-3)
(m-1-3) edge node[auto] { } (m-1-5)
(m-2-1) edge node[auto] {
} (m-1-5)
(m-2-1) edge node[auto] {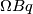 } (m-2-3)
(m-2-3) edge node[auto] {
} (m-2-3)
(m-2-3) edge node[auto] {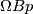 } (m-2-5)
(m-1-1) edge node[auto] {
} (m-2-5)
(m-1-1) edge node[auto] {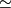 } (m-2-1)
(m-1-3) edge node[auto] {
} (m-2-1)
(m-1-3) edge node[auto] {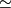 } (m-2-3)
(m-1-5) edge node[auto] {
} (m-2-3)
(m-1-5) edge node[auto] {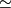 } (m-2-5);
\end{tikzpicture}
\end{center}
} (m-2-5);
\end{tikzpicture}
\end{center}
Tex syntax errorand
![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) (the latter one is the Whitney-sum
of vector bundles). Furthermore there is an action of
(the latter one is the Whitney-sum
of vector bundles). Furthermore there is an action of Tex syntax erroron
![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) induced
induced
by  .
.
Definition 0.1.
A vector bundle is called \underline{orientable} if its classifying map 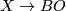 lifts along
lifts along 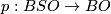 . An orientation is the choice of such a lift.
. An orientation is the choice of such a lift.
Let  denote a compact pointed space and
denote a compact pointed space and 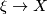 a vector bundle on
a vector bundle on  .
.
- Use Exercise 3.1 to show that
 is orientable if and only if its first Stiefel-Whitney class
is orientable if and only if its first Stiefel-Whitney class
vanishes.
- Show that the group
Tex syntax error
acts free and transitively on the set of
homotopy classes of lifts. Hint: Use the homotopy-lifting property and Exercise 3.1
- Given an interpretation of the group
Tex syntax error
.
Now there are similar results for spin structures (on oriented vector bundles).
Exercise 0.2.
Repeat Exercises 3.3 and 3.4 using the second Stiefel-Whitney class 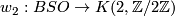 . The homotopy fibre of
. The homotopy fibre of 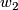 is BSpin, where Spin is the colimit over
is BSpin, where Spin is the colimit over 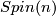 , the universal covers of
, the universal covers of 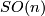 .
.
References
\to EO\to BO$. \item Calculate the homotopy groups of $BSO$ using the fibration $$BSO\to BO\to K(1,\bbz/2\bbz)\;.$$ \item Calculate the homotopy fibre of $p:BSO\to BO$. \end{enumerate} Now we can classify the orientations on a vector bundle $\xi\to X$. For this we need to know that the sequence $$\rm{hofib}(p)\to BSO \to BO$$ fits into the following diagram\footnote{It's non-trivial to see that the functor $B$ can be applied to each of those spaces.}: \begin{center} \begin{tikzpicture}[description/.style={fill=white,inner sep=2pt}] \matrix (m) [matrix of math nodes, row sep=3em, column sep=2.5em, text height=1.5ex, text depth=0.25ex] { \text{hofib}(p) & & BSO & & BO \ \Omega B\rm{hofib}(p) & & \Omega BBSO & & \Omega BBO \ }; \path[->,font=\scriptsize] (m-1-1) edge node[auto] {$q$} (m-1-3) (m-1-3) edge node[auto] {$p$} (m-1-5) (m-2-1) edge node[auto] {$\Omega B q$} (m-2-3) (m-2-3) edge node[auto] {$\Omega B p$} (m-2-5) (m-1-1) edge node[auto] {$\simeq$} (m-2-1) (m-1-3) edge node[auto] {$\simeq$} (m-2-3) (m-1-5) edge node[auto] {$\simeq$} (m-2-5); \end{tikzpicture} \end{center} Hence there are group structures on $[X,\rm{hofib}(p)]$ and $[X,BSO]$ (the latter one is the Whitney-sum of vector bundles). Furthermore there is an action of $[X,\rm{hofib}(p)]$ on $[X,BSO]$ induced by $q$. {{beginthm|Definition}} A vector bundle is called \underline{orientable} if its classifying map $X\to BO$ lifts along $p:BSO\to BO$. An ''orientation'' is the choice of such a lift. {{endthm}} Let $X$ denote a compact pointed space and $\xi\to X$ a vector bundle on $X$. #Use Exercise 3.1 to show that $\xi$ is orientable if and only if its first Stiefel-Whitney class vanishes. #Show that the group $[X,\rm{hofib}(p)]$ acts free and transitively on the set of homotopy classes of lifts. '''Hint:''' Use the homotopy-lifting property and Exercise 3.1 #Given an interpretation of the group $[X,\rm{hofib}(p)]$. Now there are similar results for spin structures (on oriented vector bundles). {{beginthm|Exercise}} Repeat Exercises 3.3 and 3.4 using the second Stiefel-Whitney class $w_2:BSO\to K(2,\Zz/2\Zz)$. The homotopy fibre of $w_2$ is BSpin, where Spin is the colimit over $Spin(n)$, the universal covers of $SO(n)$. {{endthm}} == References == {{#RefList:}} [[Category:Exercises]]g:Y\to Z of pointed topological space, we define the homotopy fiber as\footnote{Here is the space of paths starting at the base-point of
is the space of paths starting at the base-point of 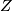 .}
.}
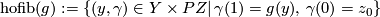
and we denote by  :
: 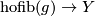 the projection.
the projection.
Show the following statement:\\A map
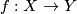 of pointed space has a lift
of pointed space has a lift Tex syntax erroralong

if and only if  is homotopic to the constant map.
is homotopic to the constant map.
Hint: This is a special case of Proposition 4.72 in [A. Hatcher, Algebraic Topology].
\subsection*{Classification of orientations and spin structures on vector bundles}
Recall the Definition of Eilenberg-MacLane-spaces.
We denote the space of pointed loops in a spaceby
.
\begin{enumerate}
\item Show: There is a homotopy equivalenceTex syntax error.\\
Hint: Use the uniqueness of Eilenberg-MacLane-spaces and the long exact sequence in homotopy
associated to the path-space-fibration
Tex syntax error
Recall that the path-space is contractible.
\item Show that the set of homotopy classes of pointed maps ![[Y,\Omega X]](/images/math/3/d/3/3d36331b6ffc21a47fc28e20effd0b46.png) has a group structure
induced by composition of paths.\\
Hint: This is similar to the group structure of the fundamental group.
has a group structure
induced by composition of paths.\\
Hint: This is similar to the group structure of the fundamental group.
\end{enumerate}
Tex syntax erroracts free and transitively on the set
of spin structures of an oriented vector bundle 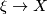 (X a compact pointed space).
(X a compact pointed space).
Tex syntax erroris due to the Exercise 3.2.}
Tex syntax error, where
Tex syntax errordenotes an Eilenberg-MacLane-space.
This looks similar to the statement of Theorem 3.45 in our talk.
So we first prove the statement about classification of spin structures. The warm-up is the classification of orientations: \begin{itemize}
\item The first Stiefel-Whitney class is a mapTex syntax error.
\item The homotopy fiber hofib() is
. \item The projection
is the map induced by
.
\end{itemize}
Assume that the homotopy groups of\item Calculate the homotopy groups ofare known. \begin{enumerate} \item Calculate the homotopy groups of
using the fibration
.
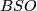 using the fibration
using the fibration Tex syntax error
\item Calculate the homotopy fibre of 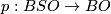 .
\end{enumerate}
.
\end{enumerate}
Now we can classify the orientations on a vector bundle 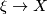 .
For this we need to know that the sequence
.
For this we need to know that the sequence
Tex syntax error
fits into the following diagram\footnote{It's non-trivial
to see that the functor  can be applied to each of those spaces.}:
\begin{center}
\begin{tikzpicture}[description/.style={fill=white,inner sep=2pt}]
\matrix (m) [matrix of math nodes, row sep=3em,
column sep=2.5em, text height=1.5ex, text depth=0.25ex]
{ \text{hofib}(p) & & BSO & & BO \\
can be applied to each of those spaces.}:
\begin{center}
\begin{tikzpicture}[description/.style={fill=white,inner sep=2pt}]
\matrix (m) [matrix of math nodes, row sep=3em,
column sep=2.5em, text height=1.5ex, text depth=0.25ex]
{ \text{hofib}(p) & & BSO & & BO \\
\Omega B\rm{hofib}(p) & & \Omega BBSO & & \Omega BBO \\ };
\path[->,font=\scriptsize]
(m-1-1) edge node[auto] { } (m-1-3)
(m-1-3) edge node[auto] {
} (m-1-3)
(m-1-3) edge node[auto] { } (m-1-5)
(m-2-1) edge node[auto] {
} (m-1-5)
(m-2-1) edge node[auto] {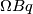 } (m-2-3)
(m-2-3) edge node[auto] {
} (m-2-3)
(m-2-3) edge node[auto] {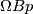 } (m-2-5)
(m-1-1) edge node[auto] {
} (m-2-5)
(m-1-1) edge node[auto] {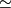 } (m-2-1)
(m-1-3) edge node[auto] {
} (m-2-1)
(m-1-3) edge node[auto] { } (m-2-3)
(m-1-5) edge node[auto] {
} (m-2-3)
(m-1-5) edge node[auto] {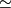 } (m-2-5);
\end{tikzpicture}
\end{center}
} (m-2-5);
\end{tikzpicture}
\end{center}
Tex syntax errorand
![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) (the latter one is the Whitney-sum
of vector bundles). Furthermore there is an action of
(the latter one is the Whitney-sum
of vector bundles). Furthermore there is an action of Tex syntax erroron
![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) induced
induced
by  .
.
Definition 0.1.
A vector bundle is called \underline{orientable} if its classifying map 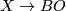 lifts along
lifts along 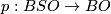 . An orientation is the choice of such a lift.
. An orientation is the choice of such a lift.
Let  denote a compact pointed space and
denote a compact pointed space and 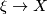 a vector bundle on
a vector bundle on  .
.
- Use Exercise 3.1 to show that
 is orientable if and only if its first Stiefel-Whitney class
is orientable if and only if its first Stiefel-Whitney class
vanishes.
- Show that the group
Tex syntax error
acts free and transitively on the set of
homotopy classes of lifts. Hint: Use the homotopy-lifting property and Exercise 3.1
- Given an interpretation of the group
Tex syntax error
.
Now there are similar results for spin structures (on oriented vector bundles).
Exercise 0.2.
Repeat Exercises 3.3 and 3.4 using the second Stiefel-Whitney class 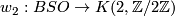 . The homotopy fibre of
. The homotopy fibre of 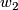 is BSpin, where Spin is the colimit over
is BSpin, where Spin is the colimit over 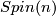 , the universal covers of
, the universal covers of 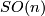 .
.