Bundle structures and lifting problems (Ex)
[edit] 1 Lifting maps
Given a (pointed) map 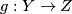 of pointed topological spaces, we define the homotopy fibre of
of pointed topological spaces, we define the homotopy fibre of  as
as
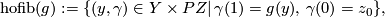
where  is the space of paths starting at the base-point of
is the space of paths starting at the base-point of 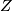 . We denote by
. We denote by  :
: 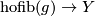 the projection.
the projection.
Exercise 2.1. Prove the following:
A map 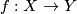 of pointed space has a lift
of pointed space has a lift 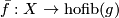 along
along  if and only if
if and only if  is homotopic to the constant map.
is homotopic to the constant map.
Hint 2.2. This is a special case of [Hatcher2002, Proposition 4.72].
[edit] 2 Classification of orientations and spin structures on vector bundles
Recall the Definition of Eilenberg-MacLane-spaces.
We denote the space of pointed loops in a space  by
by 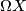 .
.
Exercise 4.1.
- Show: There is a homotopy equivalence
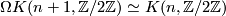 .
.
Hint: Use the uniqueness of Eilenberg-MacLane-spaces and the long exact sequence in homotopy associated to the path-space-fibration
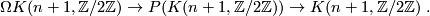
Recall that the path-space is contractible.
- Show that the set of homotopy classes of pointed maps
![[Y,\Omega X]](/images/math/3/d/3/3d36331b6ffc21a47fc28e20effd0b46.png) has a group structure induced by composition of paths.
has a group structure induced by composition of paths.
Hint: This is similar to the group structure of the fundamental group.
Maybe you've heard that the group 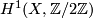 acts free and transitively on the set
of spin structures of an oriented vector bundle
acts free and transitively on the set
of spin structures of an oriented vector bundle 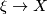 (X a compact pointed space).
Now recall that \ref{The group structure on
(X a compact pointed space).
Now recall that \ref{The group structure on 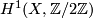 is due to the Exercise 3.2.}
is due to the Exercise 3.2.}
![H^1(X,\Zz/2\Zz)\cong [X,K(1,\Zz/2\Zz)]](/images/math/f/5/c/f5c4659152a2e7de9cb4f934ca5cf9e5.png) , where
, where 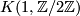 denotes an Eilenberg-MacLane-space.
denotes an Eilenberg-MacLane-space.
So we first prove the statement about classification of spin structures. The warm-up is the classification of orientations:
- The first Stiefel-Whitney class is a map
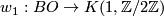 .
.
- The homotopy fiber hofib(
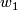 ) is
) is 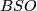 .
.
- The projection
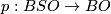 is the map induced by
is the map induced by 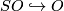 .
.
Exercise 4.2.
Assume that the homotopy groups of  are known.
are known.
- Calculate the homotopy groups of
 using the fibration
using the fibration 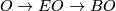 .
.
- Calculate the homotopy groups of
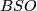 using the fibration
using the fibration 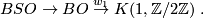
- Calculate the homotopy fibre of
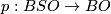 .
.
Now we can classify the orientations on a vector bundle 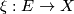 .
For this we need to know that the sequence
.
For this we need to know that the sequence
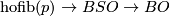
fits into the following diagram\footnote{It's non-trivial
to see that the functor  can be applied to each of those spaces.}:
can be applied to each of those spaces.}:
![\displaystyle \xymatrix{ \mathrm{hofib}(p) \ar[r]^q \ar[d]^{\simeq} & BSO \ar[r]^p \ar[d]^{\simeq} & BO \ar[d]^{\simeq} \\ \Omega B\mathrm{hofib}(p) \ar[r]^{\Omega Bq} & \Omega BBSO \ar[r]^{\Omega Bp} & \Omega BBO }](/images/math/7/5/e/75ee24c7ed2eb5bfd91d49e71ac204da.png)
Hence there are group structures on ![[X,\text{hofib}(p)]](/images/math/9/7/8/978bcfb0ba0034c61359e401a84462d3.png) and
and ![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) (the latter one is the Whitney-sum of vector bundles). Furthermore there is an action of
(the latter one is the Whitney-sum of vector bundles). Furthermore there is an action of ![[X,\text{hofib}(p)]](/images/math/9/7/8/978bcfb0ba0034c61359e401a84462d3.png) on
on ![[X,BSO]](/images/math/9/9/9/999c116c9d83c1d788b2710e4a033b65.png) induced by
induced by  .
.
Definition 4.3.
A vector bundle is called orientable if its classifying map 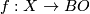 lifts along
lifts along 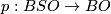 . An orientation is the choice of such a lift.
The first Stiefel-Whitney class of
. An orientation is the choice of such a lift.
The first Stiefel-Whitney class of  is defined as the composition
is defined as the composition 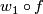 , where
, where 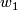 is defined as above.
is defined as above.
Exercise 4.4.
Let  denote a compact pointed space and
denote a compact pointed space and 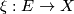 a vector bundle on
a vector bundle on  .
.
- Use Exercise 3.1 to show that
 is orientable if and only if its first Stiefel-Whitney class vanishes.
is orientable if and only if its first Stiefel-Whitney class vanishes.
- Show that the group
![[X,\text{hofib}(p)]](/images/math/9/7/8/978bcfb0ba0034c61359e401a84462d3.png) acts free and transitively on the set of homotopy classes of lifts.
acts free and transitively on the set of homotopy classes of lifts.
Hint: Use the homotopy-lifting property and Exercise 3.1
- Give an interpretation of the group
![[X,\text{hofib}(p)]](/images/math/9/7/8/978bcfb0ba0034c61359e401a84462d3.png) .
.
Now there are similar results for spin structures (on oriented vector bundles).
Exercise 4.5.
Repeat Exercises 3.3 and 3.4 using the second Stiefel-Whitney class 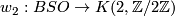 . The homotopy fibre of
. The homotopy fibre of 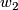 is BSpin, where Spin is the colimit over
is BSpin, where Spin is the colimit over 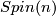 , the universal cover of
, the universal cover of 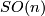 .
.
[edit] References
- [Hatcher2002] A. Hatcher, Algebraic topology, Cambridge University Press, 2002. MR1867354 (2002k:55001) Zbl 1044.55001