Bordism
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 09:46, 1 April 2011 and the changes since publication. |
|
The user responsible for this page is Taras Panov. No other user may edit this page at present. |
Contents |
1 Introduction
The theory of bordism is is one of the most deep and influential parts of the algebraic topology, which experienced a spectacular development in the 1960s. The main introductory reference is the monograph [Stong1968].
Basic geometric constructions of bordisms and cobordisms, as well as homotopical definitions are summarised here. For the more specific information, see B-Bordism and pages on specific bordism theories, such as unoriented, oriented and complex.
2 The bordism relation
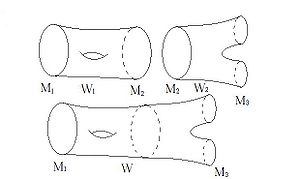
All manifolds here are assumed to be smooth, compact and closed
(without boundary), unless otherwise specified. Given two
 -dimensional manifolds
-dimensional manifolds  and
and  , a bordism
between them is an
, a bordism
between them is an  -dimensional manifold
-dimensional manifold  with boundary,
whose boundary is the disjoint union of
with boundary,
whose boundary is the disjoint union of  and
and  , that is,
, that is,
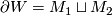 . If such
. If such  exists,
exists,  and
and  are called bordant. The bordism relation splits manifolds
into equivalence classes (see Figure), which are called
bordism classes.
are called bordant. The bordism relation splits manifolds
into equivalence classes (see Figure), which are called
bordism classes.
3 Unoriented bordism
We denote the bordism class of  by
by ![[M]](/images/math/f/a/0/fa08c3d5d2f54260952acc8a646b5025.png) , and denote by
, and denote by
 the set of bordism classes of
the set of bordism classes of  -dimensional
manifolds. Then
-dimensional
manifolds. Then  is an abelian group with respect
to the disjoint union operation:
is an abelian group with respect
to the disjoint union operation: ![[M_1]+[M_2]=[M_1\sqcup M_2]](/images/math/3/b/8/3b87653a49a8ea82afbc2c15dc9e95e6.png) .
Zero is represented by the bordism class of an empty set (which is
counted as a manifold in any dimension), or by the bordism class
of any manifold which bounds. We also have
.
Zero is represented by the bordism class of an empty set (which is
counted as a manifold in any dimension), or by the bordism class
of any manifold which bounds. We also have ![-[M]=[M]](/images/math/9/a/b/9ab407174f27a49e5094218d6baa4dbc.png) , so that
, so that
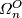 is a 2-torsion group.
is a 2-torsion group.
Set 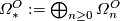 . The
product of bordism classes, namely
. The
product of bordism classes, namely ![[M_1]\times [M_2]=[M_1 \times M_2]](/images/math/b/c/a/bca399878134d24055d89500866ba471.png) , makes
, makes 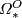 a graded commutative ring known as
the unoriented bordism ring.
a graded commutative ring known as
the unoriented bordism ring.
For any (good) space  the bordism relation can be extended to
maps of
the bordism relation can be extended to
maps of  -dimensional manifolds to
-dimensional manifolds to  : two maps
: two maps 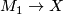 and
and
 are bordant if there is a bordism
are bordant if there is a bordism  between
between
 and
and  and the map
and the map 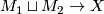 extends to a map
extends to a map
 . The set of bordism classes of maps
. The set of bordism classes of maps 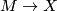 forms an
abelian group called the group of
forms an
abelian group called the group of  -dimensional unoriented
bordisms of
-dimensional unoriented
bordisms of  and denoted
and denoted 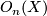 (other notations:
(other notations: 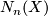 ,
,
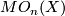 ).
).
The assignment 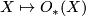 defines a generalised
homology theory, that is, satisfies the homotopy invariance, has
the excision property and exact sequences of pairs. For this
theory we have
defines a generalised
homology theory, that is, satisfies the homotopy invariance, has
the excision property and exact sequences of pairs. For this
theory we have 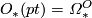 , and
, and 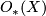 is an
is an
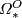 -module.
-module.
The Pontrjagin--Thom construction reduces the calculation of the bordism groups to a homotopical problem:
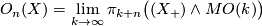
where 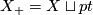 , and
, and  is the Thom space of
the universal
is the Thom space of
the universal  -dimensional bundle
-dimensional bundle 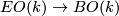 . The
cobordism groups are defined dually:
\[
. The
cobordism groups are defined dually:
\[
O^n(X)=\lim_{k\to\infty}[\Sigma^{k-n}(X_+),MO(k)]
\]
where ![[X,Y]](/images/math/e/1/9/e1921829b3d333bdf8227e9d46cb530b.png) denotes the set of homotopy classes of maps from
denotes the set of homotopy classes of maps from  to
to  . The resulting generalised cohomology theory is
multiplicative, which implies that
. The resulting generalised cohomology theory is
multiplicative, which implies that  is a
graded commutative ring. It follows from the definitions that
is a
graded commutative ring. It follows from the definitions that
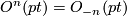 . The graded ring
. The graded ring 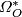 with
with
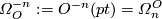 is called the
unoriented cobordism ring. It has nonzero elements only in
nonpositively graded components. The bordism ring
is called the
unoriented cobordism ring. It has nonzero elements only in
nonpositively graded components. The bordism ring 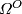 and the cobordism ring
and the cobordism ring 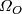 differ only by their
gradings, so the notions of the ``bordism class and ``cobordism
class of a manifold
differ only by their
gradings, so the notions of the ``bordism class and ``cobordism
class of a manifold  are interchangeable. The difference
between bordism and cobordism appears only for nontrivial
spaces
are interchangeable. The difference
between bordism and cobordism appears only for nontrivial
spaces  .
.
3.1 References
- [Stong1968] R. E. Stong, Notes on cobordism theory, Princeton University Press, Princeton, N.J., 1968. MR0248858 (40 #2108) Zbl 0277.57010
|
This page has not been refereed. The information given here might be incomplete or provisional. |
[[File:File:Example.jpg]]