6-manifolds: 2-connected
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
Let 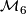 be the set of diffeomorphism classes of closed smooth simply-connected 2-connected 6-manifolds
be the set of diffeomorphism classes of closed smooth simply-connected 2-connected 6-manifolds  .
.
The classification 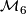 was one of Smale's first applications of the h-cobordism theorem [Smale1962a, Corollary 1.3]. The is a precise 6-dimensional analogue of the classification of orientable surfaces: every 2-connected 6-manifold
was one of Smale's first applications of the h-cobordism theorem [Smale1962a, Corollary 1.3]. The is a precise 6-dimensional analogue of the classification of orientable surfaces: every 2-connected 6-manifold  is diffeomorphic to a connected-sum
is diffeomorphic to a connected-sum
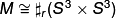
where by definition 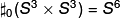 and in general
and in general  is determined by the formula for the Euler characteristic of
is determined by the formula for the Euler characteristic of 
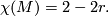
For the more general case where 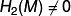 , see 6-manifolds: 1-connected.
, see 6-manifolds: 1-connected.
2 Construction and examples
The following gives a complete list of 2-connected 6-manifolds up to diffeomorphism:
-
 , the standard 6-sphere.
, the standard 6-sphere.
-
 , the
, the  -fold connected sum of
-fold connected sum of  .
.
3 Invariants
Suppose that  is diffeomorphic to
is diffeomorphic to  then:
then:
-
 ,
,
- the third Betti-number of
 is given by
is given by 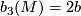 ,
,
- the Euler characteristic of
 is given by
is given by  ,
,
- the intersection form of
 is isomorphic to the sum of b-copies of
is isomorphic to the sum of b-copies of  , the standard skew-symmetric hyperbolic form on
, the standard skew-symmetric hyperbolic form on  .
.
4 Classification
Recall that the following theorem was stated in other words in the introduction:
Theorem 4.1 [Smale1962a, Corolary 1.3].
The semi-group of 2-connected 6-manifolds is generated by  .
.
Hence if  denotes the natural numbers we obtain a bijection
denotes the natural numbers we obtain a bijection
![\displaystyle \mathcal{M}_6\equiv \Nn,~~~[M] \mapsto \frac{1}{2}b_3(M).](/images/math/c/c/1/cc129827379a6fe6e748c39bfcbba618.png)
5 Further discussion
5.1 Topological 2-connected 6-manifolds
Let 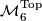 be the set of homeomorphism classes of topological 2-connected 6-manifolds.
be the set of homeomorphism classes of topological 2-connected 6-manifolds.
Theorem 5.1. Every topological 2-connected 6-manifold admits a smooth structure which is unique up to diffoemorphism. In particular, there is a bijection
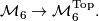
Proof.
For any such manifold  we have
we have  and so
and so  is smoothable (see 6-manifolds: 1-connected). Any two homeomorphic manifolds have the same Euler Characteristic and so by Theorem 4.1 are diffeomorphic.
is smoothable (see 6-manifolds: 1-connected). Any two homeomorphic manifolds have the same Euler Characteristic and so by Theorem 4.1 are diffeomorphic.

5.2 Mapping class groups
...
References
- [Smale1962a] S. Smale, On the structure of manifolds, Amer. J. Math. 84 (1962), 387–399. MR0153022 (27 #2991) Zbl 0109.41103