1-manifolds
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 08:32, 18 July 2013 and the changes since publication. |
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Introduction
According to the general definition of manifold, a manifold of dimension 1 is a topological space which is second countable (i.e., its topological structure has a countable base), satisfies the Hausdorff axiom (any two different points have disjoint neighborhoods) and each point of which has a neighbourhood homeomorphic either to the real line  or to the half-line
or to the half-line 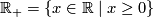 .
.
Manifolds of dimension 1 are called curves, but this name may lead to a confusion, because many mathematical objects share it. Even in the context of topology, the term curve may mean not only a manifold of dimension 1 with an additional structure, but, for instance, an immersion of a smooth manifold of dimension 1 to Euclidean space 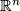 . To be on the safe side, we use an unambiguous term manifold of dimension 1 or 1-manifold.
. To be on the safe side, we use an unambiguous term manifold of dimension 1 or 1-manifold.
Specific properties of 1-manifolds can be related to the fact that the topological structure in a 1-manifold is defined by linear or cyclic ordering of points.
For other expositions about  -manifolds, see [Ghys2001], [Gale1987] and also [Fuks&Rokhlin1984, Chapter 3, Section 1.1.16-19].
-manifolds, see [Ghys2001], [Gale1987] and also [Fuks&Rokhlin1984, Chapter 3, Section 1.1.16-19].
2 Examples
- The real line:

- The half-line:
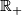
- The circle:
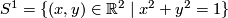
- The closed interval:
![I=[0,1]](/images/math/1/c/a/1ca01533a825141b051c7492a6fa6427.png)
3 Classification
3.1 Reduction to classification of connected manifolds
The following elementary facts hold for  -manifolds of any dimension
-manifolds of any dimension  .
.
Any manifold is homeomorphic to the disjoint sum of its connected components.
A connected component of an  -manifold is a
-manifold is a  -manifold.
-manifold.
Two manifolds are homeomorphic iff there exists a one-to-one correspondence between their components such that the corresponding components are homeomorphic.
3.2 Topological classification of connected 1-manifolds
Theorem 3.1. Any connected 1-manifold is homeomorphic to one of the following 4 manifolds:
- real line

- half-line
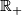
- circle
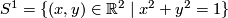
- closed interval
![I=[0,1]](/images/math/1/c/a/1ca01533a825141b051c7492a6fa6427.png) .
.
No two of these manifolds are homeomorphic to each other.
3.3 Characterizing the topological type of a connected 1-manifold
Theorem 3.2.
- Any connected non-compact 1-manifold without boundary is homeomorphic to
 .
.
- Any connected non-compact 1-manifold with non-empty boundary is homeomorphic to
 .
.
- Any connected closed 1-manifold is homeomorphic to
 .
.
- Any connected compact 1-manifold with non-empty boundary is homeomorphic to
 .
.
Thus, for connected 1-manifolds, two invariants, compactness and presence of boundary, form a complete system of topological invariants. Each of the invariants takes two values.
Theorems 3.1 and 3.2 above solve the topological classification problem for 1-manifolds in the most effective way that one can wish. Surprisingly, many Topology textbooks manage not to mention this fundamental result.
3.4 About proofs of the classification theorems
The proofs of Theorems 3.1 and 3.2 above are elementary. They can be found, e.g., in [Fuks&Rokhlin1984, Sections 3.1.1.16-19]. The core of them are the following simple lemmas:
 is homeomorphic either to
is homeomorphic either to  or
or  .
.Lemma 3.4. If a topological space  can be represented as the union of a nondecreasing sequence of open subsets, all homeomorphic to
can be represented as the union of a nondecreasing sequence of open subsets, all homeomorphic to  , then
, then  is homeomorphic to
is homeomorphic to  .
.
3.5 Corollaries
Theorem 3.5. Homotopy classification of 1-manifolds. Each connected 1-manifold is either contractible, or homotopy equivalent to circle.
Theorem 3.6. 0-manifolds cobordant to zero.
A compact 0-manifold  bounds a compact 1-manifold iff the number of points in
bounds a compact 1-manifold iff the number of points in  is even.
is even.
3.6 Characterizations of connected 1-manifolds in terms of set-theoretic topology
A subset  of a topological space
of a topological space  is said to separate
is said to separate  if
if 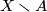 can be presented as a
union of two disjoint open sets.
can be presented as a
union of two disjoint open sets.
Theorem 3.7. (See [Moor,1920].)
Let  be a connected compact Hausdorff second countable topological space.
be a connected compact Hausdorff second countable topological space.
- If every two points separate
 , then
, then  is homeomorphic to the circle.
is homeomorphic to the circle.
- If each point, with two exceptions, separates
 , then
, then  is homeomorphic to
is homeomorphic to  .
.
Theorem 3.8. (See [Ward,1936].)
Let  be a connected locally compact Hausdorff second countable topological space.
be a connected locally compact Hausdorff second countable topological space.
- If the complement of each point in
 consists of two connected components, then
consists of two connected components, then  is homeomorphic to
is homeomorphic to  .
.
- If
 contains a point
contains a point  such that
such that  is connected and
is connected and 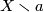 consists of two connected components for
consists of two connected components for
each 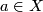 ,
, 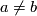 , then
, then  is homeomorphic to
is homeomorphic to  .
.
3.7 Non-Hausdorff complications
If we enlarge the collection of spaces by getting rid of the Hausdorff property, then the number of topological types of connected spaces becomes uncountable.
Indeed, one can take the disjoint union of two copies of the line  and identify
an open set in one of them with its copy in the other one by the identity map. The quotient space is connected and satisfies all the
requirements from the definition of 1-manifold except the Hausdorff axiom. In this way one can construct uncountably many pairwise non-homeomorphic spaces. To prove that they are not homeomorphic, one can use, for example, the topological type of the subset formed by those points that do not separate the space.
and identify
an open set in one of them with its copy in the other one by the identity map. The quotient space is connected and satisfies all the
requirements from the definition of 1-manifold except the Hausdorff axiom. In this way one can construct uncountably many pairwise non-homeomorphic spaces. To prove that they are not homeomorphic, one can use, for example, the topological type of the subset formed by those points that do not separate the space.
4 Invariants
As follows from the Theorems 3.1 and 3.2 above, the following invariants
- the number of connected components,
- the compactness of each connected component,
- and the number of boundary points of each connected component
determine the topological type of a 1-manifold.
The homotopy invariants of 1-manifolds are extremely simple. All homology and homotopy groups of dimensions 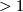 are trivial.
are trivial.
The tangent bundles of 1-manifolds are trivial.
5 Further discussion
5.1 Self-homeomorphisms
Theorem 5.1.
A map 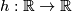 is a homeomorphism iff
is a homeomorphism iff  is a monotone bijection.
is a monotone bijection.
Proof. Let 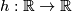 be a homeomorphism. First, observe that
be a homeomorphism. First, observe that  maps every ray to a ray. Indeed,
for any
maps every ray to a ray. Indeed,
for any 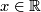 , the map
, the map  induces a homeomorphism
induces a homeomorphism 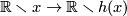 .
The rays
.
The rays 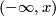 and
and 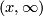 are connected components of
are connected components of 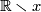 .
Therefore their images are connected components
.
Therefore their images are connected components 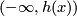 and
and 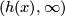 of
of 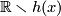 .
.
Observe that rays have the same direction iff one of them is contained in the other one, that is their intersection is a ray. Therefore two rays of the same dirction are mapped by  to rays with the same direction. Thus rays
to rays with the same direction. Thus rays 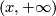 are mapped either all to rays
are mapped either all to rays 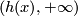 or all to
or all to 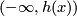 . Thus
. Thus  is monotone.
is monotone.
Let 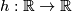 be a monotone bijection. Then the image and preimage under
be a monotone bijection. Then the image and preimage under  of any open interval is an open interval. Therefore, both
of any open interval is an open interval. Therefore, both  and
and 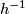 are continuous, and hence
are continuous, and hence  is a homeomorphism.
is a homeomorphism.

The following theorem can be proved similarly or can be deduced from Theorem 5.1
Theorem 5.2.
- A map
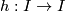 is a homeomorphism iff
is a homeomorphism iff  is a monotone bijection.
is a monotone bijection.
- A map
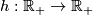 is a homeomorphism iff
is a homeomorphism iff  is a monotone increasing bijection.
is a monotone increasing bijection.
- A map
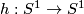 is a homeomorphism iff
is a homeomorphism iff  is a bijection that either preserves or reverses the cyclic order of points on
is a bijection that either preserves or reverses the cyclic order of points on  .
.
5.2 Orientations
An orientation of a 1-manifold can be interpreted via linear or cyclic orderings of their points.
An orientation of a connected non-closed 1-manifold is a linear order on the set of its points such that the corresponding interval topology coincides with the topology of this manifold.
An orientation of a connected closed 1-manifold is a cyclic order on the set of its points such that the topology of this cyclic order coincides with the topology of the 1-manifold.
An orientation of an arbitrary 1-manifold is a collection of orientations of its connected components (each component is equipped with an orientation).
Any 1-manifold admits an orientation.
The half-line  does not admit a homeomorphism reversing orientation. Any connected 1-manifold non-homeomorphic to
does not admit a homeomorphism reversing orientation. Any connected 1-manifold non-homeomorphic to  admits an orientation reversing map. Thus, there are 5 topological types of oriented connected 1-manifolds: the topological type of the non-oriented half-line splits into the oriented topological types of
admits an orientation reversing map. Thus, there are 5 topological types of oriented connected 1-manifolds: the topological type of the non-oriented half-line splits into the oriented topological types of  and
and 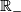 with the orientations induced by the standard order.
with the orientations induced by the standard order.
5.3 Smooth structures
Any 1-manifold admits a smooth structure.
If smooth 1-manifolds  and
and  are homeomorphic, then they are also diffeomorphic. Moreover,
are homeomorphic, then they are also diffeomorphic. Moreover,
Theorem 5.3.
Any homeomorphism between two smooth 1-manifolds can be approximated in the 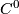 -topology by a diffeomorphism.
-topology by a diffeomorphism.
Proof. By Theorems 5.1 and 5.2, a homeomorphism is monotone in the appropriate sense. Choose a net of points in the source such that the image of each of them is sufficiently close to the images of its neighbors. Take a smooth monotone bijection coinciding with the homeomorphism at the chosen points.

5.4 Triangulations
Any 1-manifold admits a triangulation. A triangulation of a non-compact connected 1-manifold is unique up to homeomorphism.
A compact 1-manifold has non-homeomorphic triangulations, but they are easy to classify up to homeomorphism.
On circle the topological type of a triangulation is defined by the number of 1-simplices. This number can take
any integral value 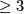 . Similarly, the topological type of a triangulation of
. Similarly, the topological type of a triangulation of  is defined by the number of 1-simplices, which can take any positive integral value.
is defined by the number of 1-simplices, which can take any positive integral value.
5.5 Inner metrics
Recall that a metric on a path-connected space is said to be inner if the distance between any two points is equal to the infimum of lengths of paths connecting the points, and that the length of a path 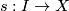 in a metric space
in a metric space  with metric
with metric 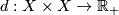 is
is
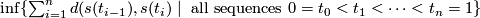 .
.
Any connected 1-manifold admits an inner metric.
A connected 1-manifold with an inner metric is defined up to isometry by the diameter of the space. Recall that the diameter of a metric space  with metric
with metric  is
is  .
.
For each value of the diameter there is a standard model for the inner metric space. For the four homeomorphism types of connected 1-manifolds these standard models are as follows.
- For
 with diameter
with diameter ![D\in (0,\infty]](/images/math/3/8/f/38f0ca5142f6305c572f6cff57d18749.png) this is
this is 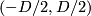 .
.
- For
 with diameter
with diameter ![D\in(0,\infty]](/images/math/a/9/8/a98f21564b6d664f43fa7a6b0614ea3a.png) this is
this is 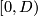 .
.
- For a circle with inner metric of diameter
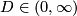 this is the circle
this is the circle 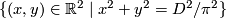 of radius
of radius 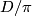 on the plane with the inner metric.
on the plane with the inner metric.
- For
 with diameter
with diameter 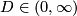 this is
this is ![[0,D]](/images/math/9/2/b/92beb9ea7237e94d7250de8c44fa6175.png) .
.
An inner metric on a connected 1-manifold defines a unique smooth structure on the manifold, namely, the smooth structure induced by the isometry to the corresponding standard model from the list above.
5.6 Mapping class groups
Recall that the mapping class group of a manifold  is the quotient group of the group
is the quotient group of the group 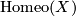 of all homeomorphisms
of all homeomorphisms  by the normal subgroup of homeomorphisms isotopic to the identity.
In other words, the mapping class group of
by the normal subgroup of homeomorphisms isotopic to the identity.
In other words, the mapping class group of  is
is  .
.
An orientation reversing homeomorphism cannot be isotopic to an orientation preserving homeomorphism. For auto-homeomorphisms of a connected 1-manifold this is the only obstruction to being isotopic:
Theorem 5.4. Any two auto-homeomorphisms of a connected 1-manifold that are either both orientation preserving, or both orientation reversing are isotopic.
This is a corollary of the following two obvious lemmas.
Lemma 5.5. On rectilinear isotopy. Let  be one of the following 1-manifolds:
be one of the following 1-manifolds:  ,
,  , or
, or  . Let
. Let 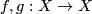 be two monotone bijections that are either both increasing or both decreasing. Then the family
be two monotone bijections that are either both increasing or both decreasing. Then the family 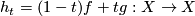 with
with ![t\in[0,1]](/images/math/4/0/d/40de672f62756d608425bb0e58f1dfa1.png) consists of monotone bijections (and hence is an isotopy between
consists of monotone bijections (and hence is an isotopy between  and
and  ).
).
Lemma 5.6. Let 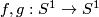 be two bijections that either both preserve or both reverse the standard
cyclic order of points on
be two bijections that either both preserve or both reverse the standard
cyclic order of points on  . Let
. Let  and
and  coincide at
coincide at 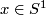 . Then
. Then  and
and  are isotopic via the canonical
isotopy which is stationary at
are isotopic via the canonical
isotopy which is stationary at  and is provided on the complement of
and is provided on the complement of  by stereographic projections and the rectilinear isotopy from Lemma 5.5 of the corresponding self-homeomorphisms of
by stereographic projections and the rectilinear isotopy from Lemma 5.5 of the corresponding self-homeomorphisms of  .
.
Corollary 5.7.
 and
and

Remark. All the statements in this section remains true, if everywhere the word homeomorphism is replaced by the word diffeomorphism and  is replaced by
is replaced by 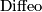 .
.
5.7 Homotopy types of groups of auto-homeomorphisms
The group 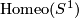 contains
contains 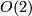 as a subgroup, which is its deformation retract. It follows from Lemma 5.6. More precisely, for each point
as a subgroup, which is its deformation retract. It follows from Lemma 5.6. More precisely, for each point 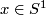 , Lemma 5.6 provides a deformation retraction
, Lemma 5.6 provides a deformation retraction 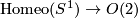 .
.
Similarly, the group of auto-homeomorphisms of  isotopic to identity contains
isotopic to identity contains 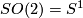 as a subgroup, which is its deformation retract.
as a subgroup, which is its deformation retract.
The groups of auto-homeomorphisms of  ,
,  and
and  which are isotopic to identity are contractible. The contraction is provided by the rectilinear isotopy from Lemma 5.5 applied to
which are isotopic to identity are contractible. The contraction is provided by the rectilinear isotopy from Lemma 5.5 applied to 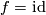 and an arbitrary
and an arbitrary  .
.
Thus for each connected 1-manifold  the group of homeomorphisms
the group of homeomorphisms 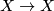 isotopic to identity is homotopy equivalent to
isotopic to identity is homotopy equivalent to  .
.
5.8 Finite group actions
There are no non-trivial free finite group actions on contractible 1-manifolds.
If a finite group  acts freely on the circle than
acts freely on the circle than  is cyclic. Any finite cyclic group has a linear
free action on
is cyclic. Any finite cyclic group has a linear
free action on  . Any free action of a finite cyclic group on
. Any free action of a finite cyclic group on  is conjugate to a linear action.
is conjugate to a linear action.
Any periodic orientation reversing homeomorphism 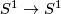 is an involution (i.e., has period 2).
It is conjugate to a symmetry of
is an involution (i.e., has period 2).
It is conjugate to a symmetry of  against its diameter.
against its diameter.
Any non-trivial periodic homeomorphism of a connected 1-manifold with a fixed point is an involution reversing orientation.
A finite group acting effectively on  is either cyclic or dihedral, and the action is conjugate to a linear one. The standard actions of cyclic and dihedral groups on circle are provided by the symmetry groups of
regular polygons.
is either cyclic or dihedral, and the action is conjugate to a linear one. The standard actions of cyclic and dihedral groups on circle are provided by the symmetry groups of
regular polygons.
A non-trivial finite group acting effectively on 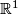 or
or ![[0,1]](/images/math/e/6/d/e6d5b5dafb82c2bf8b920a812188ac06.png) is a cyclic group of order 2. The action of the non-unit element is conjugate to the symmetry against a point.
is a cyclic group of order 2. The action of the non-unit element is conjugate to the symmetry against a point.
There is no non-trivial action of a finite group in  .
.
5.9 Surgery
Any 1-manifold can be transformed by surgeries to any other 1-manifold with the same boundary.
If given two 1-manifolds with the same boundary are oriented and the induced orientations on the boundary coincide, then the surgery can be chosen preserving the orientation (this means that the corresponding cobordism is an oriented 2-manifold and its orientation induces on the boundary the given orientation on one of the 1-manifolds and the orientation opposite to the given one on the other 1-manifold).
An index 1 surgery preserving orientation on closed 1-manifold changes the number of connected components by 1. An index 1 surgery on a closed 1-manifold, which does not preserve any orientation, preserves the number of connected components.
5.10 Connected sums
The notion of connected sum is defined for 1-manifolds, but the connectivity of the outcome is different in dimension 1 compared to other dimensions.
Indeed term connected sum can be misleading in dimension 1 since a connected sum
of connected 1-manifolds may be not connected. For example a connected sum of two
copies of  is a disjoint sum of two copies of
is a disjoint sum of two copies of  .
.
Note that connected sum is only a well defined operation on oriented manifolds and one has to be careful with the orientations. For example

6 References
- [Fuks&Rokhlin1984] D. B. Fuks and V. A. Rokhlin, Beginner's course in topology. Geometric chapters. Translated from the Russian by A. Iacob. Universitext. Springer Series in Soviet Mathematics. Springer-Verlag, Berlin, 1984. MR759162 (86a:57001) Zbl 0562.54003
- [Gale1987] D. Gale, The Teaching of Mathematics: The Classification of 1-Manifolds: A Take-Home Exam, Amer. Math. Monthly 94 (1987), no.2, 170–175. MR1541035 Zbl 0621.57001
- [Ghys2001] E. Ghys, Groups acting on the circle, Enseign. Math. (2) 47 (2001), no.3-4, 329–407. MR1876932 (2003a:37032) Zbl 1044.37033
- [Moor,1920] R.L.Moore, Concerning simple continuous curves, Trans. Amer. Math. Soc. 21 (1920), 333—347.
- [Ward,1936] A.J.Ward, The topological characterization of an open linear interval, Proc. London Math. Soc.(2) 41 (1936), 191-198.
7 External links
- The Encylopedia of Mathematics article on one-dimensional manifolds.
- The Encylopedia of Mathematics article on lines.
- The Wikipedia page about curves.
{{beginthm|Theorem}} Any homeomorphism between two smooth 1-manifolds can be approximated in the $C^0$-topology by a diffeomorphism. {{endthm}} {{beginproof}} By Theorems \ref{thm:homeomorphisms-of-line} and \ref{thm:homeomorphisms-of-others}, a homeomorphism is monotone in the appropriate sense. Choose a net of points in the source such that the image of each of them is sufficiently close to the images of its neighbors. Take a smooth monotone bijection coinciding with the homeomorphism at the chosen points. {{endproof}}
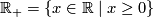 .
.
Manifolds of dimension 1 are called curves, but this name may lead to a confusion, because many mathematical objects share it. Even in the context of topology, the term curve may mean not only a manifold of dimension 1 with an additional structure, but, for instance, an immersion of a smooth manifold of dimension 1 to Euclidean space 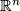 . To be on the safe side, we use an unambiguous term manifold of dimension 1 or 1-manifold.
. To be on the safe side, we use an unambiguous term manifold of dimension 1 or 1-manifold.
Specific properties of 1-manifolds can be related to the fact that the topological structure in a 1-manifold is defined by linear or cyclic ordering of points.
For other expositions about  -manifolds, see [Ghys2001], [Gale1987] and also [Fuks&Rokhlin1984, Chapter 3, Section 1.1.16-19].
-manifolds, see [Ghys2001], [Gale1987] and also [Fuks&Rokhlin1984, Chapter 3, Section 1.1.16-19].
2 Examples
- The real line:

- The half-line:
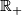
- The circle:
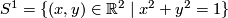
- The closed interval:
![I=[0,1]](/images/math/1/c/a/1ca01533a825141b051c7492a6fa6427.png)
3 Classification
3.1 Reduction to classification of connected manifolds
The following elementary facts hold for  -manifolds of any dimension
-manifolds of any dimension  .
.
Any manifold is homeomorphic to the disjoint sum of its connected components.
A connected component of an  -manifold is a
-manifold is a  -manifold.
-manifold.
Two manifolds are homeomorphic iff there exists a one-to-one correspondence between their components such that the corresponding components are homeomorphic.
3.2 Topological classification of connected 1-manifolds
Theorem 3.1. Any connected 1-manifold is homeomorphic to one of the following 4 manifolds:
- real line

- half-line
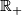
- circle
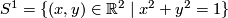
- closed interval
![I=[0,1]](/images/math/1/c/a/1ca01533a825141b051c7492a6fa6427.png) .
.
No two of these manifolds are homeomorphic to each other.
3.3 Characterizing the topological type of a connected 1-manifold
Theorem 3.2.
- Any connected non-compact 1-manifold without boundary is homeomorphic to
 .
.
- Any connected non-compact 1-manifold with non-empty boundary is homeomorphic to
 .
.
- Any connected closed 1-manifold is homeomorphic to
 .
.
- Any connected compact 1-manifold with non-empty boundary is homeomorphic to
 .
.
Thus, for connected 1-manifolds, two invariants, compactness and presence of boundary, form a complete system of topological invariants. Each of the invariants takes two values.
Theorems 3.1 and 3.2 above solve the topological classification problem for 1-manifolds in the most effective way that one can wish. Surprisingly, many Topology textbooks manage not to mention this fundamental result.
3.4 About proofs of the classification theorems
The proofs of Theorems 3.1 and 3.2 above are elementary. They can be found, e.g., in [Fuks&Rokhlin1984, Sections 3.1.1.16-19]. The core of them are the following simple lemmas:
 is homeomorphic either to
is homeomorphic either to  or
or  .
.Lemma 3.4. If a topological space  can be represented as the union of a nondecreasing sequence of open subsets, all homeomorphic to
can be represented as the union of a nondecreasing sequence of open subsets, all homeomorphic to  , then
, then  is homeomorphic to
is homeomorphic to  .
.
3.5 Corollaries
Theorem 3.5. Homotopy classification of 1-manifolds. Each connected 1-manifold is either contractible, or homotopy equivalent to circle.
Theorem 3.6. 0-manifolds cobordant to zero.
A compact 0-manifold  bounds a compact 1-manifold iff the number of points in
bounds a compact 1-manifold iff the number of points in  is even.
is even.
3.6 Characterizations of connected 1-manifolds in terms of set-theoretic topology
A subset  of a topological space
of a topological space  is said to separate
is said to separate  if
if 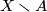 can be presented as a
union of two disjoint open sets.
can be presented as a
union of two disjoint open sets.
Theorem 3.7. (See [Moor,1920].)
Let  be a connected compact Hausdorff second countable topological space.
be a connected compact Hausdorff second countable topological space.
- If every two points separate
 , then
, then  is homeomorphic to the circle.
is homeomorphic to the circle.
- If each point, with two exceptions, separates
 , then
, then  is homeomorphic to
is homeomorphic to  .
.
Theorem 3.8. (See [Ward,1936].)
Let  be a connected locally compact Hausdorff second countable topological space.
be a connected locally compact Hausdorff second countable topological space.
- If the complement of each point in
 consists of two connected components, then
consists of two connected components, then  is homeomorphic to
is homeomorphic to  .
.
- If
 contains a point
contains a point  such that
such that  is connected and
is connected and 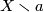 consists of two connected components for
consists of two connected components for
each 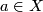 ,
, 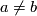 , then
, then  is homeomorphic to
is homeomorphic to  .
.
3.7 Non-Hausdorff complications
If we enlarge the collection of spaces by getting rid of the Hausdorff property, then the number of topological types of connected spaces becomes uncountable.
Indeed, one can take the disjoint union of two copies of the line  and identify
an open set in one of them with its copy in the other one by the identity map. The quotient space is connected and satisfies all the
requirements from the definition of 1-manifold except the Hausdorff axiom. In this way one can construct uncountably many pairwise non-homeomorphic spaces. To prove that they are not homeomorphic, one can use, for example, the topological type of the subset formed by those points that do not separate the space.
and identify
an open set in one of them with its copy in the other one by the identity map. The quotient space is connected and satisfies all the
requirements from the definition of 1-manifold except the Hausdorff axiom. In this way one can construct uncountably many pairwise non-homeomorphic spaces. To prove that they are not homeomorphic, one can use, for example, the topological type of the subset formed by those points that do not separate the space.
4 Invariants
As follows from the Theorems 3.1 and 3.2 above, the following invariants
- the number of connected components,
- the compactness of each connected component,
- and the number of boundary points of each connected component
determine the topological type of a 1-manifold.
The homotopy invariants of 1-manifolds are extremely simple. All homology and homotopy groups of dimensions 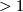 are trivial.
are trivial.
The tangent bundles of 1-manifolds are trivial.
5 Further discussion
5.1 Self-homeomorphisms
Theorem 5.1.
A map 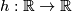 is a homeomorphism iff
is a homeomorphism iff  is a monotone bijection.
is a monotone bijection.
Proof. Let 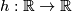 be a homeomorphism. First, observe that
be a homeomorphism. First, observe that  maps every ray to a ray. Indeed,
for any
maps every ray to a ray. Indeed,
for any 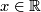 , the map
, the map  induces a homeomorphism
induces a homeomorphism 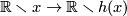 .
The rays
.
The rays 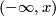 and
and 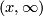 are connected components of
are connected components of 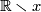 .
Therefore their images are connected components
.
Therefore their images are connected components 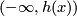 and
and 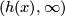 of
of 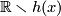 .
.
Observe that rays have the same direction iff one of them is contained in the other one, that is their intersection is a ray. Therefore two rays of the same dirction are mapped by  to rays with the same direction. Thus rays
to rays with the same direction. Thus rays 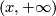 are mapped either all to rays
are mapped either all to rays 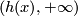 or all to
or all to 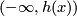 . Thus
. Thus  is monotone.
is monotone.
Let 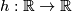 be a monotone bijection. Then the image and preimage under
be a monotone bijection. Then the image and preimage under  of any open interval is an open interval. Therefore, both
of any open interval is an open interval. Therefore, both  and
and 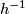 are continuous, and hence
are continuous, and hence  is a homeomorphism.
is a homeomorphism.

The following theorem can be proved similarly or can be deduced from Theorem 5.1
Theorem 5.2.
- A map
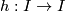 is a homeomorphism iff
is a homeomorphism iff  is a monotone bijection.
is a monotone bijection.
- A map
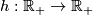 is a homeomorphism iff
is a homeomorphism iff  is a monotone increasing bijection.
is a monotone increasing bijection.
- A map
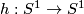 is a homeomorphism iff
is a homeomorphism iff  is a bijection that either preserves or reverses the cyclic order of points on
is a bijection that either preserves or reverses the cyclic order of points on  .
.
5.2 Orientations
An orientation of a 1-manifold can be interpreted via linear or cyclic orderings of their points.
An orientation of a connected non-closed 1-manifold is a linear order on the set of its points such that the corresponding interval topology coincides with the topology of this manifold.
An orientation of a connected closed 1-manifold is a cyclic order on the set of its points such that the topology of this cyclic order coincides with the topology of the 1-manifold.
An orientation of an arbitrary 1-manifold is a collection of orientations of its connected components (each component is equipped with an orientation).
Any 1-manifold admits an orientation.
The half-line  does not admit a homeomorphism reversing orientation. Any connected 1-manifold non-homeomorphic to
does not admit a homeomorphism reversing orientation. Any connected 1-manifold non-homeomorphic to  admits an orientation reversing map. Thus, there are 5 topological types of oriented connected 1-manifolds: the topological type of the non-oriented half-line splits into the oriented topological types of
admits an orientation reversing map. Thus, there are 5 topological types of oriented connected 1-manifolds: the topological type of the non-oriented half-line splits into the oriented topological types of  and
and 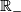 with the orientations induced by the standard order.
with the orientations induced by the standard order.
5.3 Smooth structures
Any 1-manifold admits a smooth structure.
If smooth 1-manifolds  and
and  are homeomorphic, then they are also diffeomorphic. Moreover,
are homeomorphic, then they are also diffeomorphic. Moreover,
Theorem 5.3.
Any homeomorphism between two smooth 1-manifolds can be approximated in the 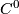 -topology by a diffeomorphism.
-topology by a diffeomorphism.
Proof. By Theorems 5.1 and 5.2, a homeomorphism is monotone in the appropriate sense. Choose a net of points in the source such that the image of each of them is sufficiently close to the images of its neighbors. Take a smooth monotone bijection coinciding with the homeomorphism at the chosen points.

5.4 Triangulations
Any 1-manifold admits a triangulation. A triangulation of a non-compact connected 1-manifold is unique up to homeomorphism.
A compact 1-manifold has non-homeomorphic triangulations, but they are easy to classify up to homeomorphism.
On circle the topological type of a triangulation is defined by the number of 1-simplices. This number can take
any integral value 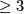 . Similarly, the topological type of a triangulation of
. Similarly, the topological type of a triangulation of  is defined by the number of 1-simplices, which can take any positive integral value.
is defined by the number of 1-simplices, which can take any positive integral value.
5.5 Inner metrics
Recall that a metric on a path-connected space is said to be inner if the distance between any two points is equal to the infimum of lengths of paths connecting the points, and that the length of a path 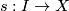 in a metric space
in a metric space  with metric
with metric 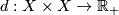 is
is
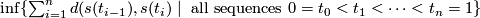 .
.
Any connected 1-manifold admits an inner metric.
A connected 1-manifold with an inner metric is defined up to isometry by the diameter of the space. Recall that the diameter of a metric space  with metric
with metric  is
is  .
.
For each value of the diameter there is a standard model for the inner metric space. For the four homeomorphism types of connected 1-manifolds these standard models are as follows.
- For
 with diameter
with diameter ![D\in (0,\infty]](/images/math/3/8/f/38f0ca5142f6305c572f6cff57d18749.png) this is
this is 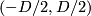 .
.
- For
 with diameter
with diameter ![D\in(0,\infty]](/images/math/a/9/8/a98f21564b6d664f43fa7a6b0614ea3a.png) this is
this is 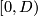 .
.
- For a circle with inner metric of diameter
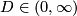 this is the circle
this is the circle 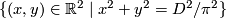 of radius
of radius 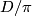 on the plane with the inner metric.
on the plane with the inner metric.
- For
 with diameter
with diameter 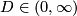 this is
this is ![[0,D]](/images/math/9/2/b/92beb9ea7237e94d7250de8c44fa6175.png) .
.
An inner metric on a connected 1-manifold defines a unique smooth structure on the manifold, namely, the smooth structure induced by the isometry to the corresponding standard model from the list above.
5.6 Mapping class groups
Recall that the mapping class group of a manifold  is the quotient group of the group
is the quotient group of the group 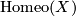 of all homeomorphisms
of all homeomorphisms  by the normal subgroup of homeomorphisms isotopic to the identity.
In other words, the mapping class group of
by the normal subgroup of homeomorphisms isotopic to the identity.
In other words, the mapping class group of  is
is  .
.
An orientation reversing homeomorphism cannot be isotopic to an orientation preserving homeomorphism. For auto-homeomorphisms of a connected 1-manifold this is the only obstruction to being isotopic:
Theorem 5.4. Any two auto-homeomorphisms of a connected 1-manifold that are either both orientation preserving, or both orientation reversing are isotopic.
This is a corollary of the following two obvious lemmas.
Lemma 5.5. On rectilinear isotopy. Let  be one of the following 1-manifolds:
be one of the following 1-manifolds:  ,
,  , or
, or  . Let
. Let 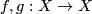 be two monotone bijections that are either both increasing or both decreasing. Then the family
be two monotone bijections that are either both increasing or both decreasing. Then the family 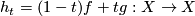 with
with ![t\in[0,1]](/images/math/4/0/d/40de672f62756d608425bb0e58f1dfa1.png) consists of monotone bijections (and hence is an isotopy between
consists of monotone bijections (and hence is an isotopy between  and
and  ).
).
Lemma 5.6. Let 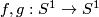 be two bijections that either both preserve or both reverse the standard
cyclic order of points on
be two bijections that either both preserve or both reverse the standard
cyclic order of points on  . Let
. Let  and
and  coincide at
coincide at 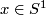 . Then
. Then  and
and  are isotopic via the canonical
isotopy which is stationary at
are isotopic via the canonical
isotopy which is stationary at  and is provided on the complement of
and is provided on the complement of  by stereographic projections and the rectilinear isotopy from Lemma 5.5 of the corresponding self-homeomorphisms of
by stereographic projections and the rectilinear isotopy from Lemma 5.5 of the corresponding self-homeomorphisms of  .
.
Corollary 5.7.
 and
and

Remark. All the statements in this section remains true, if everywhere the word homeomorphism is replaced by the word diffeomorphism and  is replaced by
is replaced by 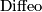 .
.
5.7 Homotopy types of groups of auto-homeomorphisms
The group 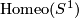 contains
contains 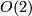 as a subgroup, which is its deformation retract. It follows from Lemma 5.6. More precisely, for each point
as a subgroup, which is its deformation retract. It follows from Lemma 5.6. More precisely, for each point 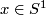 , Lemma 5.6 provides a deformation retraction
, Lemma 5.6 provides a deformation retraction 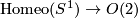 .
.
Similarly, the group of auto-homeomorphisms of  isotopic to identity contains
isotopic to identity contains 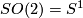 as a subgroup, which is its deformation retract.
as a subgroup, which is its deformation retract.
The groups of auto-homeomorphisms of  ,
,  and
and  which are isotopic to identity are contractible. The contraction is provided by the rectilinear isotopy from Lemma 5.5 applied to
which are isotopic to identity are contractible. The contraction is provided by the rectilinear isotopy from Lemma 5.5 applied to 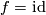 and an arbitrary
and an arbitrary  .
.
Thus for each connected 1-manifold  the group of homeomorphisms
the group of homeomorphisms 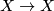 isotopic to identity is homotopy equivalent to
isotopic to identity is homotopy equivalent to  .
.
5.8 Finite group actions
There are no non-trivial free finite group actions on contractible 1-manifolds.
If a finite group  acts freely on the circle than
acts freely on the circle than  is cyclic. Any finite cyclic group has a linear
free action on
is cyclic. Any finite cyclic group has a linear
free action on  . Any free action of a finite cyclic group on
. Any free action of a finite cyclic group on  is conjugate to a linear action.
is conjugate to a linear action.
Any periodic orientation reversing homeomorphism 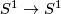 is an involution (i.e., has period 2).
It is conjugate to a symmetry of
is an involution (i.e., has period 2).
It is conjugate to a symmetry of  against its diameter.
against its diameter.
Any non-trivial periodic homeomorphism of a connected 1-manifold with a fixed point is an involution reversing orientation.
A finite group acting effectively on  is either cyclic or dihedral, and the action is conjugate to a linear one. The standard actions of cyclic and dihedral groups on circle are provided by the symmetry groups of
regular polygons.
is either cyclic or dihedral, and the action is conjugate to a linear one. The standard actions of cyclic and dihedral groups on circle are provided by the symmetry groups of
regular polygons.
A non-trivial finite group acting effectively on 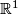 or
or ![[0,1]](/images/math/e/6/d/e6d5b5dafb82c2bf8b920a812188ac06.png) is a cyclic group of order 2. The action of the non-unit element is conjugate to the symmetry against a point.
is a cyclic group of order 2. The action of the non-unit element is conjugate to the symmetry against a point.
There is no non-trivial action of a finite group in  .
.
5.9 Surgery
Any 1-manifold can be transformed by surgeries to any other 1-manifold with the same boundary.
If given two 1-manifolds with the same boundary are oriented and the induced orientations on the boundary coincide, then the surgery can be chosen preserving the orientation (this means that the corresponding cobordism is an oriented 2-manifold and its orientation induces on the boundary the given orientation on one of the 1-manifolds and the orientation opposite to the given one on the other 1-manifold).
An index 1 surgery preserving orientation on closed 1-manifold changes the number of connected components by 1. An index 1 surgery on a closed 1-manifold, which does not preserve any orientation, preserves the number of connected components.
5.10 Connected sums
The notion of connected sum is defined for 1-manifolds, but the connectivity of the outcome is different in dimension 1 compared to other dimensions.
Indeed term connected sum can be misleading in dimension 1 since a connected sum
of connected 1-manifolds may be not connected. For example a connected sum of two
copies of  is a disjoint sum of two copies of
is a disjoint sum of two copies of  .
.
Note that connected sum is only a well defined operation on oriented manifolds and one has to be careful with the orientations. For example

6 References
- [Fuks&Rokhlin1984] D. B. Fuks and V. A. Rokhlin, Beginner's course in topology. Geometric chapters. Translated from the Russian by A. Iacob. Universitext. Springer Series in Soviet Mathematics. Springer-Verlag, Berlin, 1984. MR759162 (86a:57001) Zbl 0562.54003
- [Gale1987] D. Gale, The Teaching of Mathematics: The Classification of 1-Manifolds: A Take-Home Exam, Amer. Math. Monthly 94 (1987), no.2, 170–175. MR1541035 Zbl 0621.57001
- [Ghys2001] E. Ghys, Groups acting on the circle, Enseign. Math. (2) 47 (2001), no.3-4, 329–407. MR1876932 (2003a:37032) Zbl 1044.37033
- [Moor,1920] R.L.Moore, Concerning simple continuous curves, Trans. Amer. Math. Soc. 21 (1920), 333—347.
- [Ward,1936] A.J.Ward, The topological characterization of an open linear interval, Proc. London Math. Soc.(2) 41 (1936), 191-198.
7 External links
- The Encylopedia of Mathematics article on one-dimensional manifolds.
- The Encylopedia of Mathematics article on lines.
- The Wikipedia page about curves.