Talk:Middle-dimensional surgery kernel (Ex)
From Manifold Atlas
Revision as of 11:20, 3 April 2012 by Fabian Hebestreit (Talk | contribs)
First a little lemma: \\
Let 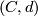 be a chain complex with
be a chain complex with 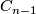 projective,
projective, 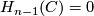 and
and 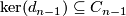 a direct summand. Then also
a direct summand. Then also 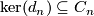 is a direct summand. \\
Proof:
Note that
is a direct summand. \\
Proof:
Note that 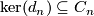 is a direct summand iff the sequence
is a direct summand iff the sequence
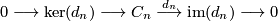
splits. By exactness at  however, we have
however, we have 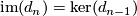 which is projective being a direct summand of a projective module.
which is projective being a direct summand of a projective module.
ad(1): Iterating the lemma we find that 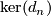 is a direct summand of
is a direct summand of 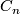 if the same statement holds for some lower
if the same statement holds for some lower  . However eventually both terms are zero, since the complex is finite. Being a direct summand in a finitely generated module
. However eventually both terms are zero, since the complex is finite. Being a direct summand in a finitely generated module 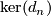 is then itself finitely generated, and hence also
is then itself finitely generated, and hence also 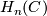 . The second assertion follows immediately from the universal coefficient theorem.
. The second assertion follows immediately from the universal coefficient theorem.