Surgery obstruction groups
|
The user responsible for this page is Hambleton. No other user may edit this page at present. |
|
This page has not been refereed. The information given here might be incomplete or provisional. |
1 Introduction
The surgery obstruction groups  of C.T.C. Wall [Wall1999], [Wall1976] contain the obstructions to doing surgery on a degree 1 normal map
of C.T.C. Wall [Wall1999], [Wall1976] contain the obstructions to doing surgery on a degree 1 normal map 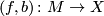 to obtain a homotopy equivalence. In this setting,
to obtain a homotopy equivalence. In this setting,  is an
is an  -dimensional Poincar\'e complex
-dimensional Poincar\'e complex 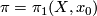 is the fundamental group of
is the fundamental group of  , and
, and 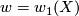 is the first Stiefel-Whitney class.
is the first Stiefel-Whitney class.
The groups 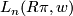 depend on a coefficient ring
depend on a coefficient ring  , a discrete group
, a discrete group  and an orientation character
and an orientation character 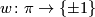 . In general the surgery obstruction groups are abelian groups. For finite groups
. In general the surgery obstruction groups are abelian groups. For finite groups  the
the  -groups are finitely-generated and the only torsion is
-groups are finitely-generated and the only torsion is  -primary.
-primary.
A Guide to the Calculation of Surgery Obstruction Groups, Hambleton & Taylor (2000), pp. 1-3
On the classification of hermitian forms: VI, Wall (1976)
2 References
- [Wall1976] C. T. C. Wall, Classification of Hermitian Forms. VI. Group rings, Ann. of Math. (2) 103 (1976), no.1, 1–80. MR0432737 (55 #5720) Zbl 0328.18006
- [Wall1999] C. T. C. Wall, Surgery on compact manifolds, American Mathematical Society, Providence, RI, 1999. MR1687388 (2000a:57089) Zbl 0935.57003