Thickenings
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
[edit] 1 Introduction
Let  be a finite connected CW-complex of dimension
be a finite connected CW-complex of dimension  . For a given
. For a given 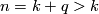 we would like to know if there is a compact manifold
we would like to know if there is a compact manifold  with boundary
with boundary 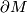 such that:
such that:
- the map
 is an isomorphism,
is an isomorphism,
-
 is homotopy equivalent to
is homotopy equivalent to  .
.
In this case we say that  thickens
thickens  . If there is such a manifold
. If there is such a manifold  , we would like to know how many up to homeomorphism or diffeomorphism if
, we would like to know how many up to homeomorphism or diffeomorphism if  is smooth.
is smooth.
In [Wall1966a] Wall introduced the notion of a thickening, defined below, to investigate the questions raised above. This page summarizes the basic results concerning thickenings.
Recall that 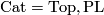 or
or 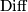 denotes respectively the topological, piecewise linear or smooth categories.
denotes respectively the topological, piecewise linear or smooth categories.
Definition 1.1 [Wall1966a, Section 1].
Let  be a finite connected CW complex. An
be a finite connected CW complex. An  -dimensional
-dimensional 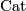 -thickening of
-thickening of  consists of
consists of
- a compact
 -dimensional
-dimensional 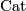 -manifold
-manifold  with connected boundary such that
with connected boundary such that 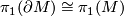
- a basepoint
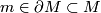 and an orientation of
and an orientation of 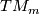 ,
,
- a simple homotopy equivalence
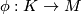 .
.
Two thickenings 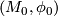 and
and  are isomorphic if there is a
are isomorphic if there is a 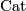 -isomorphism
-isomorphism 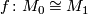 preserving
preserving  and the orientation of
and the orientation of 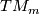 and such that
and such that  is simple homotopic to
is simple homotopic to 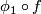 . In particular there is a simple homotopy commutative diagram:
. In particular there is a simple homotopy commutative diagram:
![\displaystyle \xymatrix{ M_0 \ar[dr]^{\phi_0} \ar[0,2]^{f} & & M_1 \ar[dl]_{\phi_1} \\ & K}](/images/math/e/7/b/e7b93f90388fbe391b8ba5e78d5a0abe.png)
The set of isomorphism classes of  -dimensional
-dimensional 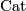 -thickenings over
-thickenings over  is denoted
is denoted
![\displaystyle \mathcal{T}^n(K) := \{ [\phi: K \simeq M ] \}.](/images/math/6/f/2/6f2f2839b8992a717559a381a9424f72.png)
[edit] 2 Constructions and examples
The simplest examples of thickenings come from  -disc bundles with sections over manifolds,
-disc bundles with sections over manifolds, 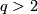 . Let
. Let  be a closed
be a closed 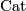 -manifold of dimension
-manifold of dimension  , let
, let 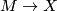 be a bundle with fibre
be a bundle with fibre 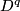 and with section
and with section  . Then
. Then
- the pair
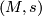 is an
is an  -thickening of
-thickening of  .
.
- the pair
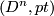 is an
is an  -thickening of a point.
-thickening of a point.
[edit] 3 Invariants
An important invariant of a thickening 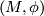 is the induced stable
is the induced stable 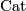 -bundle over
-bundle over  :
:
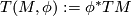
where 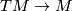 is the stable
is the stable 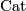 -tangent bundle of
-tangent bundle of  . Given that stable bundles over the space
. Given that stable bundles over the space  are classified by maps to the classifying space
are classified by maps to the classifying space 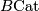 one equivalently thinks of
one equivalently thinks of
![\displaystyle T(M, \phi) = [f_{TM} \circ \phi]](/images/math/0/2/8/02809eaecaaddf353ed870a4aad4f046.png)
where 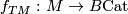 is the classifying map. Clearly the isomorphism class of the bundle
is the classifying map. Clearly the isomorphism class of the bundle 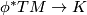 is an invariant of the thickening class
is an invariant of the thickening class ![[M, \phi]](/images/math/c/f/6/cf6dee16cb943b8e253b829fd155e4d0.png) . This if
. This if  denotes the set of isomorphism classes of stable
denotes the set of isomorphism classes of stable 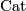 -bundles over
-bundles over  we obtain a map
we obtain a map
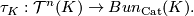
[edit] 4 Classification
An extremely useful classification theorem in manifold theory is the classification of stable thickenings where 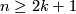 originally due to Wall in the smooth catagory.
originally due to Wall in the smooth catagory.
Theorem 4.1 [Wall1966a, Proposition 5.1] [Chazin1971, Theorem 1].
For all 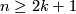 , the stable classifying map gives rise to a set bijection
, the stable classifying map gives rise to a set bijection
![\displaystyle \tau_K : \mathcal{T}^n(K) \equiv [K, B\Cat], \quad [M, \phi] \mapsto T(M, \phi) = [\phi \circ f_{TM}].](/images/math/1/8/d/18def848b04339f88703c6eb63e0310c.png)
[edit] 5 References
- [Chazin1971] R. L. Chazin, Stable thickenings in the topological category, Proc. Amer. Math. Soc. 29 (1971), 175–178. MR0296950 (45 #6009) Zbl 0214.22302
- [Wall1966a] C. T. C. Wall, Classification problems in differential topology. IV. Thickenings, Topology 5 (1966), 73–94. MR0192509 (33 #734) Zbl 0149.20501