Talk:Representing homology classes by embedded 2-spheres (Ex)
1. Let  . In the previous exercise, we showed that the intersection form
. In the previous exercise, we showed that the intersection form  Of
Of  is equivalent to
is equivalent to 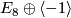 (using e.g. the classification of nondefinite even symmetric forms by rank and signature). Pick a basis
(using e.g. the classification of nondefinite even symmetric forms by rank and signature). Pick a basis 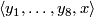 Of
Of 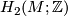 so that the intersection form of the
so that the intersection form of the 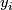 is
is 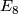 ,
, 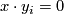 for each
for each  , and
, and 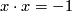 . Then
. Then  cannot be represented by a smoothly embedded
cannot be represented by a smoothly embedded  -sphere, or else blowing down the
-sphere, or else blowing down the  -sphere would yield a smooth structure on a
-sphere would yield a smooth structure on a  -manifold with intersection form
-manifold with intersection form 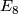 (violating Donaldson’s theorem).
(violating Donaldson’s theorem).
2. Let 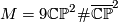 . Similarly, the intersection form
. Similarly, the intersection form 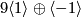 is equivalent to
is equivalent to 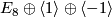 . As in Part 1, let
. As in Part 1, let 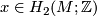 with
with 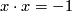 represent the
represent the  of this decomposition. Then
of this decomposition. Then  cannot be represented by a smoothly embedded
cannot be represented by a smoothly embedded  -sphere, or else blowing down the
-sphere, or else blowing down the  -sphere would yield a smooth structure of a
-sphere would yield a smooth structure of a  -manifold with intersection form
-manifold with intersection form 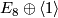 (again violating Donaldson’s theorem; note that
(again violating Donaldson’s theorem; note that 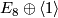 is still positive-definite and non-diagonalizeable).
is still positive-definite and non-diagonalizeable).
3. Let 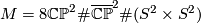 . The intersection form of
. The intersection form of  is
is 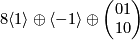 , which similarly is equivalent to
, which similarly is equivalent to 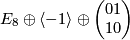 . As in parts 1 and 2, let
. As in parts 1 and 2, let 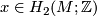 with
with 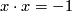 represent the
represent the 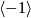 of this decomposition. Then
of this decomposition. Then  cannot be represented by a smoothly embedded
cannot be represented by a smoothly embedded  -sphere, or else blowing down this sphere would yield a smooth manifold with intersection form
-sphere, or else blowing down this sphere would yield a smooth manifold with intersection form 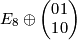 (violating Rokhlin’s theorem, as the signature of this form is
(violating Rokhlin’s theorem, as the signature of this form is  ).
).