Poincaré's homology sphere
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 09:34, 13 May 2013 and the changes since publication. |
|
The user responsible for this page is Klaus Volkert. No other user may edit this page at present. |
1 Introduction
Poincaré's homology sphere is a closed 3- manifold with the same homology as the 3-sphere but with a fundamental group which is non-trivial.
In his series of papers on Analysis situs (1892 - 1904) Poincaré introduced the fundamental group and studied Betti-numbers and torsion coefficients. He asked himself the question how strong these invariants are. In his last paper - the so-called fifth complement (1904) - he constructed an example of a closed manifold with vanishing first Betti-number, without torsion coefficient but with a fundamental group which he proved to be non trivial. This manifold today called Poincaré's homology sphere - or not correctly Poincaré's dodecahedron space (cf. below) - is constructed by a Heegaard splitting. Therefore Poincaré was the first author to use this idea in a sophisticated situation. Using a function which measures the "height" of the cuts through the 3-manifold (that is a sort of Morse function (in modern terms)) Poincaré described the process of attaching handles step by step, thus building up a handlebody. He arrived at a decomposition of the 3-manifold into two handle bodies; their surfaces are two homeomorphic surfaces of genus  which are identified by an automorphism of the surface.
which are identified by an automorphism of the surface.
To obtain his example Poincaré used two surfaces of genus 2 - two ``pretzels´´. The identifying automorphism is defined by sending curves which are not null homotopic of one surface to such curves on the other. The first set of curves contains the standard generators of the fundamental group of the pretzel, the second is indicated by a diagram. By using a sort of Seifert-van Kampen argument, Poincaré was able to show that the fundamental group of the manifold is non-trivial. In order to get this result he demonstrated that the fundamental group contains a subgroup isomorphic to the icosahedron group (actually the fundamental group is isomorphic to the extended icosahedron group). He was also able to calculate the Betti-numbers of his manifold. By using the relations on the generators of the fundamental group he showed that the first Betti number is 0 - so, by duality, the second is also zero. There are no torsion coefficients.
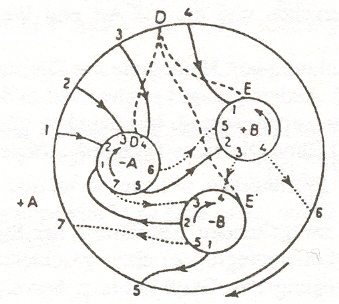
This picture in Figure 1 represents a sphere with two handles, a pretzel, with the handles cut off by two closed curve represented by the four circles + and -
and - , +
, + and -
and - (identifying +
(identifying + with -
with - yields the first handle, identifying +
yields the first handle, identifying + to -
to - the other). The pretzel has to be identified with a second surface of this type such that the two curves indicated in the scheme are identified with those closed curves which are commonly used to dissect the handles of the pretzel (``Meridianschnitte´´, that is +
the other). The pretzel has to be identified with a second surface of this type such that the two curves indicated in the scheme are identified with those closed curves which are commonly used to dissect the handles of the pretzel (``Meridianschnitte´´, that is + and -
and - , +
, + and -
and - ). Using this description Poincarè was able to calculate the fundamental group of the resulting closed 3-manifold. He showed that there is a homomorphism from the fundamental group onto the icosahedron group. So the first is definitely not trivial. Poincaré used the following presentation of the fundamental group.
). Using this description Poincarè was able to calculate the fundamental group of the resulting closed 3-manifold. He showed that there is a homomorphism from the fundamental group onto the icosahedron group. So the first is definitely not trivial. Poincaré used the following presentation of the fundamental group.
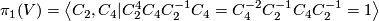
Later on Poincaré was able to demonstrate that the fundamental group is identical to its commutator subgroup (in modern terms). Poincaré discovered this fact by using generators and relations, so to him the assertion above meant that in calculating in a commutative way homology becomes trivial - i.e. the first Betti number of the manifold vanishes. Because the manifold is orientable there is no torsion coefficient in dimension one. Using duality Poincaré got all the information he needed.
At the end of his paper Poincaré asked a question: ``Est-il possible que le groupe fondamental de V se réduise à la substitution identique, et que pourtant V ne soit pas simplement connexe?´´ In modern terms this means: ``Is it possible that a manifold with a vanishing fundamental group is not homeomorphic to the 3-sphere?´´ And he ended with the remark: ``Mais cette question nous entraînerait trop loin.´´ (``But this question leads us too far away´´). This is the starting point of the history of the so-called Poincaré conjecture.
In their joint paper Analysis situs (1907), which was a contribution to the Encyklopädie der mathematischen Wissenschaften mit Einschluß ihrer Anwendungen, P. Heegard and M. Dehn discussed Poincaré's manifold. They gave a picture representing Poincaré's identification scheme in a different way: Dehn and Heegard used a ``Doppelringfläche´´ (that is a pretzel surface) instead of Poincaré's sphere with four holes. Once again the identification was defined by curves.
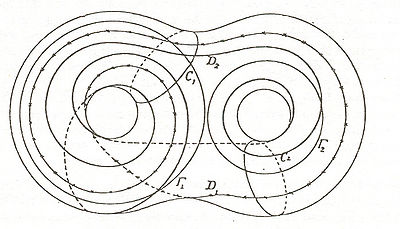
After this paper was printed Dehn noticed that the identification scheme indicated by the picture (cf. Figure 2) is not correct. In 1907 he published a ``rectification´´ (that is an erratum) of it. In reading this carefully one becomes aware of the fact that Dehn's famous method - today called Dehn's surgery - started with the analysis of the false identification scheme. In his paper of 1910, Dehn used his method to construct another example of a closed 3-manifold with a fundamental group isomorphic to the extended or binary icosahedron group, that is the full symmetry group of the icosahedron including also reflections. The ``Gruppenbild´´, that is the graph of this group, is depicted by Dehn as a dodecahedron net; see Figure 3. His starting point in doing this was the "Kleeblattschinge" (that is the torus knot (3,2); cf. [Epple1999]). Dehn coined the name ``Poincaréscher Raum´´ (Poincaré space) in order to denote ``solche dreidimensionalen Mannigfaltigkeiten, die, ohne Torsion und mit einfachem Zusammenhang, doch nicht mit dem gewöhnlichen Raum [gemeint ist die 3-Sphäre] homöomorph sind.´´ ([Dehn1910, 138]). That is, Poincaré space is the name of three-dimensional homology spheres which are not isomorphic to the 3-sphere. The example using the torus knot is unique because it is the only Poincaré space constructed by Dehn with a finite fundamental group.
During the 1920s Poincaré's conjecture became a well known problem. In particular H. Kneser mentioned it in a talk he delivered to the Versammlung Deutscher Naturforscher und Ärzte (joint meeting with the DMV) in 1928; [Kneser1929]. After citing Dehn's manifold, Kneser stated that this example is also related to the 120-cell (one of the six regular polytopes of 4-space) in ordinary 4-space. The 120-cell defines a tessellation of the 3-sphere on which the icosahedron group acts transitively and without fixed points. The fundamental domain is a spheric dodecahedron, the operations define certain identifications of the faces of the fundamental domain.
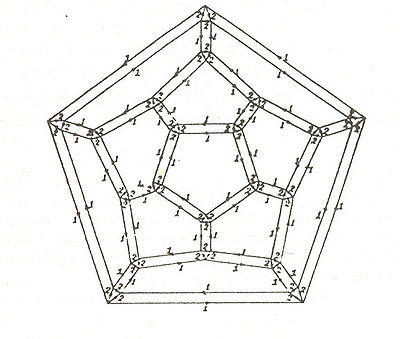
The now common way in which the dodecahedron space is defined was first given by W. Threlfall and H. Seifert in their first joint paper (1931). It is characterized by the use of a dodecahedron the opposite faces of which are identified after a turn by  . to be more precise this is the spherical dodecahedron space. There is also an hyperbolic dodecahedron space which is a different 3-manifold. The dodecahedron is called spherical because it is bounded by spherical pentagons due to the fact that it is obtained as the intersection of 12 balls. This construction is discussed in detail in [Threlfall&Seifert1931, 64-66].
. to be more precise this is the spherical dodecahedron space. There is also an hyperbolic dodecahedron space which is a different 3-manifold. The dodecahedron is called spherical because it is bounded by spherical pentagons due to the fact that it is obtained as the intersection of 12 balls. This construction is discussed in detail in [Threlfall&Seifert1931, 64-66].
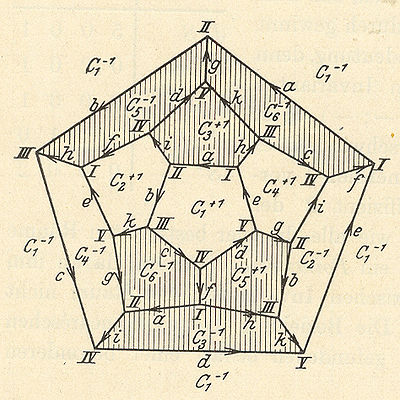
In this scheme the decomposition of the dodecahedron space into two solid pretzels is indicated.
Another representation of a homology sphere was given by the Russian mathematician M. Kreines in 1932. Generalizing the way in which lens spaces are defined he obtained the following scheme.
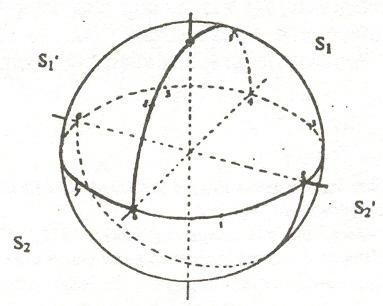
 has to identified with
has to identified with  such that the points 0123498 (pictured in small print in Figure 5) are identified with the points 8076549 in the indicated order,
such that the points 0123498 (pictured in small print in Figure 5) are identified with the points 8076549 in the indicated order,  with
with 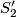 , such that the points 01267 are identified with the points 32654. Using this description it is possible to get a decomposition of the manifold into cells and to calculate its fundamental group. There are two cycles
, such that the points 01267 are identified with the points 32654. Using this description it is possible to get a decomposition of the manifold into cells and to calculate its fundamental group. There are two cycles  and
and  - the two edges in the decomposition - and two relations given by
- the two edges in the decomposition - and two relations given by  and
and 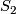 :
: 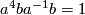
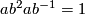

Around 1932 different homology spheres were known with a fundamental group isomorphic to the icosahedron group (or to an extension of it): Poincaré's original example, Dehn's manifold, Kreines' manifold and the dodecahedron space of Seifert and Threlfall. The obvious question was: Are they all homeomorphic? The positive answer was given by the theory of fibered spaces (today: Seifert fibered spaces) developed by Seifert in his paper of 1933 [Seifert1933b]; the detailed discussion of the different manifolds just cited can be found in a joint paper written by H. Seifert and C. Weber in 1933, [Weber&Seifert1933] (cf. also [Seifert&Threlfall1934, n. 38 (p. 224)]). The clue to this result is a complete list of invariants found by Seifert which solved the problem of homeomorphy for certain types of Seifert-fibered spaces.
2 References
- [Dehn&Heegaard1907] M. Dehn and P. Heegaard, Analysis situs, Enzyklopädie der Mathematischen Wissenschaften III AB3: (1907) 153–200. Zbl 38.0510.14
- [Dehn1910] M. Dehn, Über die Topologie des dreidimensionalen Raumes, Math. Ann. 69 (1910), no.1, 137–168. MR1511580 () Zbl 41.0543.01
- [Epple1999] M. Epple, Die Entstehung der Knotentheorie, Braunschweig/Wiesbaden, (1999).
- [Kneser1929] H. Kneser, Geschlossene Flächen in dreidimensionalen Mannigfaltigkeiten, Jahresbericht D. M. V. 38 (1929), 248-260. Zbl 55.0311.03
- [Kreines1932] M. Kreines, Zur Konstruktion der Poincaré-Räume, Rend. Circ. Mat. Palermo 56 (1932), 277-280. Zbl 0005.41705
- [Poincaré1904] H. Poincaré, Cinquième complément à l'analysis situs, Palermo Rend. 18 (1904), 45-110. Zbl 35.0504.13
- [Seifert&Threlfall1934] H. Seifert and W. Threlfall, Lehrbuch der Topologie, Leipzig und Berlin: B. G. Teubner. VII, 1934. Zbl 0009.08601
- [Seifert1933b] H. Seifert, Topologie Dreidimensionaler Gefaserter Räume, Acta Math. 60 (1933), no.1, 147–238. MR1555366 () Zbl 0006.08304
- [Threlfall&Seifert1931] W. Threlfall and H. Seifert, Topologische Untersuchung der Diskontinuitätsbereiche endlicher Bewegungsgruppen des dreidimensionalen sphärischen Raumes, Math. Ann. 104 (1931), no.1, 1–70. MR1512649 Zbl 0006.03403
- [Weber&Seifert1933] C. Weber and H. Seifert, Die beiden Dodekaederräume, Math. Z. 37 (1933), no.1, 237–253. MR1545392 Zbl 0007.02806