Manifold Atlas:Structure of a Manifolds page
The Manifold Atlas emphasises constructions and examples of manifolds. We suggest, but do not insist, that an article in the Manifolds chapter has the following structure:
Introduction Construction and examples Invariants Classification/Characterization (if available) Further discussion References
Contents |
1 Introduction
Briefly orient the reader to manifolds to be discussed: ideally specify the default category being used. This section may be omitted.
2 Construction and examples
Present the construction(s) of the manifolds of intereset. Following that give examples of manifolds falling under the construction(s).
3 Invariants
List and where necessary describe invariants of intereset. Where possible, record the known values of these invariants. Here are some simple suggestions for invariants.
- (Co)Homology groups and rings.
- Homotopy groups.
- Euler characteristic.
- Characteristic classes.
- Orientability.
4 Classification and/or characterisation
- Report what is known concerning completeness of the invariants listed for this class of manifolds or manifold.
- Statements here will typically depend upon the category chosen: the more classification results over more diverse categories, the better.
5 Further topics
These could include
- Open problems related to this collection of manifolds: ideally write a Problems page and link to it.
- Occurences of the manifolds in or other branches of mathematics.
- Existence and properties of further structures.
- Curvature and metric properties.
- Embedding/immersions into
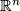 .
.
- Mapping class groups.
- Chirality.
- Group actions or other interesting self-maps.
6 Categories
To enable searching for manifolds with given properties, manifolds pages will contain links to categories. For example, an article describing an orientable manifold should contain the following link at the end of the article.
[[Category:orientable]]