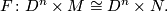Tangential homotopy equivalences (Ex)
(Difference between revisions)
(Created page with "<wikitex>; A homotopy equivalence $f \colon M \to N$ of closed smooth $n$-manifolds is called ''tangential'' if $f^*TN \cong TM$; i.e. $f$ pulls back the tangent bundle of $N$...") |
m |
||
| Line 2: | Line 2: | ||
A homotopy equivalence $f \colon M \to N$ of closed smooth $n$-manifolds is called ''tangential'' if $f^*TN \cong TM$; i.e. $f$ pulls back the tangent bundle of $N$ to $M$. | A homotopy equivalence $f \colon M \to N$ of closed smooth $n$-manifolds is called ''tangential'' if $f^*TN \cong TM$; i.e. $f$ pulls back the tangent bundle of $N$ to $M$. | ||
{{beginthm|Example}} | {{beginthm|Example}} | ||
| − | # | + | # Give an example of a tangential homotopy equivalence which is not homotopic to a diffeomorphism. |
# Show that if $f \colon M \to N$ is a tangential homotopy equivelance, then there is a diffeomorphism | # Show that if $f \colon M \to N$ is a tangential homotopy equivelance, then there is a diffeomorphism | ||
$$ F \colon D^n \times M \cong D^n \times N.$$ | $$ F \colon D^n \times M \cong D^n \times N.$$ | ||
Revision as of 23:50, 25 August 2013
A homotopy equivalence 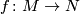 of closed smooth
of closed smooth  -manifolds is called tangential if
-manifolds is called tangential if 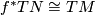 ; i.e.
; i.e.  pulls back the tangent bundle of
pulls back the tangent bundle of  to
to  .
.
Example 0.1.
- Give an example of a tangential homotopy equivalence which is not homotopic to a diffeomorphism.
- Show that if
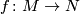 is a tangential homotopy equivelance, then there is a diffeomorphism
is a tangential homotopy equivelance, then there is a diffeomorphism