Prime decomposition theorem in high dimensions
m |
CarmenRovi (Talk | contribs) (→Problem) |
||
| Line 11: | Line 11: | ||
Nevertheless, the decomposition is not unique. | Nevertheless, the decomposition is not unique. | ||
| − | In | + | In {{cite|Kreck&Lück&Teichner1994}} it is proven that a $4$-dimensional stable version of Kneser's conjecture on the splitting of three-manifolds as connected sums. The result clearly doesn't work non-stably in dimension $4$ and this paper gives some counterexamples. Another counterexample to the uniqueness of the decomposition is as follows. |
{{beginthm|Example|}} | {{beginthm|Example|}} | ||
$\mathbb{C}P^2$ has a homotopy equivalent twin $\star \mathbb{C}P^2$. The following decompositions provide a counterexample to uniqueness. | $\mathbb{C}P^2$ has a homotopy equivalent twin $\star \mathbb{C}P^2$. The following decompositions provide a counterexample to uniqueness. | ||
| Line 17: | Line 17: | ||
{{endthm}} | {{endthm}} | ||
</wikitex> | </wikitex> | ||
| + | |||
== References == | == References == | ||
{{#RefList:}} | {{#RefList:}} | ||
Revision as of 06:53, 8 January 2019
1 Problem
Every closed topological oriented manifold  has a prime decomposition
has a prime decomposition
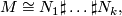
where the  are prime manifolds.
Recall that a manifold is prime if for any decomposition as a connected
sum
are prime manifolds.
Recall that a manifold is prime if for any decomposition as a connected
sum 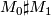 one of the summands
one of the summands  or
or  is homeomorphic to
is homeomorphic to  .
.
For  -manifolds, it was shown in [Milnor1962a] that the decomposition is unique.
For high-dimensional manifolds, there is no notion of prime decomposition of smooth manifolds, but there is a notion of prime decomposition for topological manifolds.
Nevertheless, the decomposition is not unique.
-manifolds, it was shown in [Milnor1962a] that the decomposition is unique.
For high-dimensional manifolds, there is no notion of prime decomposition of smooth manifolds, but there is a notion of prime decomposition for topological manifolds.
Nevertheless, the decomposition is not unique.
In [Kreck&Lück&Teichner1994] it is proven that a  -dimensional stable version of Kneser's conjecture on the splitting of three-manifolds as connected sums. The result clearly doesn't work non-stably in dimension
-dimensional stable version of Kneser's conjecture on the splitting of three-manifolds as connected sums. The result clearly doesn't work non-stably in dimension  and this paper gives some counterexamples. Another counterexample to the uniqueness of the decomposition is as follows.
and this paper gives some counterexamples. Another counterexample to the uniqueness of the decomposition is as follows.
Example 1.1.
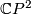 has a homotopy equivalent twin
has a homotopy equivalent twin 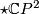 . The following decompositions provide a counterexample to uniqueness.
. The following decompositions provide a counterexample to uniqueness.
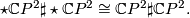
2 References
- [Kreck&Lück&Teichner1994] M. Kreck, W. Lück and P. Teichner, Stable prime decompositions of four-manifolds, Prospects in topology, Princeton Univ. Press, Princeton, NJ (1995), 251–269. MR1368662 Zbl 0928.57019
- [Milnor1962a] J. Milnor, A unique decomposition theorem for
 -manifolds, Amer. J. Math. 84 (1962), 1–7. MR0142125 Zbl 0108.36501
-manifolds, Amer. J. Math. 84 (1962), 1–7. MR0142125 Zbl 0108.36501