Petrie conjecture
| Line 8: | Line 8: | ||
Suppose that $M$ is a closed smooth manifold homotopy equivalent to $\CP^n$ and that $S^1$ acts smoothly and non-trivially on $M$. Then the total Pontrjagin class $p(M)$ of $M$ agrees with that of $\CP^n$, i.e., for a generator $x \in H^2(M; \mathbb{Z})$, $$p(M) = (1+x^2)^{n+1}.$$ | Suppose that $M$ is a closed smooth manifold homotopy equivalent to $\CP^n$ and that $S^1$ acts smoothly and non-trivially on $M$. Then the total Pontrjagin class $p(M)$ of $M$ agrees with that of $\CP^n$, i.e., for a generator $x \in H^2(M; \mathbb{Z})$, $$p(M) = (1+x^2)^{n+1}.$$ | ||
{{endthm}} | {{endthm}} | ||
| − | |||
The Petrie conjecture has not been confirmed in general (no counterexample is know at the moment). However, if $\dim M \leq 8$, the total Pontrjagin class of $M$ agrees with that of $\CP^n$ | The Petrie conjecture has not been confirmed in general (no counterexample is know at the moment). However, if $\dim M \leq 8$, the total Pontrjagin class of $M$ agrees with that of $\CP^n$ | ||
| Line 20: | Line 19: | ||
[[Category:Problems]] | [[Category:Problems]] | ||
[[Category:Group actions on manifolds]] | [[Category:Group actions on manifolds]] | ||
| + | </wikitex> | ||
Revision as of 23:43, 30 November 2010
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Introduction
The Petrie conjecture was formulated in the following context: suppose that a Lie group  acts smoothly on a closed smooth manifold
acts smoothly on a closed smooth manifold  , what constraints does this place on the topology of
, what constraints does this place on the topology of  in general and on the Pontrjagin classes of
in general and on the Pontrjagin classes of  in particular.
in particular.
Petrie restricted his attention to smooth actions of the Lie group  [Petrie1972] (or more generally, the torus
[Petrie1972] (or more generally, the torus 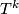 for
for 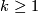 [Petrie1973]) on closed smooth manifolds
[Petrie1973]) on closed smooth manifolds  which are homotopy equivalent to
which are homotopy equivalent to 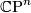 . He has formulated the following conjecture.
. He has formulated the following conjecture.
Conjecture 0.1 [Petrie1972].
Suppose that is a closed smooth manifold homotopy equivalent to
is a closed smooth manifold homotopy equivalent to 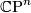 and that
and that  acts smoothly and non-trivially on
acts smoothly and non-trivially on  . Then the total Pontrjagin class
. Then the total Pontrjagin class 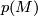 of
of  agrees with that of
agrees with that of 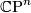 , i.e., for a generator
, i.e., for a generator 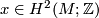 ,
, 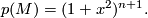
The Petrie conjecture has not been confirmed in general (no counterexample is know at the moment). However, if  , the total Pontrjagin class of
, the total Pontrjagin class of  agrees with that of
agrees with that of 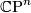 by the work of [Dejter1976] and [James1978].
by the work of [Dejter1976] and [James1978].
[Iberkleid1978], [Tsukada&Washiyama1979], [Dessai2002],[Dessai&Wilking2004], and [Tolman2010].
References
- [Dejter1976] I. J. Dejter, Smooth
 -manifolds in the homotopy type of
-manifolds in the homotopy type of  , Michigan Math. J. 23 (1976), no.1, 83–95. MR0402789 (53 #6603) Zbl 0326.57009
, Michigan Math. J. 23 (1976), no.1, 83–95. MR0402789 (53 #6603) Zbl 0326.57009
- [Dessai&Wilking2004] A. Dessai and B. Wilking, Torus actions on homotopy complex projective spaces, Math. Z. 247 (2004), no.3, 505–511. MR2114425 (2006c:57033) Zbl 1068.57034
- [Dessai2002] A. Dessai, Homotopy complex projective spaces with
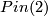 -action, Topology Appl. 122 (2002), no.3, 487–499. MR1911696 (2003f:58048) Zbl 0998.57048
-action, Topology Appl. 122 (2002), no.3, 487–499. MR1911696 (2003f:58048) Zbl 0998.57048
- [Iberkleid1978] W. Iberkleid, Pseudolinear spheres, Michigan Math. J. 25 (1978), no.3, 359–370. MR512906 (80d:57023) Zbl 0377.57008
- [James1978] Template:James1978
- [Petrie1972] T. Petrie, Smooth
 -actions on homotopy complex projective spaces and related topics, Bull. Amer. Math. Soc. 78 (1972), 105–153. MR0296970 Zbl 0247.57010
-actions on homotopy complex projective spaces and related topics, Bull. Amer. Math. Soc. 78 (1972), 105–153. MR0296970 Zbl 0247.57010
- [Petrie1973] T. Petrie, Torus actions on homotopy complex projective spaces, Invent. Math. 20 (1973), 139–146. MR0322893 (48 #1254) Zbl 0262.57021
- [Tolman2010] S. Tolman, On a symplectic generalization of Petrie's conjecture, Trans. Amer. Math. Soc. 362 (2010), no.8, 3963–3996. MR2638879 Zbl 1216.53074
- [Tsukada&Washiyama1979] E. Tsukada and R. Washiyama,
 -actions on cohomology complex projective spaces with three components of the fixed point sets, Hiroshima Math. J. 9 (1979), no.1, 41–46. MR529325 (80j:57043) Zbl 0411.57037
-actions on cohomology complex projective spaces with three components of the fixed point sets, Hiroshima Math. J. 9 (1979), no.1, 41–46. MR529325 (80j:57043) Zbl 0411.57037