Exotic spheres and chirality (Ex)

Latest revision as of 09:22, 1 April 2012

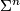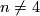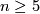Recall that $<wikitex>; Recall that $\Theta_n$ denotes the group of h-cobordism classes of manifolds $\Sigma^n$ which are homotopy equivalent to the $n$-sphere. For $n <> 4$ this is the same as the group of oriented diffeomorphism classes of such manifolds. {{beginthm|Exercise}} Let $\Sigma$ a homotopy $n$-sphere with $n \geq 5$. Show that $\Sigma$ admits an orientation reversing diffeomorphism if and only if $\Sigma$ defines and element of order two in $\Theta_n$. As a consequence, show that the boundary of the 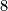$-dimensional [[E_8-manifold]] does not admit an orientation reversing diffeomorphism. {{endthm}} {{beginrem|Remark}} Note that manifolds admitting no orientation reversing diffeomorphism are often called [[Chirality|chiral]]. {{endrem}} </wikitex> == References == {{#RefList:}} [[Category:Exercises]]\Theta_n$ denotes the group of h-cobordism classes of manifolds $\Sigma^n$ which are homotopy equivalent to the $n$-sphere. For $n \neq 4$ this is the same as the group of oriented diffeomorphism classes of such manifolds.