Exotic spheres
(→External references) |
(→Plumbing) |
||
| Line 35: | Line 35: | ||
As special case of the following construction goes back at least to {{cite|Milnor1959}} | As special case of the following construction goes back at least to {{cite|Milnor1959}} | ||
| − | Let $i \in \{1, \dots, n\}$, let $(p_i, q_i)$ be pairs of positive integers such that $p_i + q_i + 2 = n$ and let $\alpha_i \in \pi_{p_i}(SO(q_i+1))$ be the clutching functions of $D^{q_i+1}$-bundles $D(\alpha_i) | + | Let $i \in \{1, \dots, n\}$, let $(p_i, q_i)$ be pairs of positive integers such that $p_i + q_i + 2 = n$ and let $\alpha_i \in \pi_{p_i}(SO(q_i+1))$ be the clutching functions of $D^{q_i+1}$-bundles over $S^{p_i + 1}$ |
| − | + | $$ D^{q_i+1} \to D(\alpha_i) \to S^{p_i+1}.$$ | |
| − | If $G$ is simply connected then $\Sigma(G, \{\alpha_i \}) : = \partial W$ is often a homotopy sphere. | + | Let $G$ be a graph with vertices $\{v_1, \dots, v_n\}$ such that the edge set between $v_i$ and $v_j$, is non-empty only if $p_i = q_j$. We form the manifold $W = W(G;\{\alpha_i\})$ from the disjoint union of the $D(\alpha_i)$ by identifying $D^{p_i+1} \times D^{q_i+1}$ and $D^{q_j+1} \times D^{p_j+1}$ for each edge in $G$. If $G$ is simply connected then |
| − | + | $$\Sigma(G, \{\alpha_i \}) : = \partial W$$ | |
| − | We establish some notation for bundles | + | is often a homotopy sphere. We establish some notation for bundles, graphs and maps: |
* let $\tau_{n} \in \pi_{n-1}(SO(n))$ denote the tangent bundle of the $n$-sphere, | * let $\tau_{n} \in \pi_{n-1}(SO(n))$ denote the tangent bundle of the $n$-sphere, | ||
* let $\gamma_{4s-1}^k \in \pi_{4s-1}(SO(k)) \cong \Zz$, $k > 4s$, denote a generator, | * let $\gamma_{4s-1}^k \in \pi_{4s-1}(SO(k)) \cong \Zz$, $k > 4s$, denote a generator, | ||
* let $E_8$ denote the $E_8$-graph, | * let $E_8$ denote the $E_8$-graph, | ||
| − | * let $T$ denote the graph with two vertices and one edge connecting them. | + | * let $T$ denote the graph with two vertices and one edge connecting them, |
| + | * let $\eta_n : S^{n+1} \to S^n$ be essential. | ||
| − | + | Then we have the following exotic spheres. | |
* $\Sigma^{4k-1}(E_8; \{\tau_{2k}, \dots \tau_{2k}\})$, the Milnor sphere, generates $bP_{4k}$, $k>1$. | * $\Sigma^{4k-1}(E_8; \{\tau_{2k}, \dots \tau_{2k}\})$, the Milnor sphere, generates $bP_{4k}$, $k>1$. | ||
| Line 51: | Line 52: | ||
* $\Sigma^{4k+1}(T; \{\tau_{2k+1}, \tau_{2k+1}\})$, the Kervaire sphere, generates $bP_{4k+2}$. | * $\Sigma^{4k+1}(T; \{\tau_{2k+1}, \tau_{2k+1}\})$, the Kervaire sphere, generates $bP_{4k+2}$. | ||
| − | * $\Sigma^{16}(T; \{\gamma_{7}^9, \eta_7\tau_8\})$, generates $\Theta_{16} = \Zz_2$. | + | * $\Sigma(T; \{\gamma_3^5, \eta_3\tau_4\})$, generates $\Theta_8 = \Zz_2$. |
| + | * $\Sigma^{16}(T; \{\gamma_{7}^9, \eta_7\tau_8\})$, generates $\Theta_{16} = \Zz_2$. | ||
| + | |||
</wikitex> | </wikitex> | ||
Revision as of 16:22, 21 November 2009
Contents |
1 Introduction
Let 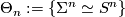 denote the set of oriented diffeomorphism classes of closed, smooth n-manifolds homotopy equivalent to
denote the set of oriented diffeomorphism classes of closed, smooth n-manifolds homotopy equivalent to  .
.
2 Construction and examples
Exotic spheres may be constructed in a variety of ways.
2.1 Brieskorn varieties
2.2 Sphere bundles
The first known examples of exotic spheres were discovered by Milnor in [Milnor1956]. They are the total spaces of certain 3-sphere bundles over the 4-sphere as we now explain: the group $\pi_3(SO(4)) \cong \Zz \oplus \Zz$ parametrises linear $3$-sphere bundles over $S^4$ ...
A little later Shimada [Shimada1957] used similar techniques to show that the total spaces of certain 7-sphere bundles over the 8-sphere are exotic 15-spheres.
By Adams' solution of the Hopf-invariant 1 problem, [Adams1960], dimensions n = 1, 3, 7 and 15 are the only dimensions where an n-sphere can be fibre over an m-sphere for m<n. </wikitex>
2.3 Plumbing
As special case of the following construction goes back at least to [Milnor1959]
Let 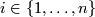 , let
, let 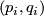 be pairs of positive integers such that
be pairs of positive integers such that 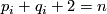 and let
and let 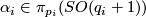 be the clutching functions of
be the clutching functions of  -bundles over
-bundles over 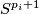
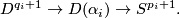
Let  be a graph with vertices
be a graph with vertices  such that the edge set between
such that the edge set between 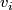 and
and 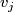 , is non-empty only if
, is non-empty only if 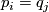 . We form the manifold
. We form the manifold 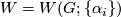 from the disjoint union of the
from the disjoint union of the 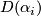 by identifying
by identifying  and
and 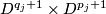 for each edge in
for each edge in  . If
. If  is simply connected then
is simply connected then
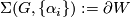
is often a homotopy sphere. We establish some notation for bundles, graphs and maps:
- let
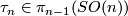 denote the tangent bundle of the
denote the tangent bundle of the  -sphere,
-sphere,
- let
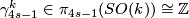 ,
, 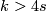 , denote a generator,
, denote a generator,
- let
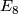 denote the
denote the 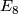 -graph,
-graph,
- let
 denote the graph with two vertices and one edge connecting them,
denote the graph with two vertices and one edge connecting them,
- let
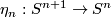 be essential.
be essential.
Then we have the following exotic spheres.
-
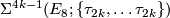 , the Milnor sphere, generates
, the Milnor sphere, generates 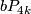 ,
,  .
.
-
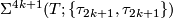 , the Kervaire sphere, generates
, the Kervaire sphere, generates 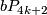 .
.
-
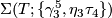 , generates
, generates 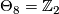 .
.
-
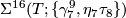 , generates
, generates  .
.
2.4 Twisting
By [Cerf1970] and [Smale1962a] there is an isomorphism 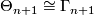 for
for 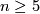 where
where 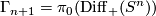 is the group of isotopy classes of orientation preserving diffeomorphisms of
is the group of isotopy classes of orientation preserving diffeomorphisms of  . The map is given by
. The map is given by
![\displaystyle \Gamma_{n+1} \to \Theta_{n+1}, ~~~~~[f] \longmapsto D^{n+1} \cup_f (-D^{n+1}).](/images/math/e/7/b/e7befcfe5bad19cfcc0035d5527a4fe8.png)
Hence one may construct exotic (n+1)-spheres by describing diffeomorphisms of  which are not isotopic to the identity.
which are not isotopic to the identity.
3 Invariants
Signature, Kervaire invaiant,  -invariant, Eels-Kuiper invariant,
-invariant, Eels-Kuiper invariant,  -invariant.
-invariant.
4 Classification
[Kervaire&Milnor1963], [Levine1983]
5 Further discussion
... is welcome
6 External references
- Wikipedia article on exotic spheres
- http://www.maths.ed.ac.uk/~aar/exotic.htm Andrew Ranicki's exotic sphere home page, with many of the original papers.
7 References
- [Adams1960] J. F. Adams, On the non-existence of elements of Hopf invariant one, Ann. of Math. (2) 72 (1960), 20–104. MR0141119 (25 #4530) Zbl 0096.17404
- [Cerf1970] J. Cerf, La stratification naturelle des espaces de fonctions différentiables réelles et le théorème de la pseudo-isotopie, Inst. Hautes Études Sci. Publ. Math. (1970), no.39, 5–173. MR0292089 (45 #1176) Zbl 0213.25202
- [Kervaire&Milnor1963] M. A. Kervaire and J. W. Milnor, Groups of homotopy spheres. I, Ann. of Math. (2) 77 (1963), 504–537. MR0148075 (26 #5584) Zbl 0115.40505
- [Levine1983] J. P. Levine, Lectures on groups of homotopy spheres, Algebraic and geometric topology (New Brunswick, N.J., 1983), Lecture Notes in Math., 1126 (1983), 62–95. MR802786 (87i:57031) Zbl 0576.57028
- [Milnor1956] J. Milnor, On manifolds homeomorphic to the
 -sphere, Ann. of Math. (2) 64 (1956), 399–405. MR0082103 (18,498d) Zbl 0072.18402
-sphere, Ann. of Math. (2) 64 (1956), 399–405. MR0082103 (18,498d) Zbl 0072.18402
- [Milnor1959] J. Milnor, Differentiable structures on spheres, Amer. J. Math. 81 (1959), 962–972. MR0110107 (22 #990) Zbl 0111.35501
- [Shimada1957] N. Shimada, Differentiable structures on the 15-sphere and Pontrjagin classes of certain manifolds, Nagoya Math. J. 12 (1957), 59–69. MR0096223 (20 #2715) Zbl 0145.20303
- [Smale1962a] S. Smale, On the structure of manifolds, Amer. J. Math. 84 (1962), 387–399. MR0153022 (27 #2991) Zbl 0109.41103
|
This page has not been refereed. The information given here might be incomplete or provisional. |