Elementary matricies (Ex)
(Difference between revisions)
m |
m |
||
| Line 1: | Line 1: | ||
<wikitex>; | <wikitex>; | ||
| − | Let $R$ be an associative ring with unit and recall that an ''elementary matrix'' over $R$ is a square matrix of the form | + | Let $R$ be an associative ring with unit and recall that an ''elementary matrix'', $E$, over $R$ is a square matrix of the form |
$$ E = \text{Id}_n + a e_{ij} $$ | $$ E = \text{Id}_n + a e_{ij} $$ | ||
where $\text{Id}_n$ is the $n \times n$ identity matrix, $a \in R$ and $e_{ij}$ is the matrix with zeros in all places except $(i, j)$ where $i \neq j$. Clearly each elementary matrix if invertible and so gives and element $E \in GL(R)$ where | where $\text{Id}_n$ is the $n \times n$ identity matrix, $a \in R$ and $e_{ij}$ is the matrix with zeros in all places except $(i, j)$ where $i \neq j$. Clearly each elementary matrix if invertible and so gives and element $E \in GL(R)$ where | ||
Revision as of 18:58, 23 March 2012
Let  be an associative ring with unit and recall that an elementary matrix,
be an associative ring with unit and recall that an elementary matrix,  , over
, over  is a square matrix of the form
is a square matrix of the form
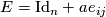
where 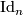 is the
is the 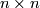 identity matrix,
identity matrix, 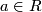 and
and 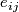 is the matrix with zeros in all places except
is the matrix with zeros in all places except 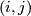 where
where 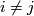 . Clearly each elementary matrix if invertible and so gives and element
. Clearly each elementary matrix if invertible and so gives and element 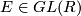 where
where
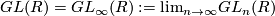
is the limit of the invertible matricies.
Exercise 0.1.
Prove that ![E(R)=[GL(R),GL(R)]](/images/math/a/2/a/a2ac3f81c640b9978b9ad187c7af1875.png) , where
, where 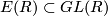 is the subgroup generated by all elements in
is the subgroup generated by all elements in  which are represented by elementary matrices.
which are represented by elementary matrices.
Hint 0.2.
For 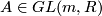 and
and 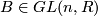 write the matrix
write the matrix
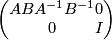
as a product of elementary matrices
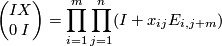
where 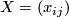 is an
is an 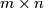 matrix.
matrix.
Recall that 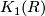 is defined to be the abelian group
is defined to be the abelian group
![\displaystyle K_1(R) : = GL(R)_{ab} = GL(R)/[GL(R), GL(R)].](/images/math/a/5/0/a50f6109d26038e574733fb869dbd3a6.png)
Exercise 0.3.
Prove that 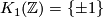 .
.