Normal maps and submanifolds (Ex)

Let $(f, b) \colon (M, \nu_M) \to (X, \xi)$$\newcommand{\Z}{\mathbb{Z}} \newcommand{\R}{\mathbb{R}} \newcommand{\C}{\mathbb{C}} \newcommand{\N}{\mathbb{N}} \newcommand{\Q}{\mathbb{Q}} \newcommand{\F}{\mathbb{F}} \newcommand{\bZ}{\mathbb{Z}} \newcommand{\bR}{\mathbb{R}} \newcommand{\bC}{\mathbb{C}} \newcommand{\bH}{\mathbb{H}} \newcommand{\bQ}{\mathbb{Q}} \newcommand{\bF}{\mathbb{F}} \newcommand{\bN}{\mathbb{N}} \DeclareMathOperator\id{id} % identity map \DeclareMathOperator\Sq{Sq} % Steenrod squares \DeclareMathOperator\Homeo{Homeo} % group of homeomorphisms of a topoloical space \DeclareMathOperator\Diff{Diff} % group of diffeomorphisms of a smooth manifold \DeclareMathOperator\SDiff{SDiff} % diffeomorphism under some constraint \DeclareMathOperator\Hom{Hom} % homomrphism group \DeclareMathOperator\End{End} % endomorphism group \DeclareMathOperator\Aut{Aut} % automorphism group \DeclareMathOperator\Inn{Inn} % inner automorphisms \DeclareMathOperator\Out{Out} % outer automorphism group \DeclareMathOperator\vol{vol} % volume \newcommand{\GL}{\text{GL}} % general linear group \newcommand{\SL}{\text{SL}} % special linear group \newcommand{\SO}{\text{SO}} % special orthogonal group \newcommand{\O}{\text{O}} % orthogonal group \newcommand{\SU}{\text{SU}} % special unitary group \newcommand{\Spin}{\text{Spin}} % Spin group \newcommand{\RP}{\Rr\mathrm P} % real projective space \newcommand{\CP}{\Cc\mathrm P} % complex projective space \newcommand{\HP}{\Hh\mathrm P} % quaternionic projective space \newcommand{\Top}{\mathrm{Top}} % topological category \newcommand{\PL}{\mathrm{PL}} % piecewise linear category \newcommand{\Cat}{\mathrm{Cat}} % any category \newcommand{\KS}{\text{KS}} % Kirby-Siebenmann class \newcommand{\Hud}{\text{Hud}} % Hudson torus \newcommand{\Ker}{\text{Ker}} % Kernel \newcommand{\underbar}{\underline} %Classifying Spaces for Families of Subgroups \newcommand{\textup}{\text} \newcommand{\sp}{^}(f, b) \colon (M, \nu_M) \to (X, \xi)$ 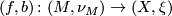be a degree one normal map. 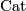For simplicity, assume that $M$$M$ and $X$$X$ are closed oriented $\text{Cat}$$\text{Cat}$-manifolds of dimension $n$$n$. 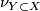Suppose that $Y \subset X$$Y \subset X$ is a codimension $k$$k$ oriented submanifold $X$$X$ with normal bundle $\nu_{Y \subset X}$$\nu_{Y \subset X}$ and that that $f$$f$ is transverse to $Y$$Y$. Prove the following:

1. There is a canonical degree one normal map $(f|_N, b') \colon (N, \nu) \to (Y, \xi|_Y \oplus \nu_{Y \subset X})$$(f|_N, b') \colon (N, \nu) \to (Y, \xi|_Y \oplus \nu_{Y \subset X})$.
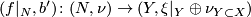2. This defines a well-defined map $\pitchfork_Y \colon \mathcal{N}(X,\xi)\rightarrow \mathcal{N}(Y,\xi|_Y \oplus \nu_{Y \subset X})$$\pitchfork_Y \colon \mathcal{N}(X,\xi)\rightarrow \mathcal{N}(Y,\xi|_Y \oplus \nu_{Y \subset X})$.

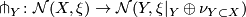Of course we have the surgery obstruction map

$\displaystyle \sigma \colon \mathcal{N}(Y, \xi_y \oplus \nu_{Y \subset X}) \to L_{n-k}(\pi_1(Y))$ $$\displaystyle \sigma \colon \mathcal{N}(Y, \xi_y \oplus \nu_{Y \subset X}) \to L_{n-k}(\pi_1(Y))$$

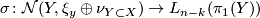
and the composite map

$\displaystyle \sigma \circ \pitchfork_Y \colon \mathcal{N}(X, \xi) \to L_{n-k}(\pi_1(Y))$ $$\displaystyle \sigma \circ \pitchfork_Y \colon \mathcal{N}(X, \xi) \to L_{n-k}(\pi_1(Y))$$

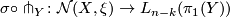
which is called the splitting obstruction map along $Y$$Y$. In addition prove the following:

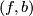1. If $(f, b)$$(f, b)$ is normally bordant to a homeomorphism then the splitting obstruction along $Y$$Y$ vanishes.