Super manifolds: an incomplete survey
|
An earlier version of this page was published in the Bulletin of the Manifold Atlas: screen, print. You may view the version used for publication as of 09:56, 1 April 2011 and the changes since publication. |
|
By Henning Hohnhold, Stephan Stolz and Peter Teichner. This page is not open for editing at present. |
We present an incomplete survey on some basic notions of super manifolds which may serve as a short introduction to this subject. Almost all the material is taken from the beautiful survey article on super manifolds [Deligne&Morgan1999]. Standard references also include [Leites1980], [Bernstein1987], [Manin1988] or [Voronov1991]. The material below is a prerequisite to our papers [Hohnhold&Kreck&Stolz&Teichner2010] and [Stolz&Teichner2008].
Contents |
1 Super Algebra
Let us begin by explaining briefly what super means in an algebraic context, working with the ground field of real numbers. The monoidal category of super vector spaces, with tensor products, is by definition the same as the monoidal category of  -graded vector spaces, with the graded tensor product. As a consequence, a super algebra is simply a monoidal object in this category and is hence the same thing as a
-graded vector spaces, with the graded tensor product. As a consequence, a super algebra is simply a monoidal object in this category and is hence the same thing as a  -graded algebra. For example, the endomorphism ring
-graded algebra. For example, the endomorphism ring 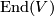 of a super vector space
of a super vector space  inherits a natural
inherits a natural  -grading from that of
-grading from that of  . The distinction between these notions only arises from the choice of symmetry operators
. The distinction between these notions only arises from the choice of symmetry operators
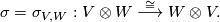
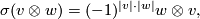
 is the
is the  -degree of a homogenous vector
-degree of a homogenous vector 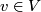 . For
. For  -graded vector spaces the signs would be omitted. This basic difference is sometimes summarized as the
-graded vector spaces the signs would be omitted. This basic difference is sometimes summarized as the
 Sign rule: Commuting two odd quantities yields a sign
Sign rule: Commuting two odd quantities yields a sign  .
.
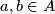 we have
we have 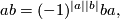
 -graded algebra. The standard examples of commutative super algebras are the exterior algebras
-graded algebra. The standard examples of commutative super algebras are the exterior algebras 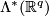 . As we shall see, the generators of
. As we shall see, the generators of 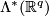 yield the so-called odd coordinates on super manifolds; these anti-commute and hence are useful when trying to describe physical systems involving Fermions. Super algebras also arise naturally in algebraic topology: for every space
yield the so-called odd coordinates on super manifolds; these anti-commute and hence are useful when trying to describe physical systems involving Fermions. Super algebras also arise naturally in algebraic topology: for every space  , the cohomology ring
, the cohomology ring  is a commutative super algebra.
is a commutative super algebra.
Let  be a commutative super algebra. The derivations of
be a commutative super algebra. The derivations of  are endomorphisms
are endomorphisms 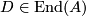 satisfying the Leibniz rule: [1]
satisfying the Leibniz rule: [1]
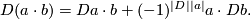
 is a super Lie algebra with respect to the bracket operation
is a super Lie algebra with respect to the bracket operation ![\displaystyle [D,E] := DE - (-1)^{|D| |E|}ED](/images/math/0/5/d/05d369cb2c98547e63aea1d945b32e5c.png)
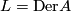 .
.
 together with a Lie bracket
together with a Lie bracket ![[\cdot, \cdot]: L\otimes L\to L](/images/math/6/1/5/615ad7472d37b5dff1fb18d6b71dbc62.png) that is skew symmetric
that is skew symmetric ![\displaystyle [ D,E ] + (-1)^{|D| |E|} [ E,D ] = 0](/images/math/6/c/1/6c1f534b9f85a41f1abe28df4b859fc4.png)
![\displaystyle [D, [ E,F ]] + (-1)^{|D| (|E| + |F|)} [ E, [F,D ]] + (-1)^{|F| (|D| + |E|)} [ F, [ D,E ]] = 0.](/images/math/f/5/9/f595128d405d34d21d951cb31b74b3c6.png)
Note that we cyclically permuted the 3 symbols and put down the signs according to the above sign rule.
2 Super Manifolds
We will define super manifolds as ringed spaces following [Deligne&Morgan1999]. By a morphism we will always mean a map of ringed spaces. The local model for a super manifold of dimension 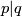 is
is  equipped with the sheaf
equipped with the sheaf  of commutative super
of commutative super  -algebras
-algebras  .
.
 of dimension
of dimension 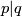 is a pair
is a pair 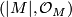 consisting of a (Hausdorff and second countable) topological space
consisting of a (Hausdorff and second countable) topological space 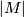 together with a sheaf of commutative super
together with a sheaf of commutative super  -algebras
-algebras 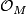 that is locally isomorphic to
that is locally isomorphic to 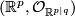 . A morphism
. A morphism 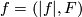 between super manifolds
between super manifolds 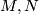 is defined to be a continuous map
is defined to be a continuous map  , together with a map
, together with a map  of sheaves covering
of sheaves covering 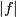 . More precisely, for every open subset
. More precisely, for every open subset 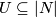 there are algebra maps
there are algebra maps 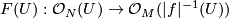
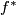 for
for  and we denote this category of super manifolds by
and we denote this category of super manifolds by 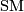 .
.
 there is an associated reduced manifold
there is an associated reduced manifold 
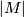 and there is an inclusion of super manifolds
and there is an inclusion of super manifolds 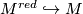 . Note that the sheaf of ideals
. Note that the sheaf of ideals 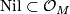 is generated by odd functions. Other geometric super objects can be defined in a similar way. For example, replacing
is generated by odd functions. Other geometric super objects can be defined in a similar way. For example, replacing  by
by 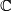 and
and 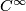 by analytic functions one obtains complex (analytic) super manifolds. There is also an important notion of cs manifolds. These are spaces equipped with sheaves of commutative super
by analytic functions one obtains complex (analytic) super manifolds. There is also an important notion of cs manifolds. These are spaces equipped with sheaves of commutative super 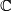 -algebras that locally look like
-algebras that locally look like 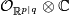 . One relevance of cs manifolds is that they appear naturally as the smooth super manifolds underlying complex analytic super manifolds. In our work, cs manifolds are essential to define the notion of a unitary field theory but this is not relevant for the current discussion.
. One relevance of cs manifolds is that they appear naturally as the smooth super manifolds underlying complex analytic super manifolds. In our work, cs manifolds are essential to define the notion of a unitary field theory but this is not relevant for the current discussion.
Example 2.2.
Let  be a real vector bundle of fiber dimension
be a real vector bundle of fiber dimension  over the ordinary manifold
over the ordinary manifold 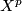 and
and  the associated algebra bundle of alternating multilinear forms on
the associated algebra bundle of alternating multilinear forms on  . Then its sheaf of sections gives a super manifold
. Then its sheaf of sections gives a super manifold 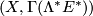 of dimension
of dimension 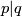 , denoted by
, denoted by 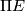 . In the current smooth setting, Marjorie Batchelor proved in [Batchelor1979] that every super manifold is isomorphic to one of this type (this is not true for analytic super manifolds). More precisely, let
. In the current smooth setting, Marjorie Batchelor proved in [Batchelor1979] that every super manifold is isomorphic to one of this type (this is not true for analytic super manifolds). More precisely, let 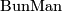 denote the category of real vector bundles over smooth manifolds, and for
denote the category of real vector bundles over smooth manifolds, and for 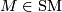 , consider the vector bundle
, consider the vector bundle  over
over 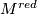 with sheaf of sections
with sheaf of sections 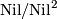 . Then the functors
. Then the functors
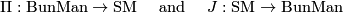
come equipped with natural isomorphisms 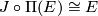 but there are only non-natural isomorphisms
but there are only non-natural isomorphisms 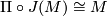 , coming from a choice of a partition of unity. In other words, these functors induce a bijection on isomorphism classes of objects and inclusions on morphisms but they are not equivalences of categories because there are many more morphims in
, coming from a choice of a partition of unity. In other words, these functors induce a bijection on isomorphism classes of objects and inclusions on morphisms but they are not equivalences of categories because there are many more morphims in 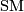 than the linear bundle maps coming from
than the linear bundle maps coming from 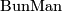 .
.
The following proposition gives two extremely useful ways of looking at morphisms between super manifolds. We shall use the notation 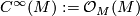 for the algebra of (global) functions on a super manifold
for the algebra of (global) functions on a super manifold  .
.
Proposition 2.3.
For 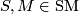 , the functor
, the functor 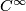 induces natural bijections
induces natural bijections
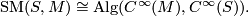
If  is an open super submanifold (a
is an open super submanifold (a 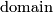 ),
), 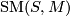 is in bijective correspondence with those
is in bijective correspondence with those 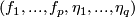 in
in 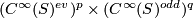 that satisfy
that satisfy

 ,
, 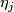 are called the coordinates of
are called the coordinates of 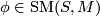 defined by
defined by 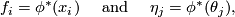
where 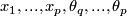 are coordinates on
are coordinates on  . Moreover, by the first part we see that
. Moreover, by the first part we see that 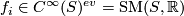 and hence
and hence 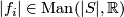 .
.
The proof of the first part is based on the existence of partitions of unity for super manifolds, so it is false in analytic settings. The second part always holds and is proved in [Leites1980].
3 The Functor of Points
 -points, i.e. instead of
-points, i.e. instead of  itself one considers the morphism sets
itself one considers the morphism sets 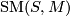 , where
, where  varies over all super manifolds
varies over all super manifolds  . More formally, embed the category
. More formally, embed the category 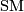 of super manifolds in the category of contravariant functors from
of super manifolds in the category of contravariant functors from 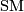 to
to Tex syntax errorby
Tex syntax error
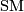 with the the category of representable functors, defined to be those in the image of
with the the category of representable functors, defined to be those in the image of  . We will sometimes refer to an arbitrary functor
. We will sometimes refer to an arbitrary functor Tex syntax erroras a generalized super manifold. Note that Proposition 2.3 makes it easy to describe the morphism sets
Tex syntax error. We'd also like to point out that the functor of points approach is closely related to computations involving additional odd quantities (the odd coordinates of
 as opposed to those of
as opposed to those of  ) in many physics papers.
) in many physics papers.
4 Super Lie Groups
These are simply group objects in 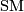 . According to the functor of points approach, such a group object in
. According to the functor of points approach, such a group object in 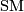 can be described by giving a functor
can be described by giving a functor 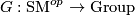 such that the composition with the forgetful functor
such that the composition with the forgetful functor 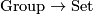 is representable.
is representable.
Example 4.1.
The simplest super Lie group is the additive group structure on 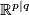 . It is given by the following composition law on
. It is given by the following composition law on 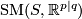 , obviously natural in
, obviously natural in  :
:
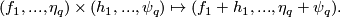
The super general linear group 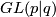 is defined by
is defined by
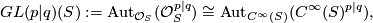
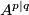 denotes the
denotes the  -module freely generated by
-module freely generated by  even and
even and  odd generators. We need to check that this is representable. We claim that
odd generators. We need to check that this is representable. We claim that 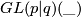 is represented by the open super submanifold
is represented by the open super submanifold 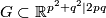 characterized by
characterized by 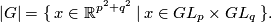
This follows directly from proposition 2.3 using that a map between super algebras is invertible if and only if it is invertible modulo nilpotent elements.
5 Super Vector Bundles
A (super) vector bundle over a super manifold  is a locally free sheaf
is a locally free sheaf 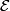 of
of 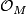 -modules of dimension
-modules of dimension 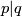 . The most basic example of a super vector bundle is the tangent bundle of a super manifold
. The most basic example of a super vector bundle is the tangent bundle of a super manifold 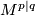 . It is the sheaf of
. It is the sheaf of 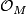 -modules
-modules 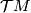 defined by
defined by
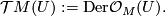
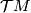 is locally free of dimension
is locally free of dimension 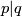 : If
: If  are local coordinates on
are local coordinates on  , then a local basis is given by
, then a local basis is given by  . Note that there is also a linear fibre bundle
. Note that there is also a linear fibre bundle 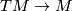 with structure group
with structure group 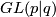 , where
, where  is a super manifold of dimension
is a super manifold of dimension  . More generally, any vector bundle
. More generally, any vector bundle 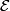 over
over  has a total space
has a total space 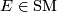 that comes with a projection map
that comes with a projection map 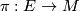 . It can be most easily described in terms of its
. It can be most easily described in terms of its  -points
-points
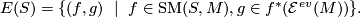
So  is an even global section of the pullback bundle on
is an even global section of the pullback bundle on  and the projection
and the projection  comes from forgetting this datum. To prove that this functor
comes from forgetting this datum. To prove that this functor 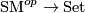 is representable one uses the local triviality of
is representable one uses the local triviality of 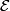 and Proposition 2.3. It follows by construction that the typical fibre of the projection
and Proposition 2.3. It follows by construction that the typical fibre of the projection  is
is 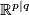 and the structure group is
and the structure group is 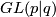 .
.
 . It is an involution
. It is an involution 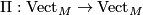
 with grading involution
with grading involution  to
to 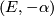 . This means that even and odd parts are exchanged. To define
. This means that even and odd parts are exchanged. To define 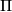 on morphisms it is easiest to give it as
on morphisms it is easiest to give it as 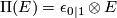 , where
, where 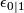 is the trivial bundle of dimension
is the trivial bundle of dimension  (aka the constant sheaf of free
(aka the constant sheaf of free 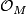 -modules).
-modules).
One can define the super Lie algebra 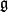 of a super Lie group
of a super Lie group  as follows. A vector field
as follows. A vector field 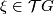 is called left-invariant if
is called left-invariant if  is related to itself under the left-translation by all
is related to itself under the left-translation by all  :
:
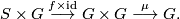
Here we interpret  as a vertical vector field on
as a vertical vector field on 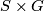 in the obvious way. The super Lie algebra
in the obvious way. The super Lie algebra 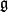 consists of all left-invariant vector fields on
consists of all left-invariant vector fields on  . Pulling back via the unit
. Pulling back via the unit 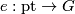 defines an isomorphism
defines an isomorphism 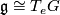 , in particular, the vector space dimension of
, in particular, the vector space dimension of 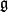 is
is 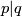 .
.
6 Footnotes
- ↑ Whenever we write formulas involving the degree
 of certain elements, we implicitly assume that these elements are homogenous.
of certain elements, we implicitly assume that these elements are homogenous.
7 References
- [Batchelor1979] M. Batchelor, The structure of supermanifolds, Trans. Amer. Math. Soc. 253 (1979), 329–338. MR536951 (80h:58002) Zbl 0413.58002
- [Bernstein1987] Template:Bernstein1987
- [Deligne&Morgan1999] P. Deligne and J. W. Morgan, Notes on supersymmetry (following Joseph Bernstein), Quantum fields and strings: a course for mathematicians, Vol. 1, 2 (Princeton, NJ, 1996/1997), 41–97, Amer. Math. Soc., Providence, RI, (1999), 41–97. MR1701597 (2001g:58007) Zbl 1170.58302
- [Hohnhold&Kreck&Stolz&Teichner2010] H. Hohnhold, M. Kreck, S. Stolz and P. Teichner, Differential Forms and 0-dimensional Super Symmetric Field Theories, to appear in Quantum Topology, Journal of the European Math. Soc. (2010).
- [Leites1980] D. A. Leites, Introduction to the theory of supermanifolds, Russian Math. Surveys 35 No 1 (1980), 3–57. MR0565567 (81j:58003) Zbl 0439.58007
- [Manin1988] Y. I. Manin, Gauge field theory and complex geometry, Springer-Verlag, Berlin, 1988. MR954833 (89d:32001) Zbl 0884.53002
- [Stolz&Teichner2008] S. Stolz and P. Teichner, Supersymmetric Euclidean field theories and generalized cohomology, Survey (2008). Available at http://math.berkeley.edu/~teichner/Papers/Survey.pdf.
- [Voronov1991] T. Voronov, Geometric integration theory on supermanifolds, Harwood Academic Publishers, Chur, 1991. MR1202882 (95b:58023) Zbl 0839.58014
8 External links
- The Wikipedia page on Super manifolds.