Quadratic forms for surgery
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
Let 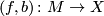 be a degree one normal map from a manifold of dimension
be a degree one normal map from a manifold of dimension 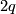 . Then the surgery kernel of
. Then the surgery kernel of 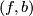 ,
,  , comes equipped with a subtle and crucial quadratic refinement. This page describes both the algebraic and geometric aspects of such quadratic refinements
, comes equipped with a subtle and crucial quadratic refinement. This page describes both the algebraic and geometric aspects of such quadratic refinements
2 Topology
2.1 The 4k+2 dimensional case
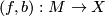
 -connected normal map,
-connected normal map, 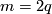 ,
,  odd and
odd and  . Assume also
. Assume also  is
is  -connected. Let
-connected. Let 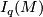
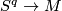 which represent elements of the surgery kernel
which represent elements of the surgery kernel  (with respect to the homomorphism
(with respect to the homomorphism 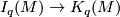 .
.
It is an abelian group using the connected summ operation (this uses the condition  ).
).
Then we have three invariants:
-
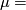 double point obstruction
double point obstruction  ,
,
-
 Browder's framing obstruction
Browder's framing obstruction  , and
, and
-
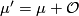 .
.
Tex syntax erroris defined. Each element of
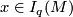 is represented by a commutative square
is represented by a commutative square
![\displaystyle \SelectTips{cm}{} \xymatrix{ S^q \ar[r]^\phi \ar[d] & M \ar[d]^{f}\\ D^{q+1} \ar[r] & X }](/images/math/6/5/4/6547c00a0ee15554d7bc5cf41ae3a917.png)
with  an immersion, and a diagram of normal bundle data
an immersion, and a diagram of normal bundle data
![\displaystyle \SelectTips{cm}{} \xymatrix{ S^q \ar[r]^{\nu_\phi} \ar[d] & B\text{O}_q \ar[d]^{f}\\ D^{q+1} \ar[r] & B\text{O} }](/images/math/1/4/d/14dcd20fe881205d067d6a82b1af71df.png)
the latter defining a stable trivialization of the normal bundle of  .
The homotopy class of the latter diagram defines an element of
.
The homotopy class of the latter diagram defines an element of 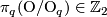 . This element
. This element
Tex syntax error.
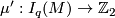
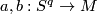 are immersions representing the same element
are immersions representing the same element 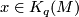 , then
, then 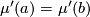 ).
).
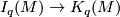 is onto and two-to-one. The distinct elements over a given
is onto and two-to-one. The distinct elements over a given 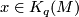 are detected by Browder's framing obstruction
are detected by Browder's framing obstruction Tex syntax error
(this uses Smale-Hirsch theory).
Let and
and  be immersions representing these elements. Then
be immersions representing these elements. Then  and
and  are not regularly homotopic. (Note: when
are not regularly homotopic. (Note: when  the normal bundles of
the normal bundles of  and
and  are distinct; when
are distinct; when  they are both trivial.) We can assume without loss in generality that
they are both trivial.) We can assume without loss in generality that Tex syntax error(so
Tex syntax error). Then
 is a framed immersion.
is a framed immersion.
- Case 1:
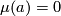 .
.
If 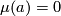 then a result of Whitney (the "Whitney trick") shows that
then a result of Whitney (the "Whitney trick") shows that  is regularly homotopic to a (framed) embedding, so assume that
is regularly homotopic to a (framed) embedding, so assume that  is a framed embedding. Whitney's method of introducing a single double point to
is a framed embedding. Whitney's method of introducing a single double point to  in a coordinate chart yields a new immersion
in a coordinate chart yields a new immersion 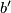 such that
such that 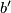 has one double point and
has one double point and 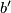 still represents
still represents  . Then
. Then 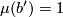 , so
, so 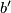 isn't regularly homotopic to
isn't regularly homotopic to  . It must therefore be regularly homotopic to
. It must therefore be regularly homotopic to  . Hence
. Hence 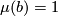 . It follows that
. It follows that 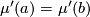 in this case.
in this case.
- Case 2:
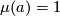 .
.
In this case  is regularly homotopic to an immersion with exactly one double point. By introducing another double point we get a
is regularly homotopic to an immersion with exactly one double point. By introducing another double point we get a 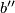 representing
representing  such that
such that 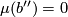 . Then
. Then 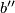 is not regularly homotopic to
is not regularly homotopic to  so it must be regularly homotopic to
so it must be regularly homotopic to  . Consequently,
. Consequently, 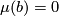 . Therefore
. Therefore 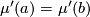 in this case.
in this case.
Let 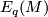 denote the isotopy classes of embeddings
denote the isotopy classes of embeddings 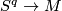 representing elements of
representing elements of  . Then we have a function
. Then we have a function 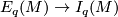 .
.
Tex syntax errorfactors through
 .
.