Symplectic manifolds
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
A symplectic manifold is a smooth manifold  together with a differential two-form
together with a differential two-form  that is nondegenerate and closed. The form
that is nondegenerate and closed. The form  is called a symplectic form. The nondegeneracy means that the highest nonzero power of
is called a symplectic form. The nondegeneracy means that the highest nonzero power of  is a volume form on
is a volume form on 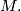 It follows that a symplectic manifold is even dimensional.
It follows that a symplectic manifold is even dimensional.
Symplectic manifolds originated from classical mechanics. The phase space of a dynamical system is the cotangent bundle of the configuration space and it is equipped with a symplectic form. This symplectic form is preserved by the flow of the system.
2 Examples
 The most basic example of a symplectic manifold is
The most basic example of a symplectic manifold is 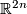 equipped with the form
equipped with the form
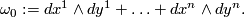
A theorem of Darboux [McDuff-Salamon] states that locally every
symplectic manifold if of this form. More precisely, if 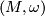 is a symplectic
is a symplectic  -manifold
then for every point
-manifold
then for every point 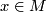 there exists an open neighbourhood
there exists an open neighbourhood 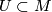 of
of  and a
diffeomorphism
and a
diffeomorphism 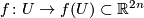 such that the restriction of
such that the restriction of  to
to  is equal to the pull-back
is equal to the pull-back 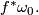 This implies that symplectic manifolds have
no local invariants.
This implies that symplectic manifolds have
no local invariants.
 An area form on an oriented surface is symplectic.
An area form on an oriented surface is symplectic.
 Let
Let  be a smooth manifold and let
be a smooth manifold and let  be a one-form on the cotangent bundle
be a one-form on the cotangent bundle 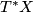 defined as follows.
If
defined as follows.
If  is a vector tangent to
is a vector tangent to 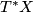 at a point
at a point  then
then 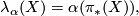 where
where
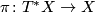 is the projection. In local coordinates the form
is the projection. In local coordinates the form  can be expressed as
can be expressed as
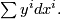 The differential
The differential 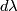 is a symplectic form on the cotangent bundle
is a symplectic form on the cotangent bundle 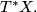
 If
If 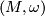 is a closed, i.e. compact and without boundary, symplectic
is a closed, i.e. compact and without boundary, symplectic  -manifold then the cohomology classes
-manifold then the cohomology classes
![[\omega]^k](/images/math/e/6/e/e6eff0918969467a4c56a4750f24fd36.png) are non-zero for
are non-zero for 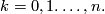 This follows from the fact that the cohomology class of the volume
form
This follows from the fact that the cohomology class of the volume
form 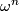 is nonzero on a closed manifold. This necessary condition implies that spheres of dimension greater than
two are not symplectic. More generally, no closed manifold of the form
is nonzero on a closed manifold. This necessary condition implies that spheres of dimension greater than
two are not symplectic. More generally, no closed manifold of the form 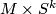 is symplectic for
is symplectic for 
3 Invariants
...
4 Classification/Characterization
...
5 Further discussion
...
6 References
= L_X\omega = d\iota_X \omega + \iota _X d\omega.$ Then the closedness of the symplectic form implies that the one-form $\iota_X\omega$ is closed. It follows that the Lie algebra of the group of symplectic diffeomorhism consists of the vector fields $X$ for which the one-form $\iota _X \omega$ is closed. Hence it can be identified with the space of closed one-forms. If the one-form $\iota _X \omega$ is exact, i.e. $\iota _X \omega = dH$ for some function $H\colon M\to \mathbb R$ then the vector field $X$ is called Hamiltonian. Symplectic diffeomorphism generated by Hamiltonian flows form a group $\operatorname{Ham}(M,\omega)$ called the group of Hamiltonian diffeomorphism. Its Lie algebra can be identified with the quotient of the space of smooth functions on $M$ by the constants. ==Constructions== that is nondegenerate and closed. The form
that is nondegenerate and closed. The form  is called a symplectic form. The nondegeneracy means that the highest nonzero power of
is called a symplectic form. The nondegeneracy means that the highest nonzero power of  is a volume form on
is a volume form on 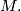 It follows that a symplectic manifold is even dimensional.
It follows that a symplectic manifold is even dimensional.
Symplectic manifolds originated from classical mechanics. The phase space of a dynamical system is the cotangent bundle of the configuration space and it is equipped with a symplectic form. This symplectic form is preserved by the flow of the system.
2 Examples
 The most basic example of a symplectic manifold is
The most basic example of a symplectic manifold is 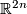 equipped with the form
equipped with the form
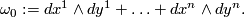
A theorem of Darboux [McDuff-Salamon] states that locally every
symplectic manifold if of this form. More precisely, if 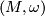 is a symplectic
is a symplectic  -manifold
then for every point
-manifold
then for every point 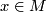 there exists an open neighbourhood
there exists an open neighbourhood 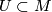 of
of  and a
diffeomorphism
and a
diffeomorphism 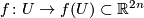 such that the restriction of
such that the restriction of  to
to  is equal to the pull-back
is equal to the pull-back 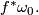 This implies that symplectic manifolds have
no local invariants.
This implies that symplectic manifolds have
no local invariants.
 An area form on an oriented surface is symplectic.
An area form on an oriented surface is symplectic.
 Let
Let  be a smooth manifold and let
be a smooth manifold and let  be a one-form on the cotangent bundle
be a one-form on the cotangent bundle 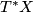 defined as follows.
If
defined as follows.
If  is a vector tangent to
is a vector tangent to 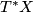 at a point
at a point  then
then 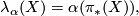 where
where
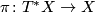 is the projection. In local coordinates the form
is the projection. In local coordinates the form  can be expressed as
can be expressed as
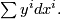 The differential
The differential 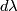 is a symplectic form on the cotangent bundle
is a symplectic form on the cotangent bundle 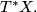
 If
If 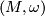 is a closed, i.e. compact and without boundary, symplectic
is a closed, i.e. compact and without boundary, symplectic  -manifold then the cohomology classes
-manifold then the cohomology classes
![[\omega]^k](/images/math/e/6/e/e6eff0918969467a4c56a4750f24fd36.png) are non-zero for
are non-zero for 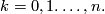 This follows from the fact that the cohomology class of the volume
form
This follows from the fact that the cohomology class of the volume
form 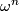 is nonzero on a closed manifold. This necessary condition implies that spheres of dimension greater than
two are not symplectic. More generally, no closed manifold of the form
is nonzero on a closed manifold. This necessary condition implies that spheres of dimension greater than
two are not symplectic. More generally, no closed manifold of the form 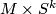 is symplectic for
is symplectic for 
3 Invariants
...
4 Classification/Characterization
...
5 Further discussion
...