Group actions on disks
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Topological actions
2 Smooth actions
2.1 Fixed point free
2.1.1 History
Floyd and Richardson [Floyd&Richardson1959] have constructed for the first time a smooth fixed point free action of  on a disk for
on a disk for 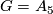 , the alternating group on five letters (see [Bredon1972, pp. 55-58] for a transparent description of the construction). Next, Greever [Greever1960] has described plenty of finite solvable groups
, the alternating group on five letters (see [Bredon1972, pp. 55-58] for a transparent description of the construction). Next, Greever [Greever1960] has described plenty of finite solvable groups  which can act smoothly on disks without fixed points. Then, Oliver [Oliver1975], [Oliver1976] has answered completely the question of which compact Lie groups admit smooth fixed point free actions on disks.
which can act smoothly on disks without fixed points. Then, Oliver [Oliver1975], [Oliver1976] has answered completely the question of which compact Lie groups admit smooth fixed point free actions on disks.
2.1.2 Oliver number
Let  be a finite group not of prime power order. Oliver [Oliver1975] has proven that the set
be a finite group not of prime power order. Oliver [Oliver1975] has proven that the set
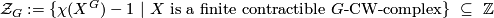
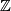 . Therefore,
. Therefore, 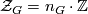 for a unique integer
for a unique integer 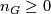 , which we refer to as the Oliver number of
, which we refer to as the Oliver number of  .
.
In the papers [Oliver1975], [Oliver1977], and [Oliver1978], Oliver has computed the integer 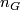 . In particular, the following lemma holds.
. In particular, the following lemma holds.
Lemma 2.1 (Oliver Lemma).
For a finite group  not of prime power order,
not of prime power order, 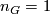 if and only if there does not exist a sequence
if and only if there does not exist a sequence 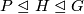 of normal subgroups such that
of normal subgroups such that  is a
is a  -group,
-group,  is a
is a  -group, and
-group, and 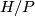 is cyclic for two (possibly the same) primes
is cyclic for two (possibly the same) primes  and
and  .
.
Moreover, the work [Oliver1977, Theorem 7] yields the following proposition.
Proposition 2.2.
For a finite nilpotent group  not of prime power order, the following conclusions hold:
not of prime power order, the following conclusions hold:
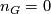 if
if  has at most one non-cyclic Sylow subgroup.
has at most one non-cyclic Sylow subgroup.
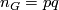 for two distinct primes
for two distinct primes  and
and  , if
, if  has just one non-cyclic
has just one non-cyclic  -Sylow and
-Sylow and  -Sylow subgroups.
-Sylow subgroups.
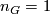 if
if  has three or more non-cyclic Sylow subgroups.
has three or more non-cyclic Sylow subgroups.
The notion of the Oliver number 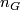 extends to compact Lie groups
extends to compact Lie groups  as follows.
as follows.
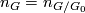 if
if 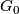 is abelian and
is abelian and 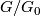 is not of prime power order.
is not of prime power order.
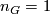 if
if 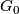 is non-abelian (see [Oliver1976]).
is non-abelian (see [Oliver1976]).
2.1.3 Oliver group
The notion of Oliver group has been introduced by Laitinen and Morimoto [Laitinen&Morimoto1998] in connection with the work on smooth one fixed point actions on spheres.
Definition 2.3.
A finite group  not of prime power order is called an Oliver group if
not of prime power order is called an Oliver group if 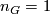 (cf. Oliver Lemma).
(cf. Oliver Lemma).
Examples of finite Oliver groups include:
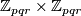 for three distinct primes
for three distinct primes  ,
,  , and
, and  .
.
- the solvable groups
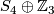 and
and 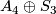 of order 72.
of order 72.
- all non-solvable groups, e.g.,
 and
and 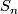 for
for 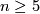 .
.
2.1.4 Results
The results of Oliver [Oliver1975], [Oliver1976] can be summarized as follows.
Theorem 2.4.
A compact Lie group  has a smooth fixed point free action on some disk if and only if the identity connected component
has a smooth fixed point free action on some disk if and only if the identity connected component 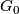 of
of  is non-abelian, or the quotient group
is non-abelian, or the quotient group 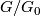 is not of prime power order and
is not of prime power order and 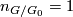 .
.
Theorem 2.5.
Let  be a compact Lie group such that the identity connected component
be a compact Lie group such that the identity connected component 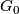 of
of  is non-abelian, or the quotient group
is non-abelian, or the quotient group 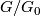 is not of prime power order. Let
is not of prime power order. Let  be a CW-complex. Then the following three statements are equivalent.
be a CW-complex. Then the following three statements are equivalent.
 is compact and the Euler-Poincaré characteristic
is compact and the Euler-Poincaré characteristic 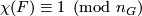 .
.
- There exists a finite contractible
 -CW-complex
-CW-complex  such that the fixed point set
such that the fixed point set 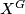 is homeomorphic to
is homeomorphic to  .
.
- There exists a smooth action of
 on a disk
on a disk 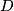 such that the fixed point set
such that the fixed point set 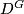 is homotopy equivalent to
is homotopy equivalent to  .
.
2.2 Fixed point sets
2.2.1 History
2.2.2 Definitions
For a compact space  , between the reduced real, complex, and quaternion
, between the reduced real, complex, and quaternion  -theory groups
-theory groups 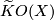 ,
, 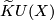 , and
, and 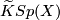 , respectively, consider
, respectively, consider
- the induction (complexification and quaternization) homomorphisms
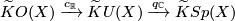
- and the forgetful (complexification and realification) homomorphisms
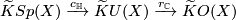 .
.
2.2.3 Results
Theorem 2.6 ([Oliver1996]).
Let  be a finite group not of prime power order. Let
be a finite group not of prime power order. Let  be a smooth manifold. Then there exists a smooth action of
be a smooth manifold. Then there exists a smooth action of  on some disk
on some disk 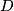 such that the fixed point
such that the fixed point 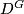 is diffeomorphic to
is diffeomorphic to  if and only if
if and only if  is compact,
is compact, 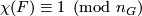 , and the class
, and the class ![[\tau_F]\in \widetilde{K}O(F)](/images/math/f/6/d/f6d05f50857ba929997be0651d9ddfef.png) satisfies the following condition depending on
satisfies the following condition depending on  .
.
- If
 , then there is no restriction on
, then there is no restriction on ![[\tau_F]](/images/math/4/3/c/43c708b9317aab9c266b5c48be3b4214.png) .
.
- If
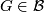 , then
, then ![c_{\mathbb{R}}([\tau_F])\in c_{\mathbb{H}}(\widetilde{K}Sp(F))+\text{Tor}(\widetilde{K}U(F))](/images/math/4/8/1/48189cb6a9ee102f372e1fc78b2f1b9f.png) .
.
- If
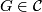 , then
, then ![[\tau_F]\in r_{\mathbb{C}}(\widetilde{K}U(F))+\text{Tor}(\widetilde{K}O(F))](/images/math/2/f/5/2f566d792f0f655f1be17a986eddb145.png) .
.
- If
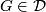 , then
, then ![[\tau_F]\in r_{\mathbb{C}}(\widetilde{K}U(F))](/images/math/c/c/3/cc3bee2faf57205cef8db8bfa608aa81.png) , i.e.,
, i.e.,  is stably complex.
is stably complex.
- If
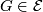 , then
, then ![[\tau_F]\in\text{Tor}(\widetilde{K}O(F))](/images/math/1/1/e/11ee4e1ffb1cd1915438a4358ed01567.png) .
.
- If
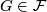 , then
, then ![[\tau_F]\in r_{\mathbb{C}}(\text{Tor}(\widetilde{K}U(F)))](/images/math/5/5/0/550a8cfd8ba25a21f01c2b556a0d39a1.png) .
.
3 References
- [Bredon1972] G. E. Bredon, Introduction to compact transformation groups, Academic Press, New York, 1972. MR0413144 (54 #1265) Zbl 0484.57001
- [Floyd&Richardson1959] E. E. Floyd and R. W. Richardson, An action of a finite group on an
 -cell without stationary points. , Bull. Amer. Math. Soc. 65 (1959), 73–76. MR0100848 (20 #7276) Zbl 0088.15302
-cell without stationary points. , Bull. Amer. Math. Soc. 65 (1959), 73–76. MR0100848 (20 #7276) Zbl 0088.15302
- [Greever1960] J. Greever, Stationary points for finite transformation groups, Duke Math. J 27 (1960), 163–170. MR0110094 (22 #977) Zbl 0113.16505
- [Laitinen&Morimoto1998] E. Laitinen and M. Morimoto, Finite groups with smooth one fixed point actions on spheres, Forum Math. 10 (1998), no.4, 479–520. MR1631012 (99k:57078) Zbl 0905.57023
- [Oliver1975] R. Oliver, Fixed-point sets of group actions on finite acyclic complexes, Comment. Math. Helv. 50 (1975), 155–177. MR0375361 (51 #11556) Zbl 0304.57020
- [Oliver1976] R. Oliver, Smooth compact Lie group actions on disks, Math. Z. 149 (1976), no.1, 79–96. MR0423390 (54 #11369) Zbl 0334.57023
- [Oliver1977] R. Oliver,
 -actions on disks and permutation representations. II, Math. Z. 157 (1977), no.3, 237–263. MR0646085 (58 #31126) Zbl 0386.20002
-actions on disks and permutation representations. II, Math. Z. 157 (1977), no.3, 237–263. MR0646085 (58 #31126) Zbl 0386.20002
- [Oliver1978] R. Oliver,
 -actions on disks and permutation representations, J. Algebra 50 (1978), no.1, 44–62. MR0501044 (58 #18508) Zbl 0386.20002
-actions on disks and permutation representations, J. Algebra 50 (1978), no.1, 44–62. MR0501044 (58 #18508) Zbl 0386.20002
- [Oliver1996] B. Oliver, Fixed point sets and tangent bundles of actions on disks and Euclidean spaces, Topology 35 (1996), no.3, 583–615. MR1396768 (97g:57059) Zbl 0861.57047