Talk:5-manifolds: 1-connected
From Manifold Atlas
(Difference between revisions)
m |
m (→Conjecture about mapping class groups of 1-connected 5-manifolds) |
||
| Line 3: | Line 3: | ||
Let $M$ be a closed, smooth, 1-connected 5-manifold, [[User:Matthias Kreck|Matthias Kreck]] and [[User:Diarmuid Crowley|Diarmuid Crowley]] conjecture that there is an isomorphism of abelian groups | Let $M$ be a closed, smooth, 1-connected 5-manifold, [[User:Matthias Kreck|Matthias Kreck]] and [[User:Diarmuid Crowley|Diarmuid Crowley]] conjecture that there is an isomorphism of abelian groups | ||
$$ \pi_0(\SDiff(M)) \cong \Omega_6(B^2(M)) $$ | $$ \pi_0(\SDiff(M)) \cong \Omega_6(B^2(M)) $$ | ||
| − | where $ | + | where $B^2(M)$ is the normal $2$-type of $M$ as defined in {{cite|Kreck1999}}. For example, if $M$ is Spinable with $H_2(M) \cong H$ then |
$$ \Omega_6(B^2(M)) \cong \Omega_6^{\Spin}(K(H, 2)).$$ | $$ \Omega_6(B^2(M)) \cong \Omega_6^{\Spin}(K(H, 2)).$$ | ||
At present we are checking the details of the proof of this conjecture using the methods of {{cite|Kreck1999}}. | At present we are checking the details of the proof of this conjecture using the methods of {{cite|Kreck1999}}. | ||
Latest revision as of 19:05, 16 December 2010
[edit] 1 Conjecture about mapping class groups of 1-connected 5-manifolds
Let  be a closed, smooth, 1-connected 5-manifold, Matthias Kreck and Diarmuid Crowley conjecture that there is an isomorphism of abelian groups
be a closed, smooth, 1-connected 5-manifold, Matthias Kreck and Diarmuid Crowley conjecture that there is an isomorphism of abelian groups

where 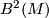 is the normal
is the normal  -type of
-type of  as defined in [Kreck1999]. For example, if
as defined in [Kreck1999]. For example, if  is Spinable with
is Spinable with 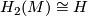 then
then
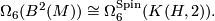
At present we are checking the details of the proof of this conjecture using the methods of [Kreck1999].
Diarmuid Crowley 10:02, 29 September 2009 (UTC)
[edit] 2 Earlier work of Fang
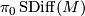 was computed in [Fang1993] provided that
was computed in [Fang1993] provided that Tex syntax errorhas no
 -torsion and no
-torsion and no  -torsion.
-torsion.
[edit] Up-date of conjecture: module structure
If the conjecture above holds, then from the short exact sequence
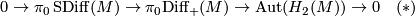
we obtain an action of 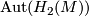 on the abelian group
on the abelian group 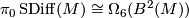 . Diarmuid Crowley and Matthias Kreck also conjecture that the action of
. Diarmuid Crowley and Matthias Kreck also conjecture that the action of 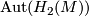 is via the induced action on
is via the induced action on 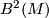 . In particular, if
. In particular, if  is spinable with
is spinable with 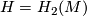 , then
, then 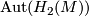 acts on
acts on 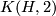 in the obvious way and so on
in the obvious way and so on 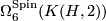 .
.