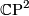Stable classification of 4-manifolds
| Line 36: | Line 36: | ||
<wikitex>; | <wikitex>; | ||
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected $4$-manifold is $\ge 2$ and it is $2$ if and only if $M$ is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least $3$, the Euler characteristic of $\mathbb {CP}^2$. By connected sum with copies of $\mathbb {CP}^2$ we can achieve all values $\ge 3$. | Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected $4$-manifold is $\ge 2$ and it is $2$ if and only if $M$ is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least $3$, the Euler characteristic of $\mathbb {CP}^2$. By connected sum with copies of $\mathbb {CP}^2$ we can achieve all values $\ge 3$. | ||
| + | |||
| + | |||
| + | If the Euler characteristic of a simply connected closed $4$-manifold is $k$, the second Betti number is $b=k-2$ and so the possible values of the signature are $-k+2 \le s \le k-2$ with $s = k mod 2$, since the Euler characteristic and the signature agree mod $2$. For given $b$ these values $s$ are realized by $\sharp _{(s+b )/2 }\mathbb {CP}^2 \sharp_ {(s+b)2-s} (-\mathbb { CP}^2$. | ||
Revision as of 17:52, 31 March 2011
|
The user responsible for this page is Matthias Kreck. No other user may edit this page at present. |
|
This page has not been refereed. The information given here might be incomplete or provisional. |
Contents |
1 Introduction
In this page we report about the stable classification of closed oriented  -manifolds. We will begin with a special class of closed oriented
-manifolds. We will begin with a special class of closed oriented  -manifolds, namely those, where the universal covering is not spinnable.
-manifolds, namely those, where the universal covering is not spinnable.
2 Construction and examples I
We begin with the construction of two classes of manifolds which can be used to give many stable diffeomorphism types of non-spinnable  -manifolds. The first is:
-manifolds. The first is:
The second is a large class of manifolds associated to certain algebraic data.
Let  be a finitely presentable group. Then for each element
be a finitely presentable group. Then for each element  in
in 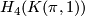 there is a smooth, closed, connected, oriented, non-spinnable manifold
there is a smooth, closed, connected, oriented, non-spinnable manifold  with signature zero, fundamental group
with signature zero, fundamental group  and
and ![u_*([M]) = \alpha](/images/math/a/2/1/a2132e46dc400eefcf637f8aa6b6ff65.png) . This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree
. This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree  ,
,  and
and  : see Oriented bordism to show that there is a closed, smooth, oriented manifold
: see Oriented bordism to show that there is a closed, smooth, oriented manifold  together with a map
together with a map 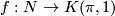 with
with ![f_*([M]) = \alpha](/images/math/8/a/a/8aa0b2b33192cb54dda92fbb8dd7c2b0.png) and signature zero. Then by surgeries on
and signature zero. Then by surgeries on  - and
- and  -dimensional spheres one changes
-dimensional spheres one changes  and
and  in such a way, that
in such a way, that  is connected and
is connected and  is an isomorphism on
is an isomorphism on 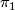 (reference). Finally we form the connected sum with
(reference). Finally we form the connected sum with  to make sure that
to make sure that  is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
3 Invariants
The following is a complete list of invariants for the stable classification of closed, smooth oriented  -manifolds whose universal covering is not spinnable:
-manifolds whose universal covering is not spinnable:
- The Euler characteristic
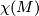
- The signature
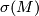
- The fundamanetal group
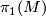
- The image of the fundamental class
![[u_*([M])]\in H_4(K(\pi_1(M),1)/Out(\pi_1(M))](/images/math/2/7/a/27a7f07e253ddaae67da2599a1a37142.png) of
of  .
.
Here 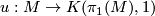 is a classifying map of the universal covering and
is a classifying map of the universal covering and 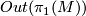 is the outer automorphism group which acts on the homology of
is the outer automorphism group which acts on the homology of 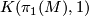 .
.
4 Classification
Theorem 4.1. Let  and
and  be
be  -dimensional compact smooth manifolds with non-spinnable universal covering. Then
-dimensional compact smooth manifolds with non-spinnable universal covering. Then  and
and  are stably diffeomorphic if and only if the invariants above agree.
are stably diffeomorphic if and only if the invariants above agree.
The proof of this result is an easy consequence of the general stable classification theorem ([Kreck1999], Stable classification of manifolds). Namely, the normal  -type is
-type is 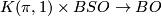 , see Stable classification of manfifolds. Thus the
, see Stable classification of manfifolds. Thus the  -bordism group is
-bordism group is 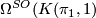 , which by the Atiyah-Hirzebruch spectral sequence is ismorphic to
, which by the Atiyah-Hirzebruch spectral sequence is ismorphic to 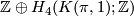 under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
5 Realization of the invariants
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected  -manifold is
-manifold is 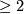 and it is
and it is  if and only if
if and only if  is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least
is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least  , the Euler characteristic of
, the Euler characteristic of 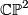 . By connected sum with copies of
. By connected sum with copies of 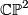 we can achieve all values
we can achieve all values 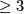 .
.
If the Euler characteristic of a simply connected closed  -manifold is
-manifold is  , the second Betti number is
, the second Betti number is 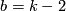 and so the possible values of the signature are
and so the possible values of the signature are 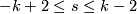 with
with 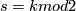 , since the Euler characteristic and the signature agree mod
, since the Euler characteristic and the signature agree mod  . For given
. For given  these values
these values  are realized by
are realized by 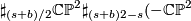 .
.
6 Further discussion
...
7 References
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
 -manifolds, namely those, where the universal covering is not spinnable.
-manifolds, namely those, where the universal covering is not spinnable.
2 Construction and examples I
We begin with the construction of two classes of manifolds which can be used to give many stable diffeomorphism types of non-spinnable  -manifolds. The first is:
-manifolds. The first is:
The second is a large class of manifolds associated to certain algebraic data.
Let  be a finitely presentable group. Then for each element
be a finitely presentable group. Then for each element  in
in 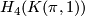 there is a smooth, closed, connected, oriented, non-spinnable manifold
there is a smooth, closed, connected, oriented, non-spinnable manifold  with signature zero, fundamental group
with signature zero, fundamental group  and
and ![u_*([M]) = \alpha](/images/math/a/2/1/a2132e46dc400eefcf637f8aa6b6ff65.png) . This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree
. This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree  ,
,  and
and  : see Oriented bordism to show that there is a closed, smooth, oriented manifold
: see Oriented bordism to show that there is a closed, smooth, oriented manifold  together with a map
together with a map 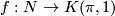 with
with ![f_*([M]) = \alpha](/images/math/8/a/a/8aa0b2b33192cb54dda92fbb8dd7c2b0.png) and signature zero. Then by surgeries on
and signature zero. Then by surgeries on  - and
- and  -dimensional spheres one changes
-dimensional spheres one changes  and
and  in such a way, that
in such a way, that  is connected and
is connected and  is an isomorphism on
is an isomorphism on 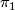 (reference). Finally we form the connected sum with
(reference). Finally we form the connected sum with  to make sure that
to make sure that  is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
3 Invariants
The following is a complete list of invariants for the stable classification of closed, smooth oriented  -manifolds whose universal covering is not spinnable:
-manifolds whose universal covering is not spinnable:
- The Euler characteristic
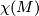
- The signature
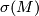
- The fundamanetal group
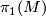
- The image of the fundamental class
![[u_*([M])]\in H_4(K(\pi_1(M),1)/Out(\pi_1(M))](/images/math/2/7/a/27a7f07e253ddaae67da2599a1a37142.png) of
of  .
.
Here 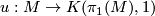 is a classifying map of the universal covering and
is a classifying map of the universal covering and 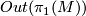 is the outer automorphism group which acts on the homology of
is the outer automorphism group which acts on the homology of 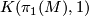 .
.
4 Classification
Theorem 4.1. Let  and
and  be
be  -dimensional compact smooth manifolds with non-spinnable universal covering. Then
-dimensional compact smooth manifolds with non-spinnable universal covering. Then  and
and  are stably diffeomorphic if and only if the invariants above agree.
are stably diffeomorphic if and only if the invariants above agree.
The proof of this result is an easy consequence of the general stable classification theorem ([Kreck1999], Stable classification of manifolds). Namely, the normal  -type is
-type is 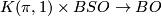 , see Stable classification of manfifolds. Thus the
, see Stable classification of manfifolds. Thus the  -bordism group is
-bordism group is 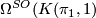 , which by the Atiyah-Hirzebruch spectral sequence is ismorphic to
, which by the Atiyah-Hirzebruch spectral sequence is ismorphic to 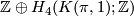 under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
5 Realization of the invariants
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected  -manifold is
-manifold is 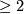 and it is
and it is  if and only if
if and only if  is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least
is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least  , the Euler characteristic of
, the Euler characteristic of 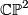 . By connected sum with copies of
. By connected sum with copies of 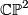 we can achieve all values
we can achieve all values 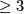 .
.
If the Euler characteristic of a simply connected closed  -manifold is
-manifold is  , the second Betti number is
, the second Betti number is 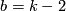 and so the possible values of the signature are
and so the possible values of the signature are 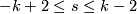 with
with 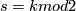 , since the Euler characteristic and the signature agree mod
, since the Euler characteristic and the signature agree mod  . For given
. For given  these values
these values  are realized by
are realized by 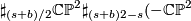 .
.
6 Further discussion
...
7 References
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
 -manifolds, namely those, where the universal covering is not spinnable.
-manifolds, namely those, where the universal covering is not spinnable.
2 Construction and examples I
We begin with the construction of two classes of manifolds which can be used to give many stable diffeomorphism types of non-spinnable  -manifolds. The first is:
-manifolds. The first is:
The second is a large class of manifolds associated to certain algebraic data.
Let  be a finitely presentable group. Then for each element
be a finitely presentable group. Then for each element  in
in 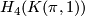 there is a smooth, closed, connected, oriented, non-spinnable manifold
there is a smooth, closed, connected, oriented, non-spinnable manifold  with signature zero, fundamental group
with signature zero, fundamental group  and
and ![u_*([M]) = \alpha](/images/math/a/2/1/a2132e46dc400eefcf637f8aa6b6ff65.png) . This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree
. This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree  ,
,  and
and  : see Oriented bordism to show that there is a closed, smooth, oriented manifold
: see Oriented bordism to show that there is a closed, smooth, oriented manifold  together with a map
together with a map 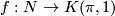 with
with ![f_*([M]) = \alpha](/images/math/8/a/a/8aa0b2b33192cb54dda92fbb8dd7c2b0.png) and signature zero. Then by surgeries on
and signature zero. Then by surgeries on  - and
- and  -dimensional spheres one changes
-dimensional spheres one changes  and
and  in such a way, that
in such a way, that  is connected and
is connected and  is an isomorphism on
is an isomorphism on 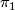 (reference). Finally we form the connected sum with
(reference). Finally we form the connected sum with  to make sure that
to make sure that  is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
3 Invariants
The following is a complete list of invariants for the stable classification of closed, smooth oriented  -manifolds whose universal covering is not spinnable:
-manifolds whose universal covering is not spinnable:
- The Euler characteristic
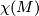
- The signature
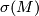
- The fundamanetal group
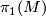
- The image of the fundamental class
![[u_*([M])]\in H_4(K(\pi_1(M),1)/Out(\pi_1(M))](/images/math/2/7/a/27a7f07e253ddaae67da2599a1a37142.png) of
of  .
.
Here 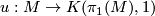 is a classifying map of the universal covering and
is a classifying map of the universal covering and 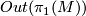 is the outer automorphism group which acts on the homology of
is the outer automorphism group which acts on the homology of 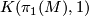 .
.
4 Classification
Theorem 4.1. Let  and
and  be
be  -dimensional compact smooth manifolds with non-spinnable universal covering. Then
-dimensional compact smooth manifolds with non-spinnable universal covering. Then  and
and  are stably diffeomorphic if and only if the invariants above agree.
are stably diffeomorphic if and only if the invariants above agree.
The proof of this result is an easy consequence of the general stable classification theorem ([Kreck1999], Stable classification of manifolds). Namely, the normal  -type is
-type is 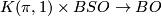 , see Stable classification of manfifolds. Thus the
, see Stable classification of manfifolds. Thus the  -bordism group is
-bordism group is 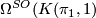 , which by the Atiyah-Hirzebruch spectral sequence is ismorphic to
, which by the Atiyah-Hirzebruch spectral sequence is ismorphic to 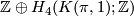 under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
5 Realization of the invariants
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected  -manifold is
-manifold is 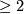 and it is
and it is  if and only if
if and only if  is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least
is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least  , the Euler characteristic of
, the Euler characteristic of 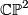 . By connected sum with copies of
. By connected sum with copies of 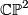 we can achieve all values
we can achieve all values 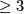 .
.
If the Euler characteristic of a simply connected closed  -manifold is
-manifold is  , the second Betti number is
, the second Betti number is 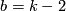 and so the possible values of the signature are
and so the possible values of the signature are 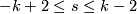 with
with 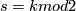 , since the Euler characteristic and the signature agree mod
, since the Euler characteristic and the signature agree mod  . For given
. For given  these values
these values  are realized by
are realized by 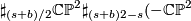 .
.
6 Further discussion
...
7 References
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
 -manifolds, namely those, where the universal covering is not spinnable.
-manifolds, namely those, where the universal covering is not spinnable.
2 Construction and examples I
We begin with the construction of two classes of manifolds which can be used to give many stable diffeomorphism types of non-spinnable  -manifolds. The first is:
-manifolds. The first is:
The second is a large class of manifolds associated to certain algebraic data.
Let  be a finitely presentable group. Then for each element
be a finitely presentable group. Then for each element  in
in 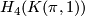 there is a smooth, closed, connected, oriented, non-spinnable manifold
there is a smooth, closed, connected, oriented, non-spinnable manifold  with signature zero, fundamental group
with signature zero, fundamental group  and
and ![u_*([M]) = \alpha](/images/math/a/2/1/a2132e46dc400eefcf637f8aa6b6ff65.png) . This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree
. This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree  ,
,  and
and  : see Oriented bordism to show that there is a closed, smooth, oriented manifold
: see Oriented bordism to show that there is a closed, smooth, oriented manifold  together with a map
together with a map 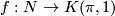 with
with ![f_*([M]) = \alpha](/images/math/8/a/a/8aa0b2b33192cb54dda92fbb8dd7c2b0.png) and signature zero. Then by surgeries on
and signature zero. Then by surgeries on  - and
- and  -dimensional spheres one changes
-dimensional spheres one changes  and
and  in such a way, that
in such a way, that  is connected and
is connected and  is an isomorphism on
is an isomorphism on 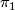 (reference). Finally we form the connected sum with
(reference). Finally we form the connected sum with  to make sure that
to make sure that  is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
3 Invariants
The following is a complete list of invariants for the stable classification of closed, smooth oriented  -manifolds whose universal covering is not spinnable:
-manifolds whose universal covering is not spinnable:
- The Euler characteristic
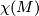
- The signature
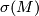
- The fundamanetal group
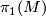
- The image of the fundamental class
![[u_*([M])]\in H_4(K(\pi_1(M),1)/Out(\pi_1(M))](/images/math/2/7/a/27a7f07e253ddaae67da2599a1a37142.png) of
of  .
.
Here 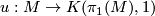 is a classifying map of the universal covering and
is a classifying map of the universal covering and 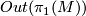 is the outer automorphism group which acts on the homology of
is the outer automorphism group which acts on the homology of 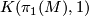 .
.
4 Classification
Theorem 4.1. Let  and
and  be
be  -dimensional compact smooth manifolds with non-spinnable universal covering. Then
-dimensional compact smooth manifolds with non-spinnable universal covering. Then  and
and  are stably diffeomorphic if and only if the invariants above agree.
are stably diffeomorphic if and only if the invariants above agree.
The proof of this result is an easy consequence of the general stable classification theorem ([Kreck1999], Stable classification of manifolds). Namely, the normal  -type is
-type is 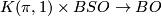 , see Stable classification of manfifolds. Thus the
, see Stable classification of manfifolds. Thus the  -bordism group is
-bordism group is 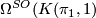 , which by the Atiyah-Hirzebruch spectral sequence is ismorphic to
, which by the Atiyah-Hirzebruch spectral sequence is ismorphic to 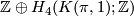 under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
5 Realization of the invariants
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected  -manifold is
-manifold is 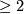 and it is
and it is  if and only if
if and only if  is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least
is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least  , the Euler characteristic of
, the Euler characteristic of 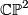 . By connected sum with copies of
. By connected sum with copies of 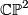 we can achieve all values
we can achieve all values 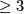 .
.
If the Euler characteristic of a simply connected closed  -manifold is
-manifold is  , the second Betti number is
, the second Betti number is 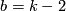 and so the possible values of the signature are
and so the possible values of the signature are 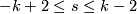 with
with 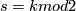 , since the Euler characteristic and the signature agree mod
, since the Euler characteristic and the signature agree mod  . For given
. For given  these values
these values  are realized by
are realized by 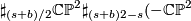 .
.
6 Further discussion
...
7 References
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039
 -manifolds, namely those, where the universal covering is not spinnable.
-manifolds, namely those, where the universal covering is not spinnable.
2 Construction and examples I
We begin with the construction of two classes of manifolds which can be used to give many stable diffeomorphism types of non-spinnable  -manifolds. The first is:
-manifolds. The first is:
The second is a large class of manifolds associated to certain algebraic data.
Let  be a finitely presentable group. Then for each element
be a finitely presentable group. Then for each element  in
in 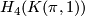 there is a smooth, closed, connected, oriented, non-spinnable manifold
there is a smooth, closed, connected, oriented, non-spinnable manifold  with signature zero, fundamental group
with signature zero, fundamental group  and
and ![u_*([M]) = \alpha](/images/math/a/2/1/a2132e46dc400eefcf637f8aa6b6ff65.png) . This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree
. This is proved in several steps by first using the Atiyah-Hirzebruch spectral sequence) and the fact that the oriented bordism groups are zero in degree  ,
,  and
and  : see Oriented bordism to show that there is a closed, smooth, oriented manifold
: see Oriented bordism to show that there is a closed, smooth, oriented manifold  together with a map
together with a map 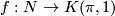 with
with ![f_*([M]) = \alpha](/images/math/8/a/a/8aa0b2b33192cb54dda92fbb8dd7c2b0.png) and signature zero. Then by surgeries on
and signature zero. Then by surgeries on  - and
- and  -dimensional spheres one changes
-dimensional spheres one changes  and
and  in such a way, that
in such a way, that  is connected and
is connected and  is an isomorphism on
is an isomorphism on 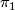 (reference). Finally we form the connected sum with
(reference). Finally we form the connected sum with  to make sure that
to make sure that  is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
is non-spinnable. This manifold is of course not unique but we will see that it is unique up to stable diffeomorphisms and we abbreviate it by
3 Invariants
The following is a complete list of invariants for the stable classification of closed, smooth oriented  -manifolds whose universal covering is not spinnable:
-manifolds whose universal covering is not spinnable:
- The Euler characteristic
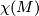
- The signature
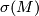
- The fundamanetal group
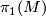
- The image of the fundamental class
![[u_*([M])]\in H_4(K(\pi_1(M),1)/Out(\pi_1(M))](/images/math/2/7/a/27a7f07e253ddaae67da2599a1a37142.png) of
of  .
.
Here 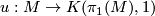 is a classifying map of the universal covering and
is a classifying map of the universal covering and 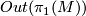 is the outer automorphism group which acts on the homology of
is the outer automorphism group which acts on the homology of 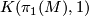 .
.
4 Classification
Theorem 4.1. Let  and
and  be
be  -dimensional compact smooth manifolds with non-spinnable universal covering. Then
-dimensional compact smooth manifolds with non-spinnable universal covering. Then  and
and  are stably diffeomorphic if and only if the invariants above agree.
are stably diffeomorphic if and only if the invariants above agree.
The proof of this result is an easy consequence of the general stable classification theorem ([Kreck1999], Stable classification of manifolds). Namely, the normal  -type is
-type is 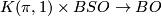 , see Stable classification of manfifolds. Thus the
, see Stable classification of manfifolds. Thus the  -bordism group is
-bordism group is 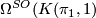 , which by the Atiyah-Hirzebruch spectral sequence is ismorphic to
, which by the Atiyah-Hirzebruch spectral sequence is ismorphic to 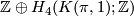 under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
under the signature and the image of the fundamental class. Now the statement follows from Theorem 3.1 of Stable classification of manifolds.
5 Realization of the invariants
Given the Theorem above one wonders about the realization of the invariants. Partial answers are easy, but in general this is a complicated open question, where the answer is only known for special fundamental groups. In the simply connected case there are only two invariants, the Euler characteristic and the signature. The Euler characteristic of of a simply connected  -manifold is
-manifold is 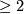 and it is
and it is  if and only if
if and only if  is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least
is a homotopy sphere. Since a homotopy sphere is spinnable it cannot occur in our context. Thus the Euler characteristic is at least  , the Euler characteristic of
, the Euler characteristic of 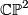 . By connected sum with copies of
. By connected sum with copies of 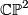 we can achieve all values
we can achieve all values 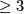 .
.
If the Euler characteristic of a simply connected closed  -manifold is
-manifold is  , the second Betti number is
, the second Betti number is 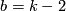 and so the possible values of the signature are
and so the possible values of the signature are 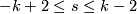 with
with 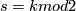 , since the Euler characteristic and the signature agree mod
, since the Euler characteristic and the signature agree mod  . For given
. For given  these values
these values  are realized by
are realized by 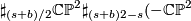 .
.
6 Further discussion
...
7 References
- [Kreck1999] M. Kreck, Surgery and duality, Ann. of Math. (2) 149 (1999), no.3, 707–754. MR1709301 (2001a:57051) Zbl 0935.57039